Bird J. Electrical Circuit Theory and Technology
Подождите немного. Документ загружается.

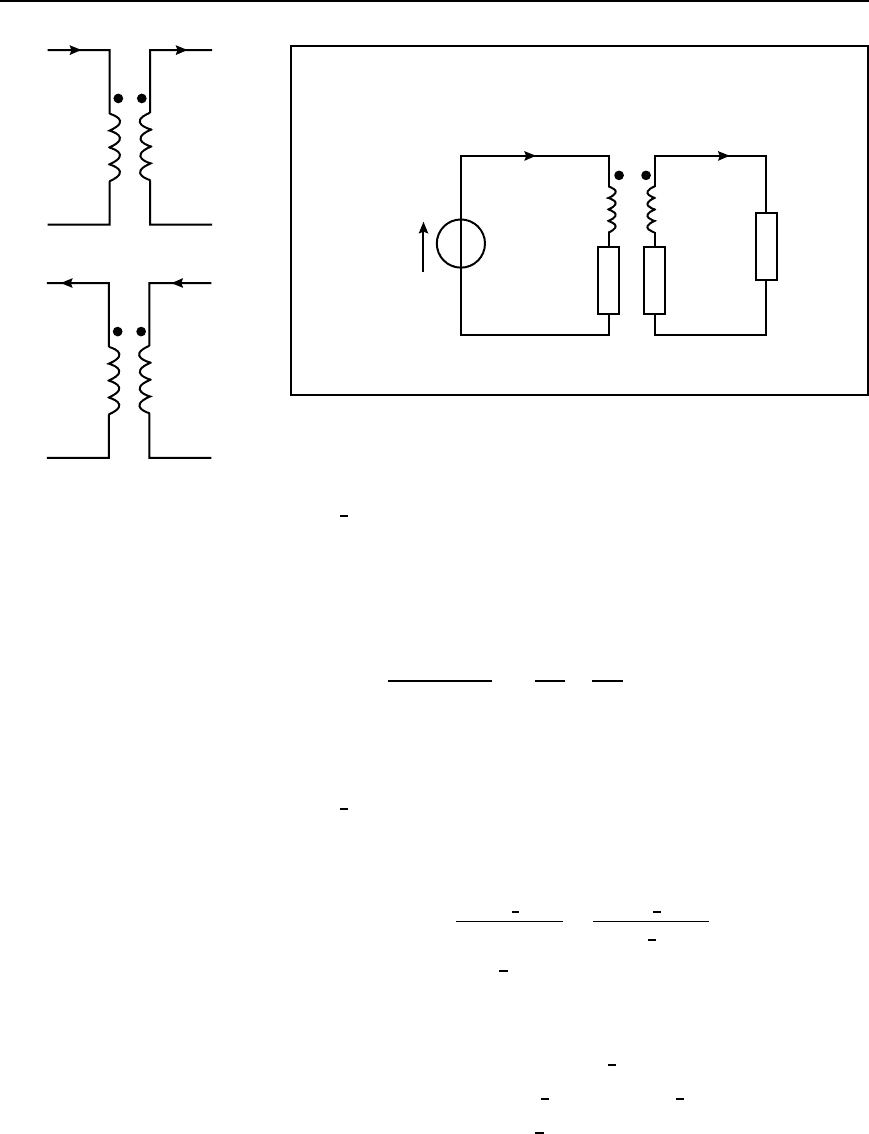
858 Electrical Circuit Theory and Technology
(a)
(b)
Figure 43.15
Problem 13. For the coupled circuit shown in Figure 43.16, deter-
mine the values of currents I
1
and I
2
I
1
I
2
M = j10 Ω
j50 Ω j50 Ω
10 Ω 10 Ω
50 Ω
250∠0° V
Figure 43.16
The position of the dots and the current directions correspond to
Figure 43.15(a), and hence the signs of the M and L terms are opposite.
Applying Kirchhoff’s voltage law to the primary circuit gives:
250
6
0
°
D 10 C j50I
1
j10I
2
1
and applying Kirchhoff’s voltage law to the secondary circuit gives:
0 D 10 C 50 C j50I
2
j10I
1
2
From equation (2), j10I
1
D 60 C j50I
2
and I
1
D
60 C j50I
2
j10
D
60
j10
C
j50
j10
I
2
D j6 C 5I
2
i.e., I
1
D 5 j6I
2
3
Substituting for I
1
in equation (1) gives:
250
6
0
°
D 10 C j505 j6I
2
j10I
2
D 50 j60 C j250 C 300 j10I
2
D 350 C j180I
2
from which, I
2
D
250
6
0
°
350 C j180
D
250
6
0
°
393.57
6
27.22
°
D 0.635
66
−27.22
°
A
From equation (3), I
1
D 5 j6I
2
D 5 j60.635
6
27.22
°
D 7.810
6
50.19
°
0.635
6
27.22
°
i.e., I
1
= 4.959
66
−77.41
°
A
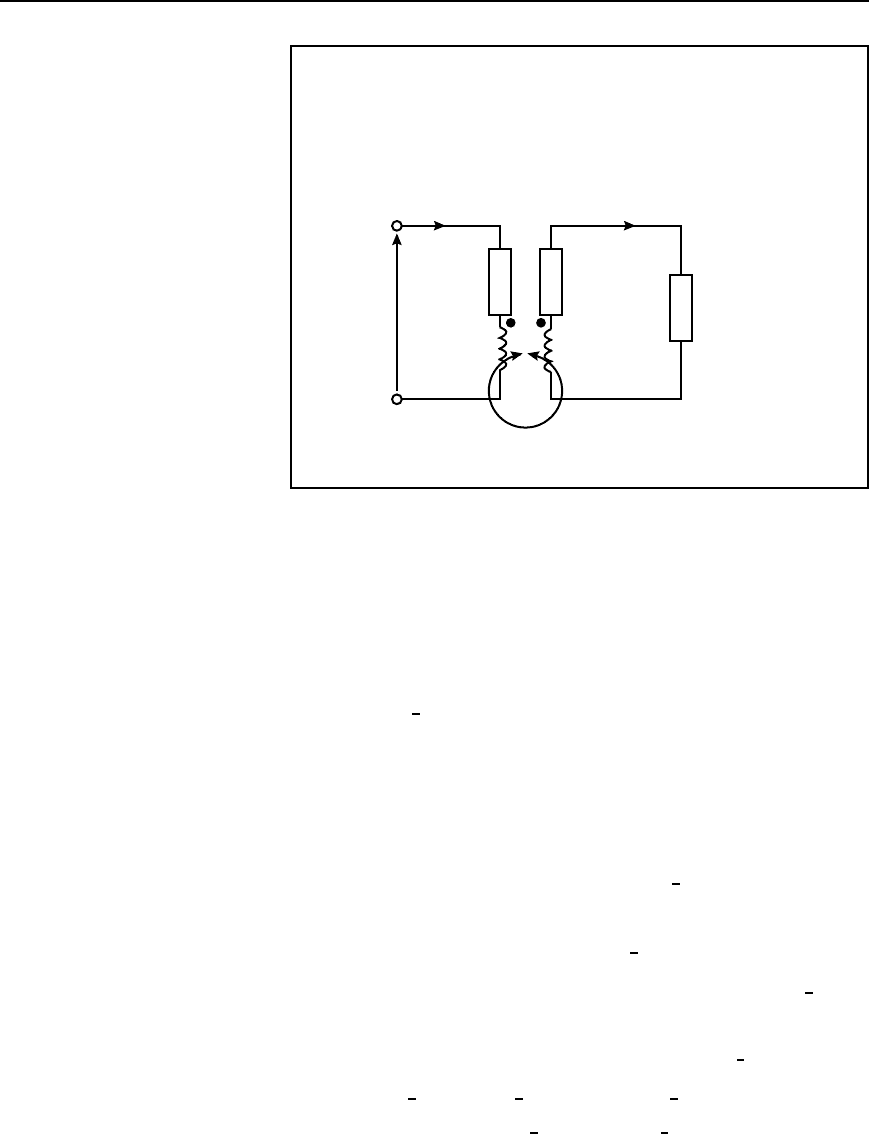
Magnetically coupled circuits 859
Problem 14. The circuit diagram of an air-cored transformer
winding is shown in Figure 43.17. The coefficient of coupling
between primary and secondary windings is 0.70. Determine for
the circuit (a) the mutual inductance M, (b) the primary current I
1
and (c) the secondary terminal p.d.
I
1
I
2
R
1
= 5 Ω R
2
= 40 Ω
L
1
=1 mH L
2
= 6 mH
M
40∠0° V
Z
L
=200∠−60° Ω
20 kHz
Figure 43.17
(a) From equation (43.9),
mutual inductance, M D k
p
L
1
L
2
D 0.70
[1 ð 10
3
6 ð 10
3
]
D 1.715 mH
(b) The two mesh equations are:
40
6
0
°
D R
1
C jωL
1
I
1
jωMI
2
1
and 0 D R
2
C jωL
2
C Z
L
I
2
jωMI
1
2
(Note that with the dots and current directions shown, the jωMI
terms are negative)
R
1
C jωL
1
D 5 Cj220 ð10
3
1 ð 10
3
D 5 C j125.66 or 125.76
6
87.72
°
jωM D j220 ð 10
3
1.715 ð 10
3
D j215.51 or 215.51
6
90
°
R
2
C jωL
2
C Z
L
D 40 C j220 ð 10
3
6 ð 10
3
C 200
6
60
°
D 40 C j753.98 C 100 j173.21
D 140 C j580.77 or 597.41
6
76.45
°
Hence 40
6
0
°
D 125.76
6
87.72
°
I
1
215.51
6
90
°
I
2
3
0 D215.51
6
90
°
I
1
C 597.41
6
76.45
°
I
2
4
860 Electrical Circuit Theory and Technology
From equation (4), I
2
D
215.51
6
90
°
597.41
6
76.45
°
I
1
D 0.361
6
13.55
°
I
1
5
Substituting for I
2
in equation (3) gives:
40
6
0
°
D 125.76
6
87.72
°
I
1
215.51
6
90
°
0.361
6
13.55
°
I
1
D I
1
125.76
6
87.72
°
77.80
6
103.55
°
D I
1
[5 C j125.66 18.23 C j75.63]
D I
1
23.23 j50.03
i.e., 40
6
0
°
D I
1
55.16
6
65.09
°
Hence primary current, I
1
D
40
6
0
°
55.16
6
65.09
°
D 0.725
66
65.09
°
A
(c) From equation (5), I
2
D 0.361
6
13.55
°
I
1
D 0.361
6
13.55
°
0.725
6
65.09
°
D 0.262
6
78.64
°
A
Hence secondary terminal p.d. D I
2
Z
L
D 0.262
6
78.64
°
200
6
60
°
D 52.4
66
18.64
°
V
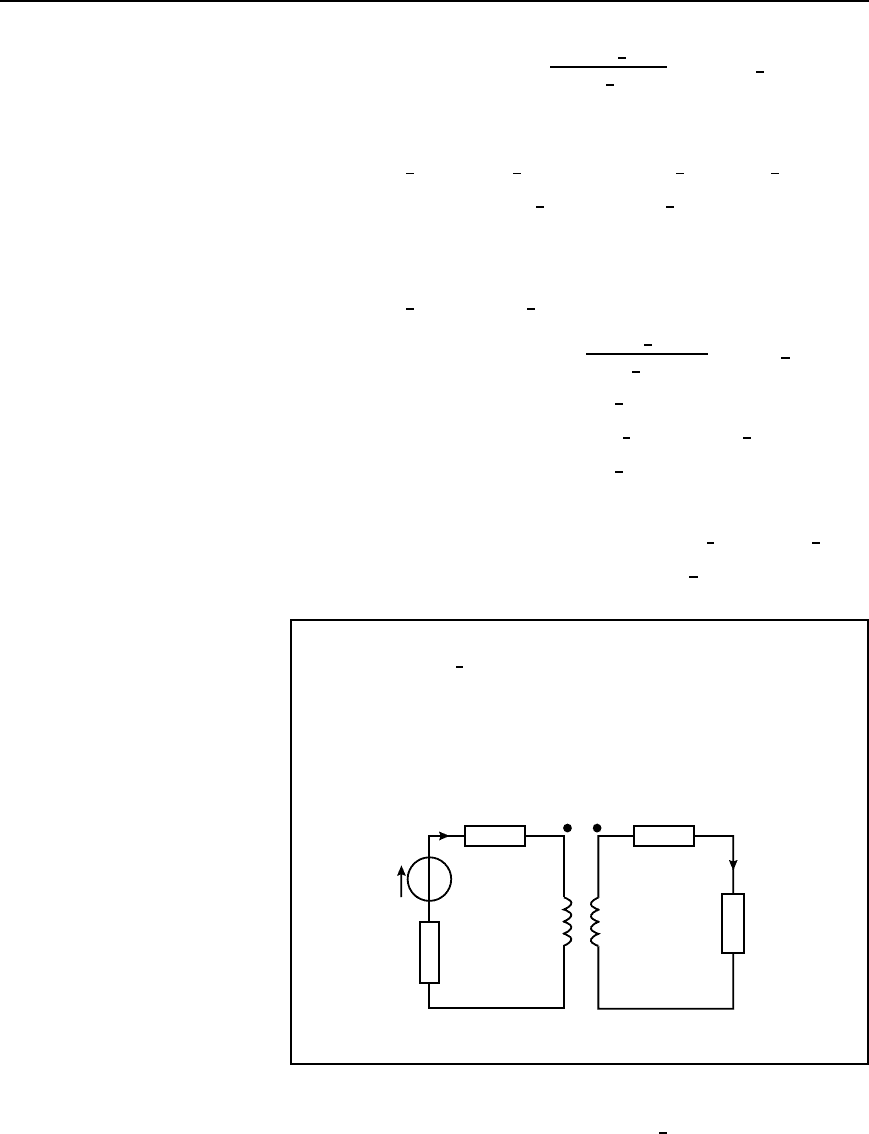
Problem 15. A mutual inductor is used to couple a 20 resis-
tive load to a 50
6
0
°
V generator as shown in Figure 43.18. The
generator has an internal resistance of 5 and the mutual inductor
parameters are R
1
D 20 , L
1
D 0.2H, R
2
D 25 , L
2
D 0.4H
and M D 0.1 H. The supply frequency is 75/ Hz. Determine
(a) the generator current I
1
and (b) the load current I
2
.
I
1
R
1
= 20 Ω R
2
= 25 Ω
I
2
E
1
=
50∠0° V
L
1
= 0.2 H
r = 5 Ω
M = 0.1 H
L
2
= 0.4 H
R
L
= 20 Ω
Figure 43.18
(a) Applying Kirchhoff’s voltage law to the primary winding gives:
I
1
r C R
1
C jωL
1
jωMI
2
D 50
6
0
°
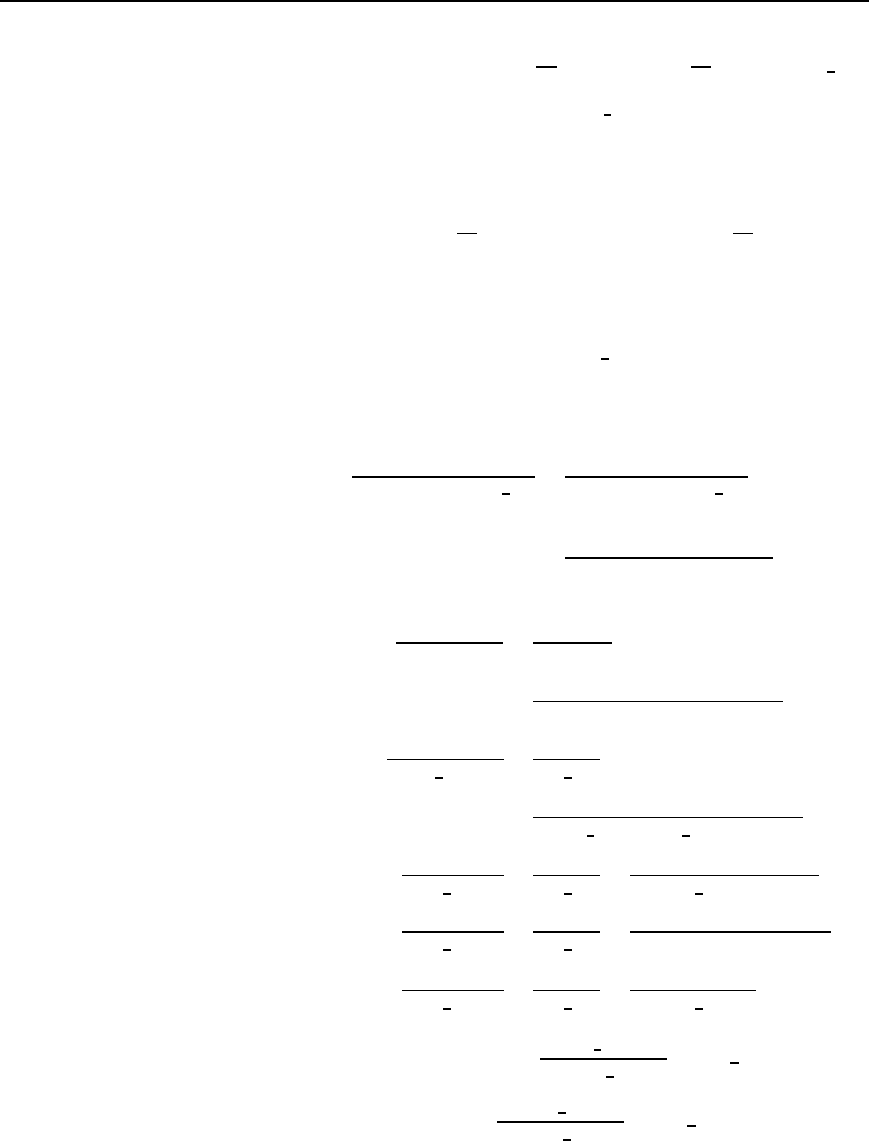
Magnetically coupled circuits 861
i.e., I
1
5 C 20 C j2
75
0.2
j2
75
0.1I
2
D 50
6
0
°
i.e., I
1
25 C j30 j15I
2
D 50
6
0
°
1
Applying Kirchhoff’s voltage law to the secondary winding gives:
jωMI
1
C I
2
R
2
C R
L
C jωL
2
D 0
i.e., j2
75
0.1I
1
C I
2
25 C 20 C j2
75
0.4
i.e., j15I
1
C I
2
45 C j60 D 0 2
Hence the equations to solve are:
25 C j30I
1
j15I
2
50
6
0
°
D 0 1
0
and j15I
1
C 45 C j60I
2
D 0 2
0
Using determinants:
I
1
j15 50
6
0
°
45 C j60 0
D
I
2
25 C j30 50
6
0
°
j15 0
D
1
25 C j30 j15
j15 45 C j60
i.e.,
I
1
5045 C j60
D
I
2
50j15
D
1
25 C j3045 C j60 j15
2
I
1
5075
6
53.13
°
D
I
2
750
6
90
°
D
1
39.05
6
50.19
°
75
6
53.13
°
C 225
I
1
3750
6
53.13
°
D
I
2
750
6
90
°
D
1
2928.75
6
103.32
°
C 225
I
1
3750
6
53.13
°
D
I
2
750
6
90
°
D
1
449.753 C j2849.962
I
1
3750
6
53.13
°
D
I
2
750
6
90
°
D
1
2885.23
6
98.97
°
(a) Generator current, I
1
D
3750
6
53.13
°
2885.23
6
98.97
°
D 1.30
66
−45.84
°
A
(b) Load current, I
2
D
750
6
90
°
2885.23
6
98.97
°
D 0.26
66
−8.97
°
A
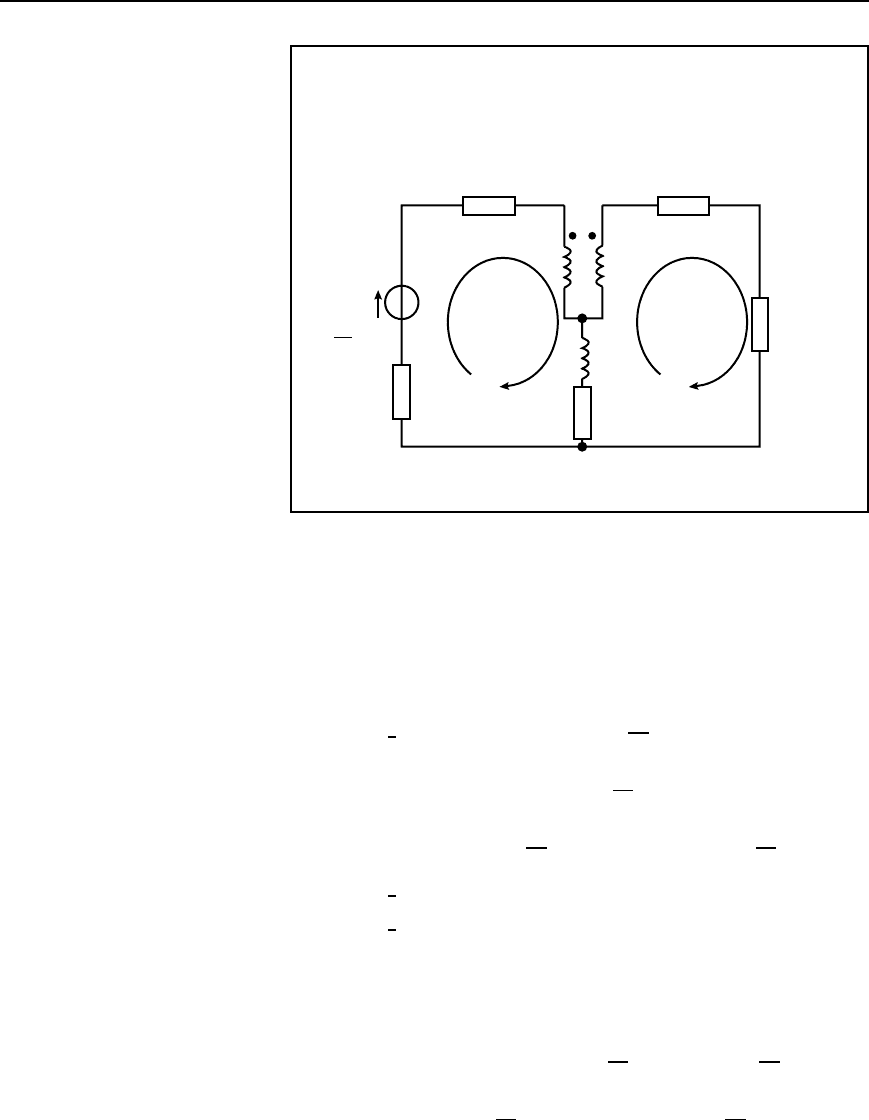
862 Electrical Circuit Theory and Technology
Problem 16. The mutual inductor of problem 15 is connected to
the circuit of Figure 43.19. Determine the source and load currents
for (a) the windings as shown (i.e. with the dots adjacent), and
(b) with one winding reversed (i.e. with the dots at opposite ends).
E
1
=
50∠0° V
f = 75 Hz
π
r = 5 Ω
R
1
= 20 Ω R
2
= 25 Ω
M = 0.1 H
L
1
= 0.2 H L
2
= 0.4 H
L = 0.1H
I
1
I
2
R = 8 Ω
R
L
= 20 Ω
Figure 43.19
(a) The left hand mesh equation in Figure 43.19 is:
E
1
D I
1
r C R
1
C R C jωL
1
C jωL jωMI
2
I
2
R C jωL
(Note that with the dots as shown in Figure 43.19, and the
chosen current directions as shown, the jωMI
2
is negative—see
Figure 43.15(a)). Hence
50
6
0
°
D I
1
5 C 20 C 8 Cj2
75
0.2
C j2
75
0.1
j2
75
0.1I
2
I
2
8 C j2
75
0.1
i.e., 50
6
0
°
D I
1
33 C j30 Cj15 j15I
2
I
2
8 C j15 (i)
i.e., 50
6
0
°
D 33 C j45I
1
8 Cj30I
2
1
The right hand mesh equation in Figure 43.19 is:
0 D I
2
R C R
2
C R
L
C jωL
2
C jωL jωMI
1
I
1
R C jωL
i.e., 0 D I
2
8 C 25 C 20 Cj2
75
0.4 C j2
75
0.1
j2
75
0.1I
1
I
1
8 C j2
75
0.1
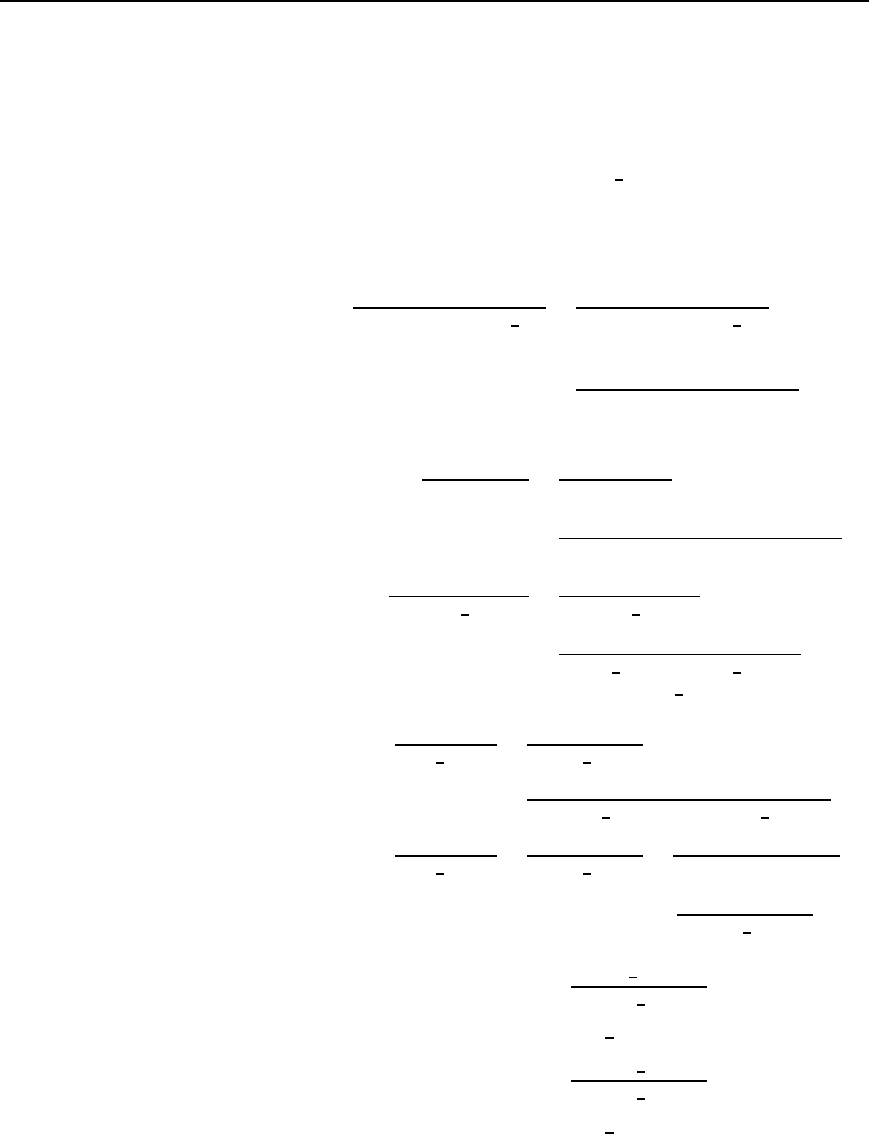
Magnetically coupled circuits 863
i.e., 0 D I
2
53 C j60 Cj15 j15I
1
I
1
8 C j15 (ii)
i.e., 0 D 53 C j75I
2
8 Cj30I
1
2
Hence the simultaneous equations to solve are:
33 C j45I
1
8 C j30I
2
50
6
0
°
D 0 1
8 C j30I
1
C 53 Cj75I
2
D 0 2
Using determinants gives:
I
1
8 C j30 50
6
0
°
53 C j75 0
D
I
2
33 C j45 50
6
0
°
8 C j30 0
D
1
33 C j45 8 C j30
8 C j3053 C j75
i.e.,
I
1
5053 C j75
D
I
2
508 C j30
D
1
33 C j4553 C j75 8 C j30
2
i.e.,
I
1
5091.84
6
54.75
°
D
I
2
5031.05
6
75.07
°
D
1
55.80
6
53.75
°
91.84
6
54.75
°
31.05
6
75.07
°
2
I
1
4592
6
54.75
°
D
I
2
1552.5
6
75.07
°
D
1
5124.672
6
108.50
°
964.103
6
150.14
°
I
1
4592
6
54.75
°
D
I
2
1552.5
6
75.07
°
D
1
789.97 Cj4379.84
D
1
4450.51
6
100.22
°
Hence source current, I
1
D
4592
6
54.75
°
4450.51
6
100.22
°
D 1.03
66
−45.47
°
A
and load current, I
2
D
1552.5
6
75.07
°
4450.51
6
100.22
°
D 0.35
66
−25.15
°
A

864 Electrical Circuit Theory and Technology
I
1
I
2
Figure 43.20
(b) When one of the windings of the mutual inductor is reversed, with,
say, the dots as shown in Figure 43.20, the jωMI terms change
sign, i.e., are positive. With both currents entering the dot ends of
the windings as shown, it compares with Figure 43.14(a), which
indicates that the ‘L’ and ‘M’ terms are of similar sign.
Thus equations (i) and (ii) of part (a) become:
50
6
0
°
D I
1
33 C j30 C j15 C j15I
2
I
2
8 C j15
and 0 D I
2
53 C j60 C j15 C j15I
1
I
1
8 C j15
i.e., I
1
33 C j45 I
2
8 50
6
0
°
D 0
and I
1
8 C I
2
53 C j75 D 0
Using determinants:
I
1
8 50
6
0
°
53 C j75 0
D
I
2
33 C j45 50
6
0
°
80
D
1
33 C j45 8
8 53 C j75
i.e.,
I
1
5053 C j75
D
I
2
400
6
0
°
D
1
33 C j4553 C j75 64
I
1
4592
6
54.75
°
D
I
2
400
6
0
°
D
1
5124.672
6
108.50
°
64
D
1
1690.08 C j4859.85
D
1
5145.34
6
109.18
°
Hence source current, I
1
D
4592
6
54.75
°
5145.34
6
109.18
°
D 0.89
66
−54.43
°
A
and load current, I
2
D
400
6
0
°
5145.34
6
109.18
°
D 0.078
66
−109.18
°
A
Further problems on the dot rule for coupled circuits may be found in
Section 43.8 following, problems 17 to 20, page 867.
43.8 Further problems
on magnetically coupled
circuits
Mutual inductance
1 If two coils have a mutual inductance of 500
µH, determine the
magnitude of the e.m.f. induced in one coil when the current in the
other coil varies at a rate of 20 ð 10
3
A/s [10 V]

Magnetically coupled circuits 865
2 An e.m.f. of 15 V is induced in a coil when the current in an adjacent
coil varies at a rate of 300 A/s. Calculate the value of the mutual
inductance of the two coils [50 mH]
3 Two circuits have a mutual inductance of 0.2 H. A current of 3 A
in the primary is reversed in 200 ms. Determine the e.m.f. induced
in the secondary, assuming the current changes at a uniform rate.
[6V]
4 A coil, x, has 1500 turns and a coil, y, situated close to x has
900 turns. When a current of 1 A flows in coil x a flux of 0.2 mWb
links with x and 0.65 of this flux links coil y. Determine (a) the
self inductance of coil x, and (b) the mutual inductance between the
coils. [(a) 0.30 H (b) 0.117 H]
Coefficient of coupling
5 Two coils have a mutual inductance of 0.24 H. If the coils have self
inductances of 0.4 H and 0.9 H respectively, determine the magnetic
coefficient of coupling. [0.40]
6 Coils A and B are magnetically coupled. Coil A has a self inductance
of 0.30 H and 300 turns, and coil B has a self inductance of 0.20 H
and 120 turns. A change of flux of 8 mWb occurs in coil B when
a current of 3 A is reversed in coil A. Determine (a) the mutual
inductance between the coils, and (b) the coefficient of coupling.
[(a) 0.16 H (b) 0.653]
Coils in series
7 Two coils have inductances of 50 mH and 100 mH respectively.
They are placed so that their mutual inductance is 10 mH. Determine
their effective inductance when the coils are (a) in series aiding (i.e.,
cumulatively coupled), and (b) in series opposing (i.e., differentially
coupled). [(a) 170 mH (b) 130 mH]
8 The total inductance of two coils connected in series is 0.1 H.
The coils have self inductance of 25 mH and 55 mH respectively.
Determine (a) the mutual inductance between the two coils, and
(b) the coefficient of coupling. [(a) 10 mH (b) 0.270]
9 A d.c. supply of 200 V is applied across two mutually coupled
coils in series, A and B. Coil A has a resistance of 2 and a
self inductance of 0.5 H; coil B has a resistance of 8 and a self
inductance of 2 H. At a certain instant after the circuit is switched
on, the current is 10 A and increasing a at rate of 25 A/s. Determine
(a) the mutual inductance between the coils, and (b) the coefficient
of coupling. [(a) 0.75 H (b) 0.75]
10 A ferromagnetic-cored coil is in two sections. One section has an
inductance of 750 mH and the other an inductance of 148 mH. The
coefficient of coupling is 0.6. Determine (a) the mutual inductance,
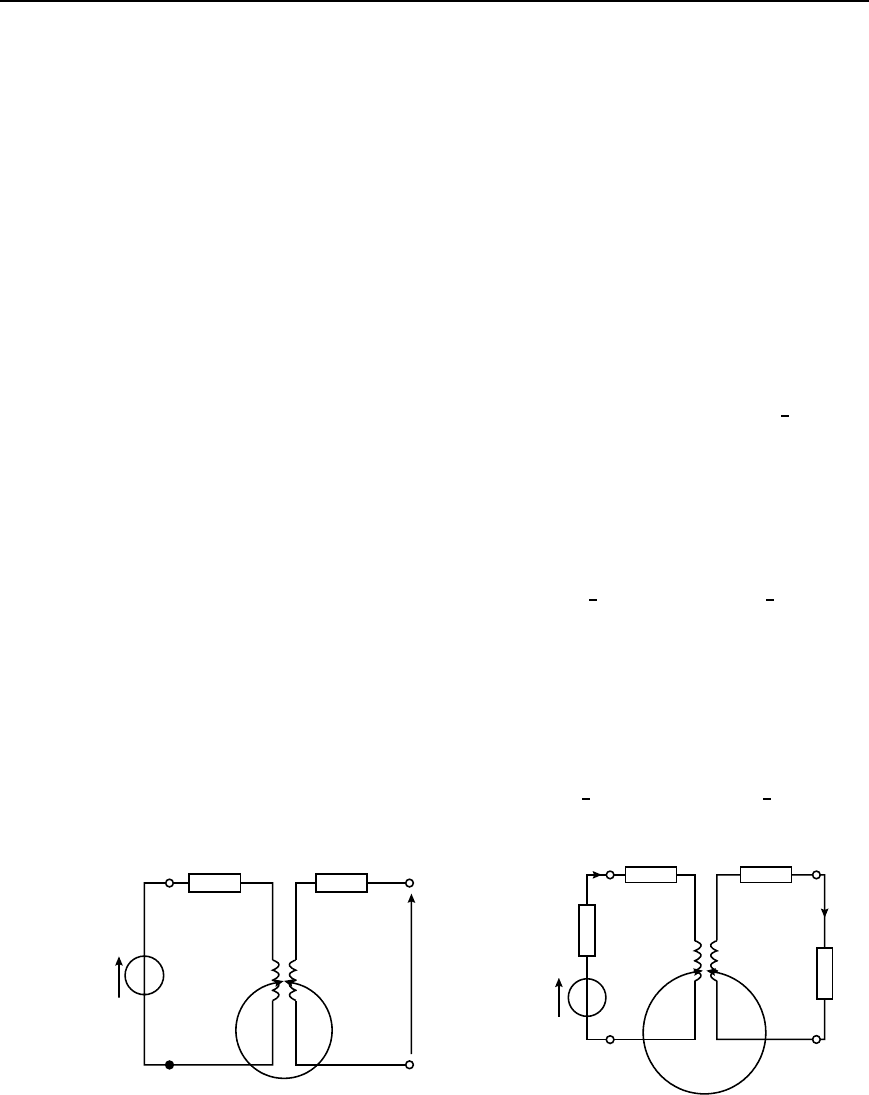
866 Electrical Circuit Theory and Technology
(b) the total inductance when the sections are connected in series
aiding, and (c) the total inductance when the sections are in series
opposing. [(a) 200 mH (b) 1.298 H (c) 0.498 H]
11 Two coils are connected in series and their total inductance is
measured as 0.12 H, and when the connection to one coil is reversed,
the total inductance is measured as 0.04 H. If the coefficient of
coupling is 0.8, determine (a) the self inductance of each coil, and
(b) the mutual inductance between the coils.
[(a) L
1
D 71.22 mH or 8.78 mH,
L
2
D 8.78 mH or 71.22 mH
(b)20mH]
Coupled circuits
12 Determine the value of voltage E
2
which appears across the open
circuited secondary winding of Figure 43.21. [0.93
6
68.20
°
V]
13 The coefficient of coupling between two coils having self inductances
of 0.5 H and 0.9 H respectively is 0.85. If a sinusoidal alternating
voltage of 50 mV is applied to the 0.5 H coil, determine the magni-
tude of the open circuit e.m.f. induced in the 0.9 H coil. [57 mV]
14 Determine the value of (a) the primary current, I
1
, and (b) the
secondary current I
2
, for the circuit shown in Figure 43.22.
[(a) 0.197
6
71.91
°
A (b) 0.030
6
48.48
°
A]
15 For the magnetically coupled circuit shown in Figure 43.23,
determine (a) the self impedance of the primary circuit, (b) the
self impedance of the secondary circuit, (c) the impedance reflected
into the primary circuit, (d) the effective primary impedance, (e) the
primary current, and (f) the secondary current.
[(a) 100 Cj200 (b) 40 C j80
(c) 40.5 j81.0 (d) 140.5 C j119
(e) 0.543
6
40.26
°
A (f) 0.546
6
13.69
°
A]
5 Ω 10 Ω
2 mH 3 mH E
2
E
1
=
10 sin 1000t volts
M = 0.5 mH
Figure 43.21
I
1
20 Ω 25 Ω
I
2
40 Ω
20 mH 30 mH
10 Ω
w = 5000 rad/s
20∠0° V
M = 5 mH
Figure 43.22
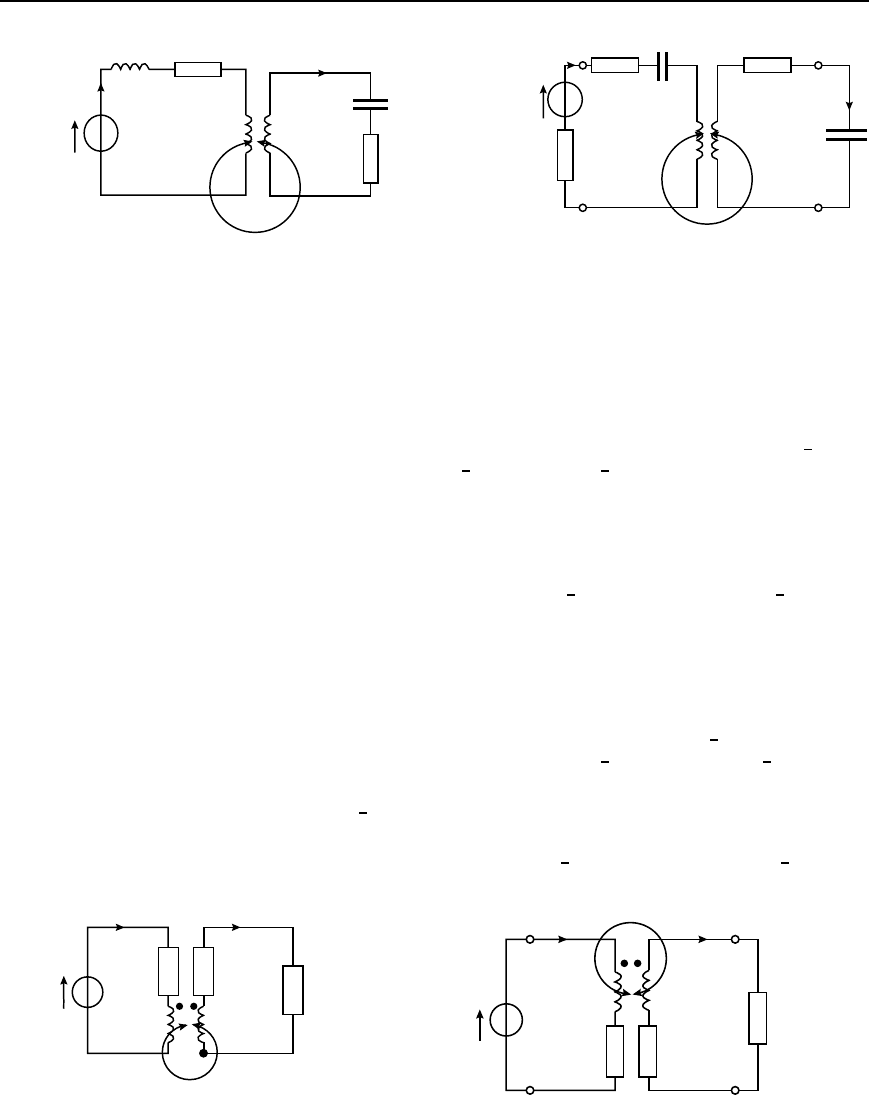
Magnetically coupled circuits 867
I
1
80 mH
100 Ω
120 mH 100 mH
I
2
50 µF
40 Ω
M = 90 mH
100∠0° V
w = 1000 rad/s
Figure 43.23
I
1
50 Ω
5 nF
80 Ω
I
2
C
S
25 mH 10 mH
10 Ω
M = 4 mH
30∠0° V
Figure 43.24
16 In the coupled circuit shown in Figure 43.24, each winding is
tuned to resonance at the same frequency. Calculate (a) the resonant
frequency, (b) the value of C
S
, (c) the effective primary impedance,
(d) the primary current, (e) the secondary current, (f) the p.d. across
capacitor C
S
, and (g) the coefficient of coupling.
[(a) 14.235 kHz (b) 12.5 nF (c) 1659.9 (d) 18.1
6
0
°
mA
(e)80.9
6
90
°
mA (f) 72.4
6
0
°
V (g) 0.253]
Dot rule for coupled circuits
17 Determine the values of currents I
p
and I
s
in the coupled circuit
shown in Figure 43.25.
[I
p
D 893.3
6
60.57
°
mA, I
s
D 99.88
6
2.86
°
mA]
18 The coefficient of coupling between the primary and secondary
windings for the air-cored transformer shown in Figure 43.26 is 0.84.
Calculate for the circuit (a) the mutual inductance M, (b) the primary
current I
p
, (c) the secondary current I
s
, and (d) the secondary
terminal p.d.
[(a) 13.28 mH(b) 1.603
6
28.98
°
A
(c) 0.913
6
17.70
°
A (d) 73.04
6
27.30
°
V]
19 A mutual inductor is used to couple a 50 resistive load to a
250
6
0
°
V generator as shown in Figure 43.27. Calculate (a) the
generator current I
g
and (b) the load current I
L
.
[(a) I
g
D 9.653
6
36.03
°
A(b)I
L
D 1.084
6
27.28
°
A]
I
p
I
s
5 Ω
15 Ω
j 10 Ω j 20 Ω
10∠0° V
M = j5 Ω
25 Ω
Figure 43.25
M
I
p
I
s
10 mH 25 mH
20 Ω 50 Ω
100∠0° V
1 kHz
Z
L
= 80∠−45° Ω
Figure 43.26
