Bird J. Electrical Circuit Theory and Technology

Подождите немного. Документ загружается.

728 Electrical Circuit Theory and Technology

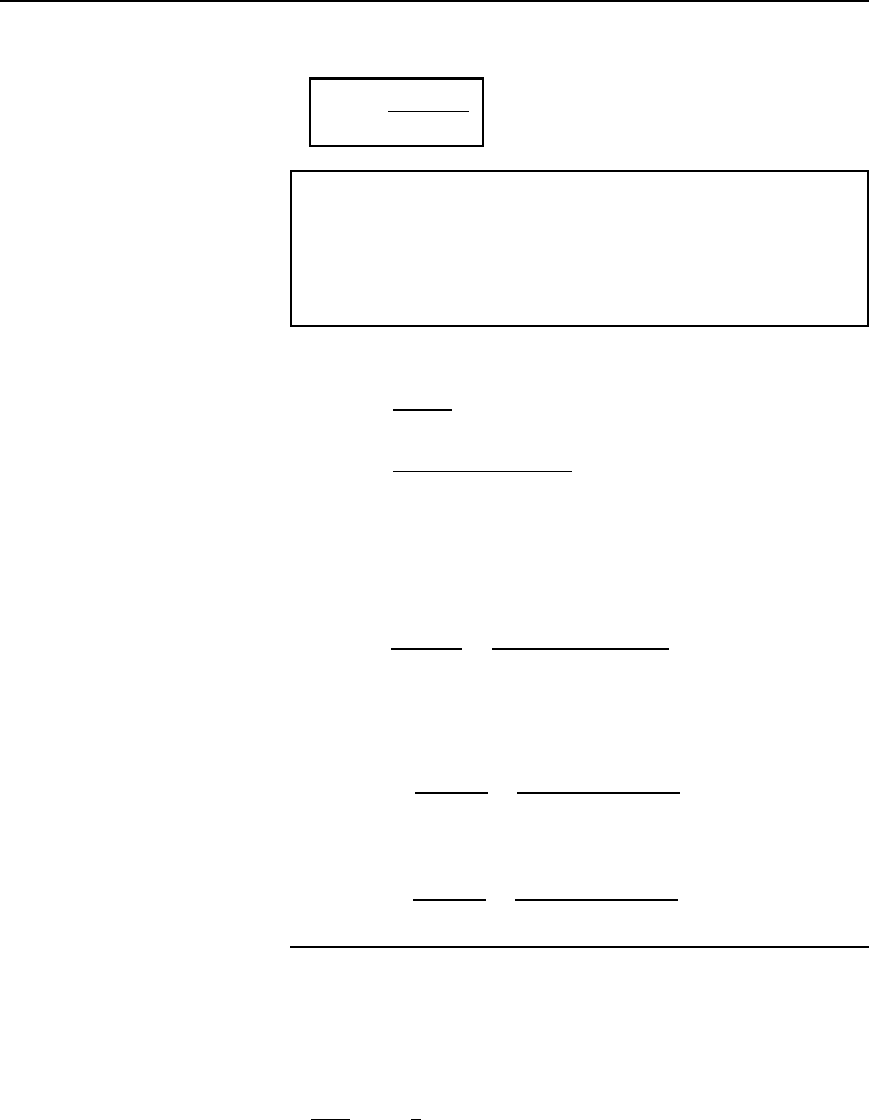
It follows that

min

b ln.b=a/

40.9



Problem 5. A concentric cable has a core diameter of 32 mm and

an inner sheath diameter of 80 mm. The core potential is 40 kV and

the relative permittivity of the dielectric is 3.5. Determine (a) the

capacitance per kilometre length of the cable, (b) the dielectric

stress at a radius of 30 mm, and (c) the maximum and minimum

values of dielectric stress.

(a) From equation (40.7), capacitance per metre length,

C D

2ε

lnb/a

28.85 ð 10

12

3.5

ln40/16

D 212.4 ð 10

12

F/m

D 212.4 ð 10

12

ð 10

F/km

D 212 nF=km or 0.212 mF=km

(b) From equation (40.8), dielectric stress at radius r,

E D

r lnb/a

40 ð 10

30 ð 10

3

 ln40/16

D 1.46

× 10

V=m or 1.46 MV=m

max

a lnb/a

40 ð 10

16 ð 10

3

ln40/16

D 2.73 MV=m

From equation (40.9

), minimum dielectric stress,

min

b lnb/a

40 ð 10

3

ln40/16

D 1.09 MV=m

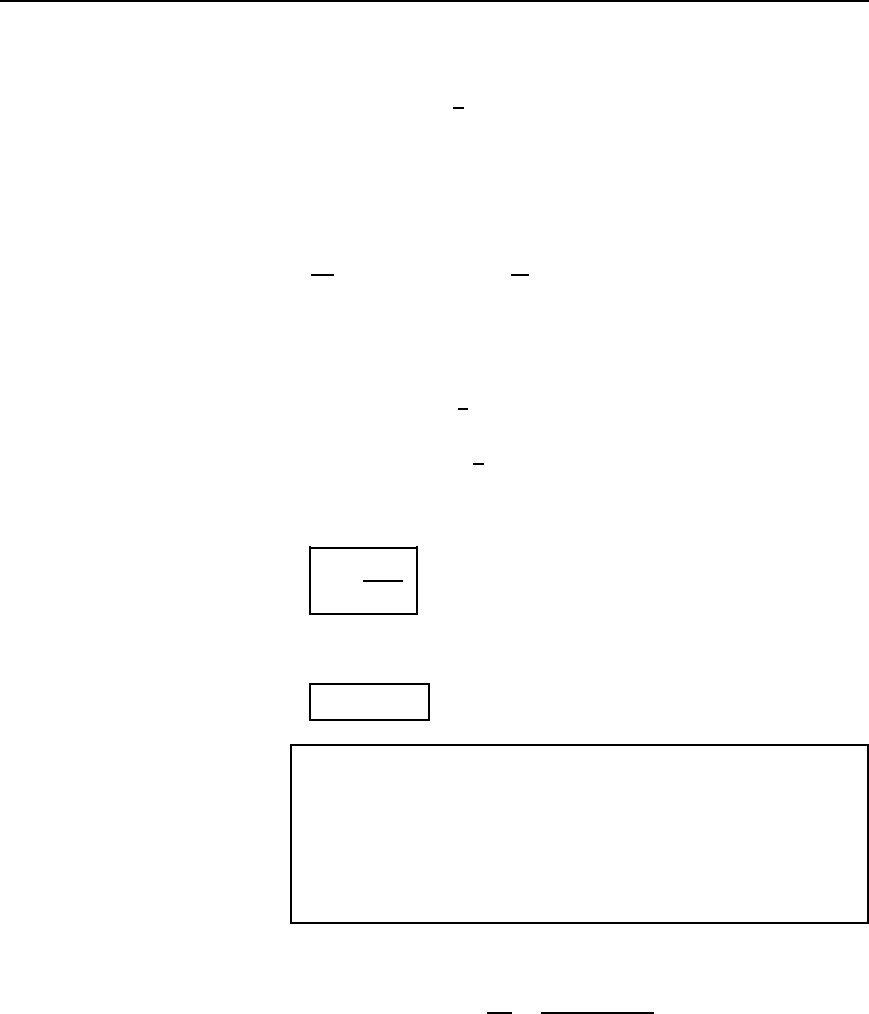
Dimensions of most economical cable

It is important to obtain the most economical dimensions when designing

a cable. A relationship between a and b may be obtained as follows. If

max

and V are both ﬁxed values, then, from equation (40.9),

max

D a ln

Field theory 729

Letting V/E

max

D k, a constant, gives

a ln

D k

from which lnb/a D k/a, b/a D e

k/a

and b D ae

k/a

40.10

For the most economical cable, b will be a minimum value. Using the

product rule of calculus,

D e

k/a

1 C a





k/a



D 0 for a minimum value.

(Note, to differentiate e

k/a

with respect to a, an algebraic substitution may

be used, letting u D 1/a).

k/a



k/a

D 0

Therefore e

k/a



1 



D 0

from which a D k. Thus

a =

max

40.11

From equation (40.10), internal sheath radius, b D ae

k/a

D ae

D ae, i.e.,

b = 2.718a

40.12

Problem 6. A single-core concentric cable is to be manufactured

for a 60 kV, 50 Hz transmission system. The dielectric used is

paper which has a maximum permissible safe dielectric stress of

10 MV/m rms and a relative permittivity of 3.5. Calculate (a) the

core and inner sheath radii for the most economical cable, (b) the

capacitance per metre length, and (c) the charging current per kilo-

metre run.

(a) From equation (40.11),

core radius, a D

60 ð 10

10 ð 10

V/m

D 6 ð 10

3

m D 6.0mm

From equation (40.12), internal sheath radius,

b D ae D 6.0e D 16.3mm

730 Electrical Circuit Theory and Technology

(b) From equation (40.7),

capacitance C D

2ε

lnb/a

F/m

Since b D ae,

C D

2ε

lne

D 2ε

D 28.85 ð 10

12

3.5

D 195

× 10

−12

F=m or 195 pF=m

1/ωC

D ωCV

D 250195 ð 10

12

60 ð 10



D 3.68 ð 10

3

A/m

Hence the charging current per kilometre D 3.68 A

Problem 7. A concentric cable has a core diameter of 25 mm and

an inside sheath diameter of 80 mm. The relative permittivity of the

dielectric is 2.5, the loss angle is 3.5 ð 10

3

rad and the working

voltage is 132 kV at 50 Hz frequency. Determine for a 1 km length

of the cable (a) the capacitance, (b) the charging current and (c) the

power loss.

(a) From equation (40.7),

capacitance, C D

2ε

lnb/a

F/m

28.85 ð 10

12

2.5

ln40/12.5

ð 10

F/km

D 0.120

µF/km

Thus the capacitance fo

ra1kmlength of the cable is

0.120 mF

(b) Charging current I D

1/ωC

D ωCV

D 2500.120 ð 10

6

132 ð 10



D 4.98 A=km

power loss D V

ωC tanυ

D 132 ð 10



2500.120 ð 10

6

 tan3.5 ð 10

3



D 2300 W

Field theory 731

θ rad

Line of

force

Equipotential

lines

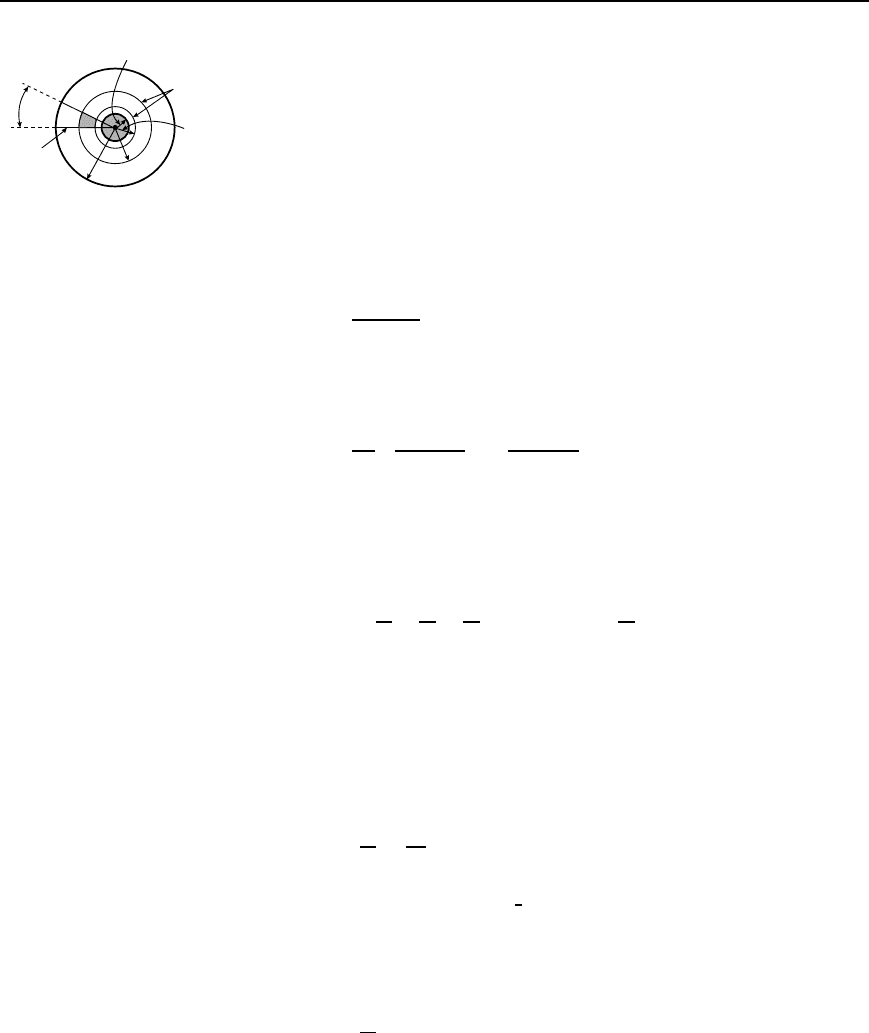
Figure 40.12

Concentric cable ﬁeld plotting

Figure 40.12 shows a cross-section of a concentric cable having a core

radius r

and a sheath radius r

. It was shown in Section 40.1 that the

capacitance of a true square is given by C D ε

farads/metre.

A curvilinear square is shown shaded in Figure 40.12. Such squares

can be made to have the same capacitance as a true square by the correct

choice of spacing between the lines of force and the equipotential surfaces

in the ﬁeld plot.

From equation (40.7), the capacitance between cylindrical equipotential

lines at radii r

and r

is given by

C D

2ε

lnr

a

farads/metre

Thus for a sector of ! radians (see Figure 40.12) the capacitance is

given by

C D

2



2ε

lnr





!ε

lnr



farads/metre

Now if ! D lnr

 then C D ε

F/m, the same as for a true square. If

! D lnr

, then e

D r

. Thus if, say, two equipotential surfaces

are chosen within the dielectric as shown in Figure 40.12, then e

D r

and e

D r

. Hence

e



, i.e., e

40.13

It follows that e

D r

Equation (40.13) is used to determine the value of ! and hence the

number of sectors. Thus, for a concentric cable having a core radius 8 mm

and inner sheath radius 32 mm, if two equipotential surfaces within the

dielectric are chosen (and therefore form three capacitors in series in each

sector).

D 4

Hence 3! D ln4 and ! D

ln4 D 0.462 rad (or 26.47

). Thus there

will be 2/0.462 D 13.6 sectors in the ﬁeld plot. (Alternatively,

360

/26.47

D 13.6 sectors.) From above,

D r

, i.e., r

D r

D 8e

20.462

D 20.15 mm

from which

D r

D 8e

0.462

D 12.70 mm

732 Electrical Circuit Theory and Technology

The ﬁeld plot is shown in Figure 40.13. The number of parallel squares

measured along each equipotential is 13.6 and the number of series

squares measured along each line of force is 3. Hence in equation (40.4),

where C D ε

xm/n, m D 13.6andn D 3.

If the dielectric has a relative permittivity of, say, 2.5, then the capacitance

per metre length,

C D 8.85 ð 10

12

2.51

13.6

D 100 pF

(From equation (40.7),

C D

2ε

lnr



F/m D

28.85 ð 10

12

2.5

ln32/8

D 100 F=m

Thus ﬁeld plotting using curvilinear squares provides an alternative

method of determining the capacitance between concentric cylinders.

Problem 8. A concentric cable has a core diameter of 20 mm and

a sheath inside diameter of 60 mm. The permittivity of the dielec-

tric is 3.2. Using three equipotential surfaces within the dielectric,

determine the capacitance of the cable per metre length by the

method of curvilinear squares. Draw the ﬁeld plot for the cable.

The ﬁeld plot consists of radial lines of force dividing the cable cross-

section into a number of sectors, the lines of force cutting the equipotential

surfaces at right angles. Since three equipotential surfaces are required in

the dielectric, four capacitors in series are found in each sector of !

radians.

In Figure 40.14, r

D 20/2 D 10 mm and r

D 60/2 D 30 mm. It

follows from equation (40.13) that e

D r

D 30/10 D 3, from which

4! D ln3 and ! D

ln3 D 0.2747 rad.

= 8 mm

= 12.70 mm

= 20.15 mm

= 32 mm

Figure 40.13

0.2747 rad

Figure 40.14

Field theory 733

Thus the number of sectors in the plot shown in Figure 40.14 is

2/0.2747 D 22.9.

The three equipotential lines are shown in Figure 40.14 at radii of r

and r

From equation (40.13),

, from which r

D r

D 10e

30.2747

D 22.80 mm

, from which r

D r

D 10e

20.2747

D 17.32 mm

, from which r

D r

D 10e

0.2747

D 13.16 mm

Thus the ﬁeld plot for the cable is as shown in Figure 40.14.

From equation (40.4), capacitance C D ε

xm/n. The number of

parallel squares along each equipotential, m D 22.9 and the number of

series squares measured along each line of force, n D 4. Thus

capacitance C D 8.85 ð10

12

3.21

22.9

D 162 pF

(Checking, from equation (40.7),

capacitance C D

2ε

lnr



28.85 ð 10

12

3.2

ln30/10

D 162 pF

Further problems on the capacitance between concentric cylinders may be

found in Section 40.9, problems 4 to 10, page 753.

40.3 Capacitance of an

isolated twin line

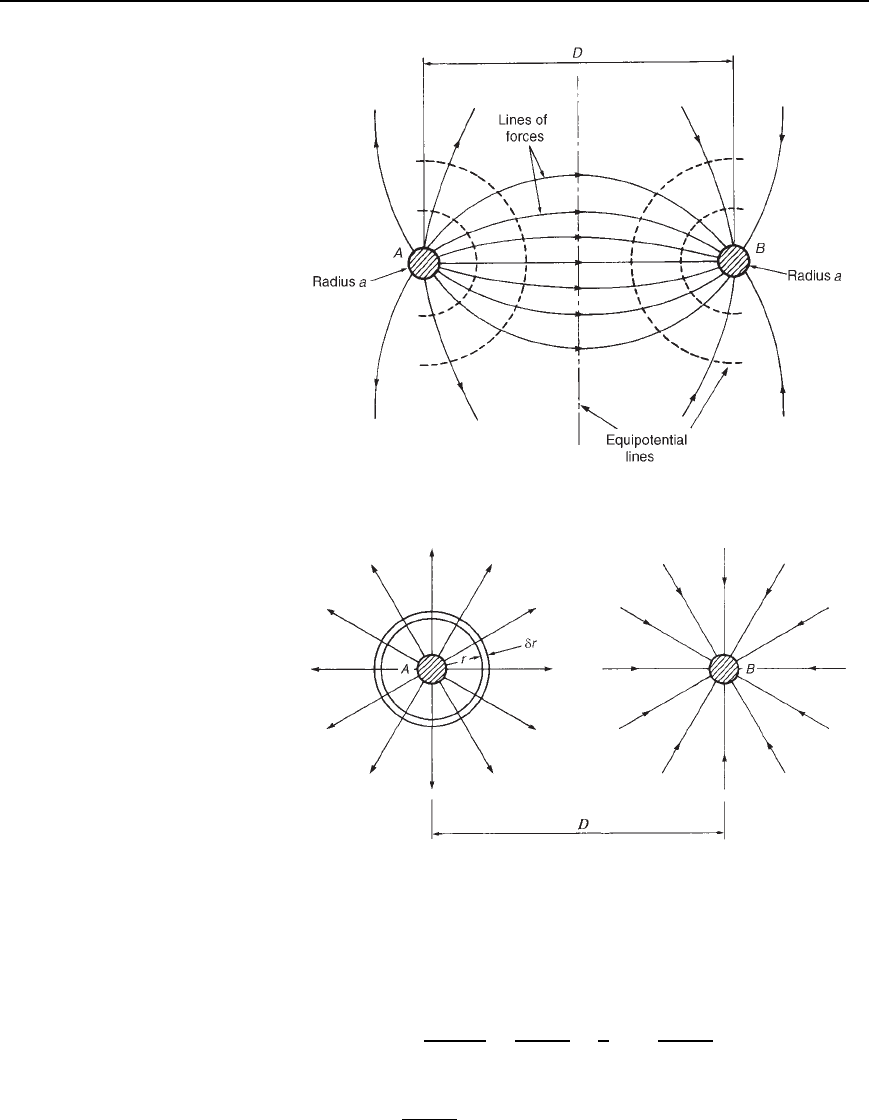
The ﬁeld distribution with two oppositely charged, long conductors, A and

B, each of radius a is shown in Figure 40.15. The distance D between

the centres of the two conductors is such that D is much greater than a.

Figure 40.16 shows the ﬁeld of each conductor separately.

Initially, let conductor A carry a charge of CQ coulombs per metre

while conductor B is uncharged. Consider a cylindrical element of radius r

about conductor A having a depth of 1 m and a thickness υr as shown in

Figure 40.16.

The electric ﬂux density D at the element (i.e. at radius r) is given by

D D

charge

area

2 ð 1

coulomb/metre

The electric ﬁeld strength at the element,

E D

Q/2r

2rε

volts/metre

Since E D V/d, potential difference, V D Ed. Thus

p.d. at the element D Eυr D

Qυr

2rε

volts

734 Electrical Circuit Theory and Technology

Figure 40.15

Figure 40.16

The potential may be considered as zero at a large distance from the

conductor. Let this be at radius R. Then the potential of conductor A

above zero, V

, is given by



Qdr

2rε

2ε



dr D

2ε

[lnr]

2ε

[lnR  lna]

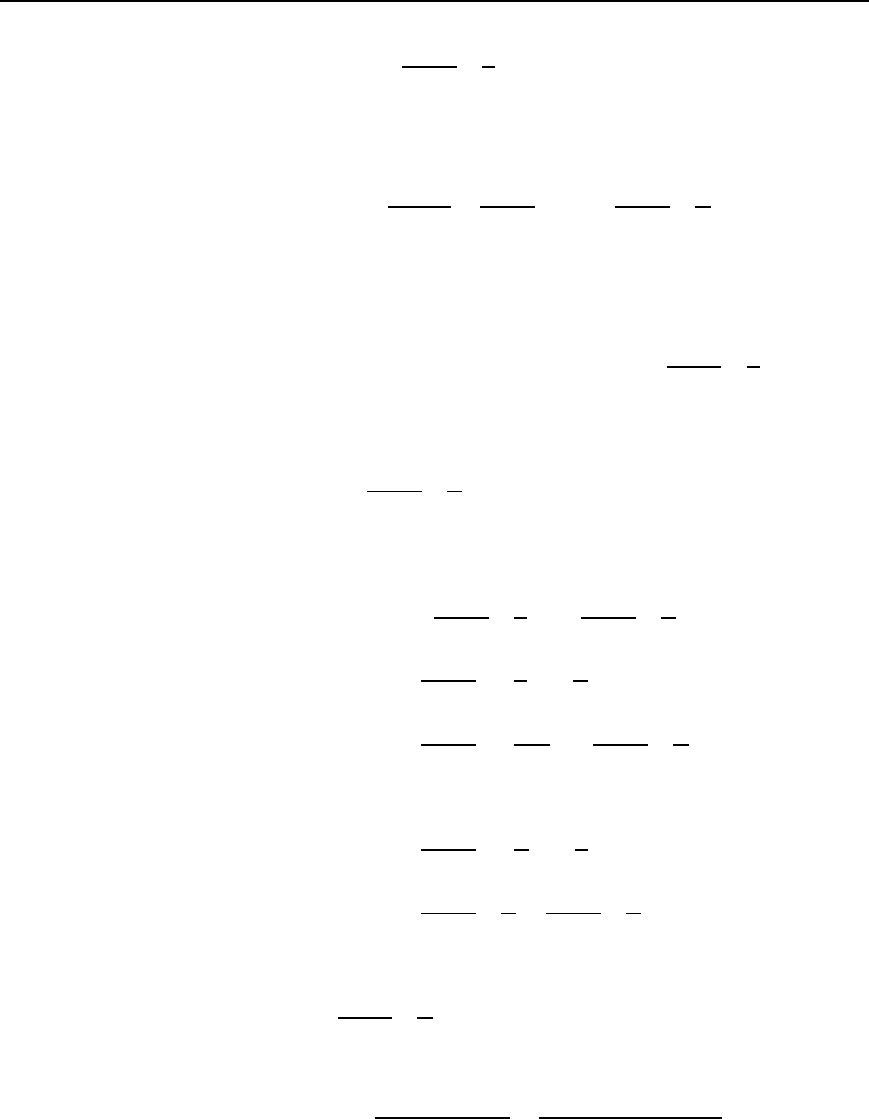
Field theory 735

i.e., V

2ε

Since conductor B lies in the ﬁeld of conductor A, by reasoning similar

to that above, the potential at conductor B above zero, V

, is given by



Qdr

2rε

2ε

[lnr]

2ε

Repeating the above procedure, this time assuming that conductor B

carries a charge of Q coulombs per metre, while conductor A is

uncharged, gives

potential of conductor B below zero, V

Q

2ε

and the potential of conductor A below zero, due to the charge on

conductor B,

Q

2ε

When both conductors carry equal and opposite charges, the total potential

of A above zero is given by

C V



2ε





Q

2ε



2ε



 ln



2ε



R/a

R/D



2ε

and the total potential of B below zero is given by

C V

2ε



 ln



2ε

Q

2ε

Hence the p.d. between A and B is



2ε



volts/metre

The capacitance between A and B per metre length,

C D

charge per metre

p.d.

2Q/2ε

 lnD/a

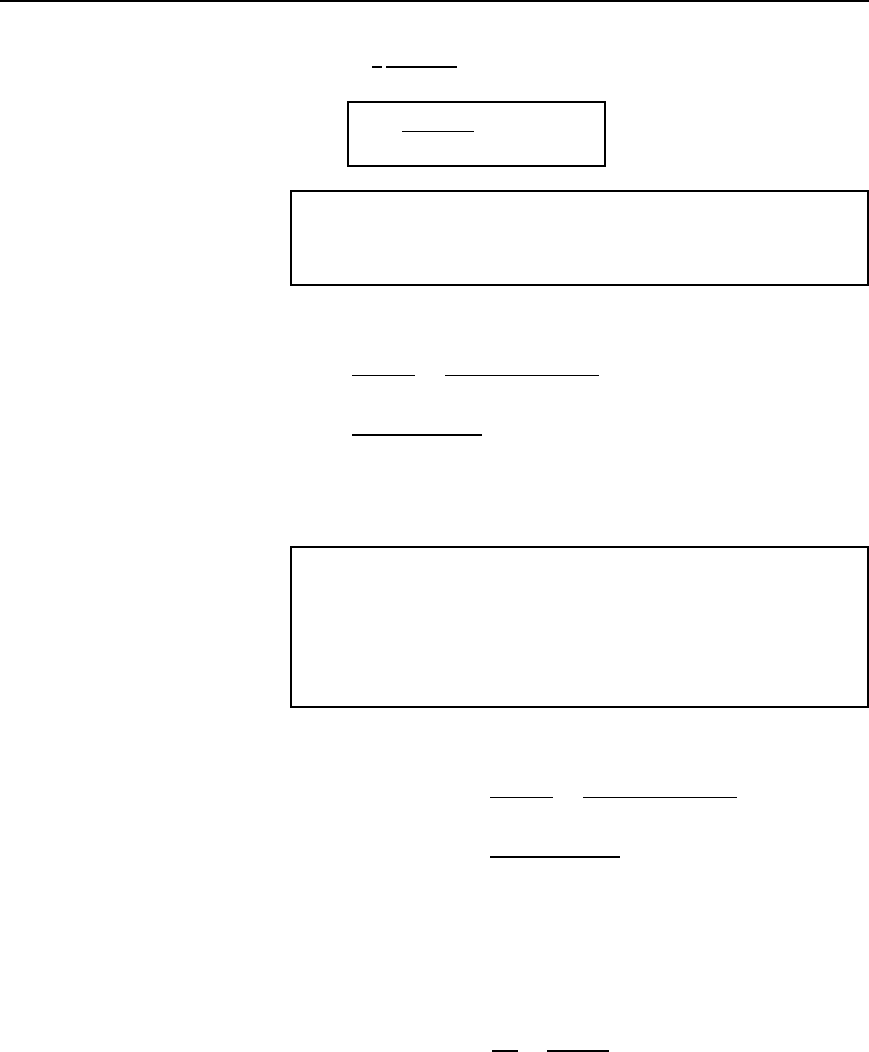
736 Electrical Circuit Theory and Technology

i.e., C=

2p"

ln.D=a/

farads=metre

C D

ln.D=a/

farads=metre

40.14

Problem 9. Two parallel wires, each of diameter 5 mm, are

uniformly spaced in air at a distance of 50 mm between centres.

Determine the capacitance of the line if the total length is 200 m.

From equation (40.14). capacitance per metre length,

C D

ε

lnD/a

8.85 ð 10

12

1

ln50/5/2

since ε

D 1 for air,

8.85 ð 10

12



ln20

D 9.28 ð 10

12

Hence the capacitance of a 200 m length is 9.28 ð 10

12

ð 200 F

D 1860 pF or 1.86 nF

Problem 10. A single-phase circuit is composed of two parallel

conductors, each of radius 4 mm, spaced 1.2 m apart in air. The

p.d. between the conductors at a frequency of 50 Hz is 15 kV.

Determine, for a 1 km length of line, (a) the capacitance of the

conductors, (b) the value of charge carried by each conductor, and

(a) From equation (40.14),

capacitance C D

ε

lnD/a

8.85 ð 10

12

1

ln1.2/4 ð 10

3



8.85 ð 10

12



ln300

D 4.875 pF/m

Hence the capacitance per kilometre length is

4.875 ð 10

12

10

 F D 4.875 nF

(b) Charge Q D CV D 4.875 ð10

9

15 ð 10

 D 73.1 mC

1/ωC

D ωCV

D 2504.875 ð 10

9

15 ð 10



D 0.023 A or 23 mA

Field theory 737

Problem 11. The charging current for an 800 m run of isolated

twin line is not to exceed 15 mA. The voltage between the lines

is 10 kV at 50 Hz. If the line is air-insulated, determine (a) the

maximum value required for the capacitance per metre length,

and (b) the maximum diameter of each conductor if their distance

between centres is 1.25 m.

(a) Charging current I D

1/ωC

D ωCV

from which,

capacitance C D

ωV

15 ð 10

3

25010 ð 10



farads per

800 metre run

D 4.775 nF

Hence the required maximum value of capacitance

4.775 ð 10

9

800

F/m D 5.97 pF=m

(b) From equation (40.14)

C D

ε

lnD/a

thus 5.97 ð10

12

8.85 ð 10

12

1

ln1.25/a

from which, ln



1.25



8.85

5.97

D 4.657

Hence

1.25

D e

4.657

D 105.3

and radius a D

1.25

105.3

m D 0.01187 m or 11.87 mm

Thus the maximum diameter of each conductor is 2 ð 11.87, i.e.,

23.7mm

Further problems on capacitance of an isolated twin line may be found in

Section 40.9, problems 11 to 15, page 754.

40.4 Energy stored in an

electric ﬁeld

Consider the p.d. across a parallel-plate capacitor of capacitance C farads

being increased by d

v volts in dt seconds. If the corresponding increase

in charge is dq coulombs, then dq D Cd

v. If the charging current at that

instant is i amperes, then dq D idt. Thus idt D Cd

v, i.e.,

i D C