Bird J. Electrical Circuit Theory and Technology
Подождите немного. Документ загружается.

488 Electrical Circuit Theory and Technology
(d) Power factor of capacitor = cos D cos88.7
°
D 0.0227
(e) Loss angle, shown as υ in Figure 27.11, is given by
υ D 90
°
88.7
°
D 1.3
°
Alternatively, loss angle υ D arctanωC
x
R
x
(see para (f), page 483)
D arctan
1
44.21
from (c) above,
i.e., υ D 1.3
°
Further problems on a.c. bridges may be found in Section 27.4 following,
problems 1 to 13.
27.4 Further problems
on a.c. bridges
1 A Maxwell-Wien bridge circuit ABCD has the following arm
impedances: AB, 250 resistance; BC, 2
µF capacitor in parallel
with a 10 k resistor; CD, 400 resistor; DA, unknown inductor
having inductance L in series with resistance R. Determine the values
of L and R if the bridge is balanced. [L D 0.20 H, R D 10 ]
2 In a four-arm de Sauty a.c. bridge, arm 1 contains a 2 k non-
inductive resistor, arm 3 contains a loss-free 2.4
µF capacitor, and
arm 4 contains a 5 k non-inductive resistor. When the bridge is
balanced, determine the value of the capacitor contained in arm 2.
[6
µF]
3 A four-arm bridge ABCD consists of: AB — fixed resistor R
1
;
BC—variable resistor R
2
in series with a variable capacitor C
2
;
CD—fixed resistor R
3
; DA — coil of unknown resistance R and
inductance L. Determine the values of R and L if, at balance,
R
1
D 1k, R
2
D 2.5k, C
2
D 4000 pF, R
3
D 1k and the supply
frequency is 1.6 kHz. [R D 4.00 , L D 3.96 mH]
4 The bridge shown in Figure 27.12 is used to measure capacitance
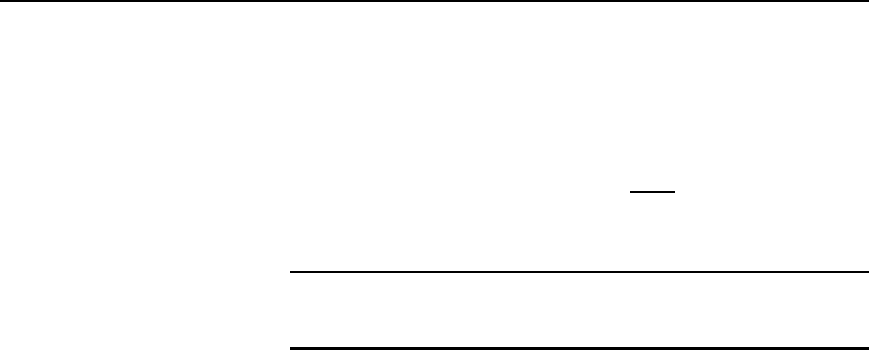
C
x
and resistance R
x
. Derive the balance equations of the bridge
and determine the values of C
x
and R
x
when R
1
D R
4
, C
2
D 0.1 µF,
R
2
D 2k and the supply frequency is 1 kHz.
[C
x
D 38.77 nF, R
x
D 3.27 k]
5 In a Schering bridge network ABCD, the arms are made up as
follows: AB—a standard capacitor C
1
; BC—a capacitor C
2
in
parallel with a resistor R
2
; CD — a resistor R
3
; DA — the capacitor
under test, represented by a capacitor C
x
in series with a resistor
R
x
. The detector is connected between B and D and the a.c. supply
is connected between A and C. Derive the equations for R
x
and
C
x
when the bridge is balanced. Evaluate R
x
and C
x
if, at balance,
C
1
D 1 nF, R
2
D 100 , R
3
D 1kand C
2
D 10 nF.
[R
x
D 10 k, C
x
D 100 pF]
A.c. bridges 489
Figure 27.12 Figure 27.13
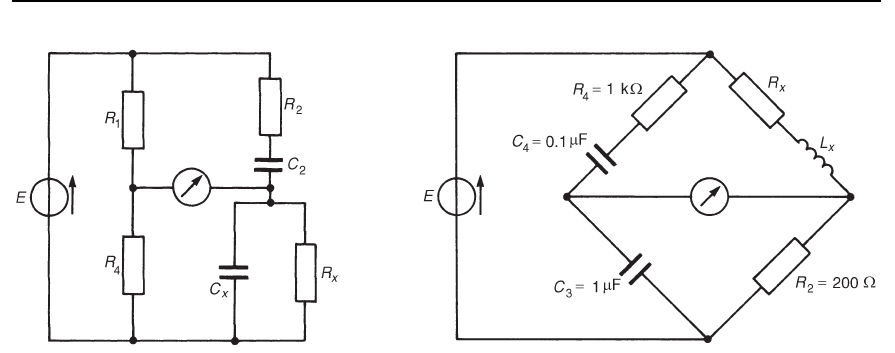
6 The a.c. bridge shown in Figure 27.13 is balanced when the values
of the components are shown. Determine at balance, the values of
R
x
and L
x
.[R D 2k, L
x
D 0.2H]
7 An a.c. bridge has, in arm AB, a pure capacitor of 0.4
µF; in arm BC,
a pure resistor of 500 ; in arm CD, a coil of 50 resistance and
0.1 H inductance; in arm DA, an unknown impedance comprising
resistance R
x
and capacitance C
x
in series. If the frequency of the
bridge at balance is 800 Hz, determine the values of R
x
and C
x
.
[R
x
D 500 , C
x
D 4 µF]
8 When the Wien bridge shown in Figure 27.9 is balanced, the
components have the following values: R
2
D R
3
D 20 k, R
4
D
500 , C
2
D C
3
D 800 pF. Determine for the balance condition
(a) the value of resistance R
1
and (b) the frequency of the bridge
supply. [(a) 250 (b) 9.95 kHz]
9 The conditions at balance of a Schering bridge ABCD used to
measure the capacitance and loss angle of a paper capacitor are
as follows: AB — a pure capacitance of 0.2
µF; BC—a pure
capacitance of 3000 pF in parallel with a 400 resistance; CD—a
pure resistance of 200 ; DA—the capacitance under test which
may be considered as a capacitance C
x
in series with a resistance
R
x
. If the supply frequency is 1 kHz determine (a) the value of R
x
,
(b) the value of C
x
, (c) the power factor of the capacitor, and (d) its
loss angle. [(a) 3 (b) 0.4
µF (c) 0.0075 (d) 0.432
°
]
10 At balance, an a.c. bridge PQRS used to measure the inductance
and resistance of an inductor has the following values: PQ — a
non-inductive 400 resistor; QR— the inductor with unknown
inductance L
x
in series with resistance R
x
;RS—a3µF capacitor in
series with a non-inductive 250 resistor; SP —a 15
µF capacitor.
A detector is connected between Q and S and the a.c. supply is
connected between P and R. Derive the balance equations for R
x
and L
x
and determine their values. [R
x
D 2k, L
x
D 1.5H]

490 Electrical Circuit Theory and Technology
11 A 1 kHz a.c. bridge ABCD has the following components in its four
arms: AB — a pure capacitor of 0.2
µF; BC — a pure resistance of
500 ; CD—an unknown impedance; DA—a 400 resistor in
parallel with a 0.1
µF capacitor. If the bridge is balanced, determine
the series components comprising the impedance in arm CD.
[R D 59.41 , L D 37.6mH]
12 An a.c. bridge ABCD has in arm AB a standard lossless capacitor
of 200 pF; arm BC, an unknown impedance, represented by a loss-
less capacitor C
x
in series with a resistor R
x
; arm CD, a pure 5 k
resistor; arm DA, a 6 resistor in parallel with a variable capacitor
set at 250 pF. The frequency of the bridge supply is 1500 Hz. Deter-
mine for the condition when the bridge is balanced (a) the values of
R
x
and C
x
, and (b) the loss angle.
[(a) R
x
D 6.25 k, C
x
D 240 pF; (b) 0.81
°
]
13 An a.c. bridge ABCD has the following components: AB—a 1k
resistance in parallel with a 0.2
µF capacitor; BC—a 1.2 k
resistance; CD—a 750 resistance; DA — a 0.8
µF capacitor in
series with an unknown resistance. Determine (a) the frequency for
which the bridge is in balance, and (b) the value of the unknown
resistance in arm DA to produce balance.
[(a) 649.7 Hz (b) 375 ]
28 Series resonance and
Q-factor
At the end of this chapter you should be able to:
ž state the conditions for resonance in an a.c. series circuit
ž calculate the resonant frequency in an a.c. series circuit,
f
r
D
1
2
p
LC
ž define Q-factor as
X
R
and as
V
L
V
or
V
C
V
ž determine the maximum value of V
C
and V
COIL
and the
frequency at which this occurs
ž determine the overall Q-factor for two components in series
ž define bandwidth and selectivity
ž calculate Q-factor and bandwidth in an a.c. series circuit
ž determine the current and impedance when the frequency
deviates from the resonant frequency
28.1 Introduction
When the voltage V applied to an electrical network containing resistance,
inductance and capacitance is in phase with the resulting current I, the
circuit is said to be resonant. The phenomenon of resonance is of great
value in all branches of radio, television and communications engineering,
since it enables small portions of the communications frequency spectrum
to be selected for amplification independently of the remainder.
At resonance, the equivalent network impedance Z is purely resistive
since the supply voltage and current are in phase. The power factor of a
resonant network is unity,(i.e., power factor D cos D cos0 D 1).
In electrical work there are two types of resonance— one associated
with series circuits,(which was introduced in Chapter 15), when the input
impedance is a minimum, (which is discussed further in this chapter),
and the other associated with simple parallel networks, when the input
impedance is a maximum (which is discussed in Chapter 29).
28.2 Series resonance
Figure 28.1 shows a circuit comprising a coil of inductance L and resis-
tance R connected in series with a capacitor C. The R–L –C series circuit
has a total impedance Z given by Z D R C jX
L
X
C
) ohms, or Z D
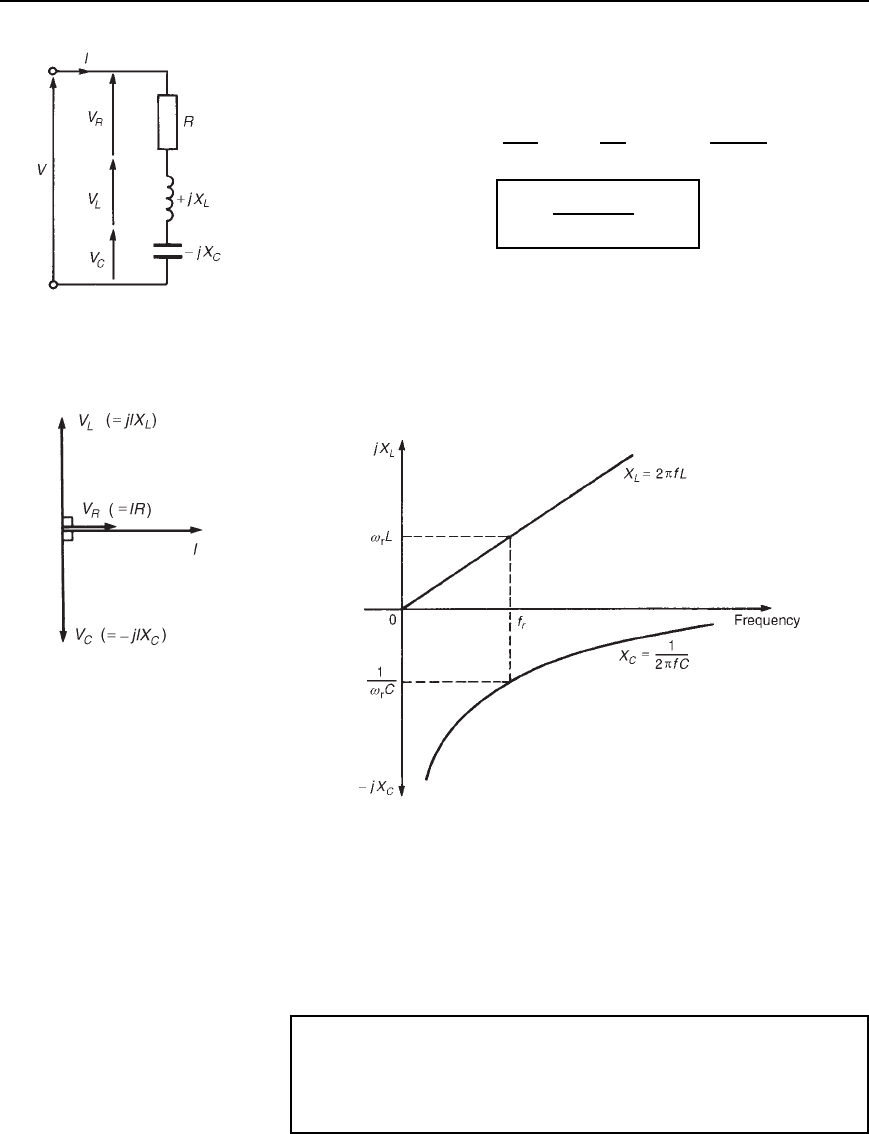
492 Electrical Circuit Theory and Technology
Figure 28.1 R L C series
circuit
R C jωL 1/ωC ohms where ω D 2f. The circuit is at resonance
when X
L
X
C
D 0, i.e., when X
L
D X
C
or ωL D 1/ωC. The phasor
diagram for this condition is shown in Figure 28.2, where jV
L
jDjV
C
j.
Since at resonance ω
r
L D
1
ω
r
C
, ω
2
r
D
1
LC
and ω D
1
p
LC
Thus resonant frequency,
f
r
=
1
2p
p
.LC/
hertz,
since ω
r
D 2f
r
Figure 28.2 Phasor diagram
jV
L
jDjV
C
j
Figure 28.3 shows how inductive reactance X
L
and capacitive reactance
X
C
vary with the frequency. At the resonant frequency f
r
, jX
L
jDjX
C
j.
Since impedance Z D R C jX
L
X
C
and, at resonance, X
L
X
C
D
0, then impedance Z
= R at resonance. This is the minimum value
possible for the impedance as shown in the graph of the modulus of
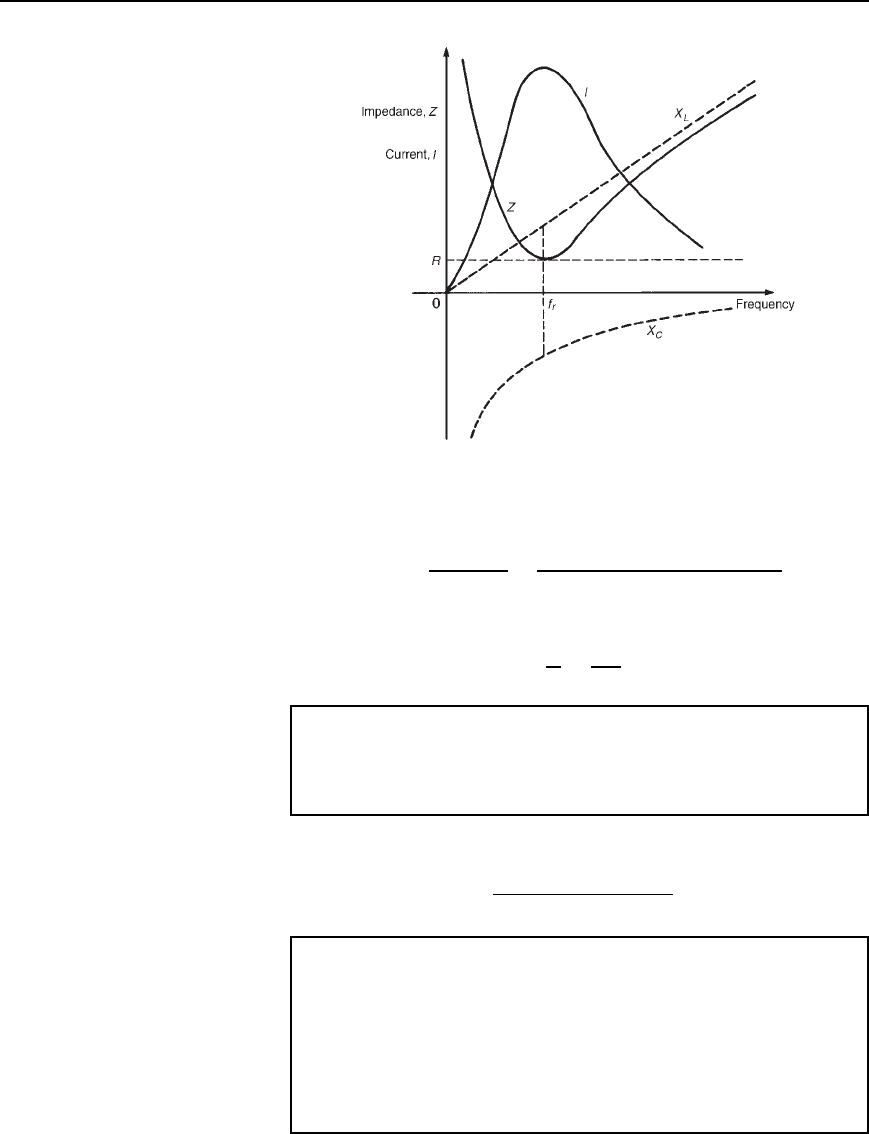
impedance, jZj, against frequency in Figure 28.4.
Figure 28.3 Variation of X
L
and X
C
with frequency
At frequencies less than f
r
, X
L
<X
C
and the circuit is capacitive; at
frequencies greater than f
r
, X
L
>X
C
and the circuit is inductive.
Current I D V/Z. Since impedance Z is a minimum value at resonance,
the current I has a maximum value. At resonance, current I D V/R.A
graph of current against frequency is shown in Figure 28.4.
Problem 1. A coil having a resistance of 10 and an inductance
of 75 mH is connected in series with a 40
µF capacitor across a
200 V a.c. supply. Determine at what frequency resonance occurs,
and (b) the current flowing at resonance.
Series resonance and Q-factor 493
Figure 28.4 jZj and I plotted against frequency
(a) Resonant frequency,
f
r
D
1
2
p
LC
D
1
2
[75 ð 10
3
40 ð 10
6
]
i.e., f
r
= 91.9Hz
(b) Current at resonance, I D
V
R
D
200
10
D 20 A
Problem 2. An R–L –C series circuit is comprised of a coil of
inductance 10 mH and resistance 8 and a variable capacitor C.
The supply frequency is 1 kHz. Determine the value of capacitor
C for series resonance.
At resonance, ω
r
L D 1/ω
r
C, from which, capacitance, C D 1/ω
2
r
L
Hence capacitance C D
1
21000
2
10 ð 10
3
D 2.53 mF
Problem 3. A coil having inductance L is connected in series with
a variable capacitor C. The circuit possesses stray capacitance C
S
which is assumed to be constant and effectively in parallel with
the variable capacitor C. When the capacitor is set to 1000 pF
the resonant frequency of the circuit is 92.5 kHz, and when the
capacitor is set to 500 pF the resonant frequency is 127.8 kHz.
Determine the values of (a) the stray capacitance C
S
, and (b) the
coil inductance L.
494 Electrical Circuit Theory and Technology
For a series R–L –C circuit the resonant frequency f
r
is given by:
f
r
D
1
2
p
LC
The total capacitance of C in parallel with C
S
is given by C C C
S
At 92.5 kHz, C D 1000 pF. Hence
92.5 ð 10
3
D
1
2
[L1000 C C
S
10
12
]
1
At 127.8 kHz, C D 500 pF. Hence
127.8 ð 10
3
D
1
2
[L500 C C
S
10
12
]
2
(a) Dividing equation (2) by equation (1) gives:
127.8 ð 10
3
92.5 ð10
3
D
1
2
[L500 C C
S
10
12
]
1
2
[L1000 C C
S
10
12
]
i.e.,
127.8
92.5
D
[L1000 C C
S
10
12
]
[L500 C C
S
10
12
]
D
1000 C C
S
500 C C
S
where C
S
is in picofarads, from which,
127.8
92.5
2
D
1000 C C
S
500 C C
S
i.e., 1.909 D
1000 C C
S
500 C C
S
Hence 1.909500 C C
S
D 1000 C C
S
954.5 C 1.909C
S
D 1000 C C
S
1.909C
S
C
S
D 1000 954.5
0.909C
S
D 45.5
Thus stray capacitance C
S
D 45.5/0.909 D 50 pF
(b) Substituting C
S
D 50 pF in equation (1) gives:
92.5 ð10
3
D
1
2
[L1050 ð 10
12
]
Series resonance and Q-factor 495
Hence 92.5 ð 10
3
ð 2
2
D
1
L1050 ð 10
12
from which, inductance L D
1
1050 ð 10
12
92.5 ð 10
3
ð 2
2
H
D 2.82 mH
Further problems on series resonance may be found in Section 28.8, prob-
lems 1 to 5, page 512
28.3 Q-factor
Q-factor is a figure of merit for a resonant device such as an L –C–R
circuit.
Such a circuit resonates by cyclic interchange of stored energy, accom-
panied by energy dissipation due to the resistance.
By definition, at resonance Q D 2
maximum energy stored
energy loss per cycle
Since the energy loss per cycle is equal to (the average power dissipated)
ð (periodic time),
Q D 2
maximum energy stored
average power dissipated ð periodic time
D 2
maximum energy stored
average power dissipated ð 1/f
r
since the periodic time T D 1/f
r
.
Thus Q D 2f
r
maximum energy stored
average power dissipated
i.e., Q D ω
r
maximum energy stored
average power dissipated
where ω
r
is the angular frequency at resonance.
In an L –C–R circuit both of the reactive elements store energy during
a quarter cycle of the alternating supply input and return it to the circuit
source during the following quarter cycle. An inductor stores energy in
its magnetic field, then transfers it to the electric field of the capacitor
and then back to the magnetic field, and so on. Thus the inductive and
capacitive elements transfer energy from one to the other successively
with the source of supply ideally providing no additional energy at all.
Practical reactors both store and dissipate energy.
Q-factor is an abbreviation for quality factor and refers to the ‘good-
ness’ of a reactive component.
496 Electrical Circuit Theory and Technology
For an inductor, Q D ω
r
maximum energy stored
average power dissipated
D ω
r
1
2
LI
2
m
I
2
R
D
ω
r
1
2
LI
2
m
I
m
/
p
2
2
R
D
ω
r
L
R
28.1
For a capacitor Q D
ω
r
1
2
CV
2
m
I
m
/
p
2
2
R
D
ω
r
1
2
CI
m
X
C
2
I
m
/
p
2
2
R
D
ω
r
1
2
CI
2
m
1/ω
r
C
2
I
m
/
p
2
2
R
i.e., Q D
1
ω
r
CR
28.2
From expressions (28.1) and (28.2) it can be deduced that
Q D
X
L
R
D
X
C
R
D
reactance
resistance
In fact, Q-factor can also be defined as
Q-factor D
reactance power
resistance
D
Q
P
where Q is the reactive power which is also the peak rate of energy
storage, and P is the average energy dissipation rate. Hence
Q-factor D
Q
P
D
I
2
X
L
or I
2
X
C
I
2
R
D
X
L
R
or
X
C
R
i.e.,
Q =
reactance
resistance
In an R–L –C series circuit the amount of energy stored at resonance is
constant.
When the capacitor voltage is a maximum, the inductor current is zero,
and vice versa, i.e.,
1
2
LI
2
m
D
1
2
CV
2
m
Thus the Q-factor at resonance, Q
r
is given by
Q
r
=
!
r
L
R
=
1
!
r
CR
28.3
However, at resonance ω
r
D 1/
p
LC
Hence Q
r
D
ω
r
L
R
D
1
p
LC
L
R
i.e,
Q
r
=
1
R
L
C
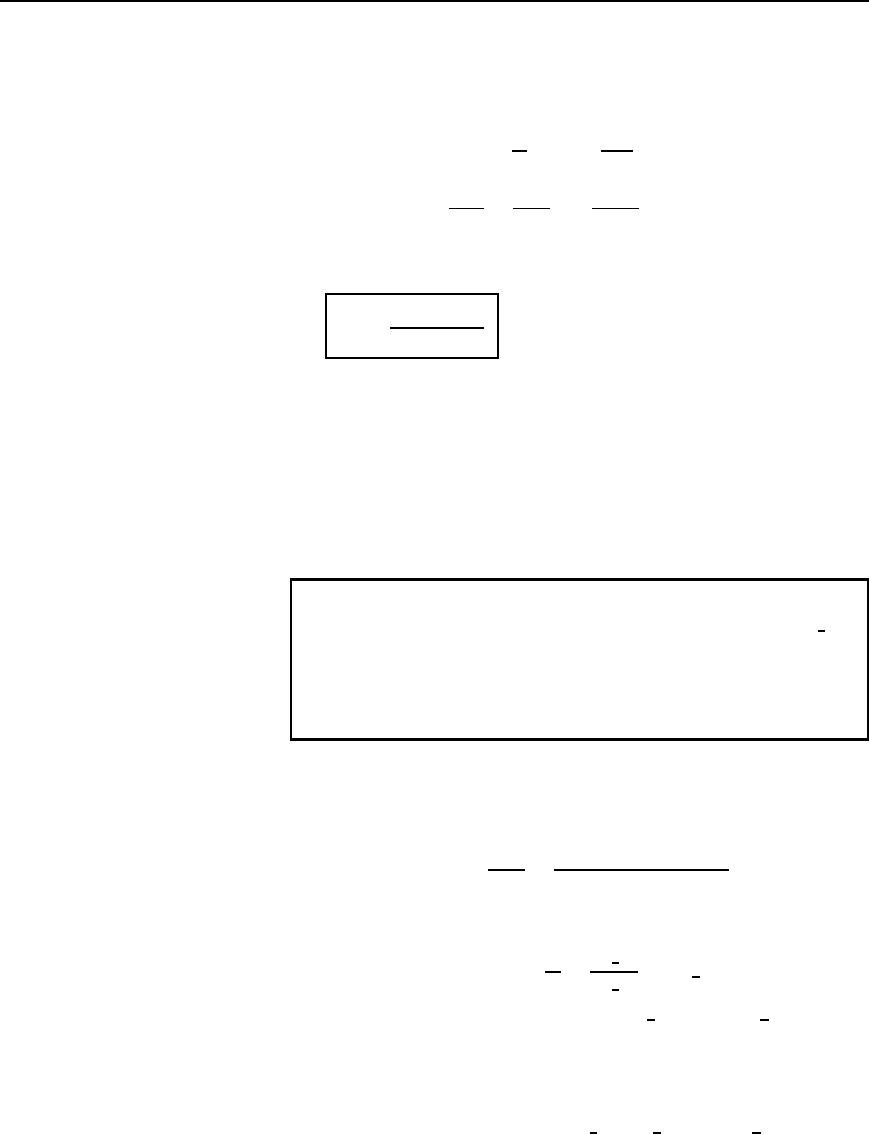
Series resonance and Q-factor 497
It should be noted that when Q-factor is referred to, it is nearly always
assumed to mean ‘the Q-factor at resonance’.
With reference to Figures 28.1 and 28.2, at resonance, V
L
D V
C
V
L
D IX
L
D Iω
r
L D
V
R
ω
r
L D
ω
r
L
R
V D Q
r
V
and V
C
D IX
C
D
I
ω
r
C
D
V/R
ω
r
C
D
1
ω
r
CR
V D Q
r
V
Hence, at resonance, V
L
D V
C
D Q
r
V
or
Q
r
=
V
L
.or V
C
/
V
The voltages V
L
and V
C
at resonance may be much greater than that
of the supply voltage V. For this reason Q is often called the circuit
magnification factor. It represents a measure of the number of times V
L
or V
C
is greater than the supply voltage.
The Q-factor at resonance can have a value of several hundreds. Reso-
nance is usually of interest only in circuits of Q-factor greater than about
10; circuits having Q considerably below this value are effectively merely
operating at unity power factor.
Problem 4. A series circuit comprises a 10 resistance, a 5 µF
capacitor and a variable inductance L. The supply voltage is 20
6
0
°
volts at a frequency of 318.3 Hz. The inductance is adjusted until
the p.d. across the 10 resistance is a maximum. Determine for
this condition (a) the value of inductance L, (b) the p.d. across each
component and (c) the Q-factor.
(a) The maximum voltage across the resistance occurs at resonance
when the current is a maximum. At resonance, ω
r
L D 1/ω
r
C,
from which
inductance L D
1
ω
2
r
C
D
1
2318.3
2
5 ð 10
6
D 0.050 H or 50 mH
(b) Current at resonance I
r
D
V
R
D
20
6
0
°
10
6
0
°
D 2.0
6
0
°
A
p.d. across resistance, V
R
D I
r
R D 2.0
6
0
°
10 D 20
66
0
°
V
p.d. across inductance, V
L
D IX
L
X
L
D 2318.30.050 D 100
Hence V
L
D 2.0
6
0
°
100
6
90
°
D 200
66
90
°
V
