Bird J. Electrical Circuit Theory and Technology
Подождите немного. Документ загружается.

498 Electrical Circuit Theory and Technology
p.d. across capacitor, V
C
D IX
C
D 2.0
6
0
°
100
6
90
°
D 200
66
−90
°
V
(c) Q-factor at resonance, Q
r
D
V
L
or V
C
V
D
200
20
D 10
Alternatively, Q
r
D
ω
r
L
R
D
100
10
D 10
or Q
r
D
1
ω
r
CR
D
1
2318.35 ð 10
6
10
D 10
or Q
r
D
1
R
L
C
D
1
10
0.050
5 ð 10
6
D 10
28.4 Voltage
magnification
For a circuit with a high value of Q (say, exceeding 100), the maximum
volt-drop across the coil, V
COIL
, and the maximum volt-drop across the
capacitor, V
C
, coincide with the maximum circuit current at the resonant
frequency f
r
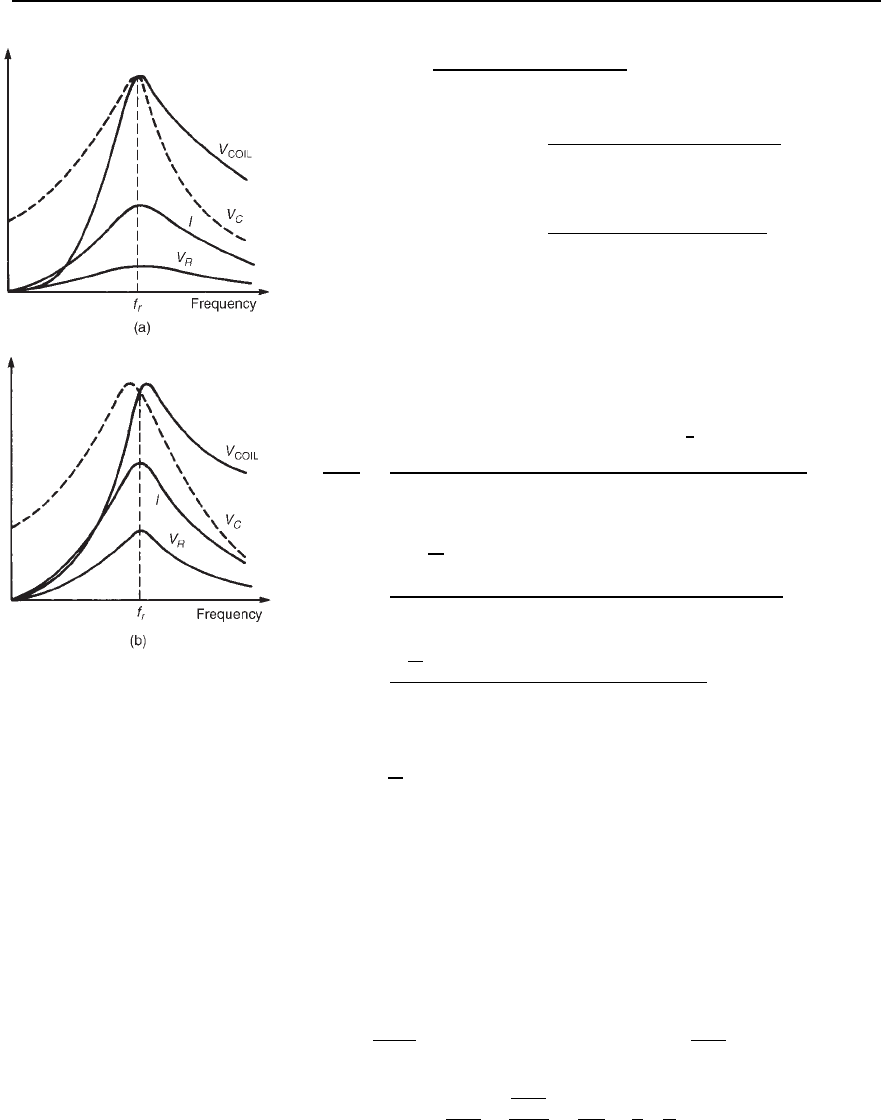
, as shown in 28.5(a). However, if a circuit of low Q (say, less
than 10) is used, it may be shown experimentally that the maximum value
of V
C
occurs at a frequency less than f
r
while the maximum value of
V
COIL
occurs at a frequency higher than f
r
, as shown in Figure 28.5(b).
The maximum current, however, still occurs at the resonant frequency
with low Q. This is analysed below.
Since Q
r
D
V
C
V
then V
C
D VQ
r
However V
C
D IX
C
D I
j
ωC
D I
1
jωC
and since I D
V
Z
,
V
C
D
V
Z
1
jωC
D
V
jωCZ
Z D R C j
ωL
1
ωC
thus V
C
D
V
jωC
R C j
ωL
1
ωC
D
V
jωCR C j
2
ω
2
CL j
2
ωC
ωC
D
V
jωCR ω
2
LC C 1
D
V
1 ω
2
LC C jωCR
D
V[1 ω
2
LC jωCR]
[1 ω
2
LC C jωCR][1 ω
2
LC jωCR]
Series resonance and Q-factor 499
Figure 28.5 (a) High Q-factor
(b) Low Q-factor
D
V[1 ω
2
LC jωCR]
[1 ω
2
LC
2
C ωCR
2
The magnitude of V
C
, jV
C
jD
V
[1 ω
2
LC
2
C ωCR
2
]
[1 ω
2
LC
2
C ωCR
2
]
from the Argand diagram
D
V
[1 ω
2
LC
2
C ωCR
2
]
28.4
To find the maximum value of V
C
, equation (28.4) is differentiated with
respect to ω, equated to zero and then solved—this being the normal
procedure for maximum/minimum problems. Thus, using the quotient
and function of a function rules:
dV
C
dω
D
[1 ω
2
LC
2
C ωCR
2
][0] [V]
1
2
[1 ω
2
LC
2
CωCR
2
]
1/2
21 ω
2
LC2ωLC C 2ωC
2
R
2
[1 ω
2
LC
2
C ωCR
2
]
2
D
0
V
2
[1 ω
2
LC
2
C ωCR
2
]
1/2
21 ω
2
LC
ð2ωLC C 2ωC
2
R
2
1 ω
2
LC
2
C ωCR
2
D
V
2
[21 ω
2
LC2ωLC C 2ωC
2
R
2
]
[1 ω
2
LC
2
C ωCR
2
]
3/2
D 0
for a maximum value
Hence
V
2
[21 ω
2
LC2ωLC C 2ωC
2
R
2
] D 0
and V[1 ω
2
LC2ωLC C ωC
2
R
2
] D 0
and 1 ω
2
LC2ωLC C ωC
2
R
2
D 0
from which, ωC
2
R
2
D 1 ω
2
LC2ωLC
i.e., C
2
R
2
D 2LC1 ω
2
LC
C
2
R
2
LC
D 2 2ω
2
LC and 2ω
2
LC D 2
CR
2
L
Hence ω
2
D
2
2LC
CR
2
L
2LC
D
1
LC
1
2
R
L
2
500 Electrical Circuit Theory and Technology
The resonant frequency, ω
r
D
1
p
LC
from which, ω
2
r
D
1
LC
Thus ω
2
D ω
2
r
1
2
R
L
2
28.5
Q D
ω
r
L
R
from which
R
L
D
ω
r
Q
and
R
L
2
D
ω
2
r
Q
2
Hence, from equation (28.5) ω
2
D ω
2
r
1
2
ω
2
r
Q
2
i.e., ω
2
D ω
2
r
1
1
2Q
2
28.6
or ω D ω
r
1
1
2Q
2
or
f = f
r
1 −
1
2Q
2
28.7
Hence the maximum p.d. across the capacitor does not occur at the reso-
nant frequency, but at a frequency slightly less than f
r
as shown in
Figure 28.5(b). If Q is large, then f ³ f
r
as shown in Figure 28.5(a).
From equation (28.4), jV
C
jD
V
[1 ω
2
LC
2
C ωCR
2
]
and substituting ω
2
D ω
2
r
1
1
2Q
2
from equation (28.6) gives:
maximum value of V
c
,
V
C
m
D
V
1 ω
2
r
1
1
2Q
2
LC
2
C ω
2
r
1
1
2Q
2
C
2
R
2
ω
2
r
D
1
LC
hence
V
C
m
D
V
1
1
LC
1
1
2Q
2
LC
2
C
1
LC
1
1
2Q
2
C
2
R
2
Series resonance and Q-factor 501
D
V
1
1
1
2Q
2
2
C
CR
2
L
1
1
2Q
2
D
V
1
4Q
4
C
CR
2
L
CR
2
L
1
2Q
2
28.8
Q D
ω
r
L
R
D
1
ω
r
CR
hence Q
2
D
ω
r
L
R
1
ω
r
CR
D
L
CR
2
from which,
CR
2
L
D
1
Q
2
Substituting in equation (28.8),
V
C
m
D
V
1
4Q
4
C
1
Q
2
1
2Q
4
D
V
1
Q
2
1
4Q
2
C 1
1
2Q
2
D
V
1
Q
1
1
4Q
2
i.e.,
V
C
m
=
QV
1 −
1
2Q
2
28.9
From equation (28.9), when Q is large, V
C
m
³ QV
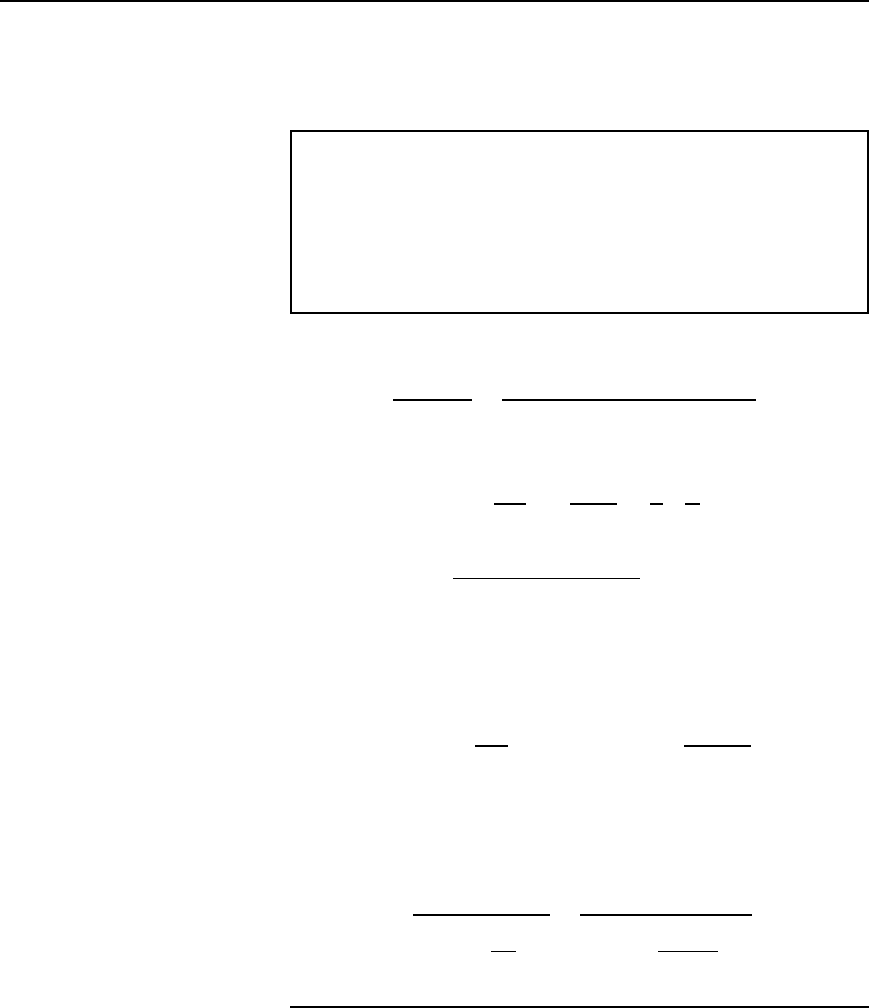
If a similar exercise is undertaken for the voltage across the inductor
it is found that the maximum value is given by:
V
L
m
=
QV
1 −
1
2Q
2
,
i.e., the same equation as for V
C
m
, and frequency,
f D
f
r
1 −
1
2Q
2
,
502 Electrical Circuit Theory and Technology
showing that the maximum p.d. across the coil does not occur at the
resonant frequency but at a value slightly greater than f
r
, as shown in
Figure 28.5(b).
Problem 5. A series L –R–C circuit has a sinusoidal input voltage
of maximum value 12 V. If inductance, L D 20 mH, resistance,
R D 80 , and capacitance, C D 400 nF, determine (a) the reso-
nant frequency, (b) the value of the p.d. across the capacitor at the
resonant frequency, (c) the frequency at which the p.d. across the
capacitor is a maximum, and (d) the value of the maximum voltage
across the capacitor.
(a) The resonant frequency,
f
r
D
1
2
p
LC
D
1
2
[20 ð 10
3
400 ð 10
9
]
D 1779.4Hz
(b) V
C
D QV and Q D
ω
r
L
R
or
1
ω
r
CR
or
1
R
L
C
Hence Q D
21779.420 ð 10
3
80
D 2.80
Thus V
C
D QV D 2.8012 D 33.60 V
(c) From equation (28.7), the frequency f at which V
C
is a maximum
value,
f D f
r
1
1
2Q
2
D 1779.4
1
1
22.80
2
D 1721.7Hz
(d) From equation (28.9), the maximum value of the p.d. across the
capacitor is given by:
V
C
m
D
QV
1
1
2Q
2
D
2.8012
1
1
22.80
2
D 34.15 V
28.5 Q-factors in series
If the losses of a capacitor are not considered as negligible, the overall
Q-factor of the circuit will depend on the Q-factor of the individual
components. Let the Q-factor of the inductor be Q
L
and that of the capac-
itor be Q
C
Series resonance and Q-factor 503
The overall Q-factor, Q
T
D
1
R
T
L
C
from Section (28.3),
where R
T
D R
L
C R
C
Since Q
L
D
ω
r
L
R
L
then R
L
D
ω
r
L
Q
L
and since
Q
C
D
1
ω
r
CR
C
then R
C
D
1
Q
C
ω
r
C
Hence Q
T
D
1
R
L
C R
C
L
C
D
1
ω
r
L
Q
L
C
1
Q
C
ω
r
C
L
C
D
1
1
p
LC
L
Q
L
C
1
Q
C
1
p
LC
C
L
C
since ω
r
D
1
p
LC
D
1
L
Q
L
L
1/2
C
1/2
C
L
1/2
C
1/2
Q
C
C
L
C
D
1
1
Q
L
L
1/2
C
1/2
C
1
Q
C
L
1/2
C
1/2
L
C
D
1
1
Q
L
L
C
C
1
Q
C
L
C
L
C
D
1
L
C
1
Q
L
C
1
Q
C
L
C
D
1
1
Q
L
C
1
Q
C
D
1
Q
C
C Q
L
Q
L
Q
C
i.e., the overall Q-factor,
Q
T
=
Q
L
Q
C
Q
L
Y Q
C
Problem 6. An inductor of Q-factor 60 is connected in series with
a capacitor having a Q-factor of 390. Determine the overall Q-factor
of the circuit.
From above, overall Q-factor,
Q
T
D
Q
L
Q
C
Q
L
C Q
C
D
60390
60 C 390
D
23400
450
D 52
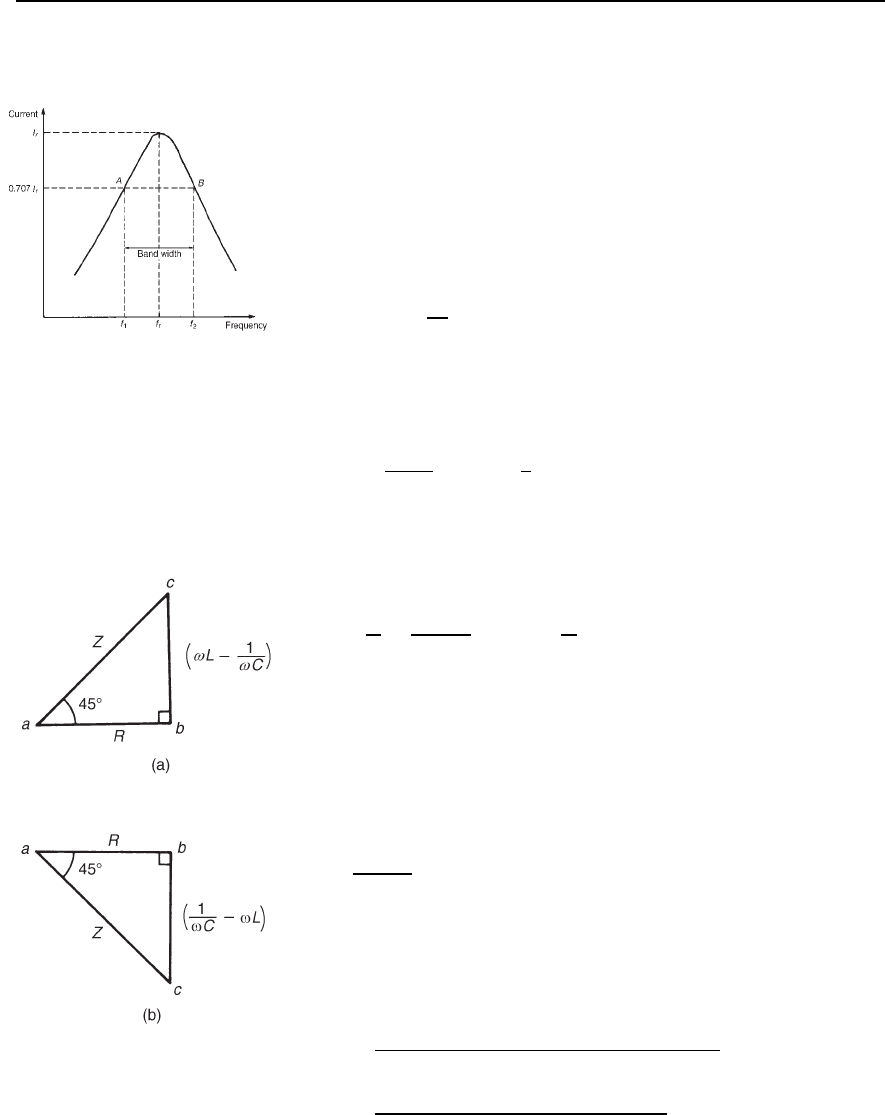
504 Electrical Circuit Theory and Technology
28.6 Bandwidth
Figure 28.6 shows how current I varies with frequency f in an R–L –C
series circuit. At the resonant frequency f
r
, current is a maximum value,
shown as I
r
Also shown are the points A and B where the current is 0.707
of the maximum value at frequencies f
1
and f
2
. The power delivered
to the circuit is I
2
R.AtI D 0.707I
r
, the power is 0.707I
r
2
R D 0.5 I
2
r
R,
i.e., half the power that occurs at frequency f
r
. The points corresponding
to f
1
and f
2
are called the half-power points. The distance between
these points, i.e., (f
2
f
1
), is called the bandwidth.
When the ratio of two powers P
1
and P
2
is expressed in decibel units,
the number of decibels X is given by:
X D 10 lg
P
2
P
1
dB (see Section 10.14, page 126)
Figure 28.6 Bandwidth and
half-power points f
1
and f
2
Let the power at the half-power points be 0.707I
r
2
R D I
2
r
R/2 and let
the peak power be I
2
r
R. then the ratio of the power in decibels is given by:
10 lg
I
2
r
R/2
I
2
r
R
D 10 lg
1
2
D
−3dB
It is for this reason that the half-power points are often referred to as ‘the
−3 dB points’.
At the half-power frequencies, I D 0.707 I
r
, thus impedance
Z D
V
I
D
V
0.707I
r
D 1.414
V
I
r
D
p
2Z
r
D
p
2R
(since at resonance Z
r
D R)
Since Z D
p
2R, an isosceles triangle is formed by the impedance
triangles, as shown in Figure 28.7, where ab D bc. From the impedance
triangles it can be seen that the equivalent circuit reactance is equal to
the circuit resistance at the half-power points.
At f
1
, the lower half-power frequency jX
C
j > jX
L
j (see Figure 28.4)
Thus
1
2f
1
C
2f
1
L D R
from which, 1 4
2
f
2
1
LC D 2f
1
CR
i.e., 4
2
LCf
2
1
C 2CRf
1
1 D 0
This is a quadratic equation in f
1
. Using the quadratic formula gives:
f
1
D
2CR š
[2CR
2
44
2
LC1]
24
2
LC
D
2CR š
[4
2
C
2
R
2
C 16
2
LC]
8
2
LC
Figure 28.7 (a) Inductive
impedance triangle
(b) Capacitive impedance
triangle
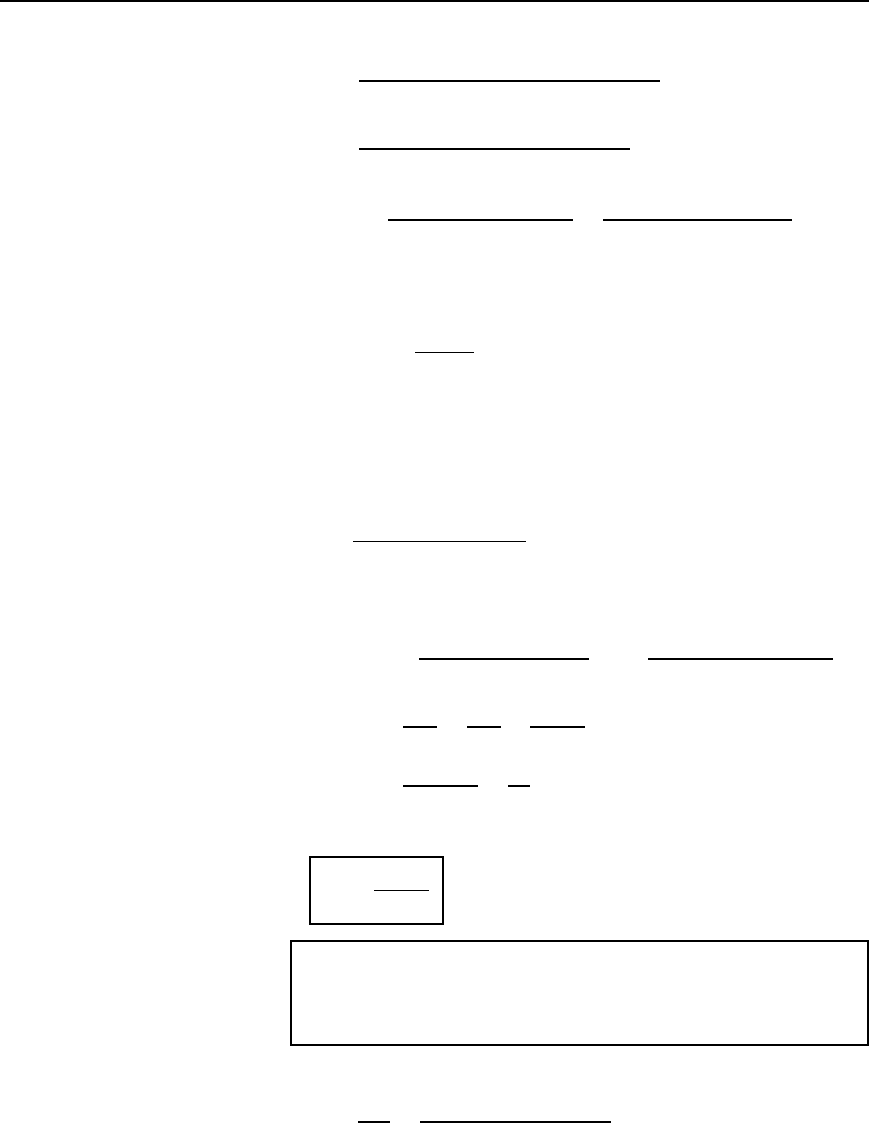
Series resonance and Q-factor 505
D
2CR š
[4
2
C
2
R
2
C 4L/C]
8
2
LC
D
2CR š 2C
[R
2
C 4L/C]
8
2
LC
Hence f
1
D
R š
[R
2
C 4L/C]
4L
D
−R Y
[R
2
Y .4L=C/]
4pL
(since
[R
2
C 4L/C] >Rand f
1
cannot be negative).
At f
2
, the upper half-power frequency jX
L
j > jX
C
j (see Figure 28.4)
Thus 2f
2
L
1
2f
2
C
D R
from which, 4
2
f
2
2
LC 1 D R2f
2
C
i.e., 4
2
LCf
2
2
2CRf
2
1 D 0
This is a quadratic equation in f
2
and may be solved using the quadratic
formula as for f
1
, giving:
f
2
=
R Y
[R
2
Y .4L=C/]
4pL
Bandwidth D f
2
f
1
D
R C
[R
2
C 4L/C]
4L
R C
[R
2
C 4L/C]
4L
D
2R
4L
D
R
2L
D
1
2L/R
D
f
r
2f
r
L/R
D
f
r
Q
r
from equation (28.3). Hence for a series R–L –C circuit
Q
r
=
f
r
f
2
− f
1
28.10
Problem 7. A filter in the form of a series L –R–C circuit is
designed to operate at a resonant frequency of 10 kHz. Included
within the filter is a 10 mH inductance and 5 resistance. Deter-
mine the bandwidth of the filter.
Q-factor at resonance is given by
Q
r
D
ω
r
L
R
D
2 1000010 ð10
3
5
D 125.66
506 Electrical Circuit Theory and Technology
Since Q
r
D f
r
/f
2
f
1
,
Bandwidth, . f
2
− f
1
/ D
f
r
Q
r
D
10000
125.66
D 79.6Hz
An alternative equation involving f
r
At the lower half-power frequency f
1
:
1
ω
1
C
ω
1
L D R
At the higher half-power frequency f
2
: ω
2
L
1
ω
2
C
D R
Equating gives:
1
ω
1
C
ω
1
L D ω
2
L
1
ω
2
C
Multiplying throughout by C gives:
1
ω
1
ω
1
LC D ω
2
LC
1
ω
2
However, for series resonance, ω
2
r
D 1/LC
Hence
1
ω
1
ω
1
ω
2
r
D
ω
2
ω
2
r
1
ω
2
i.e.,
1
ω
1
C
1
ω
2
D
ω
2
ω
2
r
C
ω
1
ω
2
r
D
ω
1
C ω
2
ω
2
r
Therefore
ω
2
C ω
1
ω
1
ω
2
D
ω
1
C ω
2
ω
2
r
,
from which, ω
2
r
D ω
1
ω
2
or ω
r
D
p
ω
1
ω
2
Hence 2f
r
D
p
[2f
1
2f
2
]and
f
r
=
p
.f
1
f
2
/
28.11
Selectivity is the ability of a circuit to respond more readily to signals of
a particular frequency to which it is tuned than to signals of other frequen-
cies. The response becomes progressively weaker as the frequency departs
from the resonant frequency. Discrimination against other signals becomes
more pronounced as circuit losses are reduced, i.e., as the Q-factor is
increased. Thus Q
r
D f
r
/f
2
f
1
is a measure of the circuit selec-
tivity in terms of the points on each side of resonance where the circuit
current has fallen to 0.707 of its maximum value reached at resonance.
The higher the Q-factor, the narrower the bandwidth and the more selec-
tive is the circuit. Circuits having high Q-factors (say, in the order 300)
are therefore useful in communications engineering. A high Q-factor in a
series power circuit has disadvantages in that it can lead to dangerously
high voltages across the insulation and may result in electrical breakdown.
For example, suppose that the working voltage of a capacitor is stated
as 1 kV and is used in a circuit having a supply voltage of 240 V. The
maximum value of the supply will be
p
2240, i.e., 340 V. The working
voltage of the capacitor would appear to be ample. However, if the Q-
factor is, say, 10, the voltage across the capacitor will reach 2.4 kV.
Series resonance and Q-factor 507
Since the capacitor is rated only at 1 kV, dielectric breakdown is more
than likely to occur.
Low Q-factors, say, in the order of 5 to 25, may be found in power
transformers using laminated iron cores.
A capacitor-start induction motor, as used in domestic appliances such
as washing machines and vacuum-cleaners, having a Q-factor as low as
1.5 at starting would result in a voltage across the capacitor 1.5 times that
of the supply voltage; hence the cable joining the capacitor to the motor
would require extra insulation.
Problem 8. An R–L –C series circuit has a resonant frequency
of 1.2 kHz and a Q-factor at resonance of 30. If the impedance
of the circuit at resonance is 50 determine the values of (a) the
inductance, and (b) the capacitance. Find also (c) the bandwidth,
(d) the lower and upper half-power frequencies and (e) the value
of the circuit impedance at the half-power frequencies.
(a) At resonance the circuit impedance, Z D R, i.e., R D 50 .
Q-factor at resonance, Q
r
D ω
r
L/R
Hence inductance, L D
Q
r
R
ω
r
D
3050
21200
D 0.199 H or 199 mH
(b) At resonance ω
r
L D 1/ω
r
C
Hence capacitance, C D
1
ω
2
r
L
D
1
21200
2
0.199
D 0.088 mF or 88 nF
(c) Q-factor at resonance is also given by Q
r
D f
r
/f
2
f
1
, from
which,
bandwidth,.f
2
− f
1
/ D
f
r
Q
r
D
1200
30
D 40 Hz
(d) From equation (28.11), resonant frequency, f
r
D
p
f
1
f
2
,
i.e., 1200 D
p
f
1
f
2
from which, f
1
f
2
D 1200
2
D 1.44 ð 10
6
28.12
From part(c), f
2
f
1
D 40 28.13
From equation (28.12), f
1
D 1.44 ð 10
6
/f
2
Substituting in equation (28.13) gives:
f
2
1.44 ð 10
6
f
2
D 40
