Bhushan B. Nanotribology and Nanomechanics: An Introduction

Подождите немного. Документ загружается.

484 Marina Ruths and Jacob N. Israelachvili

Friction force F (mN)

Time (min)0

Steady-state

Transition

Tran-

sition

n= 3 layers n = 2 layers n =1

1.6 nm

F same

Fig. 9.27. Measured change in friction during interlayer transitions of the silicone liquid oc-

tamethylcyclotetrasiloxane (OMCTS), an inert liquid whose quasi-spherical molecules have

a diameter of 0.8 nm. In this system, the shear stress S

= F/A was found to be constant as

long as the number of layers, n, remained constant. Qualitatively similar results have been

obtained with other quasi-spherical molecules such as cyclohexane [335]. The shear stresses

are only weakly dependent on the sliding velocity v. However, for sliding velocities above

some critical value, v

, the stick–slip disappears and sliding proceeds smoothly at the kinetic

value (after [275], with permission)

Adhesion hysteresis/

Friction

Temperature T (qC)

05010 20 30 40

Load, velocity,

chain density

Chain fluidity,

chain dilution

Liquid-like

Solid-like

Amorphous

Fig. 9.28. Schematic friction phase diagram representing the trends observed in the boundary

friction of a variety of diﬀerent surfactant monolayers. The characteristic bell-shaped curve

also correlates with the adhesion energy hysteresis of the monolayers. The arrows indicate

the direction in which the whole curve is dragged when the load, velocity, etc., is increased

(after [292], with permission)

9 Surface Forces and Nanorheology of Molecularly Thin Films 485

forces can, therefore, be represented on a friction phase diagram such as the one

shown in Fig. 9.28. Experiments have shown that T

, and the whole bell-shaped

curve, are shifted along the temperature axis (as well as in the vertical direction)

in a systematic way when the load, sliding velocity, etc., are varied. These shifts

also appear to be highly correlated with one another, for example, an increase in

temperature produces eﬀects that are similar to decreasing the sliding speed or load.

Such eﬀects are also commonly observed in other energy-dissipating phenom-

ena such as polymer viscoelasticity [346], and it is likely that a similar physical

mechanism is at the heart of all such phenomena. A possible molecular process un-

derlying the energy dissipation of chain molecules during boundary-layersliding is

illustrated in Fig. 9.29, which shows the three main dynamic phase states of bound-

ary monolayers.

In contrast to the characteristic relaxation time associated with ﬂuid lubricants,

it has been established that for unlubricated (dry, solid, rough) surfaces, there is

a characteristic memory distance that must be exceeded before the system loses all

memory of its initial state (original surface topography).The underlying mechanism

for a characteristic distance was ﬁrst used to successfully explain rock mechanics

and earthquake faults [347] and, more recently, the tribological behavior of unlu-

bricated surfaces of ceramics, paper and elastomeric polymers [314,348]. Recent

experiments [285,344,345,349] suggest that ﬂuid lubricants composed of complex

branched-chained or polymer molecules may also have characteristic distances (in

addition to characteristic relaxation times) associated with their tribological behav-

ior –the characteristic distancebeing thetotal sliding distancethat must beexceeded

before the system reaches its steady-state tribological conditions (see Sect. 9.8.3).

Solid-like Amorphous Liquid-like

a) b) c)

Fig. 9.29. Diﬀerent dynamic phase states of boundary monolayers during adhesive contact

and/or frictional sliding. Solid-like (a) and liquid-like monolayers (c) exhibit low adhesion

hysteresis and friction. Increasing the temperature generally shifts a system from the left

to the right. Changing the load, sliding velocity, or other experimental conditions can also

change the dynamic phase state of surface layers, as shown in Fig. 9.28 (after [292], with

permission)

486 Marina Ruths and Jacob N. Israelachvili

Abrupt versus Continuous Transitions Between Smooth and Stick–Slip Sliding

An understanding of stick–slip is of great practical importance in tribology [350],

since these spikes are the major cause of damage and wear of moving parts. Stick–

slip motionis a verycommon phenomenonand is also the cause of sound generation

(the sound of a violin string, a squeaking door, or the chatter of machinery), sensory

perception(taste textureand feel), earthquakes,granularﬂow, nonuniformﬂuid ﬂow

such as the spurtingﬂow of polymericliquids, etc. In the previoussection, the stick–

slip motion arising from freezing–melting transitions in thin interfacial ﬁlms was

described.There areother mechanismsthat can giverise to stick–slipfriction,which

will now be considered. However, before proceeding with this, it is important to

clarify exactly what one is measuring during a friction experiment.

Most tribological systems and experiments can be described in terms of an

equivalent mechanical circuit with certain characteristics. The friction force F

which is generated at the surfaces, is generally measured as F at some other place

in the set-up. The mechanical coupling between the two may be described in terms

of a simple elastic stiﬀness or compliance, K, or as more complex nonelastic coef-

ﬁcients, depending on the system. The distinction between F and F

is important

because, in almost all practical cases, the applied, measured, or detected force, F,is

not the same as the “real” or “intrinsic” friction force, F

, generated at the surfaces.

F and F

are coupled in a way that depends on the mechanical construction of the

system, for example, the axle of a car wheel that connects it to the engine. This

coupling can be modeled as an elastic spring of stiﬀness K and mass m.Thisisthe

simplest type of mechanical couplingand is also the same as in SFA- and AFM-type

experiments. More complicated real systems can be reduced to a system of springs

and dashpots, as described by Peachey et al. [351] and Luengo et al. [47].

We now consider four diﬀerent models of stick–slip friction, where the me-

chanical couplings are assumed to be of the simple elastic spring type. The ﬁrst

three mechanisms may be considered traditional or classical mechanisms or mod-

els [350], the fourth is essentially the same as the freezing–melting phase-transition

model described in Sect. 9.8.3.

Rough Surfaces or Surface Topology Model Rapid slips can occur whenever an

asperity on one surface goes over the top of an asperity on the other surface. The

extentof the slip will depend on asperity heightsand slopes, on the speed of sliding,

and on the elastic compliance of the surfaces and the moving stage. As in all cases

of stick–slip motion, the driving velocity v may be constant, but the resulting motion

at the surfaces v

will display large slips. This type of stick–slip has been described

by Rabinowicz [350]. It will not be of much concern here since it is essentially

a noise-type ﬂuctuation, resulting from surface imperfections rather than from the

intrinsic interaction between two surfaces. Actually, at the atomic level, the regular

atomic-scale corrugations of surfaces can lead to periodic stick–slip motion of the

type shown here. This is what is sometimes measured by AFM tips [10,58,59,290,

339,340].

9 Surface Forces and Nanorheology of Molecularly Thin Films 487

Distance-Dependent or Creep Model Another theory of stick–slip, observed in

solid-on-solid sliding, is one that involves a characteristic distance, but also a char-

acteristic time, τ

, this being the characteristic time required for two asperities to

increase their adhesion strength after coming into contact. Originally proposed by

Rabinowicz [350,352],this model suggeststhat two rough macroscopicsurfaces ad-

here through their microscopicasperities of a characteristiclength. During shearing,

each surface must ﬁrst creep this distance (the size of the contacting junctions) after

which the surfaces continue to slide, but with a lower (kinetic) friction force than

the original (static) value. The reason for the decrease in the friction force is that

even though, on average, new asperity junctions should form as rapidly as the old

ones break, the time-dependent adhesion and friction of the new ones will be lower

than the old ones.

The friction force, therefore, remains high during the creep stage of the slip.

However, once the surfaces have moved the characteristic distance, the friction

rapidly drops to the kinetic value. For any system where the kinetic friction is less

than the static force (or one that has a negative slope over some part of its curve

of F

versus v

) will exhibit regular stick–slip sliding motion for certain values of

K, m, and driving velocity, v.

This type of friction has been observed in a variety of dry (unlubricated) sys-

tems such as paper-on-paper [353, 354] and steel-on-steel [352, 355, 356]. This

model is also used extensively in geologic systems to analyze rock-on-rock slid-

ing [357,358]. While originally described for adhering macroscopic asperity junc-

tions, the distance-dependentmodel may also apply to molecularlysmooth surfaces.

For example, forpolymer lubricant ﬁlms, the characteristiclength would now be the

chain–chain entanglement length, which could be much larger in a conﬁned geo-

metry than in the bulk.

Velocity-Dependent Friction Model In contrast to the two friction models men-

tionedabove,which applymainly tounlubricated,solid-on-solidcontacts,the stick–

slip of surfaces with thin liquid ﬁlms between them is better described by other

mechanisms. The velocity-dependentfriction model is the most studied mechanism

of stick–slip and, until recently, was considered to be the only cause of intrinsic

stick–slip. If a friction force decreases with increasing sliding velocity, as occurs

with boundary ﬁlms exhibiting shear thinning, the force (F

) needed to initiate mo-

tion will be higher than the force (F

) needed to maintain motion.

A decreasing intrinsic friction force F

with sliding velocity v

results in the

sliding surface or stage moving in a periodic fashion, where during each cycle rapid

acceleration is followed by rapid deceleration. As long as the drive continues to

move at a ﬁxed velocity v, the surfaces will continue to move in a periodic fashion

punctuated by abrupt stops and starts whose frequency and amplitude depend not

only on the function F

),butalsoonthestiﬀness K and mass m of the moving

stage, and on the starting conditions at t = 0.

More precisely, the motion of the sliding surface or stage can be determined by

solving the following diﬀerential equation:

m¨x = (F

−F) = F

−(x

−x)K or m¨x+ (x

−x)K−F

= 0 ,

(9.47)

488 Marina Ruths and Jacob N. Israelachvili

where F

= F

,t) is the intrinsic or “real” friction force at the shearing sur-

faces, F is the force on the spring (the externally applied or measured force), and

−F) is the force on the stage. To solve (9.47) fully, one must also know the

initial (starting) conditions at t = 0, and the driving or steady-state conditions at ﬁ-

nite t. Commonly, the driving condition is: x = 0fort < 0andx = vt for t > 0,

where v = constant. In other systems, the appropriate driving condition may be

F = constant.

Various, mainly phenomenological, forms for F

= F

,t) have been pro-

posed to explain various kinds of stick–slip phenomena. These models generally

assume a particular functional form for the friction as a function of velocity only,

= F

), and they may also contain a number of mechanically coupled elements

comprising the stage [359,360]. One version is a two-state model characterized by

two friction forces, F

and F

, which is a simpliﬁed version of the phase transitions

model described below. More complicated versions can have a rich F–v spectrum,

as proposed by Persson [361]. Unless the experimental data is very detailed and ex-

tensive, these models cannot generally distinguish between diﬀerent types of mech-

anisms. Neither do they address the basic question of the origin of the friction force,

since this is assumed to begin with.

Experimental data has been used to calculate the friction force as a function of

velocity within an individual stick–slip cycle [363]. For a macroscopic granular ma-

terial conﬁned between solid surfaces, the data shows a velocity-weakeningfriction

force during the ﬁrst half of the slip. However, the data also shows a hysteresis loop

in the friction–velocity plot, with a diﬀerent behavior in the deceleration half of the

slip phase. Similar results were observedfor a 1–2 nm liquid lubricant ﬁlm between

mica surfaces [345]. These results indicate that a purely velocity-dependentfriction

law is insuﬃcient to describe such systems, and an additional element such as the

state of the conﬁned material must be considered.

Phase TransitionsModel In recent molecular dynamics computer simulationsit has

been found that thin interfacial ﬁlms undergo ﬁrst-order phase transitions between

solid-like and liquid-like states during sliding [255,364], as illustrated in Fig. 9.30.

It has been suggested that this is responsible for the observed stick–slip behavior of

simple isotropic liquids between two solid crystalline surfaces. With this interpreta-

tion, stick–slip is seen to arise because of the abruptchange in the ﬂow properties of

a ﬁlm at a transition [278,279,326], rather than the gradual or continuous change,

as occurs in the previous example. Other computer simulations indicate that it is the

stick–slip that induces a disorder (“shear melting”) in the ﬁlm, not the other way

around [365].

An interpretation of the well-known phenomenon of decreasing coeﬃcient of

friction with increasing sliding velocity has been proposed by Thompson and Rob-

bins [255] based on their computer simulation. This postulates that it is not the

friction that changes with sliding speed v, but rather the time various parts of the

system spend in the sticking and sliding modes. In other words, at any instant dur-

ing sliding, the friction at any local region is always F

or F

, corresponding to

the “static” or “kinetic” values. The measured frictional force, however, is the sum

9 Surface Forces and Nanorheology of Molecularly Thin Films 489

of all these discrete values averaged over the whole contact area. Since as v in-

creases each local region spends more time in the sliding regime (F

) and less in

the sticking regime (F

), the overall friction coeﬃcient falls. One may note that this

interpretation reverses the traditional way that stick–slip has been explained. Rather

than invoking a decreasing friction with velocity to explain stick–slip, it is now the

more fundamental stick–slip phenomenon that is producing the apparent decrease

in the friction force with increasing sliding velocity. This approach has been studied

analytically by Carlson and Batista [366], with a comprehensive rate- and state-

dependent friction force law. This model includes an analytic description of the

freezing–melting transitions of a ﬁlm, resulting in a friction force that is a function

of sliding velocity in a natural way. This model predicts a full range of stick–slip

behavior observed experimentally.

An example of the rate- and state-dependent model is observed when shearing

thin ﬁlms of OMCTS between mica surfaces [367,368]. In this case, the static fric-

tion between the surfaces is dependent on the time that the surfaces are at rest with

respect to each other, while the intrinsic kinetic friction F

k,0

is relatively constant

over the range of velocities. At slow driving velocities, the system responds with

stick–slip sliding with the surfaces reaching maximum static friction before each

slip event, and the amplitude of the stick–slip, F

−F

, is relatively constant. As the

driving velocity increases, the static friction decreases as the time at relative rest

becomes shorter with respect to the characteristic time of the lubricant ﬁlm. As the

static friction decreases with increasing drive velocity, it eventually equals the in-

trinsic kinetic friction F

k,0

, which deﬁnes the critical velocity v

, above which the

surfaces slide smoothly without the jerky stick–slip motion.

The above classiﬁcations of stick–slip are not exclusive, and molecular mecha-

nisms of real systems may exhibit aspects of diﬀerent models simultaneously. They

do, however, provide a convenient classiﬁcation of existing models and indicate

which experimental parameters should be varied to test the diﬀerent models.

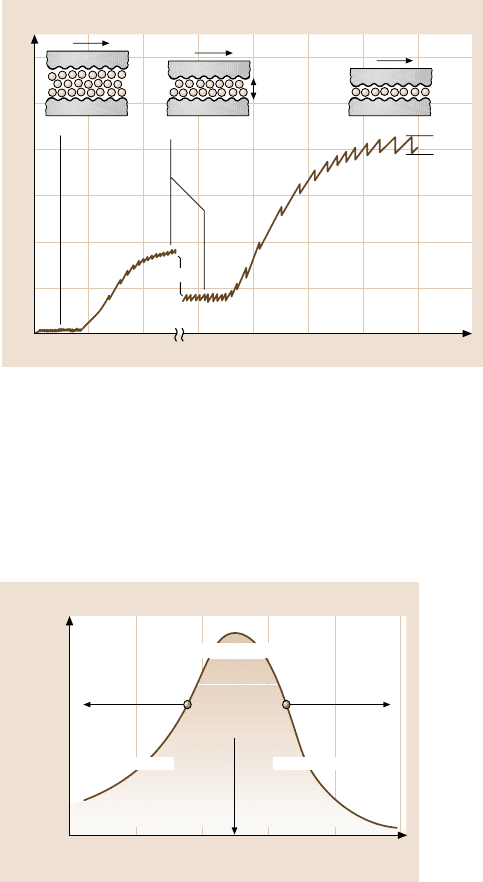
Critical Velocity for Stick–Slip For anygivensetof conditions,stick–slipdisappears

above some critical sliding velocity v

, above which the motion continues smoothly

in the liquid-like or kinetic state [261,285, 342,345, 362]. The critical velocity is

well described by two simple equations. Both are based on the phase transition

model,and bothinclude someparameter associated with the inertia ofthe measuring

instrument. The ﬁrst equation is based on both experiments and simple theoretical

modeling [362]:

≈

−F

)

5Kτ

, (9.48)

where τ

is the characteristic nucleation time or freezing time of the ﬁlm. For ex-

ample, inserting the following typically measured values for a ∼1nm thick hexa-

decane ﬁlm between mica: (F

−F

) ≈ 5 mN, spring constant K ≈ 500Nm

−1

,and

nucleation time [362] τ

≈ 5s, we obtain v

≈ 0.4µms

−1

, which is close to typically

measured values (Fig. 9.30b).

490 Marina Ruths and Jacob N. Israelachvili



Fig. 9.30. (a) “Phase transitions” model of stick–slip where a thin liquid ﬁlm alternately

freezes and melts as it shears, shown here for 22 spherical molecules conﬁned between two

solid crystalline surfaces. In contrast to the velocity-dependent friction model, the intrinsic

friction force is assumed to change abruptly (at the transitions), rather than smooth or con-

tinuously. The resulting stick–slip is also diﬀerent, for example, the peaks are sharper and

the stick–slip disappears above some critical velocity v

. Note that, while the slip displace-

ment is here shown to be only two lattice spacings, in most practical situations it is much

larger, and that freezing and melting transitions at surfaces or in thin ﬁlms may not be the

same as freezing or melting transitions between the bulk solid and liquid phases. (b)Ex-

act reproduction of a chart-recorder trace of the friction force for hexadecane between two

untreated mica surfaces at increasing sliding velocity v, plotted as a function of time. In

general, with increasing sliding speed, the stick–slip spikes increase in frequency and de-

crease in magnitude. As the critical sliding velocity v

is approached, the spikes become

erratic, eventually disappearing altogether at v = v

. At higher sliding velocities the sliding

continues smoothly in the kinetic state. Such friction traces are fairly typical for simple liq-

uid lubricants and dry boundary lubricant systems (see Fig. 9.31a) and may be referred to

as the “conventional” type of static–kinetic friction (in contrast to panel (c)). Experimental

conditions: contact area A = 4×10

−9

,loadL = 10 mN, ﬁlm thickness D = 0.4−0.8nm,

v = 0.08−0.4 µms

−1

, v

≈ 0.3 µms

−1

, atmosphere: dry N

gas, T = 18

◦

C. ((a)and(b)af-

smooth sliding to “inverted” stick–slip and to a second smooth-sliding regime with increasing

driving velocity during shear of two adsorbed surfactant monolayers in aqueous solution at

aloadofL = 4.5mNandT = 20

◦

C. The smooth sliding (open circles) to inverted stick–slip

(squares) transition occurs at v

∼ 0.3 µm/s. Prior to the transition, the kinetic stress levels

oﬀ at after a logarithmic dependence on velocity. The quasi-smooth regime persists up to the

transition at v

. At high driving velocities (ﬁlled circles), a new transition to a smooth slid-

ing regime is observed between 14 and 17 µm/s (after [342] with permission). (d) Friction

response of a thin squalane (a branched hydrocarbon) ﬁlm at diﬀerent loads and a constant

sliding velocity v = 0.08 µms

−1

, slightly above the critical velocity for this system at low

loads. Initially, with increasing load, the stick–slip amplitude and the mean friction force de-

crease with sliding time or sliding distance. However, at high loads or pressures, the mean

friction force increases with time, and the stick–slip takes on a more symmetrical, sinusoidal

shape. At all loads investigated, the stick–slip component gradually decayed as the friction

Physical Society)

The second equation is based on computer simulations [364]:

≈ 0.1



, (9.49)

where σ is a molecular dimension and m is the mass of the stage. Again, insert-

ing typical experimental values into this equation, viz., m ≈ 20 g, σ ≈ 0.5nm,and

−F

) ≈ 5mN as before, we obtain v

≈ 0.3µms

−1

, which is also close to meas-

ured values.

9 Surface Forces and Nanorheology of Molecularly Thin Films 491

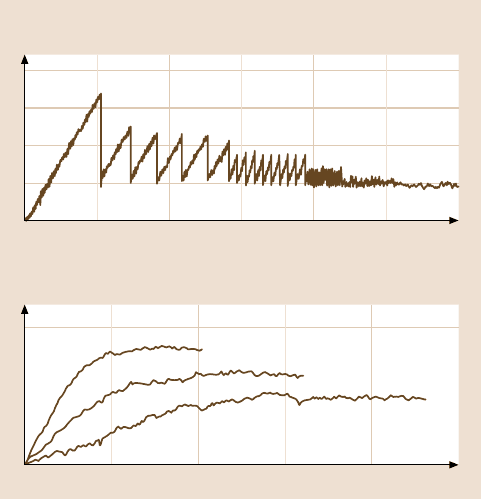
492 Marina Ruths and Jacob N. Israelachvili

Friction force F (mN)

3000

100 200

Time t (s)

100 Time t (s)500

Friction force F (mN)

Solid surfactant

v < v

v= 0.003 Pm/s

v= 0.1 Pm/s

v > v

v= 0.005 Pm/s

v= 0.0025 Pm/s

v= 0.001 Pm/s

Liquid surfactant

Fig. 9.31. (a) Exact reproduction of chart-recorder trace for the friction of closely packed

surfactant monolayers (L-α-dimirystoyl-phosphatidyl-ethanolamine, DMPE) on mica (dry

boundary friction) showing qualitatively similar behavior to that obtained with a liquid hex-

adecane ﬁlm (Fig. 9.30b). In this case, L = 0, v

≈ 0.1 µms

−1

, atmosphere: dry N

gas,

T = 25

◦

C. (b) Sliding typical of liquid-like monolayers, here shown for calcium alkylben-

zene sulfonate in dry N

gas at T = 25

◦

CandL = 0 (after [261], with permission; copyright

1993 American Chemical Society)

Stick–slip also disappears above some critical temperature T

, which is not

the same as the melting temperature of the bulk ﬂuid [285]. Certain correlations

have been found between v

and T

and between various other tribological pa-

rameters that appear to be consistent with the principle of time–temperature su-

perposition (see Sect. 9.8.3), similar to that occurring in viscoelastic polymer

ﬂuids [346,369,370].

Recent work on the coupling between the mechanical resonances of the sliding

system and molecular-scale relaxations [295, 338,341, 371] has resulted in a bet-

ter understanding of a phenomenon previously noted in various engineering appli-

cations: the vibration of one of the sliding surfaces perpendicularly to the sliding

direction can lead to a signiﬁcant reduction of the friction. At certain oscillation

amplitudes and a frequency higher than the molecular-scale relaxation frequency,

stick–slip friction can be eliminated and replaced by an ultralow kinetic-friction

state.

9 Surface Forces and Nanorheology of Molecularly Thin Films 493

9.9 Eﬀects of Nanoscale Texture on Friction

The above scenario is already quite complicated, and yet this is the situation for

the simplest type of experimental system. The factors that appear to determine the

critical velocity v

depend on the type of liquid between the surfaces, as well as on

the surface lattice structure.

9.9.1 Role of the Shape of Conﬁned Molecules

Small spherical molecules such as cyclohexane and OMCTS have been found to

have very high v

, which indicates that these molecules can rearrange relatively

quickly in thin ﬁlms. Chain molecules and especially branched-chain molecules

have been found to have much lower v

, which is to be expected, and such liquids

tendto slide smoothlyor witherraticstick–slip [345],rather thanina stick–slip fash-

ion (see Table 9.5). With highly asymmetric molecules, such as multiply branched

isoparaﬃns and polymer melts, no regular spikes or stick–slip behavior occurs at

any speed, since these molecules can never order themselves suﬃciently to solidify.

Table 9.5. Eﬀect of molecular shape and short-range forces on tribological properties

Liquid Short-range

force

Type of

friction

Friction

coeﬃcient

Bulk liquid

viscosity (cP)

Organic (water-free)

Cyclohexane Oscillatory Quantized

stick–slip

 10.6

OMCTS

Oscillatory Quantized

stick–slip

 12.3

Octane Oscillatory Quantized

stick–slip

1.50.5

Tetradecane Oscillatory

↔ smooth

stick–slip

↔ smooth

1.02.3

Octadecane (branched) Oscillatory

↔ smooth

stick–slip

↔ smooth

0.35.5

PDMS

(M = 3700 gmol

−1

, melt)

Oscillatory

↔ smooth

Smooth 0.450

PBD

(M = 3500 gmol

−1

, branched)

Smooth Smooth 0.03 800

Water

Water (KCl solution) Smooth Smooth 0.01−0.03 1.0

For molecularly thin liquid ﬁlms between two shearing mica surfaces at 20

◦

OMCTS: Octamethylcyclotetrasiloxane, PDMS: Polydimethylsiloxane,

PBD: Polybutadiene