Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001
Подождите немного. Документ загружается.


310
[2] U.G. Abdulla, On the Dirichlet problem for reaction-diffusion equations in non-smooth
domains. Nonlinear Analysis T.M.A., 47(2) (2001), 765-776.
[3] O.A. Ladyzhenskaya, V.A. Solonnikov and N.N. Uralceva, Linear and Quasilinear
Equations of Parabolic Type. American Mathematical Society, Providence RI, 1968.
[4] G.M.Lieberman, Second Order Parabolic Differential Equations. World Scientific,
1996.

Analysis of radiative transfer equation coupled with
nonlinear heat conduction equation
F.
Asllanaj
1
'
2
, G. Jeandel
1
, J.R. Roche
2
, D. Schmitt
2
1
LEMTA, Faculte des Sciences, B.P. 239, 54506,
Vandoeuvre les Nancy, France.
2
IECN, Faculte des Sciences, B.P. 239, 54506,
Vandoeuvre les Nancy, France.
Email : asllanaj@iecn.u-nancy.fr, roche@iecn.u-nancy.fr
Abstract
A model for coupled radiative-conductive heat transfer through a semi-transparent
medium is considered. The system under consideration has multiple practical ap-
plications, especially in thermal insulation. The existence and uniqueness results
are established for an anisotropically absorbing, emitting and scattering medium,
with axial symmetry and non homogeneous Dirichlet boundary conditions. The
well-posedness of the system in the steady case is investigated. The existence of
solutions is established applying Schauder's fixed point theorem. The uniqueness of
the solution in an appropriate functional space is obtained.
1 Introduction
In fibrous insulators used for domestic heating, the heat propagation is due to the radia-
tion and the conduction only and approximately one third of the heat transfer is achieved
by radiation. The forthcoming system (l)-(3) of radiative-conductive heat transfer equa-
tions is a generalisation of the system considered by Kelley [6], where the steady state is
studied. The medium is supposed to be independent of the wavelength (grey), isotropic
and the heat equation is linear. Our weaker physical assumptions result in new theoretical
difficulties which are solved in the paper. Let E be the medium thickness. The full system
of equations is written as follows :
o
a
fa, A) L° (T(x), A) - a
e
(
M
, A) L (x, fi, A)
+±J <r.(/i',A)POi'-fi,A)L(a:,M',.X)d/i'
W
V(s,/i,A) € (0,£) x
(-1,1)
x (0,oo),
311
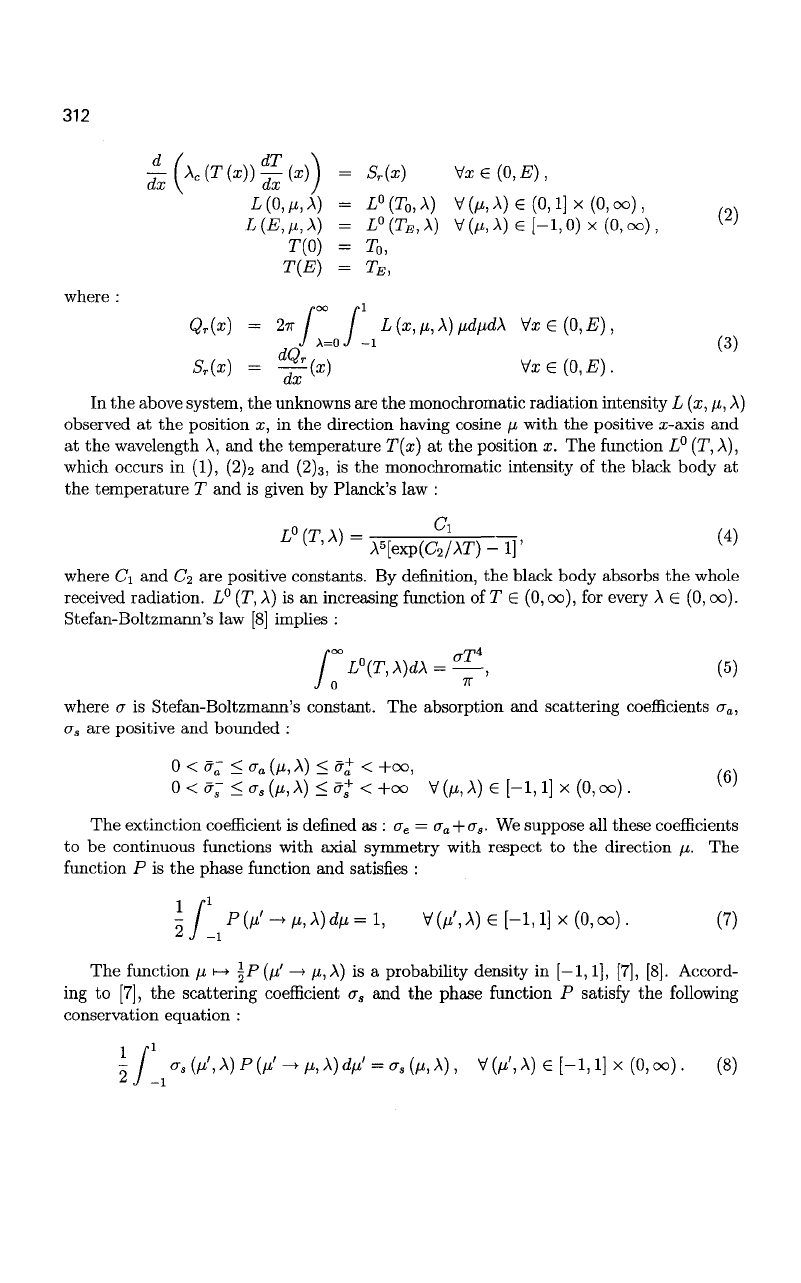
312
where
^A
c
(rW)fw) = S
r
(x) toe(0,£),
L(0,ii,X) = L°(T
0
,A) V(//,A)e(0,l]x(0,co),
L(E,fi,\)
= L°(T
E
,X) V(M,A)e[-l,0)x(0,oo),
T(0) = T
0
,
T(£) = TB,
/
OO
/*1
/ L{x,ii,X)ndfid\ Vxe(0,E),
x=o
J -1
5
r
(x) = ^(x) Vze(0,£).
ax
(2)
(3)
In the above system, the unknowns are the monochromatic radiation intensity
L(X,/J,,
X)
observed at the position x, in the direction having cosine fi with the positive x-axis and
at the wavelength A, and the temperature T(x) at the position x. The function L° (T, A),
which occurs in (1), (2)
2
and (2)
3
, is the monochromatic intensity of the black body at
the temperature T and is given by Planck's law :
L
°^
= A
5[
exp(cW-H' ^
where C\ and
C%
are positive constants. By definition, the black body absorbs the whole
received radiation.
LP
(T,
A)
is an increasing function of T G (0, oo), for every
A
6 (0, oo).
Stefan-Boltzmann's law [8] implies :
/
J i
rrT
4
L°(T,A)dA = —, (5)
0 T
where a is Stefan-Boltzmann's constant. The absorption and scattering coefficients a
a
,
a
a
are positive and bounded :
0 <a- <a
a
(/i,
A)
< a+ < +oo, . .
0 < a~ < a
s
(fj,,
A)
< a+ < +co
V
(p,
A)
€
[-1,1]
x (0, oo).
{
'
The extinction coefficient is defined as : a
e
= a
a
+
a
s
. We suppose all these coefficients
to be continuous functions with axial symmetry with respect to the direction /i. The
function P is the phase function and satisfies :
\J P(p'-*»,\)dn=l, V(//,A)e[-l,l]x(0,
(7)
The function ji
i—>
|P (//
—>
p,
A)
is a probability density in [—1,1], [7], [8]. Accord-
ing to [7], the scattering coefficient
<r
s
and the phase function P satisfy the following
conservation equation :
1
f
1
A)P(
M
'-
A
i,A)d/i' = ff,(/x,A), V(/i
,
,A)€[-l,l]x(0,oo). (8)
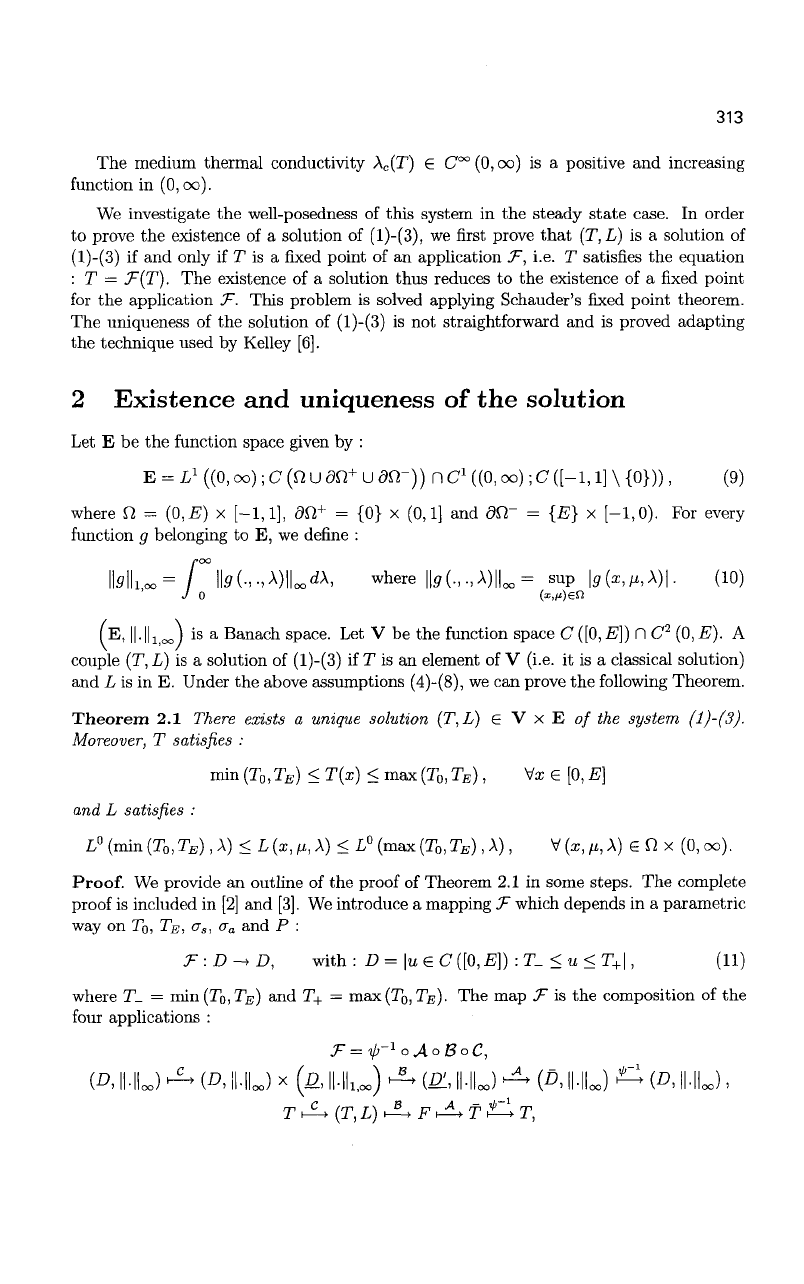
313
The medium thermal conductivity A
C
(T) £ C°° (0, oo) is a positive and increasing
function in (0,oo).
We investigate the well-posedness of this system in the steady state case. In order
to prove the existence of a solution of (l)-(3), we first prove that (T,L) is a solution of
(l)-(3) if and only if T is a fixed point of an application T, i.e. T satisfies the equation
: T = TIT). The existence of a solution thus reduces to the existence of a fixed point
for the application T. This problem is solved applying Schauder's fixed point theorem.
The uniqueness of the solution of (l)-(3) is not straightforward and is proved adapting
the technique used by Kelley [6].
2 Existence and uniqueness of the solution
Let E be the function space given by :
E
=
1}
((o,oo);
c (nu
0Q.+
u an-)) n c
1
((o,
oo)
;
c([-1,
i]
\ {o})), (9)
where Q = {0,E) x [-1,1], <9fi+ = {0} x (0,1] and <9fi" = {E} x [-1,0). For every
function g belonging to E, we define :
/
oo
\\g(.,.,\)\Ld\, where ||
S
(.,.,A)||
00
= sup |
5
(x
lA1
,A)|. (10)
o (x,/i)en
(E,
||.||
1]00
) is a Banach space. Let V be the function space C([0,E]) n C
2
(0, E). A
couple (T, L) is a solution of (l)-(3) if T is an element of V (i.e. it is a classical solution)
and L is in E. Under the above assumptions (4)-(8), we can prove the following Theorem.
Theorem 2.1 There exists a unique solution (T,L) e V x E of the system (l)-(3).
Moreover, T satisfies :
min (T
0
,T
E
) < T{x) < max (T
0
, T
E
), Vz 6
[0,
E]
and L satisfies :
L° (min (T
0
, T
E
) ,\)<L (x,
/J,
A)
< L° (max (T
0
, T
E
), A), V(i,(i,A)eilx (0, oo).
Proof. We provide an outline of the proof of Theorem 2.1 in some steps. The complete
proof is included in [2] and [3]. We introduce a mapping T which depends in a parametric
way on TQ, T
E
,
<T
S
,
a
a
and P :
F-.D-+D,
with: D= \u€C([0,E}) : T_ <u<T+\, (11)
where T_ = min(T
0
,T
B
) and T
+
= max(T
0
,T
E
). The map T is the composition of the
four applications :
(D,
\\.\U
^
{D,
!|.||J x
(D,
||.||
li00
) A
{DL,
||.||J 3,
(5,
||.|U &
(D,
\\.\\J ,

314
where L is the unique solution of (1), (2)
2
and (2)
3
, A(F) is the unique solution of the
forthcoming problem (14) and :
D = {g(x,
f
i,X)eL
i
((0,<x);L°°(Cl)):L
0
(T_,X)<g(x,fi,X)<L
0
(T
+
,X)}
U_ = {FeL
x>
{Q,E):Ma
a
L°{T
r
)<F<Ma
a
L
0
(T
+
)}
D = {ueC{[0,E]) :T_<u<?+}
/
u
\
c
(s)ds
o
/
OO pi
/
&
a
(/^
A)
L (x, //,
A) dftdX
/
OO />1
/ o-
a
{n,\)f{x,fi,\)diJ,d\.
(12)
We prove that the application T is completely continuous [8] from D to D and using
Schauder's fixed point theorem the existence of a solution is established.
Now, we give some intermediate results. For the details of the proofs, we refer the
reader to [2], [3].
Lemma 2.2 Let E £ (0, oo) and parameters T
0
, T
E
, a
a
, o
s
and P be given and assume
that all the above assumptions are satisfied. Let the functions H,
<po
and
<J>E
be given in
L
1
((0, oo); C (fi)), L
1
((0, oo); C ((0,1])) and L
1
((0, oo); C ([-1,0))), respectively. Then,
the following boundary value problem has a unique solution in the space E
H—(x,fi,\) + a
e
(iJ.,X)L(x,ii,\) = (AL)(x,n,\) + H(x,n,\)
V(x,At,A)enx(0,oo), (13)
L(x,n,X) = <t>
0
(fi,X) V(x,/x,A) e dQ
+
x (0, oo),
L(x,n,\) = 4>o{f-,X) V(x,/x,A) G 9f2~ x (0, oo),
where A is the linear integral operator defined for all
ip
6 L
1
((0, oo); L°° (O)) by:
1 f
1
(A<p){x,fj.,\) = - (7,(n',X)P(n'-*fi,X)ip(x,^',X)dfi', V(i,/i,A)€f!x(0,oo).
Proof of the Lemma
Let r be the map from (E, ||-||
loo
) —» (E,
||.||
1OO
)
defined as : L =
r(<p),
for
<p
€ E,
where L is the the solution of :
^~Q^{x,lJ.,X) + a
e
(fj,,X)L(x,iJ,,X) = (A<p)(x,/i,X) + H(x,fj,,X)
V(X,/J,A)
GOx
(0,oo),
L(x,n,X) = 4>o(n,X) V(x,/i,
A)
6 <9£2+ x (0, oo),
L(x,(i,X) =
<j>o(fJ;X) V
(a;,
/i,
A)
e 9f2~ x (0, oo),
For 0 < ^i < 1, we have :
L (x,
M)
A)
= - f
X
exp f- (x - y) ^tlR\
{Aip
+
H
) (y, p,
A)
dy
A*
y o v t
1
j
+
exp
(-z^^)
0o
(^ A).

315
Let tp and ip' in E be such that L = r{ip) and V = r (y/)- Using the expression of the
integral operator A we deduce :
|L(a:
)/
i
)
A)-L'(
a:
,/x,A)|<^^||
V
-^||
00
.
a
e
(fi,
A)
The same result can be obtained for \i = 0 and — 1 < /i < 0. Moreover, for every
(n,
A)
£
[—1,1]
x (0, oo) we have :
^
)
-l-
g
'M<c<l, c=l-- *»
Thus,
we deduce : \\L
—
L'Hj ^ < c
\\<p —
<p'\\i
:00
-
Therefore r is a strict contraction.
Moreover, for every
<p
€ E, L = r((p) € E. Indeed, defining for every A 6 (0, oo) :
G(A) = max( sup |0o(//,A)|, sup |0
B
(p,A)| ) ,
\0<I1<1
-l</i<0
/
then
||L||
li00
< (l - ^^+) llvlli.00 + ~rr llfflkoo + UGH! < 00
and L belongs to E. Finally, applying Banach's fixed point theorem, it follows that the
boundary value problem (13) has a unique solution in E. •
Remark 2.3 Under the assumptions of Lemma 2.2 and by recurrence, it is easy to prove
that the solution L of the boundary value problem (13) is an increasing function of
(j>o,
<J>E
and H.
Proposition 2.4 Let E G (0,00) and parameters T
0
, T
B
, a
a
, a
s
and P be given and
satisfying the assumptions (5)-(8). Let T G D be given. Then the problem (1), (2)2 and
(2)3 has a unique solution L
G
E.
Proof.
This result is a corollary of Lemma 2.2, setting :
H(x, n,
A)
=
<T
a
(jt,
\)L°(T(x),
A),
fo(/i, A) =
L°(T
0l
\),4>E(»,
A)
=
L°(T
E
,
A).
Clearly the definition of L° implies that H,
4>o
and
cj)
E
belong to L
1
((0,oo);C(Q)),
^((0,
00); C((0,1])) and ^((0,00); C([-l, 0))) respectively. Then, applying Lemma 2.2,
the problem (1), (2)
2
and (2)
3
has a unique solution in E. •
Remark 2.5 A corollary of Proposition 2.4 and of the previous Remark 2.3 is the fol-
lowing property :
L°(T_,A) < L{x,n,\) < L°(T+,\), V(x,/x,A) eflx (0,co)
and then L belongs to
D_.
Proposition 2.6 The map C is well defined and continuous from D-»D x
D_.
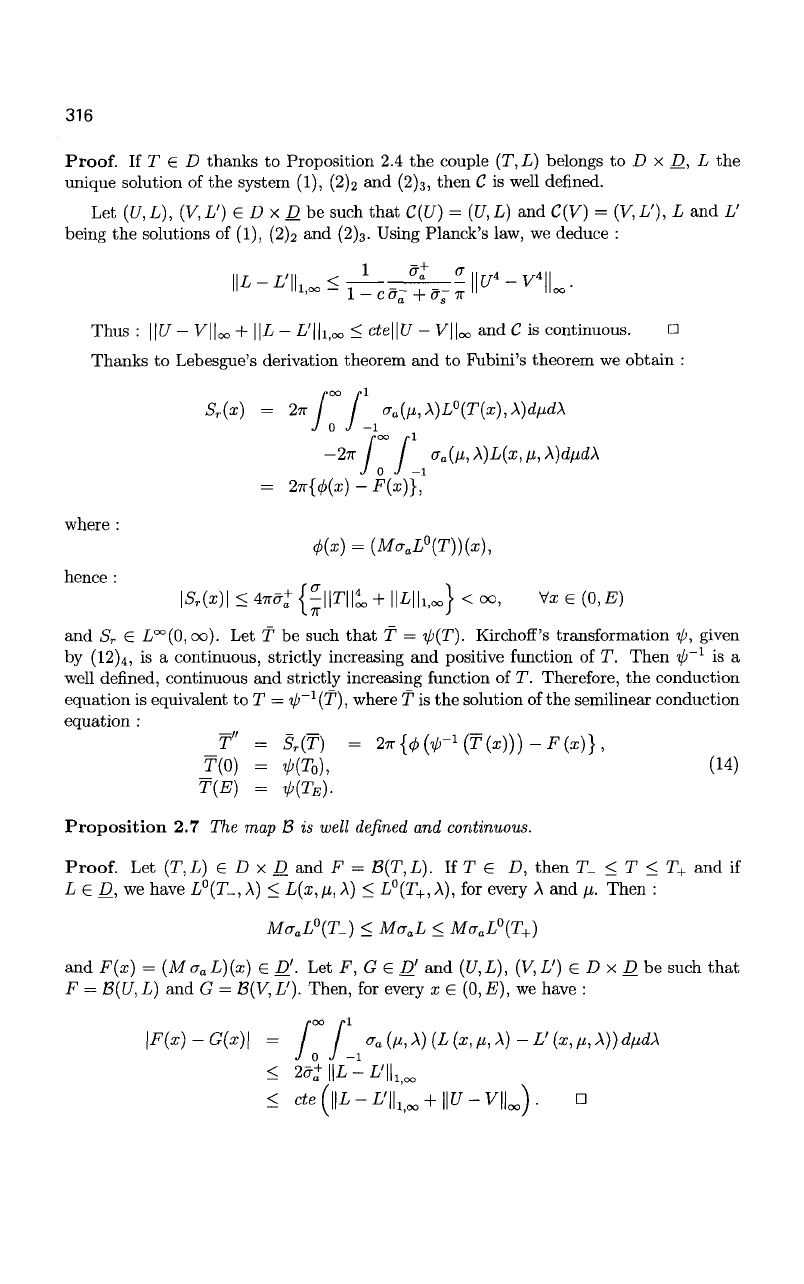
316
where :
hence :
Proof.
If T e D thanks to Proposition 2.4 the couple (T,L) belongs to D x D, L the
unique solution of the system (1), (2)
2
and (2)
3
, then C is well defined.
Let
(U,
L),
{V,
L')eDxDbe such that C{U) =
{U,
L) and C{V) = (V, L')>
L and L
'
being the solutions of (1), (2)
2
and (2)
3
. Using Planck's law, we deduce :
11
"
1
-°°-l-ci7- + i7,-7r
11 llo
°
Thus : \\U - V\\
x
+ \\L -
L'\\
hoo
< cte\\U - VW^ and C is continuous. •
Thanks to Lebesgue's derivation theorem and to Pubini's theorem we obtain :
/
oo pi
j a
a
(y,X)L°(T(x),X)dyd\
/
OO rl
/ a
a
(y,X)L(x,y,\)dyd\
=
2TT{4>(X)
-
F(x)}'
4>{x)
= (Ma
a
L°(T))(x),
\S
r
(
X
)\
<
4TT<7+
{JlTUt, + ||L||l,oo} < OO, VX £ (0,E)
and S
r
6 i°°(0, oo). Let T be such that T = ip{T). Kirchoff's transformation ip, given
by (12)
4
, is a continuous, strictly increasing and positive function of T. Then ip~
l
is a
well defined, continuous and strictly increasing function of T. Therefore, the conduction
equation is equivalent to T = ip~
l
(T), where T is the solution of the semilinear conduction
equation :
_T"
= S
r
(T) = 2,{^(ff(
I
)))-F(x)},
T(0) = V(7o), (14)
T{E) = i,(T
E
).
Proposition 2.7 The map B is well defined and continuous.
Proof.
Let (T,L) e D x D and F = B(T,L). If T e D, then T_ < T < T+ and if
L e D, we have L°(T_,
A)
< L(x, y,
A)
< L°{T+,
A),
for every
A
and y. Then :
Ma
a
L°{T_) < Ma
a
L < Ma
a
L°{T+)
and F(x) = {Ma
a
L)(x) e £'. Let F, G e jff and (U,L), (V,L') £ D x D be such that
F = £([/, L) and G = B{V, V). Then, for every x e (0, E), we have :
/
<x
a
(M,
A)
(L (x, y,
A)
- V (x, y,
A))
d/WA
< 2-ai \\L- L'||
li<x>
< ct
e
(||L-L'||
1)0O
+
||C/-^||
oo
).
D

317
Lemma
2.8
Under
the
previous assumptions,
the map B oC is an
increasing function
of
T
0
,T
E
andT.
Proof.
One has : F(x) = (B
o
C)
(T)(x)
= M (a
a
L) (x). In
Remark 2.3,
we
pointed
out
that
L is a
monotone increasing function with respect
to T
0
> TE and T. a
a
is a
positive
function
of y, and A.
Thanks
to the
positive linear operator
M the
function
B
o C
is
monotonous increasing with respect
to T
0
, TE and T. •
Now,
we
prove that
the
application
.A is
well defined
and
continuous with respect
to
the function
F.
Proposition
2.9 Let F 6 £>' be
given. Then,
the
boundary value problem
(14) has a
unique solution reflfl
W
2
'
p
(0, E) for
every
2 < p < +00 and
thus
the
application
A is
well i
Proof.
Let :
Ma
a
L\{r
l
{f^))-
i
T
+
-^
T
_
f
if?<?_,
$(f)
= {
Ma
a
L° (ip-
1
(f)) _ _ if ?_ < T < f+,
Ma
a
L°
(r
1
(f
+
)) + ^~r if f
>
f
+
.
Thanks
to the
definition
of M, a
a
and
L°(T),
we
remark that
$ is a
continuous
and
strictly increasing function
of T. If F
G
D'
then
F €
17(0,
E), for
every
p > 1 and :
$
(f_) < F(x) < $ (f+) , Vx 6
(0,
£).
Then, according
to Da
Prato's result [5],
the
boundary value problem
(14) has a
unique
solution
in W
2
'
p
(0, E), for
every
2 < p < +00. The
monotonicity
of $ and the
maximun
principle imply that
T_ < f < f'+ and
then
f e D n
W
2
'
p
(0,£). This means that
the
application
.4 is
well defined.
In order
to
prove
the
continuity
of the map A
with respect
to F, let F, G G £>' and
£/
=
.A(F),
V =
^t(G). Then,
the
difference
W = [/ - V is the
solution
of the
following
problem
:
_W" = 2rr
{$([/) -$(y)-(F-G)},
j^(0)
= 0,
W(£)
0.
Thanks
to the
monotonicity
of
<&,
we
obtain
:
||tV'||*<27r
J
E?||W||
oo
||F-G||
00
.
Using Sobolev's continuous embeddings
and
Poincare's inequality,
we
obtain
if W ^ 0
||iy|| <ctel|F-G|L.
•
II
Moo — " "°°
318
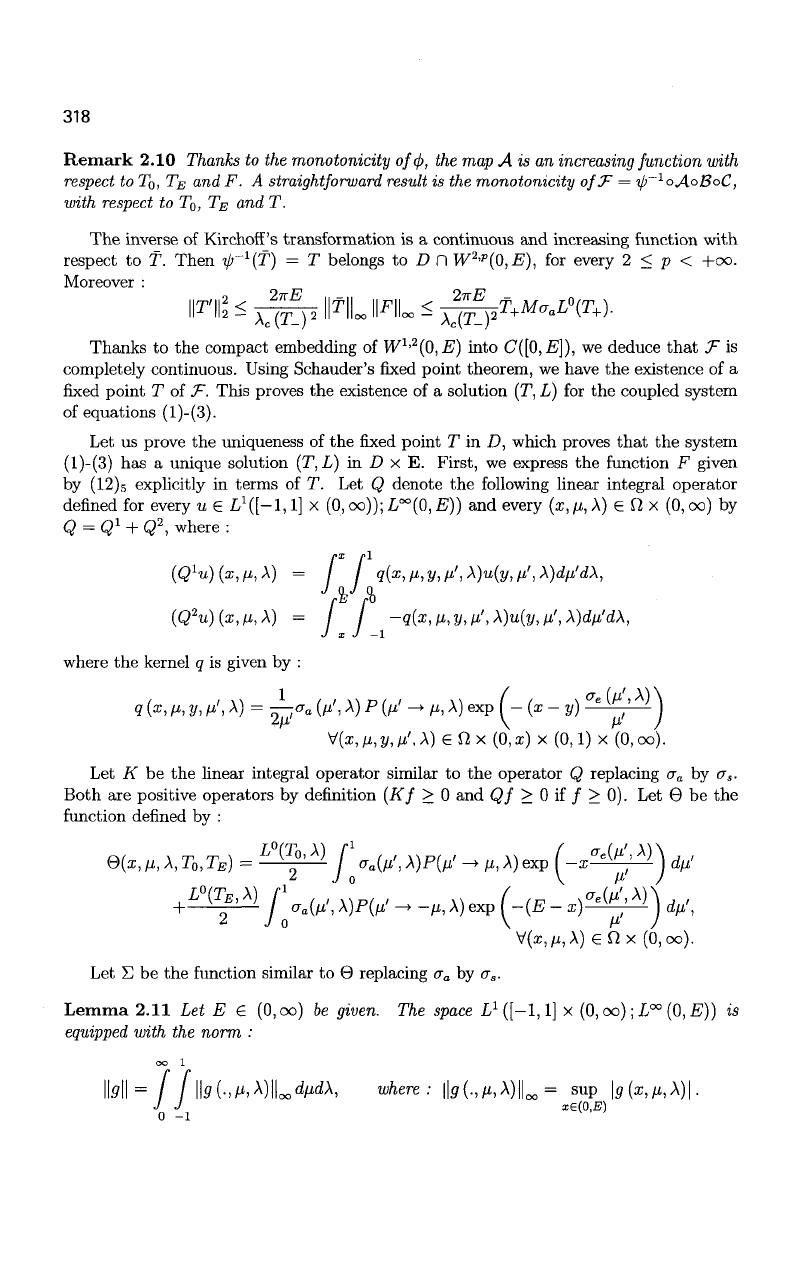
Remark
2.10
Thanks
to the
monotonicity
of<j>,
the
map
A is an
increasing function with
respect
to T
0
, T
E
and
F. A
straightforward result
is the
monotonicity of T
=
ip~
1
oAoBoC,
with respect
to
To,
TE and T.
The inverse
of
Kirchoff's transformation
is a
continuous
and
increasing function with
respect
to f.
Then
^{T) = T
belongs
to D n
W
2
'
P
{Q,E),
for
every
2 < p < +oo.
Moreover
:
Thanks
to the
compact embedding
of W
1,2
{0, E)
into C([0,E}),
we
deduce that
T is
completely continuous. Using Schauder's fixed point theorem,
we
have
the
existence
of a
fixed point
T of T.
This proves
the
existence
of a
solution (T,
L) for the
coupled system
of equations (l)-(3).
Let
us
prove
the
uniqueness
of the
fixed point
T in D,
which proves that
the
system
(l)-(3)
has a
unique solution
(T,L)
inflxE. First,
we
express
the
function
F
given
by
(12)
5
explicitly
in
terms
of T. Let Q
denote
the
following linear integral operator
defined
for
every
u €
L
1
^—1,1]
x
(0,00)); L°°(0,
E)) and
every
(x, n,
A)
€ fi x
(0,00)
by
Q
= Q
1
+ Q
2
,
where
:
(Q
1
u)(x,^,X)
=
q{x,fj,,y,
f
j,',X)u(y,fj.',X)d
f
i'dX,
J
ay 0.
/
E
pO
/ -q{x,iL,y,ii',\)u{y,ii',\)dii'd\,
where
the
kernel
q is
given
by :
q (x, n, y,
//,
A)
=
— a
a
(//,
A)
P (//
->
fj,,
A)
exp
( - (x - y) ^Jh— j
V(x,
/x,
y, //,
A)
e fi x
(0,
x) x (0,1) x
(0,00).
Let
K be the
linear integral operator similar
to the
operator
Q
replacing
a
a
by a
s
.
Both
are
positive operators
by
definition
{Kf > 0 and Qf > 0 if / > 0). Let © be the
function defined
by :
0(x,
11,
A,To,T
E
)
=
L
°
{T
°'
X)
f
o
o-.O*',
A)*V -
fi, A)exp ^-x^f^-^j
dfJ
,'
V(Z,/J,A)
e fix (0,00).
Let
E be the
function similar
to Q
replacing
a
a
by a
s
.
Lemma
2.11 Let E 6
(0,oo)
be
given.
The
space
L
1
([-1,1]
x
(0,oo); L°° (0,E))
is
equipped with
the
norm
:
00
1
llsll
= /
\\9(;V,X)\Ldnd\, where: \\g{.,fi,X)\\
00
= sup
\g{x,/j,,X)\.
J
J
x€(0,E)

319
Let parameters T
0
,TE,<J
a
,o
s
and P
be given satisfying
the
relations (4)-(8). Then,
the
operator
K is a
linear continuous
operator
from
the
space
L
1
([—1,1]
x
(0, oo); L°° (0,
E))
into itself and
I
—
K has a
bounded inverse operator
on
this space.
Proof.
Let g £
/^([-l,
1]
x
(0, oo); L°°(0,
E)) be
different from zero. Then
we
have
:
hLh"'^
\(Kg)(x,
fi
,X)\
< / /
—<7.(/i',A)P(/x'->/x,A)
x exp
I - (x - y) ^LR\ \\
g
(.,
^,
A)
|
L
dfj!dy
J
J
-Jjj°-W,X)PW-+l*,X)
xaxp(-(*-*)*<£*>)
HflO.Ai'.AJILd/i'dj,.
Using Pubini's theorem
and
integrating with respect
to y, we
obtain
the
following
inequality
:
1 f
1
<T.(M',A).
and then
\\(Kg)(x,n,\)\L<lJ
i
j|^y^(M'-M-A)ll5(.,M',A)||
00
d
M
'
ll(^)(^^A)||
00
<|y
1
i
P(M'-M,A)||
S
(.,
/
i',A)||
00
d
M
',
where
c = 1
—
a
a
~/
(a
a
+
+a
s
+
) satisfies
0 < c < 1 and is
independant
of
A. Then
an
integration
on
(n,
A)
e
[—1,1]
x
(0, oo) gives
:
||K"||
< 1.
Thus
I - K has a
bounded
inverse operator which
is
given
by the
Neumann series.
•
Now,
we can
express
S
r
explicitly
in
terms
of T. By
straightforward calculations,
we
verify that
:
S
T
{x)
=
2n{{Ma
a
L°(T)){x)
- F{x)}
=
2TTM{(J
- Q(7 - tf)~>a£
0
(T(x), A)
-Q(I
-
K)-
1
^,
n,
A,
T
0
,
T
B
) -
6(i,
p,
A,T
0
,
T
E
)}.
Lemma
2.12 Let g € L
1
(fi x
(0, oo)) be
a
positive function. Then
:
||((«-
+
Q)s)(.,.,A)||
Ll(nx(0iOo))
<
llz,
1
^)'
Proof.
Using
the
definition
of the
operators
K, Q and
Pubini's theorem,
we
have,
for
every A
€
(0, oo)
||((tf
+
Q)s)(.,.,A)||
Ll(n)
J ^=0
J
z=-oo
J
y=—ao
P \ P"
x l{o<
x
-
y
<E}9 (y, p,
A)
l{
0<y<E
}dydxdn
X1{-B<X-
V
<O}S
{y, ~M, A)
\{o
<y<E
)dydxdn
5(.,^,A)l
{0
<
y
<B}||
Ll(TC)
V
+
||fl
(-,-/*>
A)
1
{0
<„<B}||
LI
M
'
•t'(TC)
