Bemelmans J. et. al. (editor) Elliptic and Parabolic Problems: Rolduc and Gaeta 2001

Подождите немного. Документ загружается.

330

Theorem 3.7 (a.s. stabilizability). Assume that there exists a control Lyapunov func-

tion (Definition 3.2) continuous at 0 and the controlled system satisfies the convexity

condition (13). Then the null state X = 0 is almost surely stabilizable for (CS).

Remark 3.8. If the convexity condition (13) does not

hold,

we can use relaxed controls

defined,

e.g., by Haussmann and Lepeltier

[18].

The Nagumo-type geometric condition

(12) turns out to be equivalent to the viability of K by means of relaxed controls, see

Corollary 2 in

[8].

Therefore we can show that the existence of

control Lyapunov function

continuous at 0 implies that the null state X = 0 is almost surely stabilizable by means

of relaxed controls. All the details, extensions and variants of this result will appear in a

forthcoming paper.

References

[1] J.P. Aubin, Viability theory. Birkhauser, Basel, 1991.

[2] J.P. Aubin, G. Da Prato, Stochastic viability and invariance. Ann. Sc. Norm. Sup.

Pisa (IV) 17 (1990), 595-613.

[3] J.P. Aubin, G. Da Prato, The viability theorem for stochastic differential inclusions.

Stochastic Anal. Appl. 16 (1998), 1-15.

[4] J.P. Aubin, G. Da Prato, Stochastic Lyapunov method. NoDEA Nonlinear Differen-

tial Equations Appl. 2 (1995), 511-525.

[5] A. Bacciotti, L. Rosier, Liapunov functions and stability in control theory. Lecture

Notes in Control and Information Sciences 267, Springer-Verlag, London, 2001.

[6] M. Bardi, I. Capuzzo-Dolcetta, Optimal control and viscosity solutions of Hamilton-

Jacobi-Bellman equations. Birkhauser, Basel, 1997.

[7] M. Bardi, P. Goatin, Invariant sets for controlled degenerate diffusions: a viscosity

solutions approach. In "Stochastic Analysis, Control, Optimization and Applications:

A Volume in Honor of W.H. Fleming", W.M. McEneaney, G.G. Yin and Q. Zhang

eds.,

pp. 191-208, Birkhauser, Basel, 1999.

[8] M. Bardi, R. Jensen, A geometric characterization of viable sets for controlled de-

generate diffusions. Preprint 15, Univ. di Padova, 2001.

[9] G. Barles, B. Perthame, Discontinuous solutions of deterministic optimal stopping

time problems. RAIRO Model. Math. Anal. Numer. 21 (1987), 557-579.

[10] E.N. Barron, R. Jensen, Semicontinuous viscosity solutions of Hamilton Jacobi equa-

tions with convex Hamiltonians. Comm. Partial Differential Equations, 15 (1990),

1713-1742.

[11] R. Buckdahn, S. Peng, M. Quincampoix, C. Rainer, Existence of stochastic control

under state constraints. C. R. Acad. Sci. Paris Ser. I Math. 327 (1998), 17-22.

331

[12] F.H. Clarke, Yu. Ledyaev, R.J. Stern, RR. Wolenski, Nonsmooth analysis and control

theory. Springer-Verlag, New York, 1998.

[13] M.C. Crandall, H. Ishii, P.L. Lions, User's guide to viscosity solutions of second order

partial differential equations. Bull. Amer. Math. Soc. 27 (1992), 1-67.

[14] W.H. Fleming, H.M. Soner, Controlled Markov Process and Viscosity Solutions.

Springer-Verlag, New York, 1993.

[15] P. Florchinger, Feedback stabilization of affine in the control stochastic differential

systems by the control Lyapunov function method. SIAM J. Control Optim. 35

(1997),

500-511.

[16] W. Hahn, Stability of motion. Springer-Verlag, New York, 1967.

[17] R.Z. Has'minskii, Stochastic stability of differential equations. Sjithoff and Noordhoff

International Publishers, 1980.

[18] U.G. Haussmann and J.P. Lepeltier, On the existence of optimal controls, SIAM J.

Control Optim. 28 (1990), 851-902.

[19] M. Kocan, P. Soravia, Lyapunov functions for infinite-dimensional systems. J. Funct.

Anal, to appear.

[20] H.J. Kushner, Stochastic stability and control. Academic Press, New York, 1967.

[21] P.-L. Lions, Optimal control of diffusion processes and Hamilton-Jacobi-Bellman

equations. Part 1: The dynamic programming principle and applications, Part 2:

Viscosity solutions and uniqueness. Comm. Partial Differential Equations 8 (1983),

1101-1174 and 1229-1276.

[22] E.D. Sontag, Stability and stabilization: discontinuities and the effect of disturbances.

In "Nonlinear analysis, differential equations and control" (Montreal, QC, 1998), F.H.

Clarke and R.J. Stern eds., pp. 551-598, Kluwer Acad. Publ., Dordrecht, 1999.

[23] E.D. Sontag, H.J. Sussmann, Non smooth control Lyapunov functions. Proc. IEEE

Conf.

Decision and Control, New Orleans, Dec 1995, IEE Publications, 1995.

[24] P. Soravia, Stability of dynamical systems with competitive controls: the degenerate

case.

J. Math. Anal. Appl. 191 (1995), 428-449.

[25] P. Soravia, Feedback stabilization and H-infinity control of nonlinear systems affected

by disturbances. In Proceedings of the Third Nonlinear Control Network Workshop

"Dynamics, Bifurcations and Control", Springer Lecture Notes in Control and Infor-

mation Sciences, to appear.

[26] J. Yong, X.Y. Zhou, Stochastic controls, Springer-Verlag, New York, 1999.

[27] J. Yorke, Differential inequalities and non-Lipschitz scalar functions. Math. System

Theory 4 (1970), 140-153.

Mass transport through charged membranes

Diether Bothe

and Jan Prtiss

Fachbereich Mathematik und Informatik

Universitat-GH Paderborn War burger Str. 100

D-33098 Paderborn, Germany

Fachbereich Mathematik und Informatik

Martin-Luther-Universitat Halle-Wittenberg

Theodor-Lieser Str. 5, D-60120 Halle

Email : dieterb@uni-paderborn.de ;

anokd@volterr a. mathematik. uni-halle. de

Abstract

A modern technique for desalination or softening of water is the so-called nanofil-

tration by means of membranes which carry a fixed electric charge. For the mathe-

matical modeling of such processes, two features are particularly important. Firstly,

the distribution of ionic species generates an electric field which in turn affects the

fluxes of these ions. Therefore, besides diffusion and convection, electromigration

has to be taken into account. This also leads to a strong coupling of the concen-

trations of all charged species, which can often be adequately incorporated into

the model via the assumption of electroneutrality. Secondly, electrical double-layers

(Donnan potential) build up at the surface of the membrane, which cause disconti-

nuities in the ionic concentration profiles.

We deduce a mathematical model for such a nanofiltration process. This leads to

a strongly coupled quasilinear parabolic system with nonlinear transmission and

dynamical boundary conditions. By means of degree theory we obtain existence of

stationary solutions, while L^-maximal regularity is employed to get local strong

wellposedness of this model.

1 Introduction

Nanofiltration is a pressure driven separation process that employs specific membranes

consisting of a polymeric matrix which carries fixed ionic groups. If the void space of such

a membrane is filled in with an aqueous solution, these groups dissociate by separation

of mobile ions like protons or hydroxide ions. Since the latter enter the aqueous phase,

a fixed electric charge inside the polymeric matrix remains. Due to the fact that ionic

solutions are electrically neutral on the macroscopic scale, this leads to a considerable

change of the composition inside the membrane, compared to the adjacent bulk phases.

332

333

This effect forms the heart of nanofiltration processes and allows for the selective removal

of ionic species with multiple charges like calcium, magnesium, sulfate, phosphate etc.

For technically relevant nitration processes sufficiently high flow rates across the mem-

brane are necessary which require a large surface of the membrane. A common way to

achieve this within a reasonable volume is to pile up several membranes in so-called disc-

tube modules. Such modules are constructed in such a way that the feed solution flows

tangentially along one membrane after the other. A certain amount of the solution is

pressed through the membrane to yield the desired permeate, while the remaining fluid

collects the rejected ions and leaves the system as so-called retentate with higher con-

centrations. By means of this cross-flow operation, blockage of the membrane due to

precipitation is avoided. Still, a thin film of microorganism will settle down on the sur-

face of the membrane a problem present in any aqueous system. This fouling slows down

mass transport and leads to increased concentrations of ions inside the permeate phase.

In fact, the rejection rate then decreases for large pressure differences.

Concerning the intensification of such nanofiltration processes, a fundamental un-

derstanding based on mathematical models is essential. For more detailed information

concerning the technical background see [6] and in particular [5].

2 Mathematical modeling

We develop a mathematical model that incorporates the relevant mass transport phenom-

ena mentioned above, while appropriate simplifications concerning the hydrodynamics are

introduced. To be specific, we suppose the bulk phases containing retentate or permeate

to be ideally mixed and assume stationary hydrodynamics. The latter implies

V' = V

+ V

where V' denotes the volumetric flow rate for j = f (feed), R (retentate) or P (permeate),

respectively.

We consider a single planar membrane carrying a feed-sided film inside of which the

species have reduced mobility. The latter represents a combination of the biofilm men-

tioned above and a hydrodynamic boundary layer (the so-called Nernst boundary layer).

Since the concentrations inside ideally mixed bulk phases are homogeneous, mass trans-

port across the membrane is solely oriented in the normal direction as illustrated by

Figure 1.

Consequently, the mathematical model involves only one spatial dimension. Mass

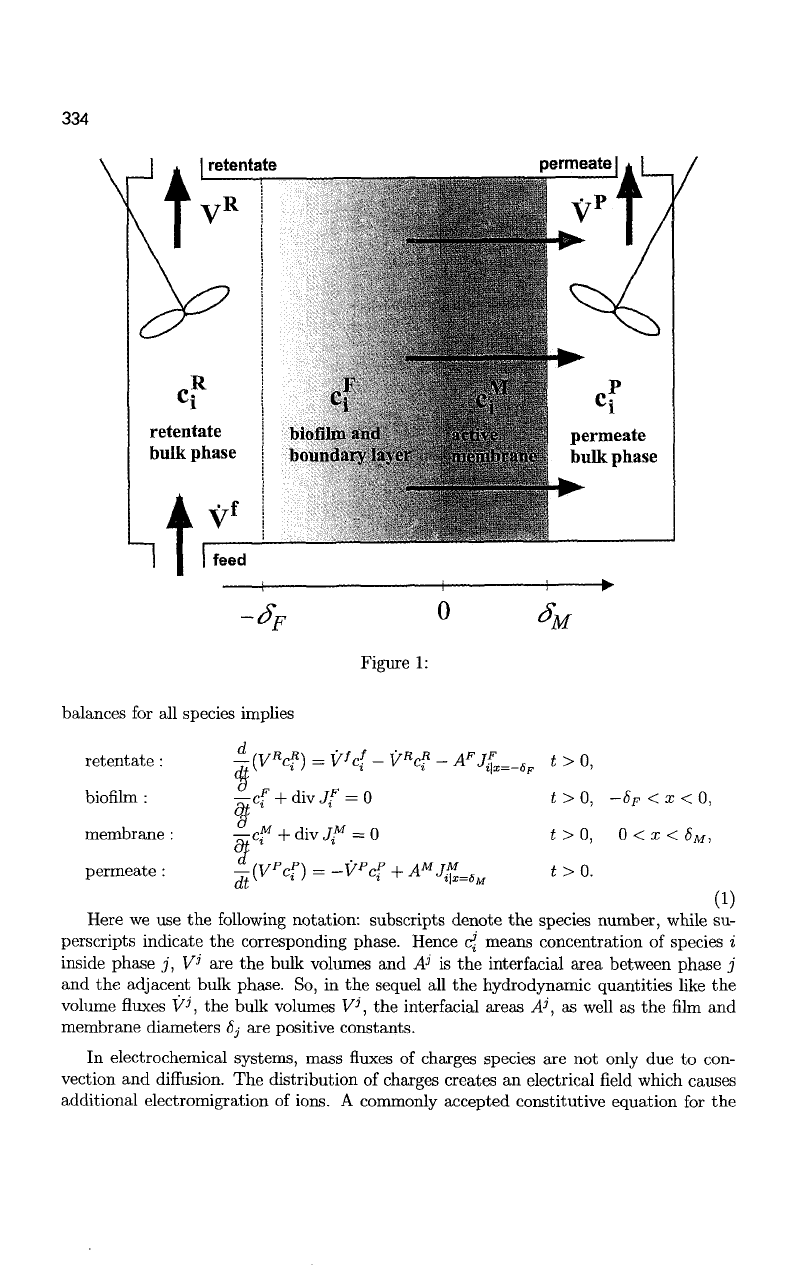
334

retentate

permeate]

retentate

bulk phase

feed

«-"l

biofilni .mil

bouml.i^ I'-iM'i'

acti\i'

membrane

permeate

bulk phase

Figure 1:

balances for all species implies

retentate :

biofilm :

membrane :

permeate :

^-{V

cf) = V

c{ -

- A

•

div Jf 0

t>0,

t >0,

cf + div J

= 0

(Vc?) = -

(

A"JM

=SM

-S

< x < 0,

t > 0, 0 < x <

t > 0.

(1)

Here we use the following notation: subscripts denote the species number, while su-

perscripts indicate the corresponding phase. Hence c^ means concentration of species i

inside phase j, V^ are the bulk volumes and Ai is the interfacial area between phase j

and the adjacent bulk phase. So, in the sequel all the hydrodynamic quantities like the

volume fluxes V

, the bulk volumes V\ the interfacial areas A

, as well as the film and

membrane diameters Sj are positive constants.

In electrochemical systems, mass fluxes of charges species are not only due to con-

vection and diffusion. The distribution of charges creates an electrical field which causes

additional electromigration of ions. A commonly accepted constitutive equation for the

335

fluxes (see [7]) is given by

-Vt%

J4-^M%

= F

), (2)

where D\ > 0 denote the diffusivities, v

the convective speed of the solvent,

the charge

numbers and

<jP

the electric potential. Furthermore F is the Faraday constant, R the

universal gas constant and T the absolute temperature. Let us note in passing that the

Nernst-Einstein equation, relating the mobility of ions to their diffusivity, has already

been incorporated into (2) for technical simplicity.

To obtain a closed model, an equation for the electric potential has to be added. If

electrodynamical effects are neglected, a reasonable choice would be the Poisson equation

F "

A0»'

= —

-5>c*,

where e is the permittivity of the electrolyte solution. Unfortunately, accurate boundary

conditions for

<jp

are hard to get and e depends on the composition of the electrolyte in a

complicated, often unknown

way.

On the other hand, on the macroscopic scale the solution

is electrically neutral to a high degree of accuracy inside bulk phases. Mathematically this

corresponds to the fact that F/e has a very large value. Therefore we add the condition

of electroneutrality, i.e. the algebraic constraint

Equation (3) refers to membranes with fixed negative charges in which case

eft

denotes

an equivalent concentration of negative ions with charge number

-1.

Finally, the model has to be complemented by boundary and transmission conditions.

These are continuity of the fluxes and continuity of the electrochemical potentials. While

the former directly translates into

Jf(0) = jf(0) forz =

l,...,n,

(4)

the latter needs some more explanation. The electrochemical potential fa of a species i is

given by

fi^rf + RTlnai + ZiFfr

where fi° is the chemical standard potential and a

{

is the activity. For dilute solutions,

<jj can be replaced by the concentration

which will be done in the sequel. At interfaces

where

<j>

is continuous, this implies continuity of the concentrations, i.e.

cf = cf(~S

) fori =

l,...,n.

(5)

The same cannot be true at the surfaces of the membrane, since this would not be con-

sistent with electroneutrality. At the film-sided interface, continuity of the electrochemical

potential yields

In cf (0) + {fti^iO) = In cf (0) + ^i<t>

(0),

336

hence

cf (0) = cf

(O)e^

with a = ^(^(0) - ^

(0)).

Electroneutrality inside the membrane yields

f>cf (0)e<-= cf,

which uniquely defines a for all compositions such that cf (0) > 0 for some i with

^ 0;

notice that tp(a) = Yl"=i

ZiCie

aZi

is strictly increasing such that lim

-,±oo

il>(a)

= ±°o-

Observe also that a ^ 0 in case cf / 0 which is physically comprehensible: due to the

fixed negative charge density inside the membrane, an electrical double layer builds up

at the surface. On the macroscopic scale this leads to a discontinuity of the electric

potential which causes a jump in the concentration profiles of charged species. The

difference

(j>

(0) —

</>

(0) is the so-called Donnan potential. Since the same arguments

apply at the interface between membrane and permeate, we obtain the conditions

cf (0) = cf (0) gf

(0)),

cf (6

) = cf gf(c

) (6)

with function gf ,gf implicitly given by

gf(c) = e"" O J2

- 9f(c) =

ef">

*• £ z

lCi

» = cf. (7)

In summary, the complete model has the form of a partial differential-algebraic system

consisting of the balance equations (1) with fluxes given by (2), the transmission and

boundary condition (4), (5), (6) and the algebraic condition (3) of electroneutrality.

There are only few papers about electrochemical systems in the mathematical litera-

ture.

While results for related problems from electrolysis can be found in [1], [2], [8] and

[9],

rigorous investigations of membrane processes including the appearance of Donnan

potentials are apparently missing.

3 The stationary problem

In the stationary case the transport equations in (1) reduce to div j{ = 0, hence by

continuity of the mass fluxes and (1), they satisfy

jf{x) = v

cf {-S

<x<0), J

(x)=v

cf {0<x<S

)

with v

Then (2) yields

_^+!M_^JL^

FM]

j A

RTdx D

Multiplication with z

and summation over all species, accounting for electroneutrality,

gives

F fd4?\ A

, A v^4-v

cf ,

337

This allows for elimination of the electrical field from the mass fluxes. Together with

the remaining retentate balance as well as (5) and (6), we obtain the following boundary

value problem. For clarity, we use the abbreviations

'= (ci>-">4)i

D_j

=diag(l/dj,...,l/4), z = (z

... ,z

) Z = diag(*i,... ,z

With this condensed notation, the stationary problem reads as

(8)

r)c

h Ti~

(i)

- v

-h =

-^)-

(

t,W

ZcM

<X<6M

with boundary and transmission conditions

( — g„)

c"(0) = c^(0)-g^(0)),

) = c

(c^ (9)

+ ~V

where a dot stands for componentwise multiplication.

Theorem 3.1 For a given nonnegative and electroneutral feed composition c?, the sta-

tionary problem (8), (9) has a nonnegative classical solution.

Proof.

If the feed contains no charged species, then the problem is a purely diffusional

one.

This case is much simpler and will not be discussed here. Hence we assume that

q > 0 for some index i with Zj ^ 0.

We reduce to a fixed-point problem, where it is favorable to solve the differential

equations backwards, since c

is involved in the boundary condition and appears on the

right-hand sides in (8).

Given any permeate composition c

> 0 such that (z,c

) = 0 and cf > 0 for some i

with

^ 0, the permeate-sided membrane concentration

(<5M)

is uniquely determined

by (9). Notice that c

(<5

) > 0 depends continuously on c

with cf

(6M)

> 0 iff cf > 0.

For this initial value, the second ODE-system in (8) has a unique backward solution up

to x = 0. Indeed, the right-hand side is locally Lipschitz continuous with at most linear

growth in c

. Hence a unique local solution exists as long as (z, Zc

) > 0. The latter

holds on all of

[Q,6

]

if the solution stays nonnegative, since (z,c

(fi

)) = cf together

with —

(z,

) = 0 implies

£^f <£Ncf<£^,

338

The solution stays in fact nonnegative, since c

) > 0 and cf{x

) = 0 imply

P P

The same argument yields cf*(0) > 0 iff cf > 0.

To pass to the film, we rewrite the transmission condition at x = 0 as

f (0) =

M{0)e-

aZi

with a such that

(0)

aZi

= 0.

Therefore c

> 0 continuously determines a unique c

(0) > 0 such that (z, 0^(0)} = 0

and cf (0) > 0 iff cf > 0. A similar reasoning as given above shows that the first ODE-

system in (8) with this initial value has a unique backward solution up to x = —

6p-

Here

)

—

X)"=i

f (

) satisfies

hence the solution exists up to x = —8p and c

(—6p) > 0 iff cf > 0.

Let F(c

) denote the vector c

(—SF)

determined by c

this way. To sum up,

F:D{F) = En{ceWl:{z,Zc)>0}-*Enm.l with E = {c £ R" :

(z,

c) = 0} (10)

is continuous with Fj(c) > 0 iff

> 0. Moreover, the above constructed solutions evidently

solve the stationary problem (8), (9) iff c.

satisfies

^(t

f-^F

)).

(11)

We shall apply degree theory to obtain a solution of this fixed-point problem, where

we work in the Banach space E (with E"-norm). Let

„,

c =

-.—

G(c) = c T- F(c)

and

= {c e E : 0 <

< c

y^ c

> rj},

where

> 0 is to be chosen later. Evidently O is open bounded convex and G is continuous

on H. To obtain existence of a fixed-point of G we shall use homotopy invariance of the

degree (cf. [3]) to pass to a simpler problem. For this purpose we define a homotopy

H(c

,\) as follows. Replace

D>byDt(\) = (l-\) + XDi ij = F,M),

v>{\)

= (1

X)v

\v>

(j = F, M),

339

Given

€ [0,1] and c

> 0, repetition of the above arguments yields a corresponding

which gives F(c

:= c

. We then define the homotopy H by

H(c

,\)=c--

^F(c

,X).

Obviously H(-, •) is continuous due to the continuous dependence of the solutions of

(8) on the parameters and initial values. We claim that H(-,

has no fixed-point on dfl

> 0 is sufficiently small. Assume that H(c

= c

for some

€ [0,1] and c

6 0.

If c

£ dQ then there are three possible cases.

(i) There exists some i such that c

= 0. Then Fi(c

= 0 yields the contradiction

Hi(c

,X) =Ci> c

(ii) There exists some i such that c[ = c

. Then Fi(c

> 0 yields the contradiction

,\)=c

-F

,\)<c

(iii) The remaining case 2

Sfc

V needs some preparation. Below we use the ab-

breviations

z£ = max{z

,0}, z

= max{-z

,0}, z+

= max* z

, z^

= ma,x

(~z

= max{v

= min{l,D{,... ,D{};

observe that D\

(A)

holds for any

[0,1].

Let

X^ f

P=^> Ci > 0,

= — > 0,

notice that

= X

(°

)

and recall (z,c

) = 0. Hence

<"?

= X

k< X

+ X

^ X ^ +

m«)

Zfc5^0 Zfc>0 z

<0 z

shows that there exists some i such that

* < 0 and cf = cf(-tf

) >

ail

_ . (12)

We fix this index i and use differential inequalities to get the contradiction cf >

for