Articolo G.A. Partial Differential Equations and Boundary Value Problems with Maple V
Подождите немного. Документ загружается.

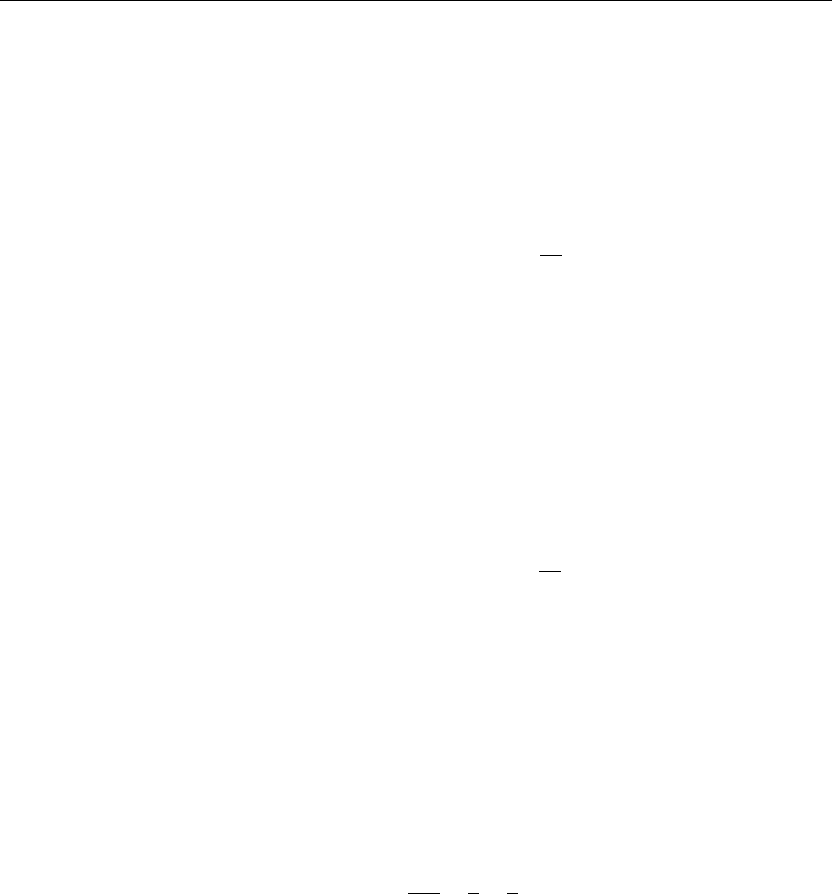
398 Chapter 6
6.8. Boundaries x = 0,x= 2, y = 0 are held at fixed temperature 0, boundary y = 1 is losing
heat by convection into a surrounding medium at 0 temperature, k = 1/10:
u(0,y,t)= 0 and u(2,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)+u(x, 1,t)= 0
Initial condition:
f(x, y) = x(2 −x)y
1 −
2y
3
6.9. Boundaries x = 1,y= 0 are held at fixed temperature 0, boundary x = 0 is insulated,
and boundary y = 1 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10:
u
x
(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)+u(x, 1,t)= 0
Initial condition:
f(x, y) = (1 −x
2
)y
1 −
2y
3
6.10. Boundaries x = 1, y = 0 are held at fixed temperature 0, boundary y = 1 is insulated,
and boundary x = 0 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10:
u
x
(0,y,t)−u(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial condition:
f(x, y) =
−
2x
2
3
+
x
3
+
1
3
y(1 −y)
6.11. Boundaries x = 0, y = 1 are held at fixed temperature 0, boundary y = 0 is insulated,
and boundary x = 1 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10:
u(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,t)= 0
u
y
(x, 0,t)= 0 and u(x, 1,t)= 0

The Diffusion Equation in Two Spatial Dimensions 399
Initial condition:
f(x, y) = x
1 −
2x
3
(1 −y
2
)
6.12. Boundaries y = 0,y = 1 are held at fixed temperature 0, boundary x = 0 is insulated,
and boundary x = 1 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10:
u
x
(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,t)= 0
u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial condition:
f(x, y) =
1 −
x
2
3
y(1 −y)
6.13. Boundaries x = 0,x= 1 are held at fixed temperature 0, boundary y = 1 is insulated,
and boundary y = 0 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10:
u(0,y,t)= 0 and u(1,y,t)= 0
u
y
(x, 0,t)−u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial condition:
f(x, y) = x(1 −x)
1 −
(y −1)
2
3
6.14. Boundaries x = 0,x= 1, and y = 0 are insulated and boundary y = 1 is losing heat by
convection into a surrounding medium at 0 temperature, k = 1/10:
u
x
(0,y,t)= 0 and u
x
(1,y,t)= 0
u
y
(x, 0,t)= 0 and u
y
(x, 1,t)+u(x, 1,t)= 0
Initial condition:
f(x, y) = x
2
1 −
2x
3
1 −
y
2
3
6.15. Boundaries x = 1 and y = 0 are both insulated and boundaries x = 0 and y = 1 are
losing heat by convection into a surrounding medium at 0 temperature, k = 1/10:
u
x
(0,y,t)−u(0,y,t)= 0 and u
x
(1,y,t)= 0
u
y
(x, 0,t)= 0 and u
y
(x, 1,t)+u(x, 1,t)= 0
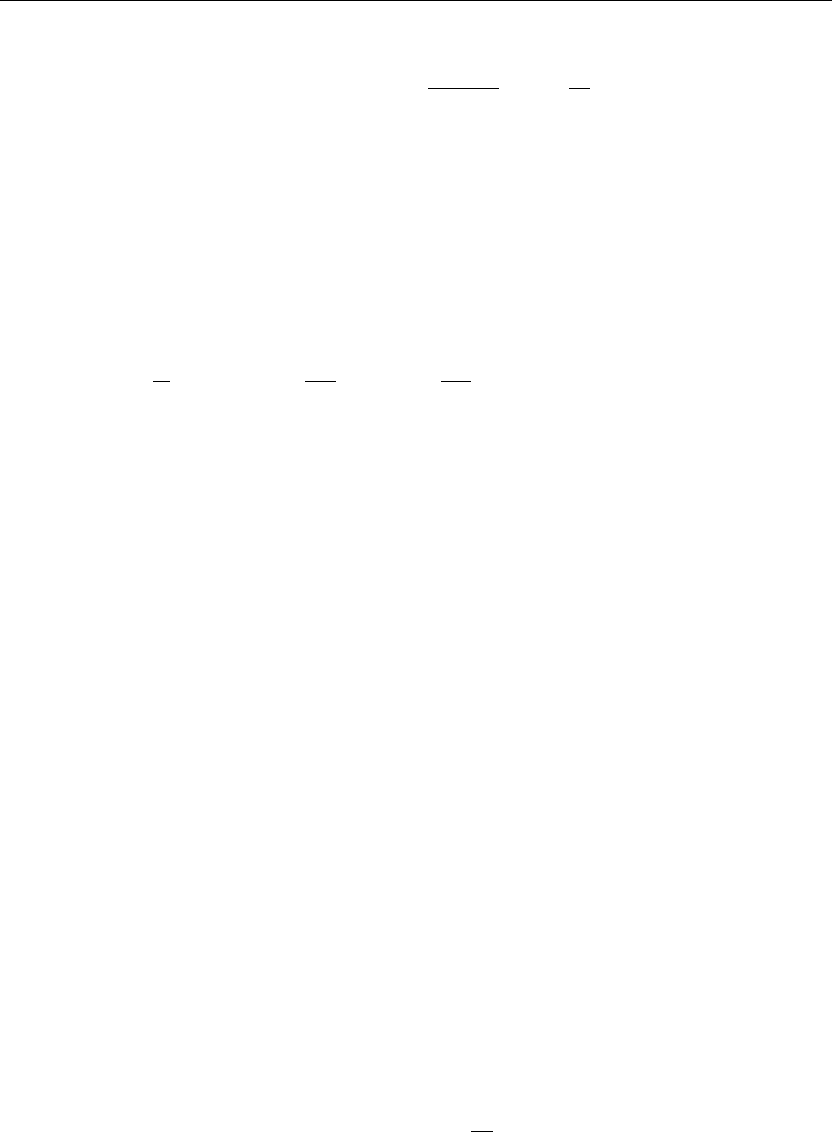
400 Chapter 6
Initial condition:
f(x, y) =
1 −
(x −1)
2
3
1 −
y
2
3
Example Exercises with Surface Heat Loss
We now consider the temperature distribution in thin plates over the two-dimensional domain
D ={(x, y) |0 <x<1, 0 <y<1} whose lateral surfaces are no longer insulated but are now
experiencing a heat loss proportional to the plate temperature and the surrounding temperature
medium at zero degrees. If we let β account for the heat loss coefficient, then the homogeneous
partial differential equation is
∂
∂t
u(x, y, t) = k
∂
2
∂x
2
u(x, y, t) +
∂
2
∂y
2
u(x, y, t)
−βu(x,y,t)
6.16. Boundaries x = 0,x= 1, y = 0,y= 2 are held at fixed temperature 0, k = 1/10,
β = 1/5:
u(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u(x, 2,t)= 0
Initial condition:
f(x, y) = x(1 −x)y(2 −y)
6.17. Boundaries x = 1, y = 0,y= 1 are held at fixed temperature 0, boundary x = 0is
insulated, k = 1/10, β = 1/5:
u
x
(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial condition:
f(x, y) = (1 −x
2
)y(1 −y)
6.18. Boundaries y = 0,y= 1 are held at fixed temperature 0, boundaries x = 0 and x = 1 are
insulated, k = 1/10, β = 1/5:
u
x
(0,y,t)= 0 and u
x
(1,y,t)= 0
u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial condition:
f(x, y) = x
2
1 −
2x
3
y(1 −y)

The Diffusion Equation in Two Spatial Dimensions 401
6.19. Boundaries x = 1, y = 0 are held at fixed temperature 0, boundaries x = 0 and y = 1 are
insulated, k = 1/10, β = 1/5:
u
x
(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial condition:
f(x, y) = (1 −x
2
)y
1 −
y
2
6.20 Boundary y = 0 is held at fixed temperature 0, boundaries x = 0,x= 1, and y = 1 are
insulated, k = 1/10, β = 1/5:
u
x
(0,y,t)= 0 and u
x
(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial condition:
f(x, y) = x
2
1 −
2x
3
y
1 −
y
2
6.21. Boundaries x = 0, x = 1, y = 0 are held at fixed temperature 0, boundary y = 1 is losing
heat by convection into a surrounding medium at 0 temperature, k = 1/10, β = 1/5:
u(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)+u(x, 1,t)= 0
Initial condition:
f(x, y) = x(1 −x)y
1 −
2y
3
6.22. Boundaries x = 1, y = 0 are held at fixed temperature 0, boundary x = 0 is insulated,
and boundary y = 1 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10, β = 1/5:
u
x
(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)+u(x, 1,t)= 0
Initial condition:
f(x, y) = (1 −x
2
)y
1 −
2y
3

402 Chapter 6
6.23. Boundaries x = 1, y = 0 are held at fixed temperature 0, boundary y = 1 is insulated,
and boundary x = 0 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10, β = 1/5:
u
x
(0,y,t)−u(0,y,t)= 0 and u(1,y,t)= 0
u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial condition:
f(x, y) =
−
2x
2
3
+
x
3
+
1
3
y
1 −
y
2
6.24. Boundaries y = 0, y = 1 are held at fixed temperature 0, boundary x = 0 is insulated,
and boundary x = 1 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10, β = 1/5:
u
x
(0,y,t)= 0 and u
x
(1,y,t)+u(1,y,t)= 0
u(x, 0,t)= 0 and u(x, 1,t)= 0
Initial condition:
f(x, y) =
1 −
x
2
3
y(1 −y)
6.25. Boundaries x = 0, x = 1 are held at fixed temperature 0, boundary y = 1 is insulated,
and boundary y = 0 is losing heat by convection into a surrounding medium at 0
temperature, k = 1/10, β = 1/5:
u(0,y,t)= 0 and u(1,y,t)= 0
u
y
(x, 0,t)−u(x, 0,t)= 0 and u
y
(x, 1,t)= 0
Initial condition:
f(x, y) = x(1 −x)
1 −
(y −1)
2
3
Two Dimensions, Cylindrical Coordinates
We now seek the temperature distribution in a thin, circular plate over the two-dimensional
domain D ={(r, θ) |0 <r<1, 0 <θ<b} whose lateral surfaces are insulated. The
The Diffusion Equation in Two Spatial Dimensions 403
homogeneous partial differential equation is
∂
∂t
u(r,θ,t)= k
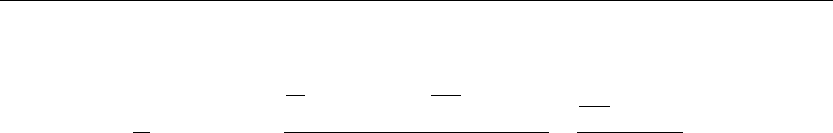
⎛
⎜
⎜
⎜
⎜
⎝
∂
∂r
u(r,θ,t)+r
∂
2
∂r
2
u(r,θ,t)
r
+
∂
2
∂θ
2
u(r,θ,t)
r
2
⎞
⎟
⎟
⎟
⎟
⎠
with initial conditions u(r, θ, 0) = f(r, θ). In Exercises 6.26 through 6.37, we seek the
temperature solution for various boundary and initial conditions. Evaluate the eigenvalues and
corresponding two-dimensional orthonormalized eigenfunctions, and write the general
solution. Generate the animated three-dimensional surface solution u(r,θ,t), and plot the
animated sequence for 0 <t<5.
6.26. The center of the plate has a finite temperature, and the periphery at r = 1 is held at a
fixed temperature of 0. The boundaries θ = 0 and θ = π are held at fixed temperature 0,
k = 1/10:
|
u(0,θ,t)
|
< ∞ and u(1,θ,t)= 0
u(r, 0,t)= 0 and u(r,π,t)= 0
Initial condition:
f(r, θ) = (r −r
3
) sin(θ)
6.27. The center of the plate has a finite temperature, and the periphery at r = 1 is held at a
fixed temperature of 0. The boundaries θ = 0 and θ = π are insulated, k = 1/10:
|
u(0,θ,t)
|
< ∞ and u(1,θ,t)= 0
u
θ
(r, 0,t)= 0 and u
θ
(r,π,t)= 0
Initial condition:
f(r, θ) = (r
2
−r
4
) cos(2θ)
6.28. The center of the plate has a finite temperature, and the periphery at r = 1 is held at a
fixed temperature of 0. The boundaries θ = 0 and θ = π are held at fixed temperature
0,k = 1/10:
|
u(0,θ,t)
|
< ∞ and u(1,θ,t)= 0
u(r, 0,t)= 0 and u(r,π,t)= 0
Initial condition:
f(r, θ) = (r
3
−r
5
) sin(3θ)

404 Chapter 6
6.29. The center of the plate has a finite temperature, and the periphery at r = 1 is held at a
fixed temperature of 0. The boundaries θ = 0 and θ = π/2 are held at fixed temperature
0,k = 1/10:
|
u(0,θ,t)
|
< ∞ and u(1,θ,t)= 0
u(r, 0,t)= 0 and u
r,
π
2
,t
= 0
Initial condition:
f(r, θ) = (r
2
−r
4
) sin(2θ)
6.30. The center of the plate has a finite temperature, and the periphery at r = 1 is held at a
fixed temperature of 0. The boundaries θ = 0 and θ = π/2 are insulated, k = 1/10:
|
u(0,θ,t)
|
< ∞ and u(1,θ,t)= 0
u
θ
(r, 0,t)= 0 and u
θ
r,
π
2
,t
= 0
Initial condition:
f(r, θ) = (r
4
−r
6
) cos(4θ)
6.31. The center of the plate has a finite temperature, and the periphery at r = 1 is held at a
fixed temperature of 0. The boundary conditions are such that we have periodic
boundary conditions on θ, k = 1/10:
|
u(0,θ,t)
|
< ∞ and u(1,θ,t)= 0
u(r, −π, t) = u(r,π,t) and u
θ
(r, −π, t) = u
θ
(r,π,t)
Initial condition:
f(r, θ) = (r −r
3
) cos(θ)
6.32. The center of the plate has a finite temperature, and the periphery at r = 1 is insulated.
The boundaries θ = 0 and θ = π are held at fixed temperature 0, k = 1/10:
|
u(0,θ,t)
|
< ∞ and u
r
(1,θ,t)= 0
u(r, 0,t)= 0 and u(r,π,t)= 0
Initial condition:
f(r, θ) =
3r −r
3
sin(θ)

The Diffusion Equation in Two Spatial Dimensions 405
6.33. The center of the plate has a finite temperature, and the periphery at r = 1 is insulated.
The boundaries θ = 0 and θ = π are insulated, k = 1/10:
|
u(0,θ,t)
|
< ∞ and u
r
(1,θ,t)= 0
u
θ
(r, 0,t)= 0 and u
θ
(r,π,t)= 0
Initial condition:
f(r, θ) = (2r
2
−r
4
) cos(2θ)
6.34. The center of the plate has a finite temperature, and the periphery at r = 1 is insulated.
The boundaries θ = 0 and θ = π are held at fixed temperature 0, k = 1/10:
|
u(0,θ,t)
|
< ∞ and u
r
(1,θ,t)= 0
u(r, 0,t)= 0 and u(r,π,t)= 0
Initial condition:
f(r, θ) =
5r
3
3
−r
5
sin(3θ)
6.35. The center of the plate has a finite temperature, and the periphery at r = 1 is insulated.
The boundaries θ = 0 and θ = π/2 are held at fixed temperature 0, k = 1/10:
|
u(0,θ,t)
|
< ∞ and u
r
(1,θ,t)= 0
u(r, 0,t)= 0 and u
r,
π
2
,t
= 0
Initial condition:
f(r, θ) =
4r
6
3
−r
8
sin(6θ)
6.36. The center of the plate has a finite temperature, and the periphery at r = 1 is insulated.
The boundaries at θ = 0 and θ = π/2 are insulated, k = 1/10:
|
u(0,θ,t)
|
< ∞ and u
r
(1,θ,t)= 0
u
θ
(r, 0,t)= 0 and u
θ
r,
π
2
,t
= 0
Initial condition:
f(r, θ) =
3r
4
2
−r
6
cos(4θ)

406 Chapter 6
6.37. The center of the plate has a finite temperature, and the periphery at r = 1 is insulated.
The boundary conditions are such that we have periodic boundary conditions on θ,
k = 1/10:
|
u(0,θ,t)
|
< ∞ and u
r
(1,θ,t)= 0
u(r, −π, t) = u(r,π,t) and u
θ
(r, −π, t) = u
θ
(r,π,t)
Initial condition:
f(r, θ) = (3r −r
3
) sin(θ)
Decay Time for Diffusion Phenomena
The time-dependent portion of the solution to the diffusion equation in two dimensions in the
rectangular coordinate system reads
T
m,n
(t) = C(m, n)e
−kλ
m,n
t
The “decay time” τ at which the (m, n)th mode decays to (1/e) of its initial value is given as
τ
m,n
=
1
kλ
m,n
where
λ
m,n
= α
m
+β
n
Here α
m
is the eigenvalue corresponding to the x-dependent solution, β
n
is the eigenvalue
corresponding to the y-dependent solution, and k is the diffusivity of the medium.
For the case of Exercise 6.1, we evaluate λ
m,n
to be
λ
m,n
=
m
2
π
2
a
2
+
n
2
π
2
b
2
for m = 1, 2, 3,...,n= 1, 2, 3,....
From the preceding expressions, we make the following observations: (1) the larger the value
of k, the more rapid the decay; (2) the higher-order modes decay most rapidly; (3) the decay
slows down as the dimensions of the domain are increased; and (4) as t goes to infinity, all
modes decay exponentially to zero. Exercises 6.38 through 6.43 deal with the “decay times” of
some of the earlier exercises.
6.38. For Exercise 6.1, evaluate the decay time τ(1, 1) of the (1, 1) mode. By what factor is
τ(1, 1) larger than τ(2, 2)?Ifk is doubled, by what factor should the dimensions of the
rectangular region be increased to give the same decay time?

The Diffusion Equation in Two Spatial Dimensions 407
6.39. For Exercise 6.3, evaluate the decay time τ(1, 1) of the (1, 1) mode. By what factor is
τ(1, 1) larger than τ(1, 2)?Ifk is tripled, by what factor should the dimensions of the
rectangular region be increased to give the same decay time?
6.40. For Exercise 6.5, evaluate the decay time τ(1, 1) of the (1, 1) mode. By what factor is
τ(1, 1) larger than τ(3, 2)?Ifk is quadrupled, by what factor should the dimensions of
the rectangular region be increased to give the same decay time?
6.41. For Exercise 6.7, evaluate the decay time τ(1, 1) of the (1, 1) mode. By what factor is
τ(1, 1) larger than τ(3, 3)?Ifk is quadrupled, by what factor should the dimensions of
the rectangular region be increased to give the same decay time?
6.42. For Exercise 6.8, evaluate the decay time τ(1, 1) of the (1, 1) mode. By what factor is
τ(1, 1
) larger than τ(3, 1)?Ifk is tripled, by what factor should the dimensions of the
rectangular region be increased to give the same decay time?
6.43. For Exercise 6.9, evaluate the decay time τ(1, 1) of the (1, 1) mode. By what factor is
τ(1, 1) larger than τ(1, 3)?Ifk is doubled, by what factor should the dimensions of the
rectangular region be increased to give the same decay time?
