Alexandrov A.S.,Theory of Superconductivity - From Weak to Strong Coupling
Подождите немного. Документ загружается.


76
Intermediate-coupling theory
is the Fourier transform of the second derivative of the ion potential energy. The
first derivative in equation (3.6) is zero in crystals with a centre of symmetry.
Different solutions of equation (3.12) are classified with the phonon branch
(mode) quantum number ν, which is 1, 2, 3 for a simple lattice and 1,...,3k
for a lattice with k ions per unit cell.
The periodic part of the Hamiltonian H
e
is diagonal in the Bloch
representation (appendix A):
s
(r) =
k,n,s
ψ
nks
(r)c
nks
(3.15)
where c
nks
are the fermion annihilation operators. The Bloch function obeys the
Schr¨odinger equation
−
∇
2
2m
e
+ V (r)
ψ
nks
(r) = E
nks
ψ
nks
(r). (3.16)
One-particle states are sorted with the momentum k in the Brillouin zone, band
index n and spin s. The solutions of this equation allow us to calculate the periodic
electron density n
(0)
(r), which determines the crystal field potential V (r).The
LDA can explain the shape of the Fermi surface of wide-band metals and gaps
in narrow-gap semiconductors. The spin-polarized version of LDA can explain a
variety of properties of many magnetic materials. This is not the case in narrow
d- and f-band metals and oxides (and other ionic lattices), where the electron–
phonon interaction and Coulomb correlations are strong. These materials display
much less band dispersion and wider gaps compared with the first-principle band
structure calculations. Using the phonon and electron annihilation and creation
operators, the Hamiltonian is written as
H = H
0
+ H
e−ph
+ H
e−e
(3.17)
where
H
0
=
k,n,s
ξ
nks
c
†
nks
c
nks
+
q,ν
ω
qν
(d
†
qν
d
qν
+ 1/2) (3.18)
describes independent Bloch electrons and phonons, ξ
nks
= E
nks
−µ is the band
energy spectrum with respect to the chemical potential. The part of the electron–
phonon interaction, which is linear in phonon operators, can be written as
H
e−ph
=
1
√
2N
k,q,n,n
,ν,s
γ
nn
(q, k,ν)ω
qν
c
†
nks
c
nk−qs
d
qν
+ H.c. (3.19)
where
γ
nn
(q, k,ν) =−
N
M
1/2
ω
3/2
qν
dr (e
qν
· ∇v(r))ψ
∗
nks
(r)ψ
n
k−qs
(r) (3.20)

Phonons in metal
77
is the dimensionless matrix element. Low-energy physics is often described by a
single-band approximation with the matrix element γ
nn
(q, k,ν) depending only
on the momentum transfer q (the Fr¨ohlich interaction):
γ
nn
(q, k,ν) = γ(q,ν). (3.21)
The terms of H
e−ph
which are quadratic and higher orders in the phonon operators
are small. They have a role to play only for those phonons which are not coupled
with electrons by the linear interaction (3.20). The electron–electron correlation
energy of a homogeneous electron system is often written as
H
e−e
=
1
2
q
V
c
(q)ρ
†
q
ρ
q
(3.22)
where V
c
(q) is a matrix element, which is zero for q = 0 because of
electroneutrality and
ρ
†
q
=
k,s
c
†
ks
c
k+qs
(3.23)
is the density fluctuation operator. H
0
should also include a random potential in
doped semiconductors and amorphous metals.
3.2 Phonons in metal
In wide-band metals such as Na or K, the correlation energy is relatively small
(r
s
≤ 1) and carriers are almost free. Core electrons together with nuclei form
compact ions with an effective Z . The carrier wavefunction outside the core can
be approximated by a plane wave
ψ
nks
(r) e
ik·r
(3.24)
and the carrier density n
(0)
(r) is a constant. Therefore, the only relevant
interaction in the dynamic matrix (equation (3.7)) is the Coulomb repulsion
between ions, which yields
D
αβ
(l − m) =
Z
2
e
2
2
∂
2
∂l
α
∂m
β
l
=m
1
|l
− m
|
. (3.25)
The electron–ion interaction is a pure Coulomb attraction v(r) =−Ze
2
/r, which
is expanded in the Fourier series as
1
r
= 4π lim
κ→0
q
1
q
2
+ κ
2
e
iq·r
. (3.26)
Substituting this expansion into equations (3.25) and (3.20), we obtain
D
αβ
(m) = 4π lim
κ→0
q
q
α
q
β
q
2
+ κ
2
cos(q · m) (3.27)
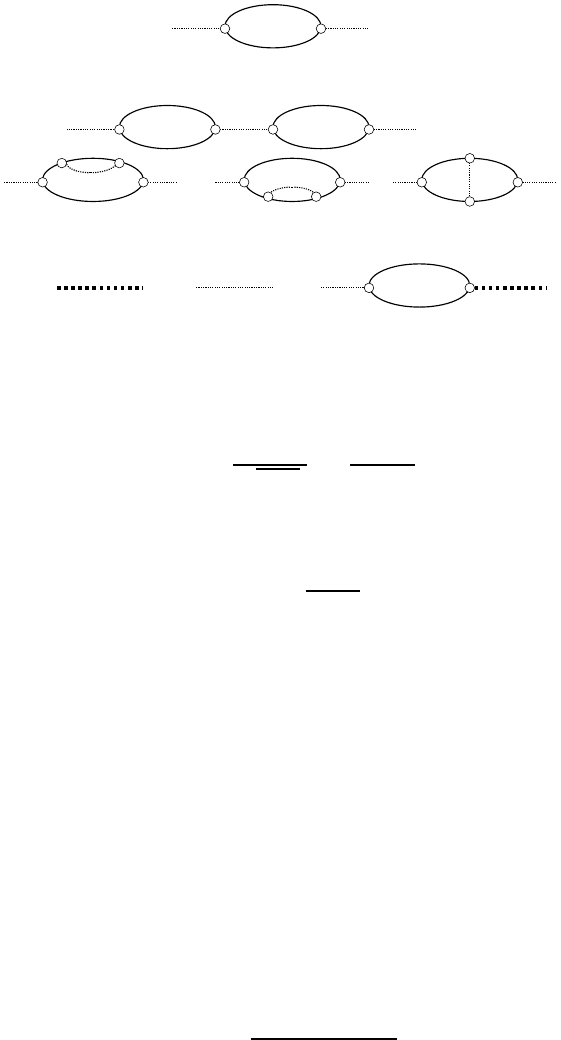
78
Intermediate-coupling theory
a
+
+
+ +
b
cd
e
= +
f
D(q,ω)
Figure 3.1. Second (a) and fourth order (b), (c), (d), (e) corrections to the phonon GF. The
phonon GF in the Migdal approximation (f ).
and
γ(q,ν) = i
4π NZe
2
Mω
3
q
lim
κ→0
e
qν
· q
q
2
+ κ
2
. (3.28)
Calculating the Fourier transform of equation (3.27), one obtains the following
equation for phonon frequencies and polarization vectors:
ω
2
q
e
qν
= ω
2
i
q
e
qν
· q
q
2
. (3.29)
A longitudinal mode with e q is the ion plasmon
ω
q
= ω
i
(3.30)
and two shear (transverse) modes with e ⊥ q have zero frequencies, which is
the result of our approximation considering ions as rigid charges. In fact, core
electrons undergo a polarization, when ions are displaced from their equilibrium
positions, which yields a finite shear mode frequency.
According to equation (3.28), the carriers interact only with longitudinal
phonons. The interaction gives rise to a significant renormalization of the
bare phonon frequency (equation (3.30)). We apply the Green’s function (GF)
formalism (appendix D) to calculate the renormalized phonon frequency. The
Fourier component of the free-electron GF is given by equation (2.163) with
ξ
k
= k
2
/2m
e
−µ. For interacting electrons, the electron self-energy is introduced
as
(k,ω) =[G
(0)
(k,ω)]
−1
−[G(k,ω)]
−1
(3.31)
and
G(k,ω) =
1
ω − ξ
k
− (k,ω)
. (3.32)
Phonons in metal
79
The phonon GF is defined as
D(q, t) =−i
ω
q
2
T
t
(d
q
(t)d
†
q
+ d
†
q
(t)d
q
), (3.33)
and its Fourier transform for free phonons is a dimensionless even function of
frequency,
D
(0)
(q,ω) =
ω
2
q
ω
2
− ω
2
q
+ iδ
. (3.34)
The phonon self-energy is
(q,ω) =[D
(0)
(q,ω)]
−1
−[D(q,ω)]
−1
. (3.35)
The Feynman diagram technique is convenient, see figure 3.1. Thin straight
and dotted lines correspond to G
(0)
and D
(0)
, respectively, a vertex (circle)
corresponds to the interaction matrix element γ(q)
ω
q
/N and bold lines
represent G and D.TheFr¨ohlich interaction is the sum of two operators
describing the emission and absorption of a phonon. Both events are taken into
account in the definition of D. Therefore wavy lines have no direction. There are
no first- or higher-odd orders corrections to D because the Fr¨ohlich interaction is
off-diagonal with respect to phonon occupation numbers. The second-order term
in D (figure 3.1(a)) includes the so-called polarization bubble,
(0)
e
,whichisa
convolution of two G
(0)
. Among different fourth-order diagrams the diagram in
figure 3.1(b) with two polarization loops is the most ‘dangerous’ one. Differing
from others, it is proportional to 1/q
2
, which is large for small q.However,the
singularity of internal vertices is ‘integrated out’ in the diagrams in figures 3.1(c)–
(d). The sum of all dangerous diagrams is given in figure 3.1(f )whichis
(q,ω) =
|γ(q)|
2
ω
q
N
e
(q,ω) (3.36)
where
e
(q,ω) =
(0)
e
(q,ω) (3.37)
and
(0)
e
(q,ω) =−
2i
(2π)
4
dk d G
(0)
(k + q, + ω)G
(0)
(k,). (3.38)
The additional factor 2 in the phonon self-energy is due to a contribution of two
electron spin states. It is convenient to integrate over frequency in equation (3.38)
first with the following result:
(0)
e
(q,ω) =
1
4π
3
dk
(ξ
k
) − (ξ
k+q
)
ω + ξ
k
− ξ
k+q
+ iδ sign(ξ
k+q
)
. (3.39)
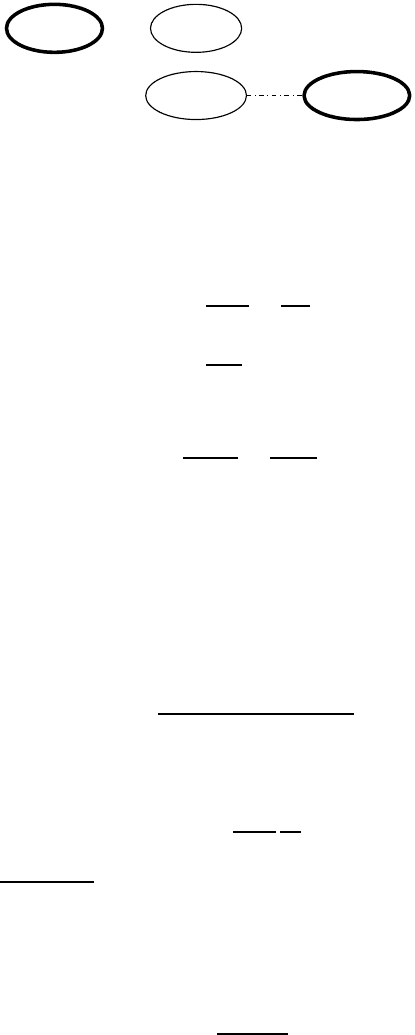
80
Intermediate-coupling theory
πH
T
Figure 3.2. Screened polarization bubble
e
(q,ω).
The phonon frequency ω is small, ω µ. Thus, we can take the limit ω → 0in
(0)
e
and obtain
Re
0
e
(q,ω) =−
m
e
k
F
2π
2
h
q
2k
F
(3.40)
Im
0
e
(q,ω) =−
m
2
e
2πq
|ω|(2k
F
− q) (3.41)
where
h(x ) = 1 +
1 − x
2
2x
ln
1 + x
1 − x
.
We should also take the Coulomb electron–electron interaction into account
because the corresponding vertex is singular in the long-wavelength limit,
V
c
(q) = 4πe
2
/q
2
. This leads to a drastic renormalization of the long wave-
length behaviour of
e
. In the ‘bubble’ or random phase approximation (RPA),
we obtain figure 3.2 as in the case of the electron–ion plasmon interaction,
figure 3.1(f ), but with the Coulomb (dashed-dotted) line instead of the dotted
phonon line. In the analytical form, we have
e
(q,ω) =
(0)
e
(q,ω)
1 − (4πe
2
/q
2
)
0
e
(q,ω)
. (3.42)
As a result, in the long-wavelength limit q q
s
one obtains
e
(q,ω) =−
m
e
k
F
π
2
q
2
q
2
s
(3.43)
where q
s
=
4m
e
k
F
e
2
/π is the inverse (Debye) screening radius.
While
(0)
e
is finite at q → 0, the screened
e
(q,ω) iszerointhis
limit. Using the RPA expressions (equations (3.40)–(3.42)) and γ
q
determined
in equation (3.28) for ω
q
= ω
i
, we obtain the phonon GF as
D(q,ω) =
ω
2
i
ω
2
−˜ω
2
q
. (3.44)
Electrons in metal
81
The poles of D determine a new phonon dispersion and a damping due to
interaction with electrons:
˜ω
q
=
ω
i
(q, ˜ω
q
)
1/2
(3.45)
where
(q,ω) = 1 −
4πe
2
q
2
(0)
e
(q,ω) (3.46)
is the electron dielectric function. In the long-wavelength limit,
(q, 0) = 1 +
q
2
s
q
2
(3.47)
and we obtain the sound wave as the real part of ˜ω:
˜ω
q
= sq (3.48)
where s = Zk
F
/
√
3Mm
e
is the sound velocity. The imaginary part of ˜ω
determines the damping of the sound,
s
v
F
˜ω. (3.49)
Because the ratio of the sound velocity to the Fermi velocity (v
F
) is adiabatically
small (s/v
F
√
m
e
/M), the damping is small ( ˜ω). Electrons screen the
bare ion–ion Coulomb repulsion and the residual short-range dynamic matrix has
the sound-wave linear dispersion of the eigenfrequencies in the long-wavelength
limit.
3.3 Electrons in metal
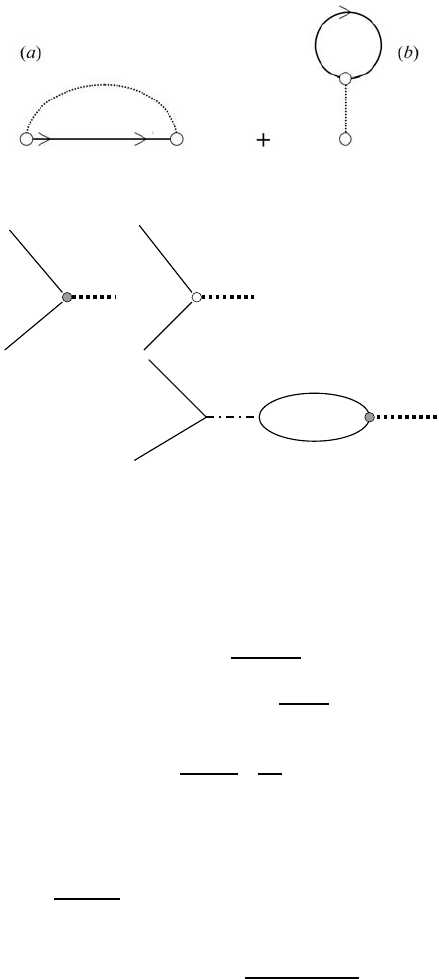
The lowest contribution to the electron self-energy is given by two second-order
diagrams, see figures 3.3(a)and(b). The diagram in figure 3.3(b) is proportional
to |γ(q)|
2
with q ≡ 0, which is zero according to equation (3.28).
Higher-order diagrams are taken into account by replacing the bare ionic
plasmon GF by a renormalized one, equation (3.44), and the bare electron–
phonon interaction γ(q) by a screened one, γ
sc
(q,ω), as shown in figure 3.4.
Presented analytically, the diagram in figure 3.4 corresponds to
γ
sc
(q,ω) = γ(q) +
4πe
2
q
2
(0)
e
(q,ω)γ
sc
(q,ω) (3.50)
so that
γ
sc
(q,ω) =
γ(q)
(q,ω)
. (3.51)
82
Intermediate-coupling theory
Figure 3.3. Second-order electron self-energy.
Figure 3.4. Screened electron–phonon interaction (dark circle).
For low-energy excitations (ω µ) a static approximation of the dielectric
function (3.47) is appropriate. Instead of the D and γ
sc
given by equations (3.44)
and (3.51), respectively, we introduce the acoustic phonon GF
˜
D(q,ω) =
˜ω
2
q
ω
2
−˜ω
2
q
(3.52)
and the electron–acoustic phonon vertex ˜γ(q)
˜ω
q
/N ,where
˜γ(q) =
γ(q)
(q, 0)
ω
i
˜ω
q
3/2
. (3.53)
Finally we obtain the diagram in figure 3.5 for the electron self-energy as a
result of the summation of the most divergent diagrams, which is
(k,) =
2i
(2π)
4
N
dq dω E
p
G(k − q, −ω)
˜
D(q,ω) (3.54)
where
2E
p
=|˜γ(q)|
2
˜ω
q
=
2µ
Z(1 +q
2
/q
2
s
)
. (3.55)
Because Z is of the order of one, the electron–acoustic phonon interaction
E
p
is generally of the order of the Fermi energy. Therefore, one has to consider

Electrons in metal
83
Figure 3.5. Electron self-energy in the Migdal approximation.
Figure 3.6. Adiabatically small corrections to the electron self-energy.
fourth- and higher-order diagrams with the crossing phonon lines as in figure 3.6,
which are absent in figure 3.5. These diagrams are known as vertex corrections.
Fortunately, as shown by Migdal [48], their contribution is adiabatically small
(∼s/v
F
) compared with equation (3.54). This result is known as Migdal’s
‘theorem’.
The electron energy spectrum, renormalized by the electron–phonon
interaction, is determined as the pole of the electron GF,
˜
ξ
k
≡
˜
E
k
−˜µ = v
F
(k − k
F
) + δE
k
(3.56)
where
δ E
k
= (k,
˜
ξ
k
) − (k
F
, 0) (3.57)
and ˜µ = µ + (k
F
, 0) is the renormalized Fermi energy. The region of large
q k
F
q
s
contributes mostly to the integral in equation (3.54). The
dimensionless coupling constant λ = 2E
p
N(E
F
) is small in this region,
λ ≈ r
s
1 (3.58)
and the second order is sufficient in our calculations of . Hence, we can
replace the exact GF in the integral equation (3.54) by the free GF. This is
appropriate for almost all quasi-particle energies. The difference between G and
G
(0)
appears to be important only for the damping calculations in a very narrow
region |
˜
ξ
k
|ω
D
s/v
F
near the Fermi surface. As a result, we obtain
δ E
k
=
2iE
p
(2π)
4
N
dq dω
˜
D(q,ω)[G
(0)
(k − q,
˜
ξ
k
− ω) − G
(0)
(k
F
− q, −ω)].
(3.59)
To simplify the calculations, we take E
p
as a constant and apply the Debye
approximation ˜ω
q
= sq for q < q
D
where q
D
π/a is the Debye momentum.
We also consider a half-filled band, k
F
≈ π/2a with the energy-independent DOS
near the Fermi level N(E
F
) = m
e
a
2
/4π.
The main contribution to the integral in equation (3.59) comes from the
momentum region close to the Fermi surface,
|k − q|k
F
. (3.60)
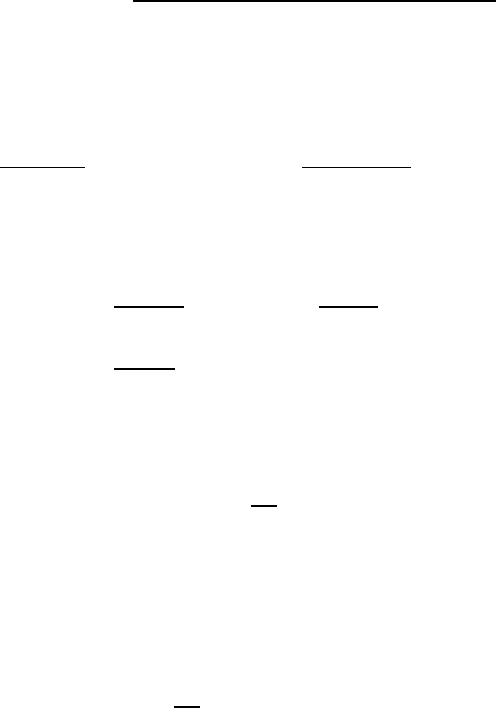
84
Intermediate-coupling theory
It is convenient to introduce a new variable k
=|k − q| instead of the angle
between k and q, and extend the integration to ±∞ for ξ = v
F
(k
− k
F
).Then
the angular integration in equation (3.59) yields
d sin (...)∼
∞
−∞
dξ
˜
ξ
[
˜
ξ − ω − ξ + iδ sign(ξ)][ω + ξ − iδ sign(ξ)]
.
(3.61)
This integral is non-zero only if
˜
ξ>ω>0or
˜
ξ<ω<0. It is −2πiinthe
first region and 2πi in the second region. Taking into account that
˜
D is an even
function of ω, we obtain
δ E
k
=
2E
p
(2π)
2
v
F
N
q
D
0
dqq
|
˜
ξ|
0
dω sign(
˜
ξ)
˜ω
2
q
ω
2
−˜ω
2
q
+ iδ
. (3.62)
The real and imaginary parts of equation (3.62) determine the renormalized
spectrum and the lifetime of quasi-particles, respectively:
Re(δ E
k
) =
E
p
4π
2
v
F
N
q
D
0
dqq˜ω
q
ln
˜ω
q
−
˜
ξ
˜ω
q
+
˜
ξ
(3.63)
Im(δE
k
) =
E
p
4πv
F
N
q
m
0
dqq˜ω
q
sign(
˜
ξ) (3.64)
with q
m
=|
˜
ξ|/s if |
˜
ξ| <ω
D
and q
m
= q
D
if |
˜
ξ| >ω
D
. For excitations far away
from the Fermi surface (|
˜
ξ|ω
D
),wefind
Re(δ E
k
) =−λ
ω
2
D
2
˜
ξ
(3.65)
and for low-energy excitations with |
˜
ξ|ω
D
,
Re(δ E
k
) =−λ
˜
ξ. (3.66)
This means an increase in the effective mass of the electron due to the electron–
phonon interaction,
˜
ξ =
k
F
m
∗
(k − k
F
) (3.67)
where the renormalized mass is
m
∗
= (1 + λ)m
e
. (3.68)
Hence, the excitation spectrum of metals has two different regions with two
different values of the effective mass. The thermodynamic properties of a metal at
low temperatures T ω
D
involve m
∗
but the optical properties in the frequency
range ν ω
D
are determined by high-energy excitations, where, according to
equation (3.65), corrections are small and the mass is equal to the band mass m
e
.
Eliashberg equations
85
Damping shows just the opposite behaviour. The integral in equation (3.64),
yields
Im(δE
k
) = sign(
˜
ξ)
πλω
D
3
(3.69)
if |
˜
ξ| >ω
D
and
Im(δ E
k
) = sign(
˜
ξ)
πλ|
˜
ξ|
3
3ω
2
D
(3.70)
if |
˜
ξ|ω
D
.
These expressions describe the rate of decay of quasi-particles due to the
emission of phonons. In the immediate neighbourhood of the Fermi surface,
|
˜
ξ|ω
D
, the decay is small compared with the quasi-particle energy |
˜
ξ| even for
a relatively strong coupling λ ∼ 1 and the concept of well-defined quasi-particles
has a definite meaning. Within the Migdal approximation the electron–phonon
interaction does not destroy the Fermi-liquid behaviour of electrons. The Pauli
exclusion principle is responsible for the stability of the Fermi liquid. In the
intermediate-energy region |
˜
ξ|∼ω
D
, the decay is comparable with the energy
and the quasi-particle spectrum loses its meaning. In the high-energy region
|
˜
ξ|ω
D
, the decay becomes small again in comparison with |
˜
ξ| and the quasi-
particle concept recovers its meaning.
Going beyond the Migdal approximation, we have to consider adiabatically
small higher-order diagrams, that is to solve the Hamiltonian of free electrons and
acoustic phonons coupled by an interaction:
H
e−ph
=
1
√
2N
k,q,s
˜γ(q) ˜ω
q
c
†
ks
c
k−qs
˜
d
q
+ H.c. (3.71)
where
˜
d
q
is the acoustic-phonon annihilation operator. From our consideration,
it follows that, in applying this Hamiltonian to electrons, one should not consider
the acoustic phonon self-energy. Acoustic phonons in a metal appear as a result
of the electron-plasmon coupling and the Coulomb screening, so their frequency
already includes the self-energy effect (section 3.2).
3.4 Eliashberg equations
Based on Migdal’s theorem, Eliashberg [36] extended BCS theory towards the
intermediate-coupling regime (λ
1), applying the Gor’kov formalism. The
condensed state is described by a classical field, which is the average of the
product of two annihilation field operators
ψψ or two creation operators
+
ψ
†
ψ
†
. These averages are macroscopically large below T
c
.The
appearance of anomalous averages cannot be seen perturbatively but they should
be included in the self-energy diagram, figure 3.5, from the very beginning. This
