Alexandrov A.S.,Theory of Superconductivity - From Weak to Strong Coupling

Подождите немного. Документ загружается.

Intermediate-coupling theory

can be done in a compact form by introducing the matrix GF [49]:

(k,τ) =−



T

k↑

(τ )c

†

k↑

 T

k↑

(τ )c

−k↓



T

†

−k↓

(τ )c

†

k↑

 T

†

−k↓

(τ )c

−k↓





(3.72)

and the matrix self-energy

(k,ω

) = (

(0)

(k,ω

))

−1

−

−1

(k,ω

) (3.73)

where

(0)

(k,ω

) = (iω

−ξ

)

−1

is the normal-state matrix GF. Here τ

0,1,2,3

are the Pauli matrices,











0 −i

i 0





0 −1



The generalized equation for the matrix

 is given by the same diagram as in the

normal state (ﬁgure 3.5) but replacing ˜γ(q) by ˜γ(q)τ

and the integral over ω by

the sum over the Matsubara frequencies:

(k,ω

) =−

(2π)







dq ˜γ

(q) ˜ω

3 s

(k − q,ω



)τ

(q,ω

− ω



(3.74)

Here the temperature GF of phonons is given by

(q,

) =−

˜ω



+˜ω

(3.75)

where 

= 2π Tn are the Matsubara frequencies for bosons. The most

important difference between equation (3.74), ﬁgure 3.7, and the normal state

equation (3.54), ﬁgure 3.5, is a ﬁnite value of the anomalous GF in the

self-consistent solution. If we replace

(0)

on the right-hand side of

equation (3.74), we do not ﬁnd any anomalous

. Hence, there are no anomalous

averages and no phase transition in the second or in any ﬁnite order of the

perturbation theory. However, if we solve equation (3.74) self-consistently, we

ﬁnd the ﬁnite anomalous averages.

The diagrams in ﬁgure 3.7 are expressed in analytical form as a set

of Eliashberg equations, similar to Gor’kov’s equations (2.186) but with a

microscopically determined pairing potential. They include all non-crossing

(‘ladder’) diagrams in every order of the perturbation theory. For simplicity, let

us consider a momentum-independent ˜γ

(q) ˜ω

as in the previous section and

approximate the phonon GF in equation (3.74) as

(q,

) ≈−1 (3.76)

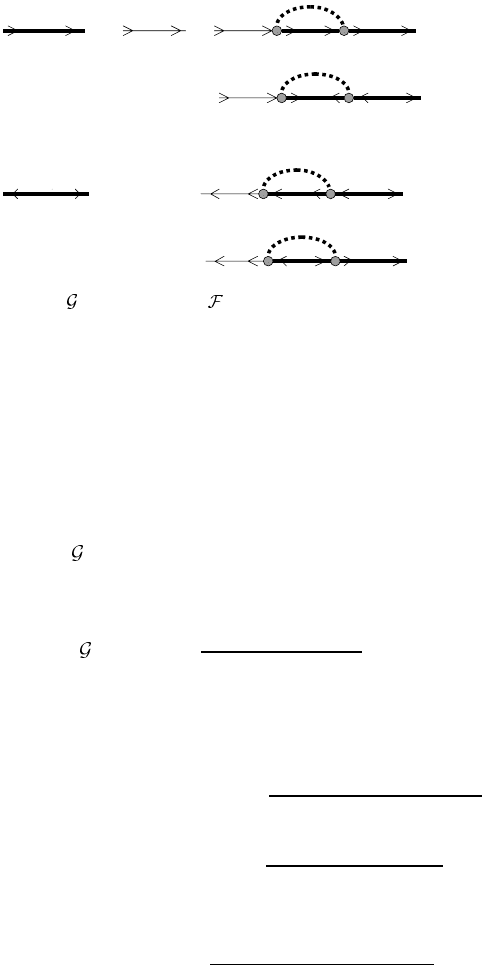
Eliashberg equations

 



)



Figure 3.7. Normal and anomalous

GFs of the BCS superconductor in the

Eliashberg theory.

if |

| <ω

, and zero otherwise.

 is the sum of three Pauli matrices τ

0,1,3

with the coefﬁcients (1 − Z )iω

,  and χ, respectively, which in this case are

functions of frequency alone,

(k,ω

) = i(1 − Z )ω

+ τ

+ χτ

(3.77)

and

−1

(k,ω

) = iZ ω

− τ

−

. (3.78)

Transforming the inverse matrix (equation (3.78)) back into the original one yields

(k,ω

) =−

iZω

+ τ

+||

(3.79)

where

= ξ

+ χ. Substituting equations (3.76) and (3.79) into the master

equation (3.74) leads to the following simpliﬁed Eliashberg equations:

[1 − Z(ω

)]iω

=−λT



∞

−∞





(ω

−|ω

− ω



|)iω



+||

(3.80)

χ(ω

) =−λT







∞

−∞

(ω

−|ω

− ω



+||

(3.81)

and

(ω

) = λT







∞

−∞

(ω

−|ω

− ω



|)(ω



)



+||

. (3.82)

We can satisfy equations (3.80) and (3.81) with Z = 1andχ = 0. In the last

equation, we extend the summation over frequencies to inﬁnity but cut the integral

Intermediate-coupling theory

over |

ξ| at ω

. Then applying equation (2.54) for tanh yields the familiar BCS

equation for the order parameter,

1 = λ





+||

tanh



+||

. (3.83)

Hence the Migdal–Eliashberg theory reproduces the BCS results, if a similar

approximation for the attraction between electrons is adopted. The critical

temperature and the BCS gap are adiabatically small (

) compared with

the Fermi energy and we could worry about the adiabatically small crossing

diagrams in ﬁgure 3.6, which are neglected in the master equation, (3.74).

However, the BCS state is essentially the same as the normal state outside the

narrow momentum region around the Fermi surface. The outside regions mainly

contribute to the integrals of the crossing diagrams, which makes them small as

in the normal state. As a result, the vertex corrections are small and Migdal’s

theorem holds in the BCS state as well.

Within a more general consideration the master equation (3.74) takes

properly into account the phonon spectrum, retardation and realistic matrix

elements of the electron–phonon interaction in metals [50–52]. The important

feature of the intermediate-coupling theory is the explicit frequency dependence

of the order parameter (ω

), which is transformed into the energy-dependent

gap in the quasi-particle DOS as

ρ() =







−|()|

. (3.84)

The DOS (equation (3.84)) can be measured in the tunnelling experiments

(section 2.4). As the gap depends on the phonon spectrum, phonons affect the

tunnelling I –V characteristics. Let us deﬁne the phonon density of states

F(ω) =



qν

δ(ω −˜ω

qν

) (3.85)

and the so-called Eliashberg function α

(ω)F(ω) =

N(E



k,k



˜γ

(k − k



,ν)˜ω

k−k



,ν

δ(ω −˜ω

k−k



,ν

)δ(ξ



)

(3.86)

which is an average over the Fermi surface of the interaction matrix element

squared multiplied by the phonon spectral density. The quasi-particle DOS

(equation (3.84)) can be obtained in a direct fashion using the tunnelling

conductivity of the superconducting tunnel junctions. Then the function α

can be calculated to ﬁt the experimental energy dependence of the gap ()

[54] and compared with the phonon density of states measured independently,

Coulomb pseudopotential

for example, in neutron scattering experiments. The comparison shows that

frequency dependence of the Eliashberg function and the phonon density of

states are similar in many low-temperature superconductors with a weak or

intermediate electron–phonon coupling, for example in lead. The observation

of the characteristic phonon frequencies in tunnelling (usually in the second

derivative of the current versus voltage) and the isotope effect are used to verify

the phonon-mediated pairing mechanism.

3.5 Coulomb pseudopotential

The theory of superconductivity has to take account of the Coulomb repulsive

correlations between electrons, which might be much stronger than the attraction

induced by phonons. There is no adiabatic parameter for this interaction because

the electron plasma frequency ω



4πn

has about the same order of

magnitude as the Fermi energy in metals. Nevertheless, we can account for the

Coulomb repulsion in the same fashion as for the electron–phonon interaction but

replacing ˜γ

(q) ˜ω

D(q,ω

− ω



) in equation (3.74) by the Fourier component

of the Coulomb potential V

. The Coulomb interaction is non-retarded for

frequencies less than ω

. Then the kernel K (ω

− ω



) in the BCS equation,

(ω

) = T



dξ





K (ω

− ω



)

(ω



)



+ ξ

+|(ω



(3.87)

can be parametrized as

K (ω

− ω



) = λ(ω

−|ω

− ω



|) − µ

(ω

−|ω

− ω



|) (3.88)

where µ

= V

N(E

).AtT = T

, we neglect second and higher powers of the

order parameter and integrating over ξ obtain

(ω

) = π T





K (ω

− ω



)

(ω



)

|ω



. (3.89)

Let us adopt the BCS-like approximation of the kernel:

K (ω

− ω



)  λ(2ω

−|ω

|)(2ω

−|ω



− µ

(2ω

−|ω

|)(2ω

−|ω



and replace the summation in equation (3.89) by the integral:

π T



≈



∞

π T

dω (3.90)

because T

 ω

. Then the solution is found in the form

(ω) = 

(2ω

−|ω|) + 

(2ω

−|ω|)(|ω|−2ω

) (3.91)

Intermediate-coupling theory

with constant but different values of the order parameter 

and 

below and

above the cut-off energy 2ω

, respectively. Substituting equation (3.91) into

equation (3.89) yields the following equations for 

1,2





1 − (λ −µ

) ln

2ω

π T



+ 

= 0 (3.92)



2ω

π T

+ 



1 + µ



= 0. (3.93)

A non-trivial solution of these coupled equations is found, if

2ω

exp



−

λ − µ

∗



(3.94)

where

∗

1 + µ

ln(ω

/ω

)

(3.95)

is the so-called Coulomb pseudopotential [53]. This is a remarkable result.

It shows that even a large Coulomb repulsion µ

>λdoes not destroy the

Cooper pairs because its contribution is suppressed down to the value about

−1

(ω

/ω

)  1. The retarded attraction mediated by phonons acts well after

two electrons meet each other. This time delay is sufﬁcient for two electrons to

be separated by a relative distance, at which the Coulomb repulsion is small.

The Coulomb correlations also lead to a damping of excitations of the order

of ξ

/µ, which is relevant only in a narrow region around the Fermi surface

|ξ|

√

/M. The damping due to the Fr¨ohlich interaction dominates outside

this region.

Computational analysis of the Eliashberg equations led McMillan [54] to

suggest an empirical formula for T

, which works well for simple metals and

their alloys,

1.45

exp



−

1.04(1 +λ)

λ −µ

∗

(1 + 0.62λ)



. (3.96)

However, in materials with a moderate T

20 K (like Nb

Sn, V

Si and in other

A-15 compounds), the discrepancy between the value of λ, estimated from this

equation and from the ﬁrst-principle band-structure calculations, exceeds the limit

allowed by the experimental and computation accuracy by several times [55].

In the original papers, Migdal [48] and Eliashberg [36] restricted the

applicability of their approach to the intermediate region of coupling λ<1. With

the typical values of λ = 0.5andµ

∗

= 0.14 and with the Debye temperature

as high as ω

= 400 K, McMillan’s formula predicts T

≈ 2 K, clearly too low

to explain high T

values in novel superconductors. One can formally compute

and the gap using the Eliashberg equations (3.74) also in the strong-coupling

regime λ>1. In particular, Allen and Dynes [56] found that in the extreme

strong-coupling limit (λ  1), the critical temperature may rise as

≈

1/2

2π

. (3.97)

Cooper pairing of repulsive fermions

However, the Migdal–Eliashberg theory is based on the assumption that the Fermi

liquid is stable and the adiabatic condition µ  ω

is satisﬁed. As we shall later

discuss in chapter 4, this assumption cannot be applied in the strong-coupling

regime and the proper extension of the BCS theory to λ>1 inevitably involves

small polarons and bipolarons.

3.6 Cooper pairing of repulsive fermions

The phenomenon of superconductivity is due to the interaction of electrons with

vibrating ions, which mediates an effective attractive potential V (k, k



)<0in

equation (2.98). In recent years, great attention has been paid to a possibility of

superconductivity mediated by strong electron–electron correlations without any

involvement of phonons. Some time ago, Kohn and Luttinger [57] pointed out

that such possibility exists at least theoretically. They found that a dilute Fermi

gas cannot remain normal down to absolute zero of temperature even in the case

of purely repulsive short-range interaction between the particles. A system of

fermions with purely repulsive short-range forces will inevitably be superﬂuid at

zero temperature.

To understand what is involved, one should consider the screening of a

charge placed in a metal. It has long been known [58] that if fermions are

degenerate (i.e. their Fermi surface is well deﬁned), the screening produces an

oscillatory potential of the form cos(2k

r + ϕ)/r

at the distance r from the

charge (here ϕ is a constant) which has attractive regions. Using these regions

of screened interaction, unconventional Cooper pairs can form with non-zero

orbital momentum (section 2.10). Following Kohn and Luttinger, let us consider a

simpliﬁed model of spin-

fermions with a weak short-range repulsive interaction

between them. The critical temperature of unconventional Cooper pairing with

the orbital momentum l is found as (see equation (2.104))

≈ µ exp



−



. (3.98)

Here we replaced the Debye temperature by the Fermi energy µ because

the fermion–fermion interaction is non-retarded. The coupling constant λ

expressed in terms of spherical harmonics V (l) of the Fourier transform of the

interaction potential,

≡−

V (l)mk

2π(2l + 1)

(3.99)

on condition that V (l) is negative. For simplicity, we choose the repulsive bare

potential as V (r −r



) = U with a positive U, if |r −r



| r

, and V (r −r



) = 0,

if |r − r



| > r

. We also assume that the gas is diluted, that is the radius of the

potential is small compared with the characteristic wave-length of fermions,

 1. (3.100)

Intermediate-coupling theory

It is easy to estimate V (l) for such a potential,

V (l) =

2l + 1



d sin P

(cos )V (q) (3.101)

where q

= 2k

(1 − cos ) is about 2k

or less and

V (q) = U



r a

dr exp(iq ·r) ≈

4π



1 −

(qr

)

(qr

)

[(k

)

]



(3.102)

Substituting the Fourier transform (3.102) into equation (3.101), we obtain a

positive V (l) of the order of

V (l)

)

. (3.103)

We conclude that the repulsive bare potential does not produce any pairing. Let us

now take screening into account. It is sufﬁcient to consider it perturbatively, if the

potential is weak, U  µ. The diagrams contributing to the effective interaction

up to the second order in U are shown in ﬁgure 3.8. In the analytical form, the

Fourier transform of the effective interaction of two electrons on the Fermi surface

is given by

V (k, k



) = V (q) −

(2π)





d p [2V

(q) − 2V (q)V (k



− p)]

(0)

( p,ω

)

(0)

( p + q,ω

)

(2π)





d p V (k − p)V (k



− p)

(0)

( p,ω

)

(0)

( p − k



− k,ω

). (3.104)

Because the density is low (equation (3.100)) we can neglect the q-

dependence of V (q) and take V (q) = 4πUr

/3 ≡ v in the second-order terms of

equation (3.104). Then the diagrams (b)and(c) + (d) cancel each other and the

second term in the right-hand side of equation (3.104) vanishes. The remaining

last term yields the familiar polarization bubble (equation (3.40)) which ﬁnally

leads to

≡−

V (l)mk

2π(2l + 1)

= λ

− λ(−1)



d sin P

(cos )



1 +

− q



+ q

− q





(3.105)

where λ = (ak

/π)

 1. Here the ﬁrst repulsive term is about

(ak

)(k

)

, where a = vm

/(2π) is the s-wave scattering amplitude in

Cooper pairing of repulsive fermions



G H

Figure 3.8. The ﬁrst (a) and second-order diagrams (b–e) which contribute to the screened

electron–electron interaction. Note that the non-crossing second-order diagram (f) as well

as all higher-order non-crossing diagrams are fully taken into account in the BCS equation

(section 3.4) and should not be included in the irreducible scattering vertex.

the Born approximation. The second ‘polarization’ contribution to effective

interaction is of the order of (ak

)

. Hence, if the gas parameter is sufﬁciently

small,

)

2l−3



(3.106)

the polarization contribution overcomes direct repulsion for all l

2and

it is attractive. For example, for l = 2 (d-wave pairing), the integral in

equation (3.105) yields

= λ

105

(8 − 11 ln 2) ≈ 0.015λ. (3.107)

The corresponding critical temperature is

≈ µ exp



−

0.015λ



(3.108)

which is practically zero at any λ<1. The situation is slightly better when

the repulsive potential U is strong (U  µ) (i.e. for hard-core spheres) [59].

Partial scattering amplitudes f

in a vacuum are of the order of r

)

in this

limit. They are small compared with the attractive polarization contribution to the

amplitudes (

)) starting from l = 1. Hence, at T = 0, hard-core fermions

with repulsive scattering in a vacuum are necessarily in a superﬂuid p-wave state.

Calculating the integral in equation (3.105) for l = 1, we obtain

= λ

(2ln2− 1) ≈ 0.15λ (3.109)

Intermediate-coupling theory

and

≈ µ exp



−

0.15λ



. (3.110)

The Fermi energy could be as large as µ = 10

KbutT

still remains very low

because λ  1 in the dilute limit.

Chapter 4

Strong-coupling theory

The electron–phonon coupling constant λ is about the ratio of the electron–

phonon interaction energy E

to the half-bandwidth D N(E

)

−1

(appendix A).

We expect [11] that when the coupling is strong (λ>1), all electrons in the

Bloch band are ‘dressed’ by phonons because their kinetic energy (<D)issmall

compared with the potential energy due to a local lattice deformation, E

, caused

by an electron. If phonon frequencies are very low, the local lattice deformation

traps the electron. This self-trapping phenomenon was predicted by Landau [60].

It has been studied in greater detail by Pekar [61], Fr¨ohlich [62], Feynman [63],

Devreese [64] and other authors in the effective mass approximation, which leads

to the so-called large polaron. The large polaron propagates through the lattice

like a free electron but with the enhanced effective mass. In the strong-coupling

regime (λ>1), the ﬁnite bandwidth becomes important, so that the effective

mass approximation cannot be applied. The electron is called a small polaron

in this regime. The self-trapping is never ‘complete’, that is any polaron can

tunnel through the lattice. Only in the extreme adiabatic limit, when the phonon

frequencies tend to zero, is the self-trapping complete and the polaron motion

no longer translationally continuous (section 4.2). The main features of the small

polaron were understood by Tjablikov [65], Yamashita and Kurosava [66], Sewell

[67], Holstein [68] and his school [69, 70], Lang and Firsov [71], Eagles [72]

and others and described in several review papers and textbooks [13, 64, 73–76].

The exponential reduction of the bandwidth at large values of λ is one of those

features (section 4.3). The small polaron bandwidth decreases with increasing

temperature up to a crossover region from the coherent small polaron tunnelling

to a thermally activated hopping. The crossover from the polaron Bloch states to

the incoherent hopping takes place at temperatures T ≈ ω

/2 or higher, where ω

is the characteristic phonon frequency. In this chapter, we extend BCS theory to

the strong-coupling regime (λ>1) with the itinerant (Bloch) states of polarons

and bipolarons.