Zdunkowski W., Bott A. Dynamics of the atmosphere: A course in theoretical meteorology
Подождите немного. Документ загружается.

16.3 Normal-mode considerations 481
In case of n = 0 the phase velocity c
0
=−c
R
=−β/k
2
x
. This is the zeroth normal
mode of the pure Rossby wave. This solution is consistent with the requirement
that pure Rossby waves form in the absence of vertical velocities. For n = 1, 2,...
higher-order vertical modes are obtained. The corresponding pure waves also prop-
agate from east to west since c
n
< 0, but they move more slowly than in case of
the zeroth normal mode. The amplitudes of the generalized velocities for normal
modes in this case differ from zero as shown in
B(p
r
) = C
2
sin(l
n
p
r
) = 0(16.53)
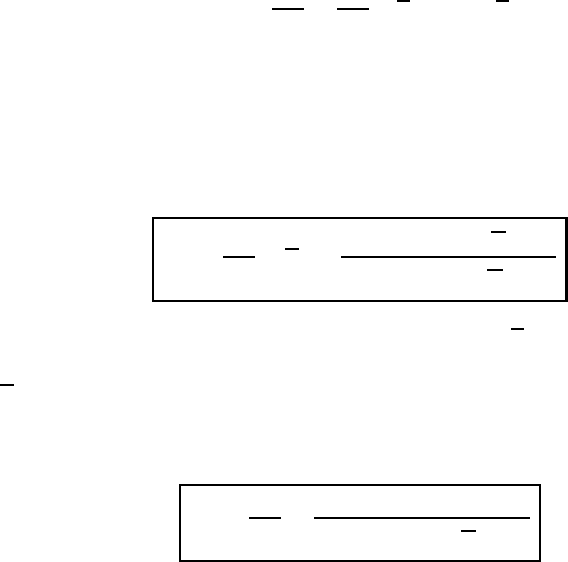
For large values of n equation (16.52) can be approximated by
c
n
≈−
a
2
c
R
n
2
π
2
c
2
I
=−
a
2
β
n
2
π
2
f
2
0
(16.54)
showing that c
n
no longer depends on the wavelength of the wave. This particular
situation is known as the ultra-long-wave approximation.
We will now show that the approximation leading to (16.54) is equivalent to ig-
noring the time-dependent term in the vorticity equation (16.44a). This assumption
implies an approximate balance between the β-effect and the divergence of the
flow. In fact, the expression ∂ω
/∂p represents vertical stretching, which is coupled
with the occurrence of divergence. This also follows directly from the continuity
equation in pressure coordinates which will be formally derived in a later chapter.
The mathematical development is the same as that above leading to the form
(16.49) but now l
2
(p
r
) has a different meaning so that we formally replace B(p
r
)
by B
1
(p
r
). Instead of (16.49) we now obtain
d
2
B
1
dp
2
r
+ l
2
1
B
1
= 0withl
2
1
=−
a
2
β
cf
2
0
(16.55)
Assuming that l
1
is a constant and that l
2
1
> 0, we again obtain a wave solution,
which is given by
B
1
(p
r
) = D
1
cos(lp
r
) + D
2
sin(lp
r
)(16.56)
By imposing the boundary conditions that the vertical velocity vanishes at the
top of the atmosphere and at the ground where p = p
0
, we immediately find the
conditions
B(p
r
= 0) = 0,B(p
r
= 1) = 0 =⇒ D
1
= 0,l= nπ, n = 0, 1,...
(16.57)
so that c
n
is given by
c
n
=−
a
2
β
n
2
π
2
f
2
0
(16.58)
482 Rossby waves
This verifies the above statement that ignoring the term a
2
in the denominator
of (16.52) is equivalent to ignoring the time-dependency in the vorticity equation
(16.44a).
16.4 Energy transport by Rossby waves
It is well known that the horizontal as well as the vertical transport of energy by
Rossby waves plays an important role in the maintenance of the general circulation
of the troposphere and the lower sections of the stratosphere. We have previously
shown that the direction of the group velocity coincides with the direction of the
transport of energy.
We begin by repeating the fundamental equation (14.91):
c
g,x
=
∂χ
∂k
x
=
∂ω
∂k
x
− u = c
g,x
− u (16.59)
giving the relation between the group velocity (indicated by a tilde), relative to the
basic current, and the intrinsic frequency χ. Using the basic frequency equations
(16.39), we obtain immediately the components of the group velocity for the three
directions. The specialization to lower dimensions is obvious. Note that, in case of
the vertical direction, we are dealing with a generalized velocity. From
c
g,x
=
∂ω
∂k
x
= u + β
k
2
x
− k
2
y
−
f
2
0
σ
0
k
2
p
k
2
x
+ k
2
y
+
f
2
0
σ
0
k
2
p
2
(16.60)
we see that, for pure Rossby waves (k
y
= k
p
= 0) and u = 0, the energy flux
is always downstream so that c
g,x
> 0. Comparison with (16.40a) shows that, for
u = 0, the phase and group velocities have opposite directions. In the general case,
however, c
g,x
may be either positive or negative so that energy may flow in both
directions.
From
c
g,y
=
∂ω
∂k
y
=
2βk
x
k
y
k
2
x
+ k
2
y
+
f
2
0
σ
0
k
2
p
2
(16.61)
we recognize that meridional transport of energy is possible only if k
y
differs from
zero.
The basic requirement for meridional transport of energy to occur is that the axis
of troughs (ridges) must be inclined with respect to the south–north direction. For
a southward transport (k
y
< 0) the axis must assume a direction from the south-
west to the north-east. In case of a northward transport of energy (k
y
> 0) the axis

16.5 The influence of friction on the stationary Rossby wave 483
must be directed from the south-east to the north-west. Comparison with equation
(16.40b) shows that, for pure Rossby waves, the phase velocity c
y
and the group
velocity c
g,y
are opposite in direction.
Let us now consider the vertical transport of energy. From
c
g,p
=
∂ω
∂k
p
=
2βk
x
f
2
0
/σ
0
k
p
k
2
x
+ k
2
y
+
f
2
0
/σ
0
k
2
p
2
(16.62)
it follows that, for a positive vertical wavenumber (k
p
> 0), the transport of energy
is upward. If the vertical wavenumber is negative (k
p
< 0), then the transport is
downward. For
u = 0 we recognize immediately that c
p
and c
g,p
have opposite
signs (see equation (16.40c)).
16.5 The influence of friction on the stationary Rossby wave
There is much observational evidence that Rossby waves may be amplified by
external forcing due to large-scale thermal inhomogeneities resulting, for example,
from the distribution of continents and oceans. Inspection of weather maps shows
that their dimensions are of the same order as the lengths of stationary Rossby
waves. Not only does forcing play an important role in the dynamics of the Rossby
waves, but also dissipating factors are of importance. As in any physical problem,
dissipation is usually very difficult to treat mathematically if realistic situations are
assumed. In order to assess the influence of friction between the atmosphere and
the earth’s surface, we will accept the simple Guldberg and Mohn scheme,which
assumes that the frictional force is proportional to the wind velocity. Therefore,
we must include the frictional effect in the vorticity equation. We proceed by
including on the right-hand sides of the horizontal components of the equation
of motion (15.27b) the terms −ru and −rv,wherer is a frictional factor. We
then derive the divergence-free vorticity equation analogously to (15.20), but now
the term rζ will be added. With Panchev (1985) we assume that we are dealing
with stationary conditions; we also ignore any y-dependence of the variables. This
leads to
u
∂ζ
∂x
+ βv + rζ = 0(16.63a)
In order to obtain an analytic solution, we linearize this equation with u =
constant,
v = 0 and obtain
u
dζ
dx
+ βv
+ rζ
= 0(16.63b)
Since ζ
= dv
/dx we obtain an ordinary second-order differential equation for v
:
u
d
2
v
dx
2
+ βv
+ r
dv
dx
= 0(16.64)
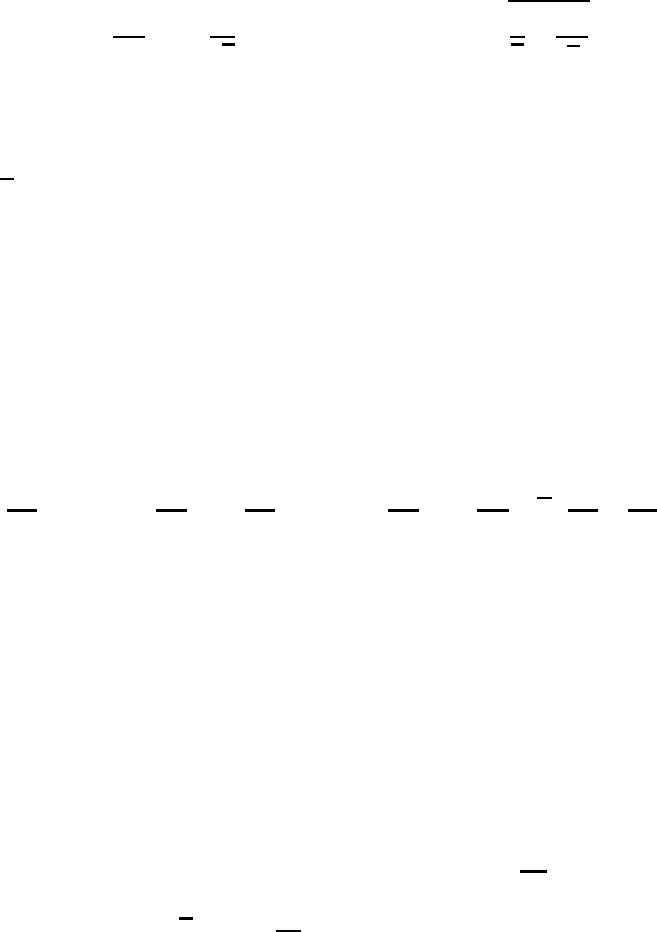
484 Rossby waves
Assuming that, at x = 0, the velocity v
= 0, and (dv
/dx)
0
= ζ
0
,whichisthe
initial vorticity, the solution to the frictional problem is given by
v
=
ζ
0
k
stat
exp
−
r
2u
x
sin(k
stat
x) with k
stat
=
β
u
−
r
2
4u
2
(16.65)
Comparison of L
stat
= 2π/k
stat
with (16.10) shows that the stationary wavenumber
has been modified due to the existence of surface friction. Accepting Panchev’s
value r = 10
−6
s
−1
, the amplitude of the wave decreases to about half its value for
u = 10 m s
−1
if x = 2L
stat
for a midlatitude situation. We may conclude that the
frictional effect surely counteracts quite efficiently any forcing that would produce
amplification of the Rossby wave.
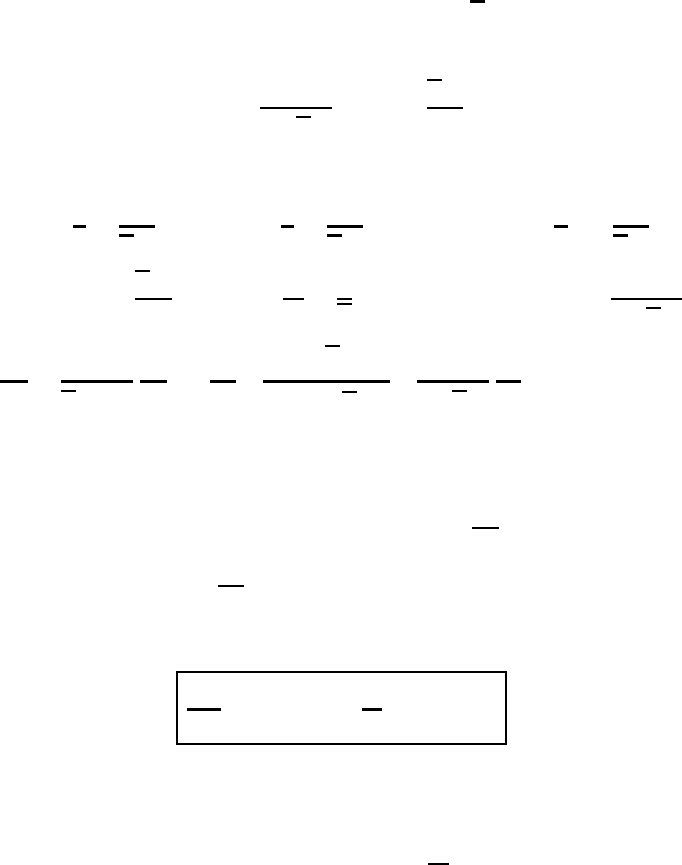
16.6 Barotropic equatorial waves
In this section we will briefly consider Rossby waves forming at the equator of the
earth. The starting points of the analysis are the shallow-water equations (15.23b)
and (15.27b), which will be linearized and simplified. Assuming that the basic
current is zero means that we must also set the inclination of the mean height of
the fluid equal to zero. For a flat ground the basic equations are
∂u
∂t
− βyv
=−
∂φ
∂x
,
∂v
∂t
+ βyu
=−
∂φ
∂y
,
∂φ
∂t
+
φ
∂u
∂x
+
∂v
∂y
= 0
(16.66)
The Coriolis parameter is approximated according to (16.1) with f
0
= 0. We wish
to obtain a solution of the form
u
v
φ
=
u(y)
v(y)
φ(y)
exp[i(k
x
x − ωt)] (16.67)
Substitution of this equation into (16.66) results in the coupled system
−iωu − βyv =−ik
x
φ, −iωv + βyu =−
d
φ
dy
,
−iω
φ +
φ
ik
x
u +
dv
dy
= 0
(16.68)
consisting of one purely algebraic equation and two differential equations. At this
point it is of advantage to introduce the dimensionless form of (16.68). The only
16.6 Barotropic equatorial waves 485
dimensional parameters in this set of equations are β and φ, which will be combined
to introduce scales of time and length:
τ =
1
β
1/2
φ
1/4
,l=
φ
1/4
β
1/2
(16.69)
These scales are then used to define the dimensionless variables
u =u
τ
l
=
u
φ
1/2
, v =v
τ
l
=
v
φ
1/2
, y =
y
l
= y
β
1/2
φ
1/4
k
x
= k
x
l = k
x
φ
1/4
β
1/2
,
φ =
φ
τ
2
l
2
=
φ
φ
, ω = ωτ =
ω
β
1/2
φ
1/4
d
φ
dy
=
1
φ
3/4
β
1/2
d
φ
dy
,
dv
dy
=
d
v/
φ
1/2
d
yβ
1/2
/φ
1/4
=
1
β
1/2
φ
1/4
dv
dy
(16.70)
By introducing these variables into (16.68), we find the equivalent dimensionless
set
−iωu −yv + i
k
x
φ = 0, −iωv +yu +
d
φ
dy
= 0,
−iω
φ + i
k
x
u +
dv
dy
= 0
(16.71)
Eliminating u and
φ by obvious steps yields
d
2
v
dy
2
+
ω
2
−
k
2
x
−
k
x
ω
−y
2
v = 0
(16.72)
This is Schr
¨
odinger’s equation for the simple harmonic oscillator. Application of
the natural conditions v(±∞) = 0 gives the general solution
v(y) = A
n
H
n
(y)exp
−
y
2
2
(16.73)
to (16.72) in terms of the Hermite polynomials H
n
(y) upon assuming the validity
of the dispersion relation
ω
2
−
k
2
x
−
k
x
/ω = 2n + 1,n= 0, 1,... (16.74)
The lowest-order Hermite polynomials are H
0
= 1,H
1
= 2y,andH
2
= 4y
2
− 2.
It will be observed that (16.74) is a cubic equation in the reduced form. Conditions
under which this equation provides three real and unequal roots are known. In the
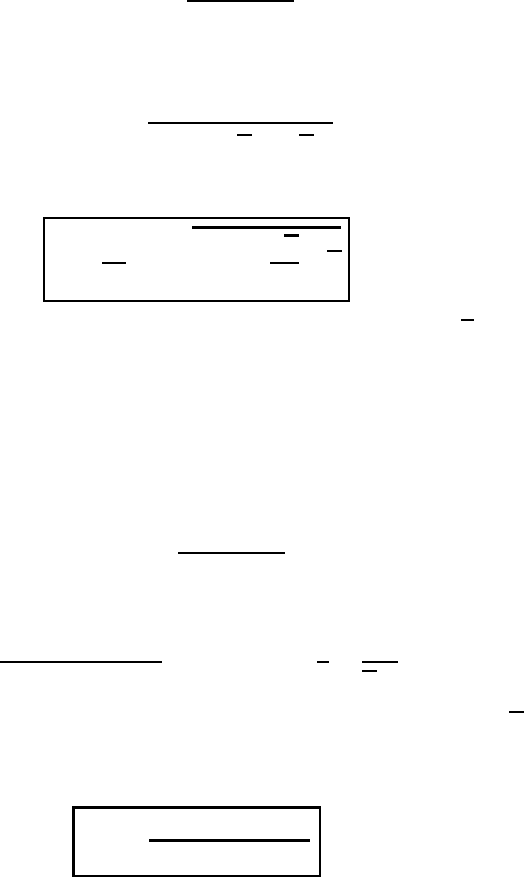
486 Rossby waves
present case the three real roots correspond to different types of waves. From the
type of the basic equations (16.66) we should expect two inertial gravity waves and
one Rossby wave. The solutions can be separated by considering two approximate
cases.
Let us consider the conditions stated for the first approximate case
ω
2
−
k
2
x
k
x
/ω (16.75)
so that the dimensionsless frequency equation (16.74) reduces to
ω
n
=±
2n + 1 +
k
2
x
(16.76)
The lowest possible frequency is found by setting n = 0. On returning to the
dimensional form by means of (16.70) we obtain
ω
n
=±
(2n + 1)βφ
1/2
+ φk
2
x
(16.77)
The phase speeds of the two waves are given by
c
n
=
ω
n
k
x
=±
(2n + 1)
βφ
k
2
x
+ φ
(16.78)
Recalling that at the equator the Coriolis parameter is very small, for
u = 0this
equation is very similar to (15.55). Clearly, (16.78) describes a pair of eastward-
and westward-propagating inertial gravity waves.
The conditions for the second approximate case are
ω
2
k
x
/ω +
k
2
x
(16.79)
so that (16.74) reduces to the frequency equation
ω
n
=−
k
x
k
2
x
+ 2n + 1
(16.80)
The dimensional form is given by
ω
n
=−
βk
x
k
2
x
+ (2n + 1)k
2
scale
with k
scale
=
1
l
=
β
1/2
φ
1/4
(16.81)
Here the wavenumber scale k
scale
has been introduced. Assuming that φ =
10
5
m
2
s
−2
, the corresponding wavelength scale l is about 4000 km. By divid-
ing ω
n
by k
x
we obtain the phase speed
c
n
=−
β
k
2
x
+ (2n + 1)k
2
scale
(16.82)
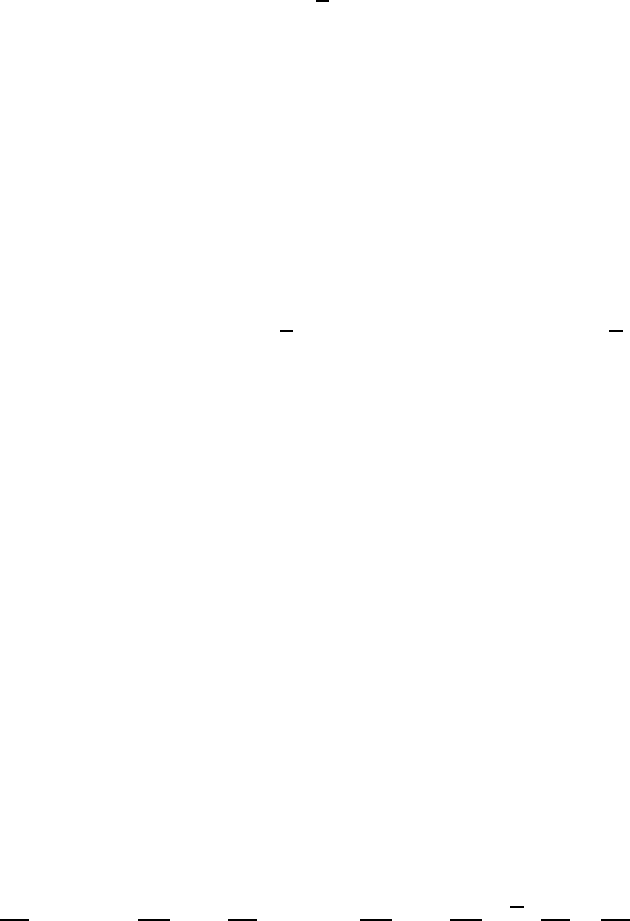
16.7 The principle of geostrophic adjustment 487
We observe that, unlike the inertial gravity waves, this type of wave is unidirectional
and propagating in the westward direction only, as is implied by the negative sign.
Therefore, we are dealing with a Rossby-type wave since all Rossby waves move
from east to west if the basic current
u = 0, as follows from (16.9). This section
originates from the work of Matsuno (1966).
16.7 The principle of geostrophic adjustment
In the previous chapter we considered the properties of the homogeneous atmo-
sphere by discussing the solution of the system (15.49). We found that the frequency
equation has three real roots. One of these represents the meteorologically inter-
esting wave moving with a speed c
1
, see (15.53), which is of the order of the wind
velocity. This is not an actual Rossby wave since the Coriolis parameter f was not
permitted to vary with latitude. Nevertheless, loosely speaking, we sometimes call
the slow-moving waves displaced with c
1
also Rossby waves. Had we permitted
f to vary with the coordinate y,
u would have had to be replaced by u − β/k
x
.
The remaining two roots resulted in the phase velocities c
2,3
of the external gravity
waves, see (15.55), moving at approximately the Newtonian speed of sound.
The solution of the predictive meteorological equations requires a complete
set of initial data. These observational data are usually measured independently
with a certain observational error. In contrast, the meteorological variables are
connected by a system of prognostic and diagnostic equations that must be satisfied
by the meteorological variables at all times, including the initial time. Owing to
observational errors, the initital data will introduce a perturbation, which cannot be
completely avoided. Observational evidence shows that the large-scale atmospheric
motion is quasi-geostrophic and quasi-static, implying a balance among the Coriolis
force, the pressure-gradient force, and the gravitational force. If this balance is
disturbed in some region by frontogenesis or by some other phenomenon, fast
wave motion is generated and perturbation energy is exported to other regions of
the atmosphere. After a period of adjustment the quasi-balance is restored. In fact,
this process of adjustment operates continually to maintain a state of approximate
geostrophic balance.
Let us reconsider the linearized shallow-water equations assuming that we have
a resting basic state. From (15.23b) with φ
s
= 0 and from (15.27b) we obtain
directly
∂u
∂t
− fv
=−
∂φ
∂x
,
∂v
∂t
+ fu
=−
∂φ
∂y
,
∂φ
∂t
+
φ
∂u
∂x
+
∂v
∂y
= 0
(16.83)
We could have found this equation also from (15.66) by replacing β by f .Inorder
to simplify the notation, the primes on u, v,andφ will be omitted henceforth. Note
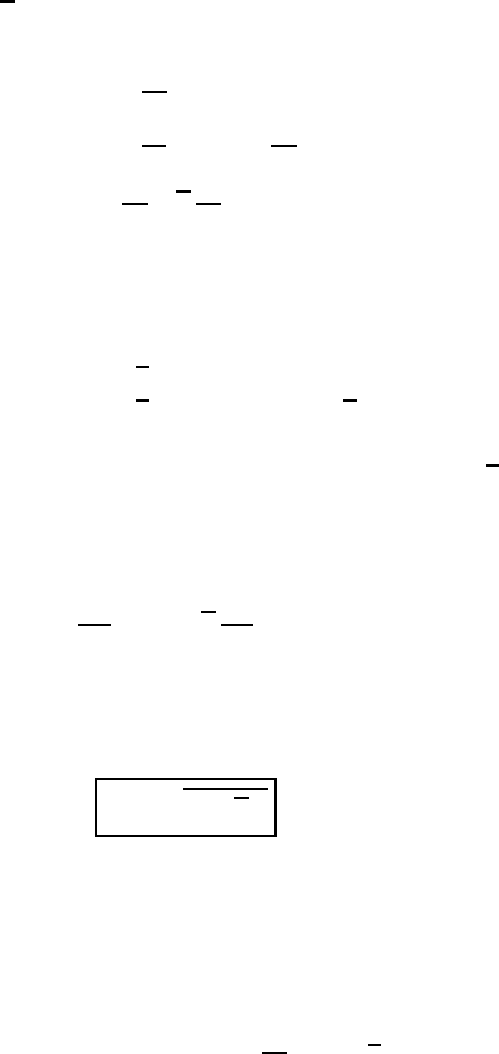
488 Rossby waves
well that the gradient of the mean geopotential must be zero for a resting mean
state to exist, that is φ = constant. In order to simplify the system we set ∂/∂x = 0,
thus obtaining a problem in one-dimensional space:
(a)
∂u
∂t
− fv = 0
(b)
∂v
∂t
+ fu =−
∂φ
∂y
(c)
∂φ
∂t
+
φ
∂v
∂y
= 0
(16.84)
It turns out that the result we are going to obtain is quite general and does not
depend on the dimension of the adjustment process. For the treatment of the
higher-dimensional adjustment processes see Panchev (1985).
The initial conditions will be specified by
v(y,t = 0) =
v = 0, ∇φ(y, t = 0) = 0
|
y
|
≤ a: u(y, t = 0) =
u,
|
y
|
>a: u = 0
(16.85)
We need to point out that the initial fields of the velocity and the geopotential are
not balanced since, on the line segment
|
y
|
≤ a, the basic state velocity
u = 0
while the gradient of the geopotential φ is assumed to vanish. This inconsistency
stimulates a disturbance that will propagate away from the region of imbalance.
We proceed by eliminating u and φ in (16.84) and obtain the partial differential
equation
∂
2
v
∂t
2
+ f
2
v − φ
∂
2
v
∂y
2
= 0(16.86)
If we seek a solution of the form
v = V exp[i(k
y
y − ωt)],V= constant (16.87)
we find the frequency equation
ω =±
f
2
+ φk
2
y
(16.88)
This dispersion relation shows that inertial gravity waves, which will eventually
propagate out of the imbalance region, are generated. After a period of adjustment
the geostrophic balance will be restored.
In order to study the adjustment process itself, it is not sufficient to consider the
frequency equation; we must actually solve an initial-value problem. We will now
solve equation (16.86) subject to the initial conditions
t = 0,
|
y
|
≤ a: v(y, 0) = 0,
∂v
∂t
t=0
=−uf (16.89)
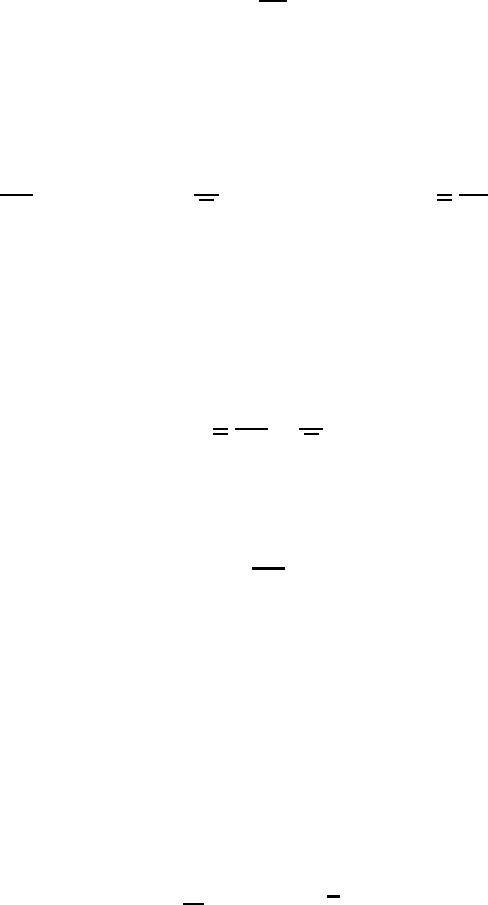
16.7 The principle of geostrophic adjustment 489
noting that the second condition is formulated with the help of (16.84) and (16.85).
The method of solution we choose is based on operational calculus. By introducing
the pair of Fourier transforms
(a) v(k
y
,t) =
∞
−∞
v(y,t)exp(−ik
y
y) dy
(b) v(y, t) =
1
2π
∞
−∞
v(k
y
,t) exp(ik
y
y) dk
y
(16.90)
we transform the partial differential equation (16.86) into an ordinary second-order
differential equation. We could also apply the Laplace-transform method to find
the solution to this equation. Multiplying (16.86) by exp(−ik
y
y) and integrating
over y from −∞ to +∞ as required by (16.90a), we obtain
∞
−∞
∂
2
v
∂y
2
exp(−ik
y
y) dy =
f
2
φ
∞
−∞
v exp(−ik
y
y) dy +
1
φ
∂
2
∂t
2
∞
−∞
v exp(−ik
y
y) dy
(16.91)
We have extracted the partial derivative with respect to time from under the integral
sign since the integration is over y and the limits of the integral are independent
of t. In the appendix to this chapter it is briefly shown how to take the Fourier
transform of a derivative and of a constant. With reference to the appendix, see
equation (16.117), we immediately find the differential equation
1
φ
d
2
v
dt
2
+
f
2
φ
v = (ik
y
)
2
v (16.92)
in the transformed plane, which is now an ordinary differential equation. Using
(16.88), we may write equation (16.92) more succinctly as
d
2
v
dt
2
+ ω
2
v = 0(16.93)
The solution to this equation can be written down immediately:
v(k
y
,t) = A(k
y
)cos(ωt) + B(k
y
)sin(ωt)(16.94)
Since the differentiation variable is the time t, the integration constants, in general,
should depend on k
y
.
From the boundary condition (16.89) it follows that
v(k
y
, 0) = 0 =⇒ A(k
y
) = 0(16.95a)
From (16.89) we obtain
∂
∂t
[v(y,0)] =−
uf = constant (16.95b)
490 Rossby waves
so that from (16.120) derived in the appendix we find the integration constant
B(k
y
):
∂
∂t
∞
−∞
v(y,0) exp(−ik
y
y) dy =
∂
∂t
[v(k
y
, 0)] =−
2uf
k
y
sin(k
y
a)
=⇒ B(k
y
) =−
2uf
k
y
ω
sin(k
y
a)
(16.96)
Hence, v(k
y
,t) is known. The Fourier integral (16.90b) then provides the solution
to the differential equation (16.93), which is given by
v(y,t) =−
uf
π
∞
−∞
sin(k
y
a)sin(ωt)
k
y
ω
exp(ik
y
y) dk
y
(16.97)
By splitting the integral into two parts ranging from −∞ to 0 and from 0 to +∞
we get the final form of the solution:
v(y,t) =−
2uf
π
∞
0
sin(k
y
a)sin(ωt)
k
y
ω
cos(k
y
y) dk
y
(16.98)
The imagninary part of (16.97) vanishes, as can readily be verified. If desired,
u(y,t)andφ(y, t) can easily be found from (16.84a) and (16.48c).
We now direct our attention to the behavior of v(y,t)ast →∞. For convenience
we consider the central point y = 0, and then change the integration variable from
k
y
to ω using the positive root (16.88). After a few obvious steps we find
v(0,t) =−
2
uf
φ
∞
f
ω sin
a
√
γ
γ
sin(ωt)
πω
dω =−
2
uf
φ
∞
f
g(ω)
sin(ωt)
πω
dω
(16.99)
where the abbreviations γ = (ω
2
− f
2
)/φ and g(ω) = ω sin
a
√
γ
/γ have been
introduced. The integrand is quite suitable for use of the Dirac delta function in the
form
δ(ω) = lim
t→∞
sin(ωt)
πω
(16.100)
In the limit t →∞we may therefore write for (16.99)
v(0,t →∞) =−
2
uf
φ
∞
f
g(ω)δ(ω − 0) dω = 0(16.101)
where the integral has been evaluated by means of (M6.80). We obtain the identical
result for the negative sign of the root in (16.88).
