Zdunkowski W., Bott A. Dynamics of the atmosphere: A course in theoretical meteorology

Подождите немного. Документ загружается.

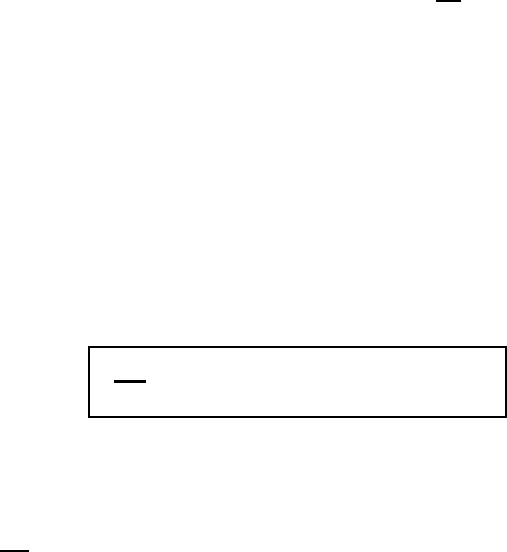
15.2 The unﬁltered barotropic prediction model 441

For this purpose let us formally rewrite equation (15.7) as ∂v

/∂t = F(x, y,t),

where the right-hand side is independent of height. Let us now formally carry

out the integration over time. After the ﬁrst time step t theright-handsideis

height-independent again:

(x,y, t

+ t) = v

(x,y, t

) + t F(x,y, t

)(15.17)

On repeating this procedure for the following time steps the right-hand side remains

height-independent. Hence, it may be concluded for the barotropic model that the

horizontal wind remains height-independent if it is height-independent initially.

15.2.2 The barotropic model of the homogeneous atmosphere

In place of the surface pressure p

(x,y, t) it is of advantage to introduce the height

of the free surface H (x,y, t) as a model variable. In order to construct a ﬁnite-

height model it is necessary to specify the barotropy function ρ(p)inasuitable

manner. The condition of barotropy must not necessarily be invertible to guarantee

barotropy. The simplest condition of barotropy is given by assuming that we have

a homogeneous model atmosphere speciﬁed by

ρ = ρ

= constant, ∇ρ = 0,

dρ

= 0(15.18)

For a ground temperature of 273 K the pressure scale height or the height of the

homogeneous atmosphere is about 8000 m. Instead of the height of the free surface

itself we may just as well use the corresponding geopotential so that the upper and

lower boundaries of the model atmosphere are speciﬁed by

φ(x,y, t) = gH(x,y,t),φ

(x,y) = gz

(x,y)(15.19)

Owing to the constant-density assumption the surface pressure is given by

= gρ (H − z

) = ρ(φ − φ

)(15.20)

Introducing this expression into (15.7) leads to the equation of horizontal motion

assuming the form



∂v

∂t





+ v

·∇

+ f i

× v

+∇

φ = 0

(15.21)

Owing to (15.18) the continuity equation (15.8) reduces to the statement that the

three-dimensional velocity divergence vanishes. Height integration of the reduced

continuity equation between the lower and upper boundaries gives



∂w

∂z

dz = w(H ) − w(z

) =−∇

· v



dz =−(H − z

) ∇

· v

(15.22)

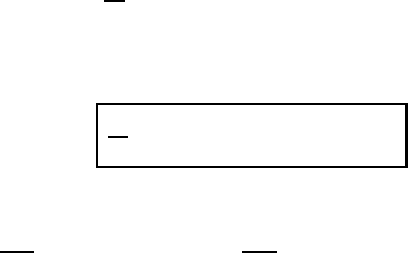
442 The barotropic model

since the horizontal velocity is height-independent. By introducing the kinematic

boundary conditions (15.10) into (15.22) and recalling that z

is time-independent

we obtain

∂

∂t

(H − z

) =−∇

· [v

(H − z

)] (15.23a)

Multiplying this equation by the acceleration of gravity results in the corresponding

expressions for the geopotentials:

∂

∂t

(φ − φ

) =−∇

· [v

(φ − φ

)]

(15.23b)

By multiplying both sides of (15.23a) by the constant model density, we obtain

∂ρ

∂t

=−∇

· (v

)or

dρ

+ ρ

∇

· v

= 0(15.23c)

where ρ

= ρ(H − z

) is the density per unit area. This form of the continuity

equation for the two-dimensional space closely resembles the normal continuity

equation (15.8) for the three-dimensional space, where the density represents mass

per unit volume.

Let us consider the prognostic set (15.21) and (15.23b), where the density does

not appear at all as a parameter. At ﬁrst glance there seems to be no connection

between the model variables φ(x,y, t) and the horizontal velocity v

(x,y, t)onthe

one hand and the variables of the real atmosphere on the other. It is postulated and

veriﬁed by experience that the height-independent horizontal wind vector of the

model atmosphere is a reasonable approximation to the height-averaged wind ﬁeld

of the real atmosphere. This wind is assumed to apply to the so-called equivalent

barotropic level, which for practical reasons is taken as the 500-hPa pressure level.

As mentioned previously, this surface is located near the level of nondivergence

which approximately divides the mass of the atmosphere into two equal parts. This

results in the following correspondence of variables:

= v(p = 500 hPa),φ= φ(p = 500 hPa) (15.24)

Various empirical reductions of the geopotential of the earth’s surface may be

necessary in order to prevent the orography modifying the predicted ﬁelds too

strongly. These modiﬁcations are based on experience and are of entirely empirical

character.

The model consisting of equations (15.21) and (15.23b) is best described by a

cylinder ﬁlled with water having a free surface that is rotating about its vertical

axis of symmetry. The assumption of autobarotropy is satisﬁed reasonably well

by pure water and deviations from the hydrostatic pressure are small as long as

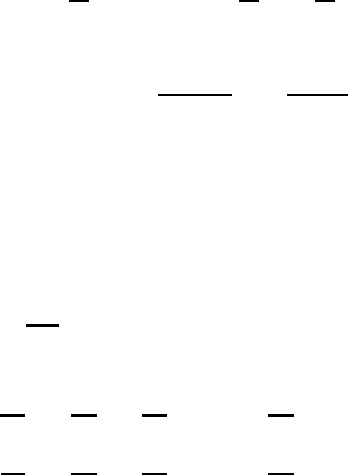
15.2 The unﬁltered barotropic prediction model 443

the ﬂow velocities are not too large. Height-independent horizontal velocities,

however, cannot be realized in general. In this rotating cylinder characteristic

ﬂow patterns evolve with embedded vortices. In addition to this, one observes

expanding ring-type waves of short periods, which are superimposed on the larger-

scale characteristic ﬂow patterns.

In close correspondence, the model equations of the homogeneous atmosphere

describe relevant characteristic synoptic ﬂow patterns and the displacement of

ridges and troughs. Superimposed on the large-scale features are high-speed ex-

ternal gravity waves of short periods. These waves, which we have described as

“meteorological noise,” have little direct inﬂuence on the processes associated with

the formation of the synoptic waves. As mentioned before, due to the existence

of the rapidly moving external gravity waves, the time integration of the model

necessitates the use of very small time steps. Moreover, the amplitudes of the noise

waves may be large enough to falsify meteorologically relevant results. In a later

section we will brieﬂy discuss how to ﬁlter out the noise waves without signiﬁcantly

modifying the synoptic waves.

15.2.3 The energy budget of the homogeneous model and the available

potential energy

First of all we deﬁne the kinetic energy



dz = ρ(H − z

)

= ρ

(15.25)

and the potential energy



gρz d z = gρ

− z

= ρ

φ + φ

(15.26)

contained in a vertical column of unit cross-sectional area. These deﬁnitions are

then used to set up the budget equations. It will be shown that only limited amounts

of potential energy can be transformed into kinetic energy, otherwise the principle

of conservation of mass would be violated. The amount of potential energy that

can be transformed into kinetic energy is known as the available potential energy.

First we rewrite (15.21) as







+ f i

× v

=−∇

φ (15.27a)

and, in component form, as

∂u

∂t

+ u

∂u

∂x

+ v

∂u

∂y

− fv =−

∂φ

∂x

∂v

∂t

+ u

∂v

∂x

+ v

∂v

∂y

+ fu =−

∂φ

∂y

(15.27b)

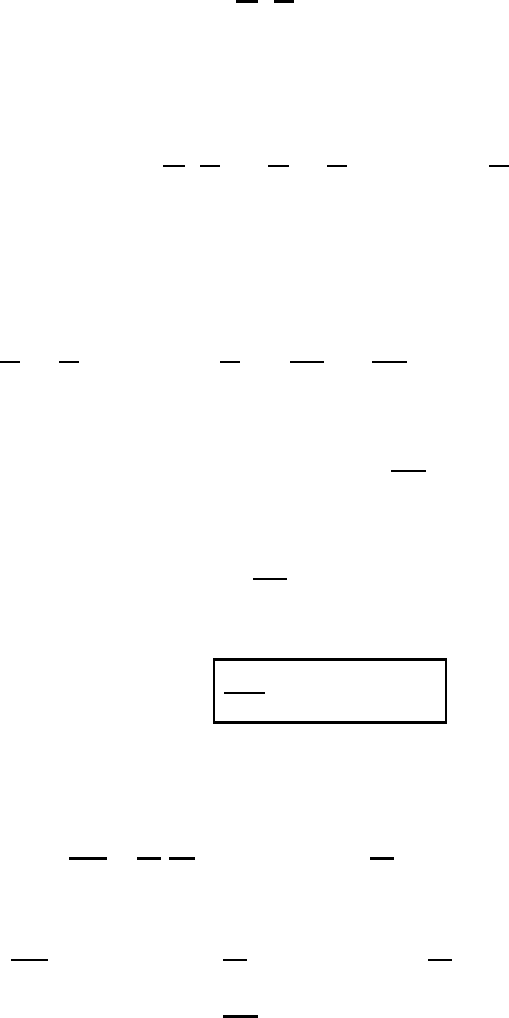
444 The barotropic model

Scalar multiplication of (15.27a) by ρ

, using simpliﬁed notation, gives





=−ρ

·∇

φ (15.28)

As expected, the Coriolis term vanishes since the Coriolis force cannot perform

any work. The left-hand side of (15.28) may be rewritten in budget form. With the

help of the two-dimensional continuity equation (15.23c) we ﬁnd immediately





∂

∂t





+∇





(15.29)

Expressing the geopotential in terms of ρ

= ρ(H − z

), equation (15.19) yields

φ = φ

+ gρ

/ρ (15.30)

After a slight rearrangement of terms we obtain from (15.28)

∂

∂t





+∇



+ v

gρ

2ρ



gρ

2ρ

∇

· v

− ρ

·∇

(15.31)

which has the desired budget form. Denoting the ﬂux of the kinetic energy by

= v

+ v

gρ

2ρ

(15.32)

and the corresponding source by

gρ

2ρ

∇

· v

− ρ

·∇

(15.33)

we may write the budget equation for the kinetic energy in the usual form

∂K

∂t

+∇

· F

= Q

(15.34)

Next we derive the budget equation for the potential energy by ﬁrst multiplying

(15.23c) by the factor (φ + φ

)/2. Recalling that φ

is independent of time, we

obtain immediately

∂P

∂t

−

∂φ

∂t

=−∇

· (v

) +

·∇

(φ + φ

)(15.35)

Replacing ∂φ/∂t in this expression by means of (15.23b) yields

∂P

∂t

+∇

· (v

) =−

∇

· [v

(φ − φ

)] +

·∇

(φ + φ

)

=−

gρ

2ρ

∇

· v

+ ρ

·∇

= Q

(15.36)

15.2 The unﬁltered barotropic prediction model 445

where (15.30) was used to obtain the second equation. In (15.36) the source term

of the potential energy of the column has been introduced. Comparison of Q

with Q

as given by (15.33) shows that Q

=−Q

. This yields the desired

budget equation

∂P

∂t

+∇

· (v

) =−Q

(15.37)

If Q

> 0 then kinetic energy is produced from potential energy and vice versa.

We will now show that only a limited amount of the potential energy P

contained

in a column of air can be transformed into kinetic energy due to the constraint of

conservation of mass. Let us decompose the height of the free surface H into its

mean value

H and the ﬂuctuation H



H =

H + H



(15.38)

According to equation (15.26) the potential energy is given by

gρ



+ 2HH



+ H

2

− z



(15.39)

Let us now consider a materially closed region of cross-section S. On averaging

the potential energy we ﬁnd

gρ





+ 2HH



+ H

2

− z





(15.40)

This equation may be simpliﬁed since the average over the ﬂuctuation must vanish:







HdS



−



HdS



= H − H = 0(15.41)

Therefore, the average value of the potential energy within the domain deﬁned by

the upper and lower boundaries as well as by the side walls is given by

gρ



+ H

2

− z



with

2



2



(15.42)

Since the quantity

− z

is ﬁxed, it deﬁnes the minimum amount of potential

energy contained within the region:

min

gρ



− z



(15.43)

The potential energy which is free or available for transformation into kinetic

energy leads to the deﬁnition of the available potential energy,

= P

− P

min

gρH

2

(15.44)

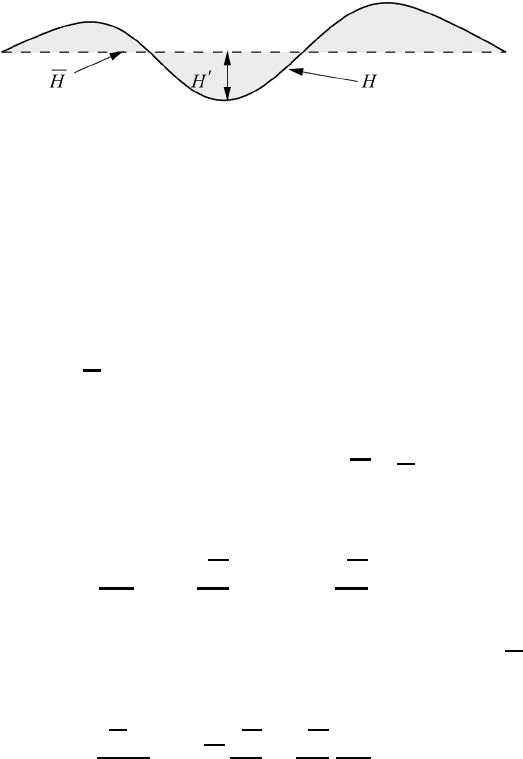
446 The barotropic model

Fig. 15.2 Available potential energy.

which is proportional to the mean value of the square of the ﬂuctuation; see

Figure 15.2.

It will be recognized that the transformation is solely due to the appearance of

short-periodic noise processes. This transformation has nothing to do with baro-

clinic developments, whereby the kinetic energy is gained from potential energy

due to a rearrangement of cold and warm air in the same volume.

To complete the discussion, we must show that the potential energy cannot

become smaller than

min

if the principle of conservation of mass is not violated.

The mass contained within the volume is deﬁned by

M =



ρ(H − z

) dS



= ρS(H − z

)(15.45)

From

∂M

∂t

= ρS

∂

∂t

= 0or

∂

∂t

= 0(15.46)

it follows that conservation of mass is equivalent to the statement that the average

height of the model region does not change with time. Therefore,

min

cannot

change with time either:

∂

min

∂t

= gρ H

∂

∂t

∂M

∂t

= 0(15.47)

We will close this section by remarking that the available potential energy of

baroclinic processes has been investigated by E. Lorenz (1955) and van Miegham

(1973). For adiabatic processes invariance of the potential temperature must be

observed.

15.2.4 Properties of the homogeneous model

In order to survey all types of wave motions admitted by the barotropic model,

we apply the linearization method described in the previous chapter to the basic

model equations (15.21) and (15.23b). For simplicity we ignore the occurrence

of orographic effects by setting φ

= 0. Furthermore, we assume that we have a

15.2 The unﬁltered barotropic prediction model 447

stationary basic state (denoted by the overbar) that is independent of the coordinates

(x,t) and we hold the Coriolis parameter constant, i.e. f ≈ f

u =−

∂φ

∂y

φ(y) = gH (y)(15.48)

Now we are able to obtain analytic solutions that are easy to interpret. Superimposed

on the basic current are the periodic disturbances u



(x,t),v



(x,t)andφ



(x,t). This

results in the basic set of equations describing the motion and the mass continuity:

∂u



∂t

∂u



∂x

∂φ



∂x

− f



= 0

∂v



∂t

∂v



∂x

+ f



= 0

∂φ



∂t

∂φ



∂x

∂u



∂x

−



= 0

(15.49)

Here the geopotential of the mean height

φ(y) has been approximated by an average

value φ

= constant. This is not quite consistent mathematically but it makes it

possible to take a solution of the form





















∗







exp[ik

(x − ct)] (15.50)

where the amplitudes are constants. Substitution of (15.50) into (15.49) results in

the cubic equation

(c −

−





(c − u) −

u = 0(15.51)

from which the unknown phase velocities can be determined. This cubic equation

in the variable c −

u is in the reduced form of the general cubic equation. The

coefﬁcients in the reduced form (15.51) satisfy a condition that guarantees the

existence of three real solutions. One of the three waves, whose phase speed,

say c

, is of the order of the mean wind speed u, is of meteorological relevance.

Therefore, we may apply the inequality

− u

 φ + f



(15.52)

to obtain an approximate expression for c

. Owing to (15.52) the ﬁrst term in

(15.51) may be neglected in comparison with the second term and we obtain the

448 The barotropic model

approximate solution for c

≈ u

φ + f



(15.53)

Inspection shows that shorter waves (L

< 3000 km) move practically with the

mean wind speed

u whereas longer waves move with a lesser speed. The remaining

two waves c

2,3

are external or surface gravity waves moving approximately with

the Newtonian speed of sound so that the inequality

2,3

− u)

 uf



(15.54)

is satisﬁed. Therefore, in obtaining c

2,3

, the last term in (15.51) may be neglected.

The resulting phase speeds are approximately given by

2,3

= u ±



φ + f



(15.55)

demonstrating that the gravity waves are moving in the positive and negative

x-directions. Comparison with equation (14.113) shows that the speed of the grav-

itational surface waves is slightly modiﬁed due to the rotation of the earth which

resulted in the term f

under the square root in (15.55).

Finally, by neglecting gravitational effects, i.e. putting g = 0, and by setting

u =0 we obtain the so-called inertial waves. In this case (15.49) reduces to two

equations for the components of the horizontal wind ﬁeld:

∂u



∂t

− f



= 0,

∂v



∂t

+ f



= 0(15.56)

Taking the solution in the form (15.50), we ﬁnd the frequency equation



−ik

−f

−ik



=−k

+ f

= 0(15.57)

The phase speed for pure inertial waves is then

=±f

(15.58)

For midlatitudes and a wavelength of 3000 km the phase speed c

is about 50 m s

−1

In a later chapter we will discuss the inertial waves in more detail.

15.2 The unﬁltered barotropic prediction model 449

15.2.5 Adaptation of the initial data

In the previous section we have shown that the barotropic model equations admit

two different types of processes. These are noise processes and the so-called me-

teorologically relevant Rossby processes. On weather maps drawn entirely on the

basis of observational data, noise processes are totally absent. Therefore, it is desir-

able to eliminate noise processes that might be produced by numerical procedures

of weather prediction.

A well-proven method for eliminating noise processes from the solutions of the

barotropic model equations (15.21) and (15.23) is to require that, at time t = 0, the

divergence of the wind vector and its time derivative vanish:

h,0

= (∇

· v

)

t=0

= 0,



∂D

∂t



t=0

= 0(15.59)

These ﬁlter conditions do not inﬂuence the prognostic system. What happens is that

the application of (15.59) restricts the free speciﬁcation of the initial wind velocity

(t = 0) and the geopotential φ(t = 0). To assure that the condition (15.59) truly

holds we introduce the stream function ψ, see equation (7.21), by means of

(t = 0) = i

×∇

ψ or u(t = 0) =−

∂ψ

∂y

,v(t = 0) =

∂ψ

∂x

(15.60)

Simple substitution veriﬁes that D

is zero. The wind deﬁned by this equation is

tangential to the lines ψ

= constant. The geostrophic wind possesses the same

property, but in this case the geopotential assumes the role of the stream function.

In order to apply (15.59) we must derive an expression wherein the divergence

appears explicitly. This is done by scalar multiplication of the equation of motion

(15.21) by the two-dimensional nabla operator. The result is

∂D

∂t

+∇

··∇

·∇

+∇

·(f i

×v

) =−∇

·∇

φ =−∇

φ (15.61)

where now the divergence and its time derivative appear explicitly so that the

condition (15.59) can be enforced. According to

∇

··∇

= D

− 2J (u, v)withJ (u, v) =



∂u

∂x

∂v

∂x

∂u

∂y

∂v

∂y



(15.62)

the double scalar product in (15.61) can be replaced, resulting in

∂D

∂t

+ D

− 2J (u, v) + v

·∇

+∇

· (f i

× v

) =−∇

φ (15.63)

450 The barotropic model

Here we have also introduced the Jacobian J for brevity. This is the barotropic

divergence equation.Fort = 0, by applying the conditions (15.59) we obtain the

so-called balance equation

−2J (u, v)



t=0

+∇

· (f i

× v

)



t=0

=−∇



t=0

(15.64a)

Without the nonlinear term 2J (u, v)|

t=0

the balance equation reduces to the

geostrophic wind relation, as may be easily veriﬁed.

In order to insure that the divergence of the horizontal wind vector is zero, we

have to introduce the stream function (15.60) into (15.64a) . This results in

−2J



∂ψ

∂x

∂ψ

∂y



−∇

· (f ∇

ψ) =−∇



t=0

(15.64b)

or, by expanding the Jacobian, we ﬁnd



−

∂

∂x

∂

∂y



∂

∂x ∂y





− f ∇

ψ −∇

f ·∇

ψ =−∇



t=0

(15.64c)

This is a partial differential equation of the Monge–Amp

ere type.

We summarize: Before starting the integration over time of the barotropic pre-

diction equations (15.21) and (15.23), it is necessary to solve the balance equation

(15.64c). From a given geopotential ﬁeld φ(t = 0) the stream function ψ must be

calculated, from which the initial velocities u(t = 0) and v(t = 0) can be obtained

by using (15.60). Now the wind ﬁeld is free from divergence, and it is so adjusted to

the geopotential ﬁeld φ(t = 0) that the time derivative of the divergence, as required

by (15.59), also vanishes at time t = 0. This mathematical process suppresses the

undesirable noise processes.

We will not discuss the numerical evaluation of the prediction equations and

of the balance equation but leave this to textbooks on numerical weather predi-

ction.

15.3 The ﬁltered barotropic model

By assuming that the conditions listed in (15.59) are valid at all times, not only at

t = 0, we obtain the so-called ﬁltered barotropic model wherein the high-speed

gravity waves are completely eliminated. The model condition is now given by

=∇

· v

= 0fort ≥ 0(15.65)