Zdunkowski W., Bott A. Dynamics of the atmosphere: A course in theoretical meteorology
Подождите немного. Документ загружается.

M4.2 Transformation relations of time-dependent coordinate systems 71
of the absolute kinetic energy in this system is available. In later chapters these
expressions will be very useful for us.
M4.2.2 Orthogonal q
i
systems
We recall from Section M1.5 that, in the case of orthogonal q
i
systems, there exist
some important simplifications, that is g
ij
= 0fori = j , g
ii
g
ii
= 1, and e
i
= e
i
.
Furthermore, if orthogonal systems are employed, it is advantageous to use unit
vectors instead of basis vectors. Whenever the time derivative of a unit or basis
vector is taken, we should expect some relationship involving the velocity v
P
.
The local time derivative of the unit vector e
i
follows from the local time deriva-
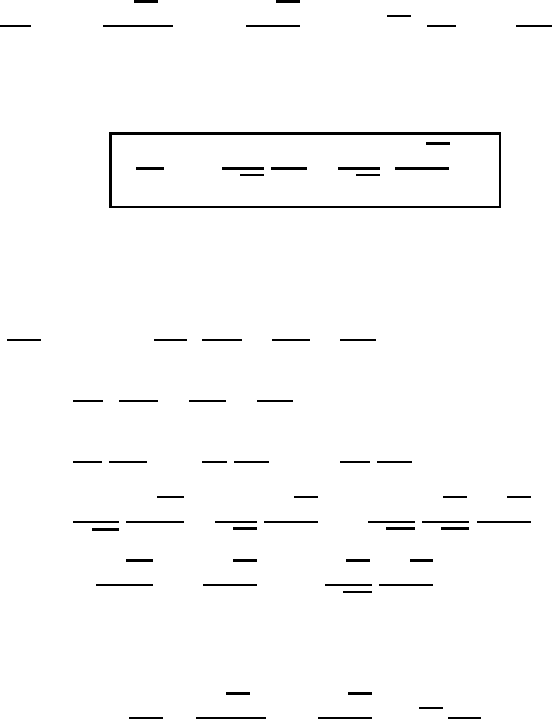
tive of the basis vector,
∂q
i
∂t
q
i
=
∂
√
g
ii
e
i
∂t
q
i
=
∂
√
g
ii
∂t
q
i
e
i
+
√
g
ii
∂e
i
∂t
q
i
=
∂v
P
∂q
i
(M4.41)
Thus, we have
∂e
i
∂t
q
i
=
1
√
g
ii
∂v
P
∂q
i
−
1
√
g
ii
∂
√
g
ii
∂t
q
i
e
i
(M4.42)
In order to find the spatial derivatives of e
i
, we return to equation (M3.44) and
introduce the condition of orthogonality. This results in
∂q
i
∂q
j
=
m
ij
q
m
=
g
mn
2
∂g
jn
∂q
i
+
∂g
in
∂q
j
−
∂g
ij
∂q
n
q
m
=
g
nn
2
∂g
jn
∂q
i
+
∂g
in
∂q
j
−
∂g
ij
∂q
n
q
n
=
g
jj
2
∂g
jj
∂q
i
q
j
+
g
ii
2
∂g
ii
∂q
j
q
i
− δ
j
i
g
nn
2
∂g
ii
∂q
n
q
n
=
q
j
√
g
jj
∂
√
g
jj
∂q
i
+
q
i
√
g
ii
∂
√
g
ii
∂q
j
− δ
j
i
q
n
√
g
nn
√
g
ii
√
g
nn
∂
√
g
ii
∂q
n
= e
j
∂
√
g
jj
∂q
i
+ e
i
∂
√
g
ii
∂q
j
− δ
j
i
e
n
√
g
ii
√
g
nn
∂
√
g
ii
∂q
n
(M4.43)
Observing the identity
∂q
i
∂q
j
=
∂
√
g
ii
e
i
∂q
j
= e
i
∂
√
g
ii
∂q
j
+
√
g
ii
∂e
i
∂q
j
(M4.44)
72 Coordinate transformations
after some slight rearrangements we obtain from (M4.43)
∂e
i
∂q
j
=
e
j
√
g
ii
∂
√
g
jj
∂q
i
− δ
j
i
e
n
√
g
nn
∂
√
g
ii
∂q
n
= e
j
∇
*
i
√
g
jj
− δ
j
i
e
n
∇
*
n
√
g
ii
(M4.45)
In the last step we have also introduced the physical measure numbers of the nabla
operator ∇
*
i
= (1/
√
g
ii
)(∂/∂q
i
).
Applications often require knowledge of the gradient of the unit vectors. With
the help of (M4.45) we most easily find the required result as
∇e
i
= q
m
∇
m
e
i
=
e
m
√
g
mm
∂e
i
∂q
m
=
e
m
√
g
mm
e
m
∇
*
i
√
g
mm
− δ
m
i
e
n
∇
*
n
√
g
ii
(M4.46)
M4.2.3 The generalized vertical coordinate
Often it is of advantage to meteorological analysis to replace the vertical coordinate
q
3
in the dynamic equations by the generalized coordinate ξ . According to the
specific problem which might be considered, this coordinate will be specified as
pressure, potential temperature, density, or some other suitable scalar function. A
necessary condition for the use of the generalized vertical coordinate is the existence
of a strictly monotonic relationship between q
3
and ξ . Usually the generalized
coordinate depends on the horizontal and the vertical coordinates as well as on
time. This becomes obvious on considering, for example, ξ = p. In general the
pressure varies in the horizontal direction, with height, and with time.
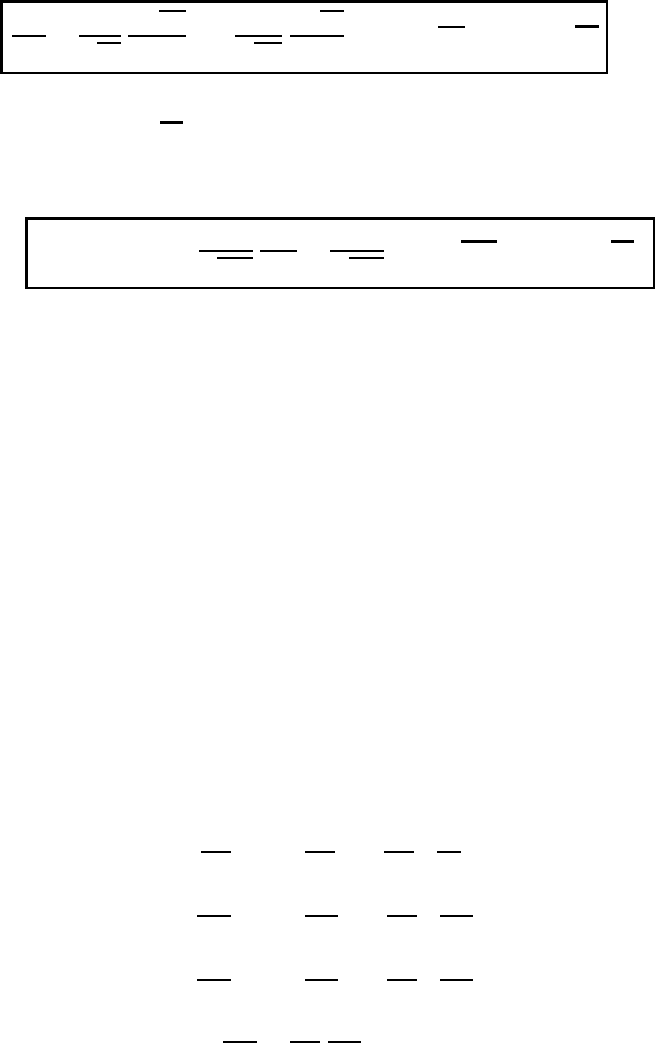
For a scalar quantity ψ the transformation relation for going from the (q
1
,q
2
,q
3
)
system to the (q
1
,q
2
,ξ) system is given by
ψ(q
1
,q
2
,q
3
,t) = ψ[q
1
,q
2
,ξ(q
1
,q
2
,q
3
,t),t](M4.47)
From this equation we directly obtain the transformation equations for the partial
derivatives
∂ψ
∂t
q
3
=
∂ψ
∂t
ξ
+
∂ψ
∂ξ
∂ξ
∂t
q
3
∂ψ
∂q
1
q
3
=
∂ψ
∂q
1
ξ
+
∂ψ
∂ξ
∂ξ
∂q
1
q
3
∂ψ
∂q
2
q
3
=
∂ψ
∂q
2
ξ
+
∂ψ
∂ξ
∂ξ
∂q
2
q
3
∂ψ
∂q
3
=
∂ψ
∂ξ
∂ξ
∂q
3
(M4.48)
M4.3 Problems 73
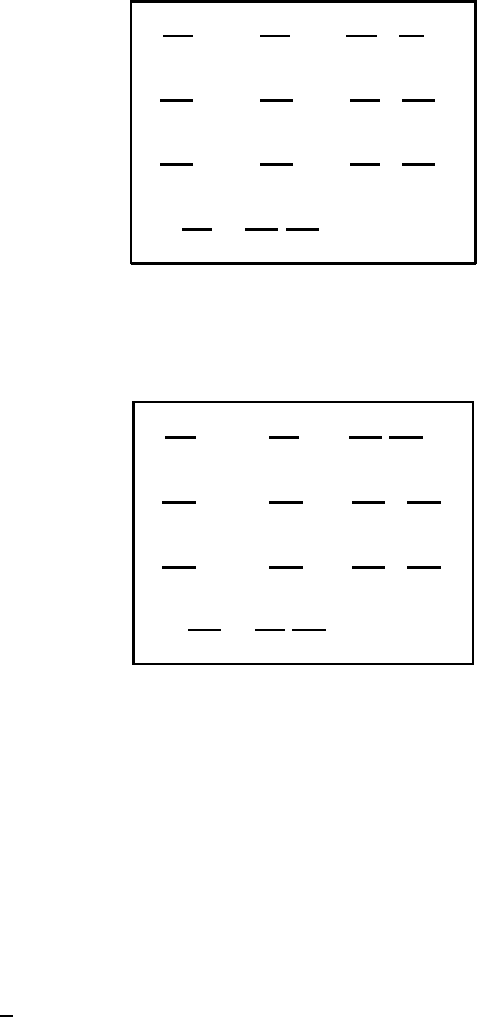
It is customary to state the transformation equations in the form
∂ψ
∂t
ξ
=
∂ψ
∂t
q
3
−
∂ψ
∂ξ
∂ξ
∂t
q
3
∂ψ
∂q
1
ξ
=
∂ψ
∂q
1
q
3
−
∂ψ
∂ξ
∂ξ
∂q
1
q
3
∂ψ
∂q
2
ξ
=
∂ψ
∂q
2
q
3
−
∂ψ
∂ξ
∂ξ
∂q
2
q
3
∂ψ
∂ξ
=
∂ψ
∂q
3
∂q
3
∂ξ
(M4.49)
We may also write the transformation relation for ψ as
ψ(q
1
,q
2
,ξ,t) = ψ[q
1
,q
2
,q
3
(q
1
,q
2
,ξ,t),t](M4.50)
Now the independent variables are q
1
,q
2
,ξ,andt. We obtain analogously
∂ψ
∂t
q
3
=
∂ψ
∂t
ξ
−
∂ψ
∂q
3
∂q
3
∂t
∂ψ
∂q
1
q
3
=
∂ψ
∂q
1
ξ
−
∂ψ
∂q
3
∂q
3
∂q
1
ξ
∂ψ
∂q
2
q
3
=
∂ψ
∂q
2
ξ
−
∂ψ
∂q
3
∂q
3
∂q
2
ξ
∂ψ
∂q
3
=
∂ψ
∂ξ
∂ξ
∂q
3
(M4.51)
The terms (∂q
3
/∂q
i
)
ξ
are the inclinations of the ξ -surface along the q
i
-directions.
M4.3 Problems
M4.1: Identify the a system in (M4.19) as the Cartesian system. The transforma-
tion relations between the Cartesian system and the rotating geographical system
of the earth (q
1
= λ – longitude, q
2
= ϕ – latitude, q
3
= r – radial distance) are
given by
x
1
= r cos ϕ cos(λ + t),x
2
= r cos ϕ sin(λ + t),x
3
= r sin ϕ
where is the rotational speed of the earth.
(a) Find the covariant metric fundamental quantities g
ij
of the geographical system
and
√
g
q
.
(b) Find the contravariant quantities g
ij
.

74 Coordinate transformations
M4.2: In the geographical system the absolute kinetic energy per unit mass is
given by
K
A
=
1
2
r
2
cos
2
ϕ (
˙
λ + )
2
+ r
2
˙ϕ
2
+ ˙r
2
(a) By utilizing this equation, verify the results of problem M4.1.
(b) Find the contravariant measure numbers of v
p
= v
.
M4.3: Find an expression for the functional determinant
√
g
ξ
in the (q
1
,q
2
,ξ)
system, where ξ is the generalized vertical coordinate.
M5
The method of covariant differentiation
The numerical investigation of specific meteorological problems requires the selec-
tion of a suitable coordinate system. In many cases the best choice is quite obvious.
Attempts to use the same coordinate system for entirely different geometries usu-
ally introduce additional mathematical complexities, which should be avoided. For
example, it is immediately apparent that the rectangular Cartesian system is not
well suited for the treatment of problems with spherical symmetry. The inspection
of the metric fundamental quantities g
ij
or g
ij
and their derivatives helps to decide
which coordinate system is best suited for the solution of a particular problem. The
study of the motion in irregular terrain may require a terrain-following coordinate
system. However, it is not clear from the beginning whether the motion is best
described in terms of covariant or contravariant measure numbers.
From the thermo-hydrodynamic system of equations, consisting of the dynamic
equations, the continuity equation, the heat equation, and the equation of state,
we will direct our attention mostly to the equation of motion using covariant and
contravariant measure numbers. We will also briefly derive the continuity equation
in general coordinates. In addition we will derive the equation of motion using
physical measure numbers of the velocity components if the curvilinear coordinate
lines are orthogonal.
In order to proceed efficiently, it is best to extend the tensor-analytical treatment
presented in the previous chapters by introducing the method of covariant differ-
entiation. What may seem strange and difficult to begin with is, in fact, a very
easy and efficient mathematical treatment that requires no additional theory. Our
discussion in this section will necessarily be quite formal.
M5.1 Spatial differentiation of vectors and dyadics
The situation is particularly simple if we consider the differentiation of a vector A
in a rectangular Cartesian coordinate system:
75
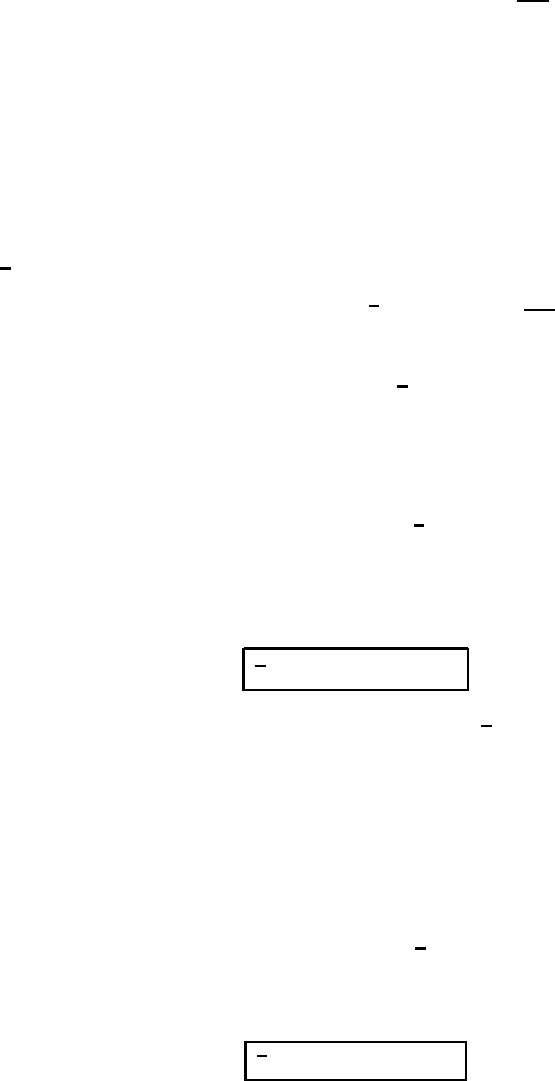
76 The method of covariant differentiation
∇
i
x
A =∇
i
x
(A
m
i
m
) = i
m
∇
i
x
A
m
, ∇
i
x
=
∂
∂x
i
(M5.1)
In this case the unit vector i
j
is independent of position on a particular coordinate
line or axis and thus may be extracted and placed in front of the differentiation
operator. Suppose that we express the same vector A in terms of contravariant
measure numbers and covariant basis vectors whose directions change with position
on a coordinate line. In this case we cannot simply extract the basis vector q
i
without
making a serious mistake. Nevertheless, guided by (M5.1), we still go ahead and
extract the basis vector and correct the error we have made by formally changing
the ordinary differential operator ∇
i
to the so-called covariant differential operator
∇
i
:
∇
i
A =∇
i
(A
m
q
m
) = q
m
∇
i
A
m
, ∇
i
=
∂
∂q
i
(M5.2)
This operation is of course formal and of no help unless we find a relation between
the two types of differential operators ∇
i
and ∇
i
. To establish the relation between
the two types of partial derivatives, we first carry out the ordinary differentiation,
using (M3.43), and then introduce the covariant derivative as
∇
i
(A
m
q
m
) = q
m
∇
i
A
m
+ A
m
∇
i
q
m
= q
m
∇
i
A
m
+ A
m
n
mi
q
n
= q
n
∇
i
A
n
+ A
m
n
mi
= q
n
∇
i
A
n
(M5.3)
Scalar multiplication by the vector q
k
gives the required relationship between the
two differential operators operating on the contravariant measure number A
k
:
∇
i
A
k
=∇
i
A
k
+ A
m
k
mi
(M5.4)
The result is that the covariant differential operator ∇
i
applied to A
k
is equal
to the ordinary differential operator applied to A
k
plus an additional term involving
the Christoffel symbol. The covariant derivative is of tensorial character whereas
the ordinary spatial derivatives are not tensorial expressions.
We may proceed in the same way if A is expressed in terms of covariant measure
numbers. Employing (M3.48) we find
∇
i
(A
m
q
m
) = q
m
∇
i
A
m
+ A
m
∇
i
q
m
= q
m
∇
i
A
m
− A
m
m
in
q
n
= q
n
∇
i
A
n
− A
m
m
in
= q
m
∇
i
A
m
(M5.5)
Scalar multiplication by the covariant basis vector q
k
leads to the desired relation
∇
i
A
k
=∇
i
A
k
− A
m
m
ik
(M5.6)
M5.1 Spatial differentiation of vectors and dyadics 77
Thus, the covariant operator is defined in different ways depending on the co-
ordinate definition of the vector A. If we choose contravariant measure numbers
to represent A, then we must apply (M5.4). Expressing A in terms of covariant
measure numbers requires the use of (M5.6).
So far we have considered only individual components or measure numbers of
A. Next we consider the local dyadic ∇A:
∇A = q
n
∇
n
(A
m
q
m
) = q
n
∇
n
(A
m
q
m
)(M5.7)
where we may select either covariant or contravariant measure numbers to represent
A. Application of the covariant differential operator leads to
∇A = q
n
q
m
∇
n
A
m
= q
n
q
m
∇
n
A
m
(M5.8)
where ∇
i
A
k
or ∇
i
A
k
are measure numbers of the local dyadic ∇A .
Finally we wish to remark that, for the covariant and contravariant (not yet
defined) derivatives, the sum and the product rules of ordinary differential calculus
are valid for tensors of any rank that are free from basis vectors
∇
i
(XY) = X ∇
i
Y + (∇
i
X)Y (M5.9)
where X, Y are tensors of arbitrary rank. Recall that the rank of a tensor is fixed by
the numbers of indices attached to the measure number.
Now we are going to discuss the covariant differentiation of the local triadic
which in our case is the gradient of a dyadic. What at first glance looks involved
and difficult is, in fact, a very easy operation. The formal covariant differentiation
is introduced in
∇
B = q
s
∇
s
(B
mn
q
m
q
n
) = q
s
q
m
q
n
∇
s
B
mn
(M5.10)
No further comment is needed. The ordinary differentiation is carried out in
q
s
∇
s
B = q
s
q
m
q
n
∇
s
B
mn
+ B
mn
q
s
∇
s
(q
m
)q
n
+ B
mn
q
s
q
m
∇
s
(q
n
)(M5.11)
making sure that the sequence of the basis vectors q
i
is not changed. The second
and the third term are rewritten yielding
q
s
∇
s
B = q
s
q
m
q
n
∇
s
B
mn
+ B
mn
q
s
−q
r
m
sr
q
n
+ B
mn
q
s
q
m
−q
r
n
sr
= q
s
q
m
q
n
∇
s
B
mn
− B
rn
r
sm
− B
mr
r
sn
(M5.12)
In the last expression of (M5.12) some indices have been rewritten with the goal
of extracting the common factor q
s
q
m
q
n
. This operation is quite valid since the
summation indices may be given any name without changing the meaning of the
78 The method of covariant differentiation
expression. According to (M5.10) the left-hand side of (M5.12) can be written as
q
s
q
m
q
n
∇
s
B
mn
so that the sequence of contravariant basis vectors appears on both
sides of this equation. Scalar multiplication from the right with q
j
, q
i
, q
k
then leads
to
∇
k
B
ij
=∇
k
B
ij
− B
nj
n
ki
− B
in
n
kj
(M5.13)
In the same way we find the covariant derivatives of the contravariant and mixed
measure numbers so that we have
∇
k
B
ij
=∇
k
B
ij
− B
nj
n
ki
− B
in
n
kj
, ∇
k
B
ij
=∇
k
B
ij
+ B
nj
i
kn
+ B
in
j
kn
∇
k
B
j
i
=∇
k
B
j
i
− B
j
n
n
ki
+ B
n
i
j
kn
, ∇
k
B
i
j
=∇
k
B
i
j
+ B
n
j
i
kn
− B
i
n
n
kj
(M5.14)
Let us now direct our attention to some operations involving the unit dyadic
E,
which is an invariant operator in space and time. Therefore we may write for the
gradient of
E
∇E = q
s
∇
s
E = 0, E = g
mn
q
m
q
n
= g
mn
q
m
q
n
= δ
n
m
q
m
q
n
= δ
m
n
q
m
q
n
(M5.15)
The various representations of
E are reviewed in this equation. We now wish to
find out how the metric fundamental quantities g
ij
and g
ij
are affected by covari-
ant differentiation. By simply introducing the covariant derivative analogously to
(M5.10),
q
s
∇
s
(g
mn
q
m
q
n
) = q
s
q
m
q
n
∇
s
g
mn
= 0, q
s
∇
s
(g
mn
q
m
q
n
) = q
s
q
m
q
n
∇
s
g
mn
= 0
q
s
∇
s
δ
n
m
q
m
q
n
= q
s
q
m
q
n
∇
s
δ
n
m
= 0, q
s
∇
s
δ
m
n
q
m
q
n
= q
s
q
m
q
n
∇
s
δ
m
n
= 0
(M5.16)
we find that the metric fundamental quantities g
ij
and g
ij
may be treated as constants
in covariant differentiation. The results, summarized in
∇
k
g
ij
= 0, ∇
k
g
ij
= 0, ∇
k
δ
j
i
= 0, ∇
k
δ
i
j
= 0
(M5.17)
will be very helpful and important in our studies. For instance, often it is necessary
to raise or lower the indices of measure numbers. Utilizing (M5.17) we find
∇
i
A
k
=∇
i
(g
kn
A
n
) = g
kn
∇
i
A
n
∇
i
A
k
=∇
i
(g
kn
A
n
) = g
kn
∇
i
A
n
(M5.18)
We are now ready to consider the gradient of the dyadic
B and the divergence
of
B in terms of the covariant derivatives. It is sometimes advantageous, though
not necessary, to involve the mixed measure numbers in order to apply the properties
M5.2 Time differentiation of vectors and dyadics 79
of the reciprocal basis vectors. The covariant derivative is introduced in
∇B = q
s
∇
s
B
m
n
q
m
q
n
= q
s
q
m
q
n
∇
s
B
m
n
(M5.19)
so that the divergence of the dyadic B is given by
∇·
B = q
s
·q
m
q
n
∇
s
B
m
n
= q
n
∇
m
B
m
n
(M5.20)
which is a rather simple expression.
We conclude this section by applying (M5.13) to a situation of considerable
importance. For the special case B
ij
= g
ij
, recalling that the metric fundamental
quantity is a constant in covariant differentiation, we obtain
∇
k
g
ij
=∇
k
g
ij
− g
nj
n
ki
− g
in
n
kj
= 0
=⇒
∇
k
g
ij
=
kij
+
kj i
(M5.21)
Finally we make use of the tensor properties of the covariant derivative to
introduce contravariant differentiation. By raising or by lowering the index we find
∇
k
= g
kn
∇
n
, ∇
k
= g
kn
∇
n
(M5.22)
It may be appropriate to give an example employing the contravariant derivative
for two representations of the vector A. With the help of (M5.22) we may replace
the contravariant derivative by the more common covariant derivative:
∇A = q
n
∇
n
(A
m
q
m
) = q
n
q
m
∇
n
A
m
= q
n
q
m
g
ns
∇
s
A
m
∇A = q
n
∇
n
(A
m
q
m
) = q
n
q
m
∇
n
A
m
= q
n
q
m
g
ns
∇
s
A
m
(M5.23)
M5.2 Time differentiation of vectors and dyadics
Let us consider the velocity of a point relative to the absolute system as defined
by (M4.33) with v
T
= 0. If the point is fixed somewhere in the atmosphere it
participates in the rotation of the earth so that v
P
= v
. If, for example, the
vertical coordinate of this point is located on a pressure surface, then this point
also participates in the vertical motion of the material surface as described by the
deformation velocity v
D
. If the measure numbers of the rotational and deformational
velocities are given by W
i
and W
i
D
then the change with time of the basis vector is
given by
∂q
i
∂t
q
k
=
∂
∂t
∂r
∂q
i
q
k
=∇
i
v
P
=∇
i
(W
n
q
n
+W
n
D
q
n
) = q
n
∇
i
(W
n
+W
n
D
)(M5.24)
where we have also applied the definition (M5.2) of the covariant differentiation.

80 The method of covariant differentiation
For simplicity the notation ( )
q
i denoting that the local time derivative is taken at
constant coordinates q
i
will henceforth be omitted, except where confusion may
occur. Next we consider some relationships involving the time differentiation of
the reciprocal basis vectors. From the definition
q
i
· q
j
= 0fori = j (M5.25)
we obtain
q
i
·
∂q
j
∂t
+ q
j
·
∂q
i
∂t
= 0(M5.26)
Dyadic multiplication with q
j
and then summing over j, i.e. replacing j by n,
results in the introduction of the unit dyadic
E
q
n
q
i
·
∂q
n
∂t
+
E·
∂q
i
∂t
= 0(M5.27)
Since
E is invariant in space and time, we may place E inside of the differential
operator and perform the scalar multiplication, yielding
∂q
i
∂t
=−q
n
q
i
·
∂q
n
∂t
=−q
n
q
i
·∇
n
v
P
=−q
n
q
i
·q
m
∇
n
W
m
=−q
n
∇
n
W
i
=−q
n
∇
n
(W
i
+ W
i
D
) =−q
n
∇
n
(W
i
+ W
i
D
)
with q
n
∇
n
= g
nm
q
m
∇
n
= q
m
∇
m
(M5.28)
We will now summarize some important results. From (M5.24) we find
q
j
·
∂q
i
∂t
=∇
i
(W
j
+ W
j
D
), q
j
·
∂q
i
∂t
=∇
i
(W
j
+ W
j
D
)
(M5.29)
and from (M5.28) we obtain
q
j
·
∂q
i
∂t
=−∇
j
(W
i
+ W
i
D
), q
j
·
∂q
i
∂t
=−∇
j
(W
i
+ W
i
D
)
(M5.30)
Let us now perform the local time differentiation of an arbitrary vector A.
Extracting the basis vector in analogy to the covariant nabla operator
∂A
∂t
=
∂
∂t
(A
n
q
n
) = q
n
∂A
n
∂t
(M5.31)
leads to the introduction of the covariant time operator ∂/∂t which must now be
related to the ordinary local time derivative. This is most easily done by carrying
out the differentiation in (M5.31) and replacing ∂q
n
/∂t by (M5.28), yielding
q
n
∂A
n
∂t
= q
n
∂A
n
∂t
+ A
n
−q
m
∇
m
(W
n
+ W
n
D
)
(M5.32)
