Tsoulfanidis N. Measurement and detection of radiation
Подождите немного. Документ загружается.


88
MEASUREMENT
AND
DETECTlON
OF
RADIATION
3.5
NUCLEAR BINDING ENERGY
The mass of a nucleus is given by Eq. 3.18 in terms of the masses of its
constituents. That same equation also defines the binding energy of the nucleus:
B(A, Z)
=
[ZM,
+
NM,
-
MN(A, z)]c2
(3.18)
The factor
c2,
which multiplies the mass to transform it into energy, will be
omitted from now on. It will always be implied that multiplication or division by
c2 is necessary to obtain energy from mass or vice versa. Thus, Eq. 3.18 is
rewritten as
B(A, Z)
=
ZM,
+
NM,
-
MN(A, Z) (3.19)
The meaning of B(A, Z) may be expressed in two equivalent ways:
1.
The binding energy B(A, Z) of a nucleus is equal to the mass transformed
into energy when the Z protons and the
N
=A
-
Z
neutrons got together
and formed the nucleus.
An
amount of energy equal to the binding energy
was released when the nucleus was formed.
2. The binding energy B(A, Z) is equal to the energy necessary to break the
nucleus apart into its constituents, Z free protons and N free neutrons.
As
mentioned in Section 3.4, atomic masses rather than nuclear masses are
measured in most cases. For this reason, Eq. 3.19 will be expressed in terms of
atomic masses by adding the appropriate masses of atomic electrons. If one adds
and subtracts Zrn in Eq. 3.19,
B(A, Z)
=
ZM,
+
Zrn
+
NM,,
-
MN(A,
Z)
-
Zrn
=
Z(M,
+
rn)
+
NM,
-
[MN(A, Z)
+
Zrn] (3.20)
Let
MH
=
mass of the hydrogen atom
Be
=
binding energy of the electron in the hydrogen atom
B,(A, Z)
=
binding energy of all the electrons of the atom whose nucleus
has mass MN(A, Z)
M(A, Z)
=
mass of the atom with nuclear mass equal to MN( A, Z)
Then
MH=Mp+rn-Be
Combining Eqs. 3.20, 3.21, and 3.22, one obtains
B(A, Z)
ZMH
+
NM,
-
M(A, Z)
-
B,(A,
Z)
+
ZB,
(3.23)

REVIEW
OF ATOMIC
AND
NUCLEAR PHYSICS
89
Unless extremely accurate calculations are involved, the last two terms of
Eq.
3.23
are neglected. The error introduced by doing so is insignificant because
ZB,
and
B,(A, Z)
are less than a few keV and they tend to cancel each other,
while
B(A, Z)
is of the order of MeV. Equation
3.23
is, therefore, usually
written as
B(A, Z)
=
ZMH
+
NM,
-
M(A, Z)
(3.24)
Example
3.4
What is the total binding energy of l~e?
Answer
Using
Eq.
3.24
and data from App. b,
B(4,2)
=
2MH
+
2M,
-
M(4,2)
=
[2(1.00782522)
+
2(1.00866544)
-
4.00260361]
u
=
0.03037771
u
=
(0.0303771 u)93l.478
MeV/u
=
28.296
MeV
=
4.53
X
10-l2
J
Example
3.5
What is the binding energy of the nucleus
';;u?
Answer
B(238,92)
=
[%(I .OO782522)
+
l46(l .OO866544)
-
238.050761
u
=
1 .!I3431448
u
=
(1.93431448 u)931.478
MeV/u
=
1801.771
MeV
=
2.886
x
J
The energy necessary to remove one particle from the nucleus is the
separation
or
binding energy
of that particle for that particular nuclide.
A
"particle" may be a neutron, a proton, an alpha particle, a deuteron, etc. The
separation or binding energy of
a
nuclear particle is analogous to the ionization
potential of an electron. If a particle enters the nucleus, an amount of energy
equal to its separation energy is released.
The separation or binding energy of a neutron
(B,)
is defined by the
equation
B,
=
M[(A
-
I), Z]
+
M,
-
M(A, Z) (3.25)
Using Eq.
3.24,
Eq.
3.25
is written
B,
=
B(A,
Z)
-
B[(A
-
I),
Z] (3.26)
which shows that the binding energy of the last neutron is equal to the
difference between the binding energies of the two nuclei involved. Typical
values of
B,
are a few MeV (less than
10
MeV).
The separation or binding energy of a proton is
Bp=M(A- 1,Z- l)+MH-M(A,Z) (3.27)
or, using Eq.
3.24,
Bp=B(A,Z)
-
B(A
-
1,Z- 1)
(3.28)
90
MEASUREMENT
AND
DETECTION OF RADIATION
The separation energy for an alpha particle is
B,=M(A-4,Z-2)+MH,-M(A,Z)
(3.29)
or, using
Eq.
3.24,
B,
=
B(A,Z)
-
B(A
-
4,
Z
-
2)
-
B(4,2)
(3.30)
Example
3.6
What is the separation energy of the last neutron of the i~e
nucleus?
Answer
Using data from
App.
B
and
Eq.
3.25, one obtains
Bn
=
M(3,2)
+
Mn
-
M(4,2)
=
[(3.016030
+
1.008665
-
4.002604) ~1931.478 MeV/u
=
0.022091(931.478 MeV)
=
20.58 MeV
=
3.3
X
lo-''
J
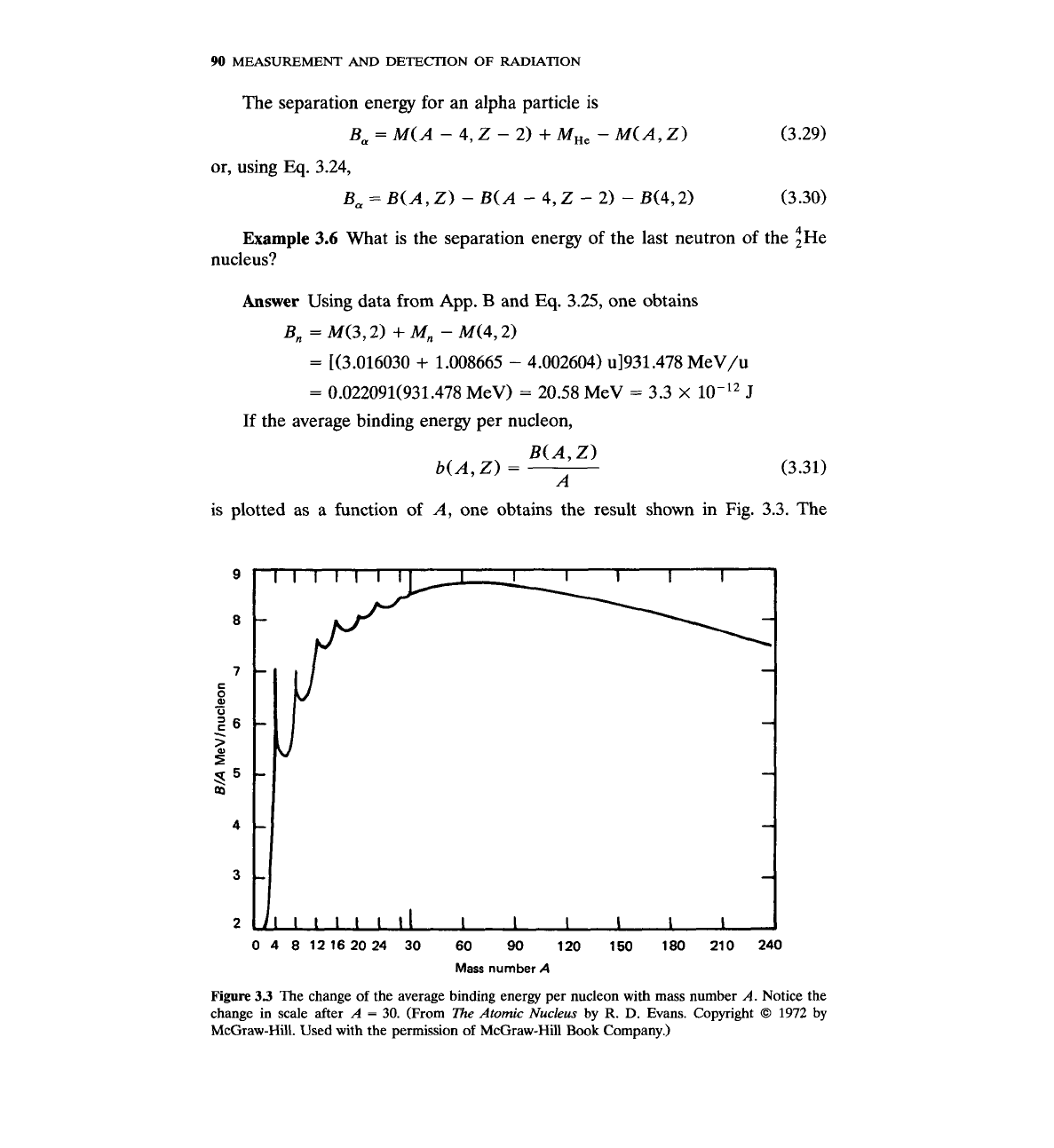
If the average binding energy per nucleon,
is plotted as
a
function of A, one obtains the result shown in
Fig.
3.3. The
04 812162024 30 60 90 120 150 180 210 240
Mass
number
A
Figure
33
The change of the average binding energy per nucleon with mass number
A.
Notice the
change in scale after
A
=
30.
(From
The
Atomic
Nucleus
by
R.
D.
Evans. Copyright
O
1972
by
McGraw-Hill. Used with the permission of McGraw-Hill
Book
Company.)
REVIEW
OF
ATOMIC
AND
NUCLEAR
PHYSICS
91
average binding energy changes relatively little, especially for A
>
30. Notice
that Fig.
3.3
has a different scale for
A
<
30.
Figure 3.3 is very important because it reveals the processes by which energy
may be released in nuclear reactions. If one starts with a very heavy nucleus
(A
=
240) and breaks it into two medium-size nuclei (fission), energy will be
released because the average binding energy per nucleon is larger for nuclides
in the middle of the periodic table than it is for heavy nuclides. On the other
hand, if one takes two very small nuclei (A
=
2,3) and fuses them into a larger
one, energy is again released due to similar increase in the average binding
energy per nucleon.
3.6
NUCLEAR ENERGY LEVELS
Neutrons and protons are held together in the nucleus by nuclear forces.
Although the exact nature of nuclear forces is not known, scientists have
successfully predicted many characteristics of nuclear behavior by assuming a
certain form for the force and constructing nuclear models based on that form.
The success of these models is measured by how well their predicted results
agree with the experiment. Many nuclear models have been proposed, each of
them explaining certain features of the nucleus; but as of today, no model exists
that explains all the facts about all the known nuclides.
All the nuclear models assume that the nucleus, like the atom, can exist
only in certain discrete energy states. Depending on the model, the energy states
may be assigned to the nucleons-neutrons and protons-or the nucleus as a
whole. The present discussion of nuclear energy levels will be based on the
second approach.
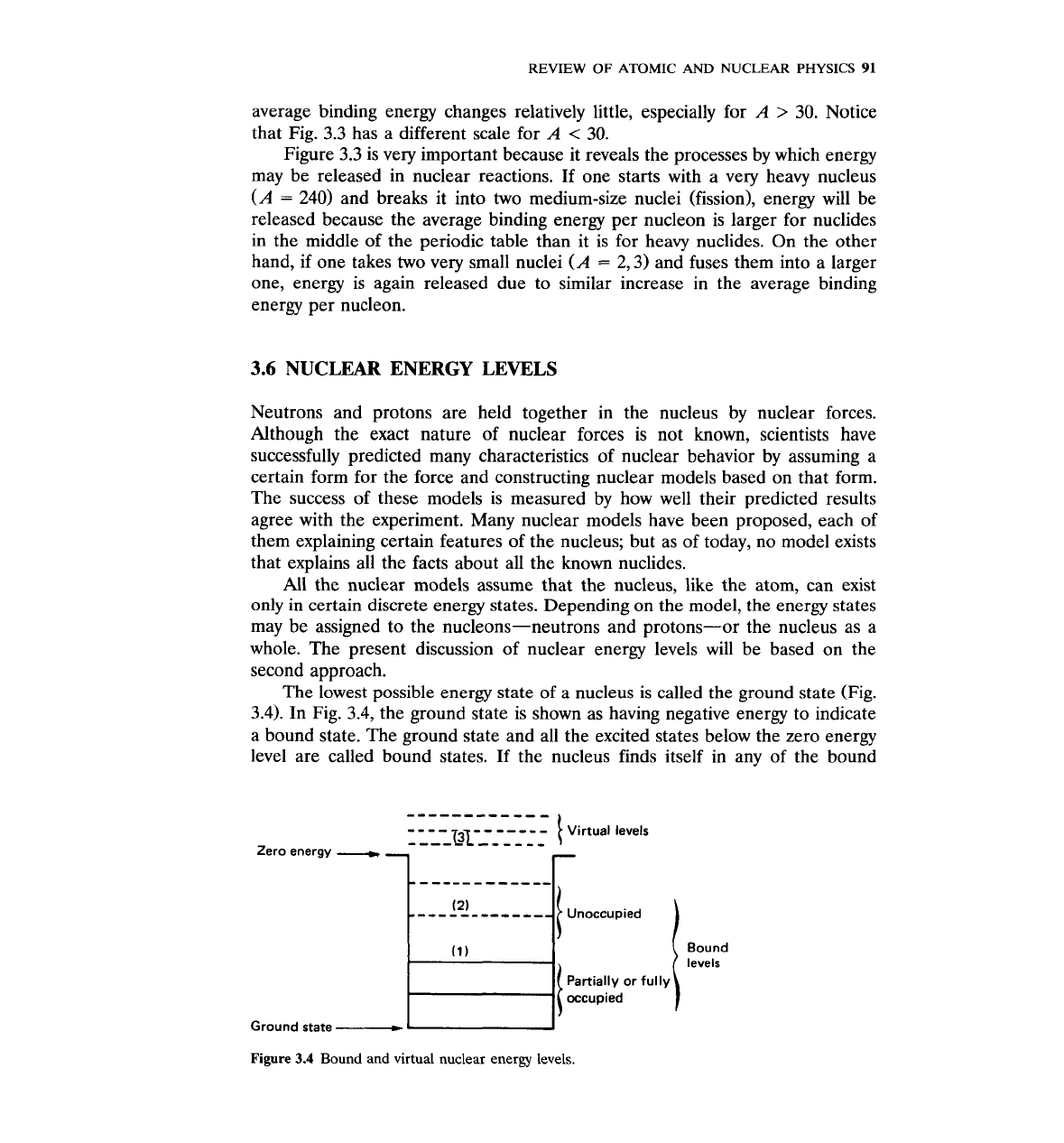
The
lowest possible energy state of a nucleus is called the ground state (Fig.
3.4).
In Fig. 3.4, the ground state is shown as having negative energy to indicate
a bound state. The ground state and all the excited states below the zero energy
level are called bound states.
If
the nucleus finds itself in any of the bound
levels
Partially or fully
occupied
Ground state
-
-
Figure 3.4
Bound and virtual nuclear energy levels.

92
MEASUREMENT
AND
DETECTION
OF
RADIATION
states, it deexcites after a time of the order of
lo-''
to
10
-lo
s by dropping to a
lower state. Deexcitation is accompanied by the emission of a photon with
energy equal to the difference between the energies of the initial and final
states. Energy states located above the zero energy level are called virtual
energy levels. If the nucleus obtains enough energy to be raised to a virtual
level, it may deexcite either by falling to one of the bound levels or by emitting a
nucleon.
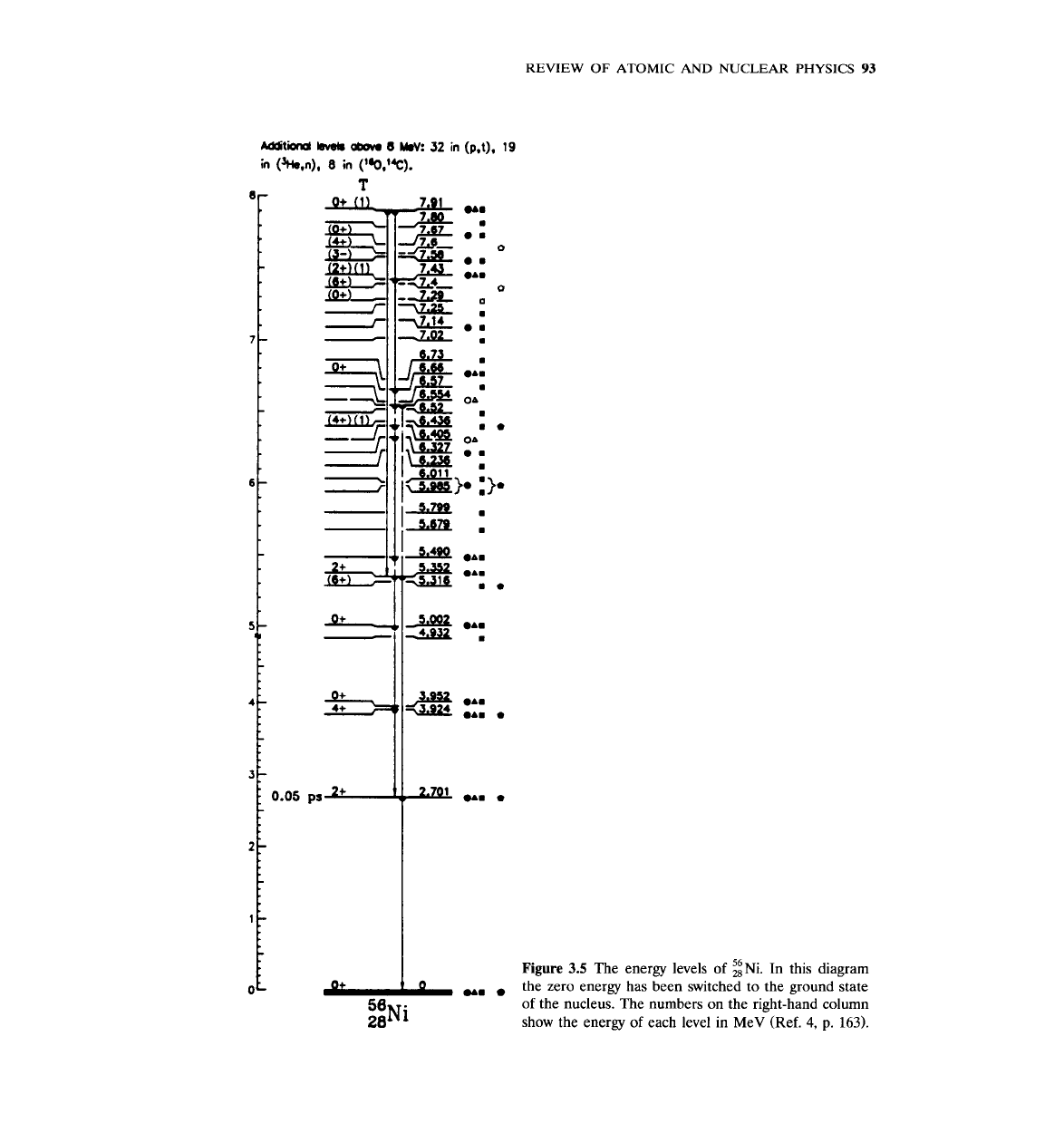
Studies of the energy levels of all the known nuclides reveal the following:
1.
The distance between nuclear energy levels is of the order of keV to MeV.
By contrast, the distance between atomic levels is of the order of eV.
2.
The distance between levels decreases as the excitation energy increases (Fig.
3.5). For very high excitation energies, the density of levels becomes so high
that it is difficult to distinguish individual energy levels.
3.
As
the mass number
A
increases, the number of levels increases; i.e., heavier
nuclei have more energy levels than lighter nuclei (in general-there may be
exceptions).
4.
As
A
increases, the energy of the first excited state decreases (again, in
general-exceptions exist). For example,
9~e: first excited state is at
1.68
MeV
56~e: first excited state is at
0.847
MeV
238~:
first excited state is at
0.044
MeV
3.7
ENERGETICS
OF
NUCLEAR DECAYS
This section discusses the energetics of
a,
P,
and
y
decay, demonstrating how
the kinetic energies of the products of the decay can be calculated from the
masses of the particles involved. In all cases, it will be assumed that the original
unstable nucleus is at
rest-i.e., it has zero kinetic energy and linear momen-
tum. This assumption is very realistic because the actual kinetic energies of
nuclei due to thermal motion are of the order of
kT
(of the order of eV), where
k
is the Boltzmann constant and
T
the temperature (Kelvin), while the energy
released in most decays is of the order of MeV.
In writing the equation representing the decay, the following notation will
be used:
M
=
atomic mass (or
MC'
=
rest mass energy)
E,
=
energy of a photon
T,
=
kinetic energy of a particle type
i
4.
=
linear momentum of a particle type
i
REVIEW OF ATOMIC
AND
NUCLEAR PHYSICS
93
I
-
*C
I
-
r
=
qr
-.
0
--
urn
the zero energy has been switched to the ground state
of the nucleus. The numbers on the right-hand column
show the energy of each level in MeV (Ref. 4,
p.
163).
Figure
3.5
The energy levels
of
:i~i.
In this diagram

94
MEASUREMENT
AND
DETECTION OF RADIATION
3.7.1
Gamma Decay
In
y
decay, a nucleus goes from an excited state to a state of lower energy and
the energy difference between the two states is released in the form of a photon.
Gamma decay is represented by
where
A,
X*
indicates the excited nucleus.
Applying conservation of energy and momentum for the states before and
after the decay, we havet
Conservation of energy:
Conservation of momentum:
0
=
PM
+
Py
Using these two equations and the nonrelativistic form of the kinetic energy of
the nucleus,
Use has been made of the relationship
Ey
=
Pyc
(the photon rest mass is zero).
Equation 3.34 gives the kinetic energy of the nucleus after the emission of a
photon of energy
Ey.
This energy is called the
recoil energy.
The recoil energy is small. Consider a typical photon of
1
MeV emitted by a
nucleus with
A
=
50. Then, from
Eq.
3.34,
Most of the time, this energy is neglected and the gamma energy is written as
However, there are cases where the recoil energy may be important,
e.g., in
radiation damage studies.
Sometimes the excitation energy of the nucleus is given to an atomic
electron instead of being released in the form of a photon. This type of nuclear
transition is called internal conversion
(IC), and the ejected atomic electron is
called an internal conversion electron.
'~~uations in this chapter are written in terms of atomic, not nuclear, masses. This notation
introduces a slight error because the binding energy of the atomic electrons is not taken into
account (see Sec.
3.5).
REVIEW OF ATOMIC
AND
NUCLEAR
PHYSICS
95
Let
T,
be the kinetic energy of an electron ejected from shell
i
and
Bi
be
the binding energy of an electron in shell
i.
Equation 3.32 now takes the form
M*(A, Z)
=
M(A, Z)
+
Ti
+
Bi
+
TM
(3.35)
Even though the electron has some nonzero rest mass energy, it is so much
lighter than the nucleus that
TM
c
T,.
Consequently,
TM
is neglected and Eq.
3.35 is written as
T,
=
M*(A,Z)
-
M(A,Z)
-
Bi
(3.36)
If
Q
=
M*(A, Z)
-
M(A, Z)
=
energy released during the transition, then
T,=Q-Bi
When internal conversion occurs, there is a probability than an electron
from the
K
shell,
L
shell, or another shell, may be emitted. The corresponding
equations for the electron kinetic energies are
TK
=
Q
-
B,
TL
=
Q
-
B,
(3.37)
TM=Q-BM
etc.
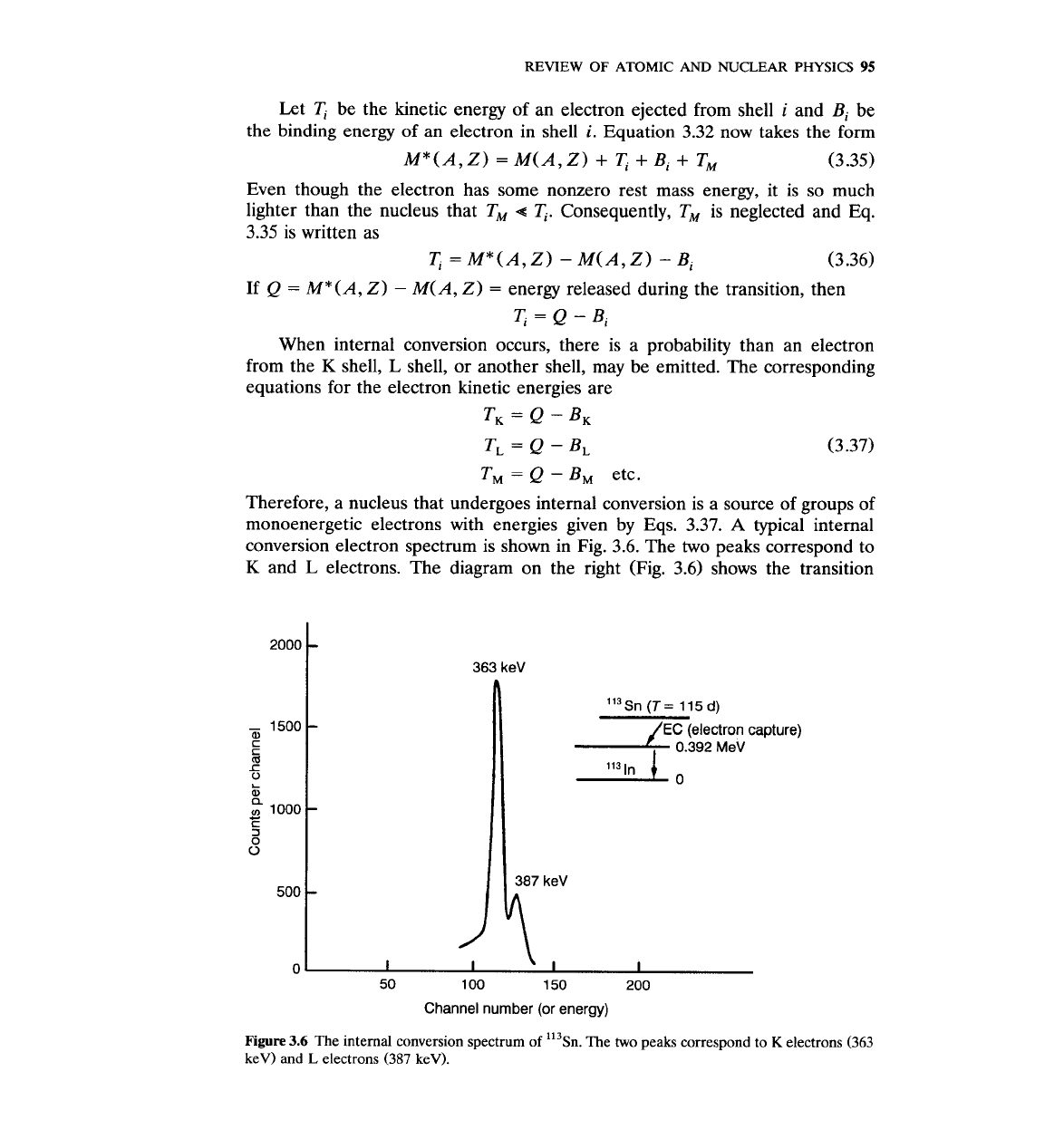
Therefore, a nucleus that undergoes internal conversion is a source of groups of
monoenergetic electrons with energies given by Eqs. 3.37.
A
typical internal
conversion electron spectrum is shown in Fig. 3.6. The
two
peaks correspond to
K
and
L
electrons. The diagram on the right (Fig. 3.6) shows the transition
Il3~n(T=
115d)
-
1500
-
a,
EC
(electron capture)
C
5
0.392
MeV
1
0
L
n
I
,
(U
:
1000
-
-
C
3
0
0
500
-
0
50 100 150 200
Channel number (or energy)
Figure
3.6
The internal conversion spectrum of 'l3~n. The two peaks correspond to
K
electrons (363
keV) and
L
electrons
(387
keV).

96
MEASUREMENT
AND DETECTION OF RADIATION
energy to be 392 keV. The K-shell energy is then
B,
=
392
-
363
=
29 keV
and the L-shell binding energy is
BL
=
392
-
387
=
5
keV. Let:
A,
=
probability that internal conversion will occur
A,
=
probability that a photon will be emitted
A,
=
probability that an electron from shell i will be emitted
A
=
total probability for y decay
and
A
=
A,
+
A,
For most nuclei,
A,
=
0, but there is no y-decaying nucleus for which
A,
=
0.
This means radioisotopes that internally convert, emit gammas as well as
electrons. After an atomic electron is emitted, the empty state that was created
will quickly be filled by another electron that "falls in" from the outer shells. As
a result of such a transition, an X-ray is emitted. Therefore, internally convert-
ing nuclei emit y-rays, electrons, and X-rays.
Radioisotopes that undergo internal conversion are the only sources of
monoenergetic electrons, except for accelerators. They are very useful as
instru-
113
137
ment calibration sources. Three isotopes frequently used are Sn, Cs, and
207
Bi.
3.7.2
Alpha
Decay
Alpha decay is represented by the equation
Applying conservation of energy and momentum,
M(A,Z)=M(A-4,2-2)+M(4,2)
+
T,+T, (3.40)
and
0
=
Pa
+
P,
The energy that becomes available as a result of the emission of the alpha
particle is called the decay energy Q,, defined by
Q,
=
(mass of parent)
-
(mass of decay products)
(3.42)
Q,=M(A,Z)-M(A-4,Z-2) -M(4,2)
'~ables of isotopes usually give, not the values of the different A's, but the so-called
IC
coefficients, which are the ratios
A,/A,, AJA,,
etc. (see Ref. 4).

REVIEW
OF
ATOMIC
AND
NUCLEAR
PHYSICS
97
Obviously, for
a
decay to occur,
Q,
should be greater than zero. Therefore,
a
decay is possible only when
If the daughter nucleus is left in its ground state, after the emission of the alpha,
the kinetic energy of the two products is (from
Eq.
3.401,
In many cases, the daughter nucleus is left in an excited state of energy
Ei,
where
i
indicates the energy level. Then,
Eq.
3.44
becomes
which shows that the available energy
(Q,)
is decreased by the amount
Ei.
The kinetic energies
T,
and
TM
can be calculated from Eqs.
3.41
and
3.44.
The result is
M(A
-4,Z-2) A-4
T,
=
(Q,
-
Ei)
s
7
(Q,
-
Ei)
(3.46)
M(A
-
4,
Z
-
2)
+
M(4,2)
Example
3.7
What are the kinetic energies of the alphas emitted by
238~?
Answer
The decay scheme of
'39;~
is shown in Fig.
3.7.
After the alpha is
emitted, the daughter nucleus,
'ii~h,
may be left in one of the two excited states
at
0.16
MeV and
0.048
MeV or go to the ground state.
The decay energy
Q,
is
(Eq.
3.42)
Q,
=
M(238,92)
-
M(234,90)
-
M(4,2)
=
238.050786
-
234.043594
-
4.002603
=
0.004589
u
=
0.004589
X
931
A81
MeV
=
4.27
MeV
Depending on the final state of ';;Th, the energy of the alpha particle is
T,
=
EQ,
=
4.20
MeV
T,
=
z(Q,
-
0.048)
=
4.15
MeV
T,
=
%(Q,
-
0.16)
=
4.04
MeV
3.7.3
Beta
Decay
In
p
decay, a nucleus emits an electron or a positron and is transformed into a
new element. In addition to the electron or the positron, a neutral particle with
rest mass zero is also emitted. There are two types of
P
decay,
P
and
P+.
