Tsebelis G. Veto Players: How Political Institutions Work

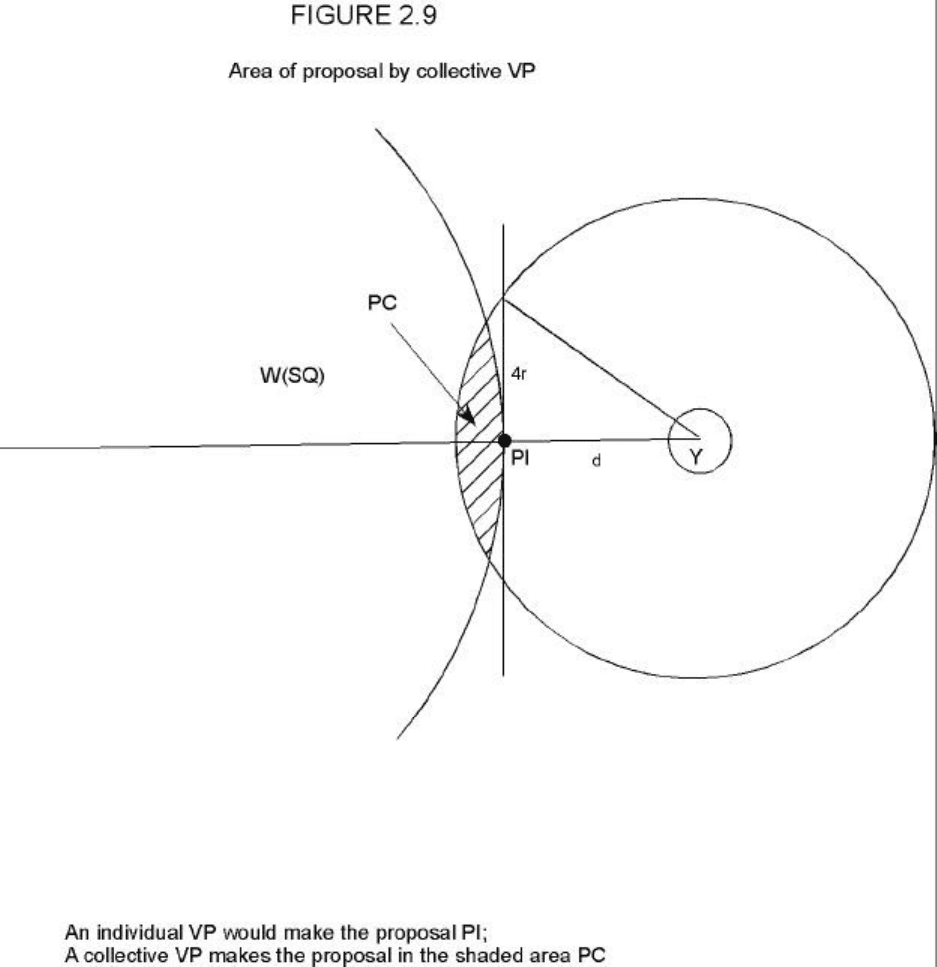
collective agenda setter makes a proposal inside the winset of existing veto players, this proposal

will closely approximate the proposal that an individual agenda setter

would have made.

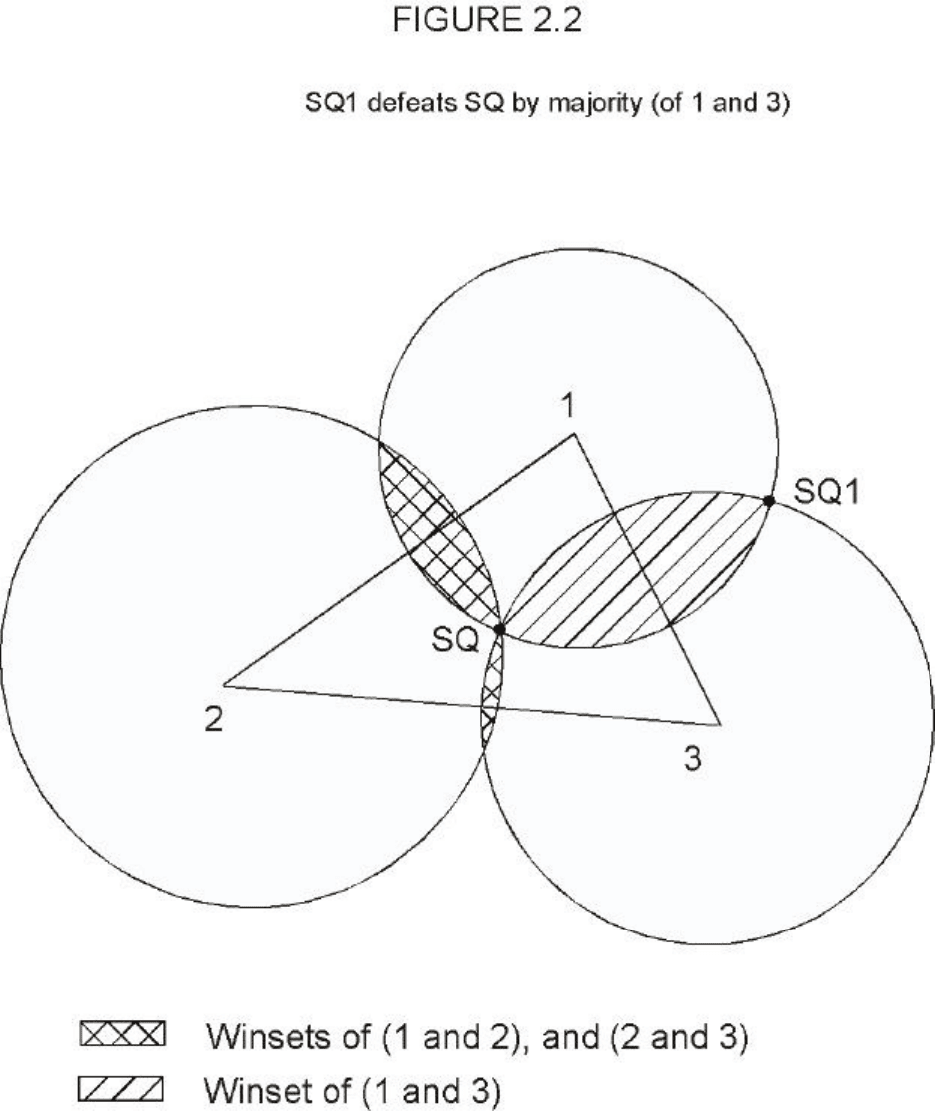
There are two points in this chapter that are counterintuitive, and that I need to single out.

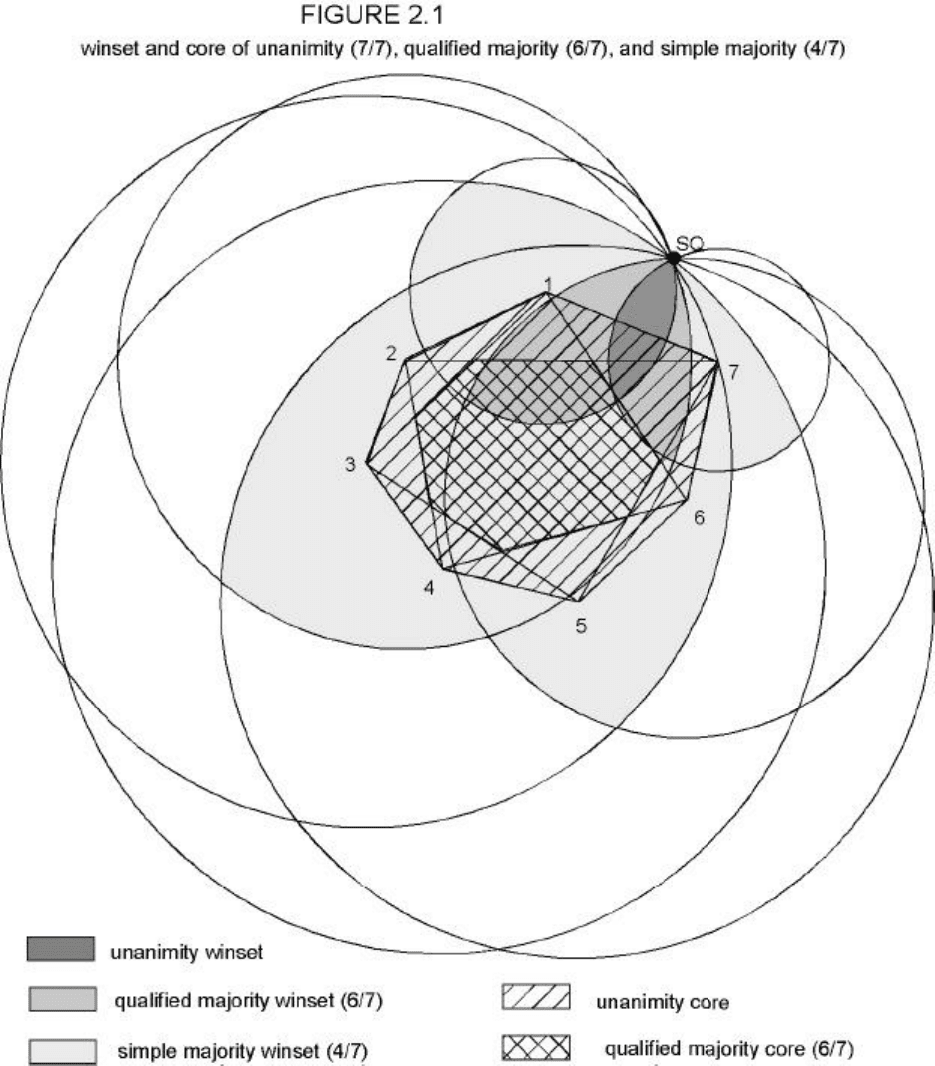
The first relates to the cohesion of collective veto players and policy stability: the more cohesive

a collective veto player deciding by majority rule, the higher policy stability, while the more

cohesive a collective veto player deciding by qualified majority the lower policy stability. The

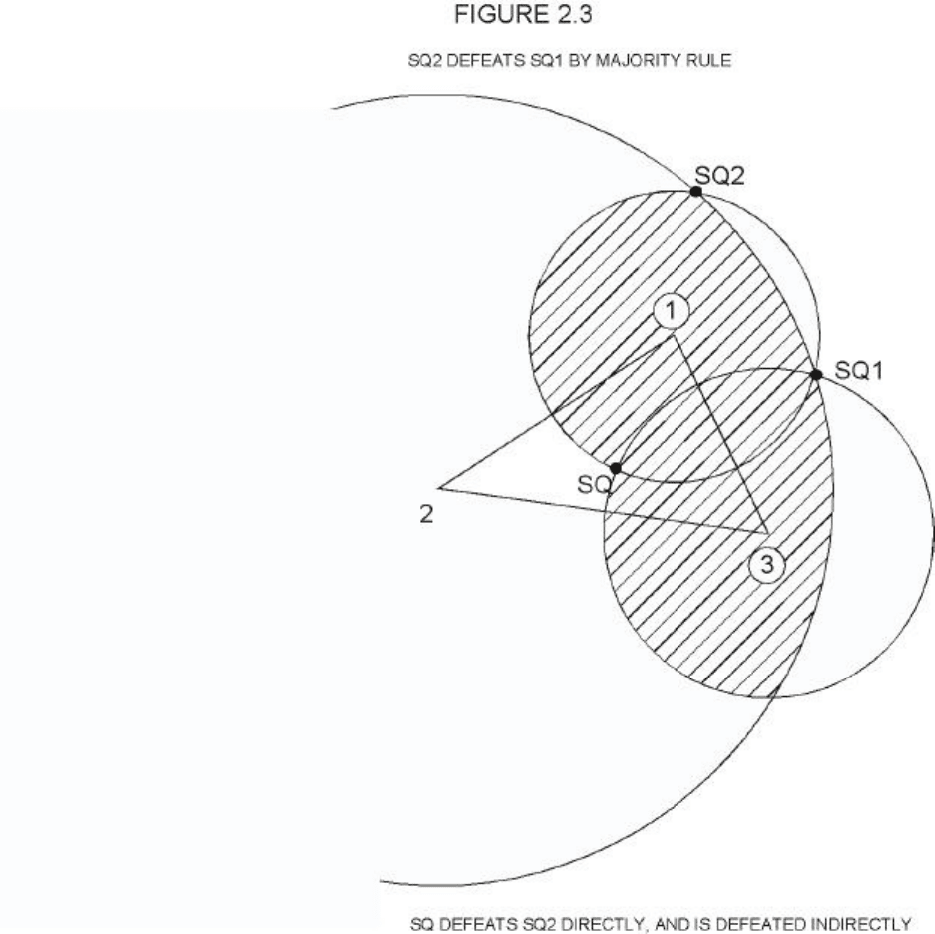
second refers to the restrictions under which collective veto players will make similar proposals

with individual ones: they should be making proposals that are are not defeated both directly and

indirectly by other available alternatives.

In conclusion, collective veto players approximate the behavior of individual ones. We

can approximate their preferences by a wincircle (which includes the actual winset) whether they

decide by simple or qualified majorities. We have also good reasons to assume that they will

make approximately the same proposals with individual veto players located in the center of their

yolk.

The goal of this chapter was to move from individual to collective veto players. The

introduction and conclusions provided the intuition behind my approach. The main part of the

chapter provided the algorithm of identification of wincircles and q-wincircles, that is of

proposals that may be accepted by collective veto players whether by simple or qualified

majorities, as well as the algorithm to identify the proposals that collective veto players will

make (assuming that covered points will not be chosen). I now move to the analysis of existing

political systems on the basis of the theory presented.

Located in the center of the yolk Y of the collective one.

W(SQ)

d+2r

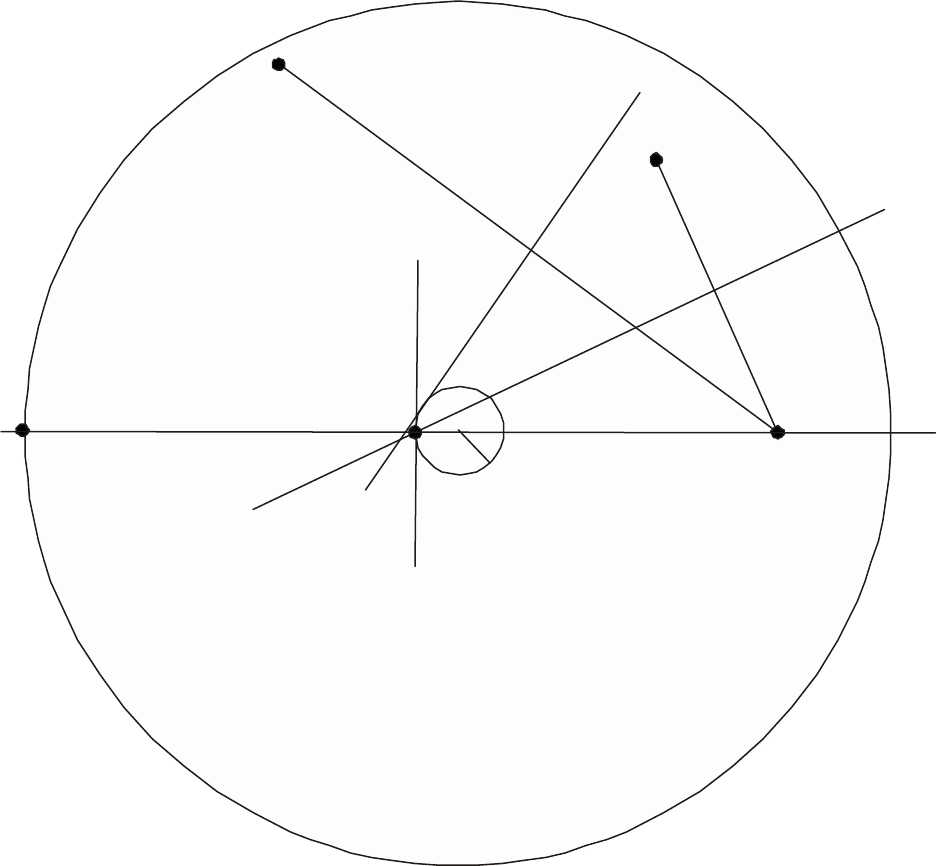
Circle(Y,d+2r) contains the Winset of the status quo ofcollective VP(ABCDE)

SQ2

SQ1

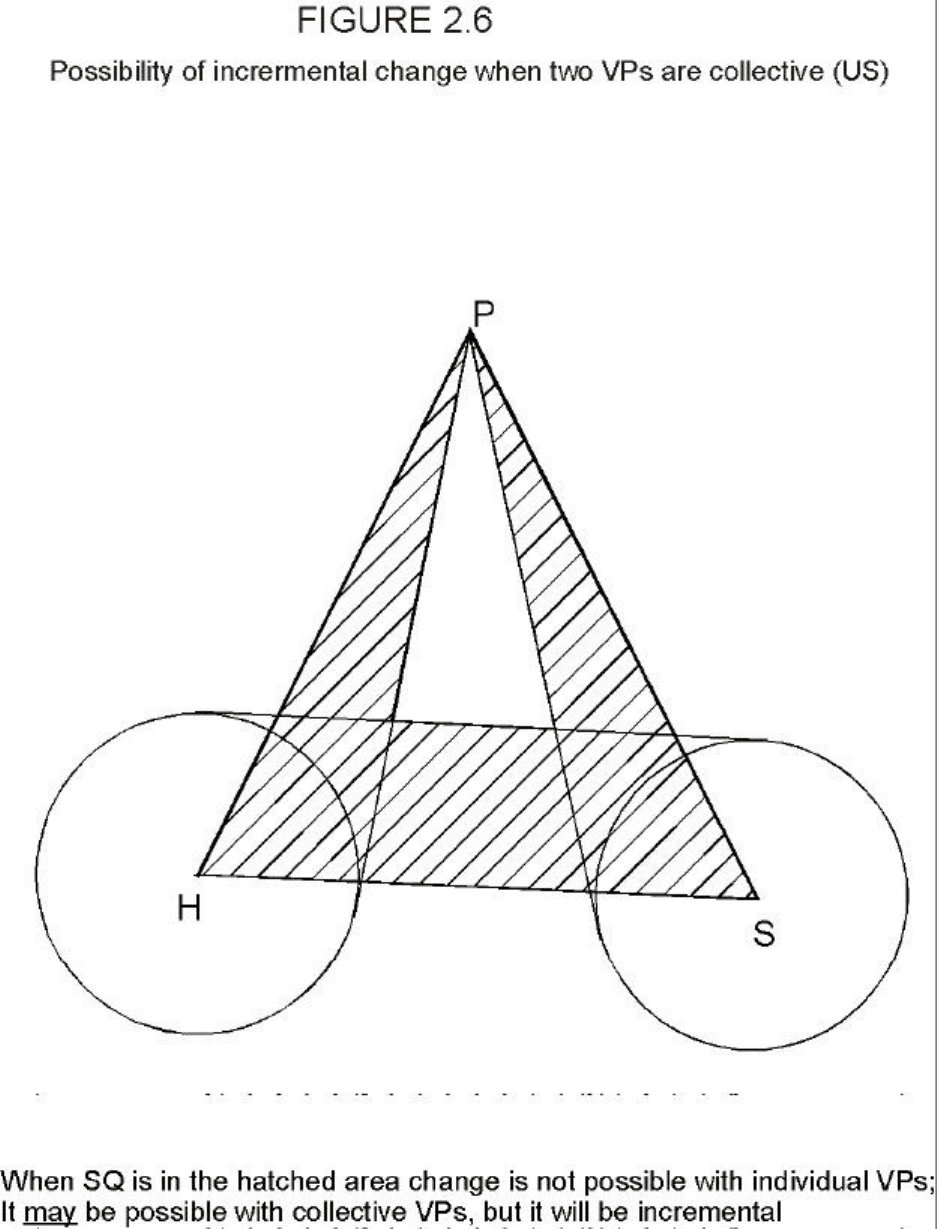
FIGURE 2.5

W(SQ) IS CONTAINED INSIDE THE WINCIRCLE (Y, d+2r)

SQ3

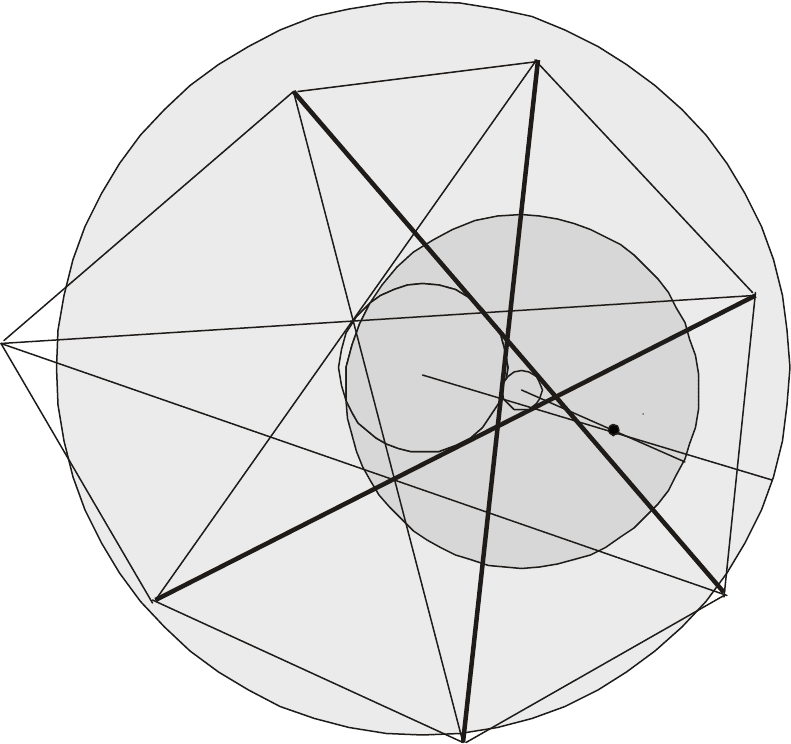
FIGURE 2.7

Comparison of simple majority wincircle (4/7)

with qualified majority wincircle (5/7)

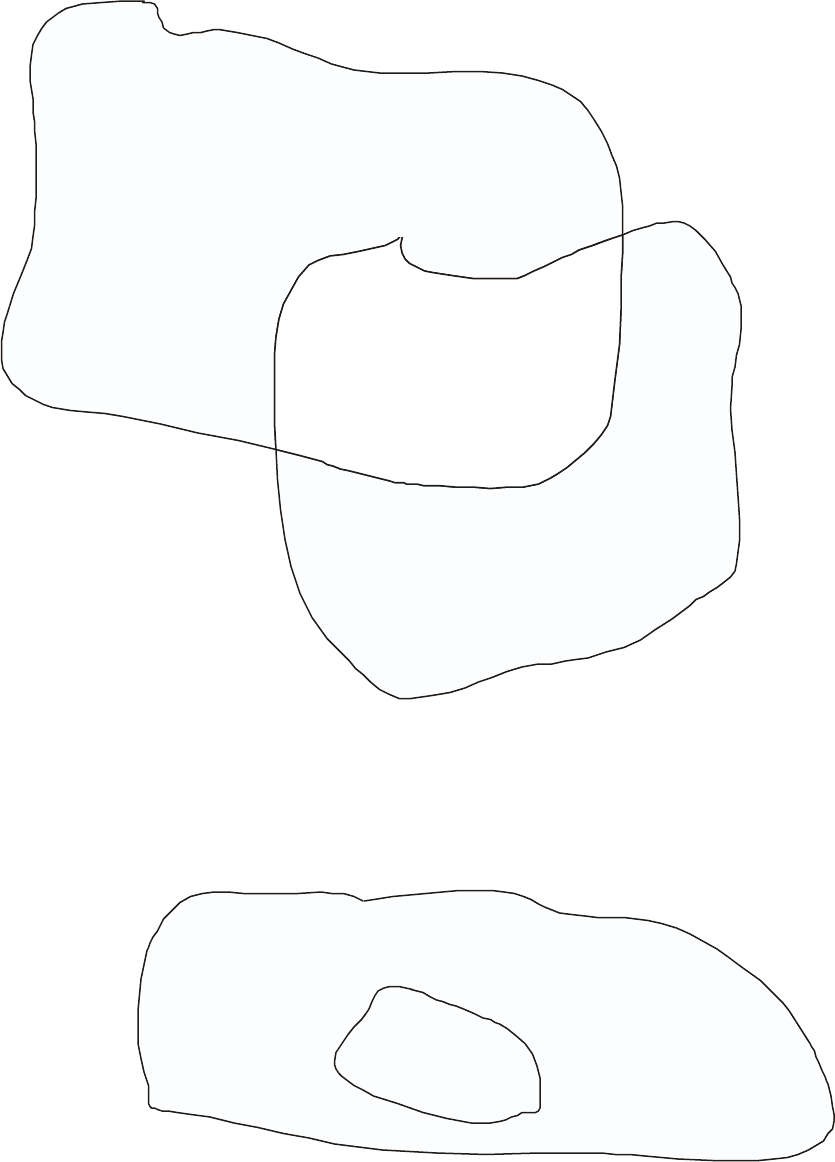
FIGURE 2.8A

Y COVERS X

W(X)

W(Y)

FIGURE 2.8B

W(X)

W(Y)

Y DOES NOT COVER X

100