Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

MECHANICAL SYSTEMS, CLASSICAL MODELS

522

roots are negative and

t increases, then the representative point moves close to the

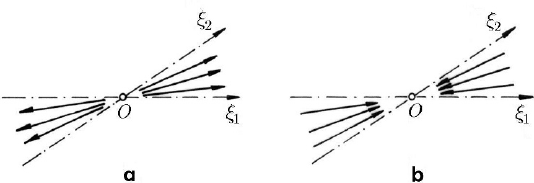
singular point, resulting a stable star (Fig. 23.9b).

= 0λ , then the general solution is

112212

, , , constCCCCξξ== =,

(23.1.24)

and any point of the phase space is a point of equilibrium.

Fig. 23.9 Representation of the solution for double eigenvalues

λλλ==:

(a) 0

λ > , instable star; (b) 0λ < , stable star

(b) The equations cannot be uncoupled and the solutions are

1122

e, e

CCt

λλ

ξξ

(23.1.24')

Eliminating the time, we get

21121

lnKKξξξ

(23.1.24'')

where

,KK are other constants of integration, hence a family of logarithmic curves,

the singular point being a node; this node is asymptotically stable if

< 0λ and

instable if

> 0λ .

= 0λ , then the general solution is

=+ =

11222

,CCt Cξξ,

(23.1.24''')

the phase trajectories being straight lines

constλ

, parallel to the

Oξ

-avis, the

velocities being constant, in direct proportion to

ξ (Fig. 23.8); there exist points of

equilibrium

(,0)C , different from the origin, on the straight line =

0ξ .

(v) Complex roots. If complex roots are

± iαβ

, then the solutions will be

11 22

ecos, esin

CtCt

αα

ξβξβ,

(23.1.25)

where we used Euler’s formula. The trajectories of the representative point

P form a

family of spirals, the singular point being called focus; if

> 0α

, then the point

moves away from the origin, which is an instable focus (Fig. 23.10a), while if

< 0α ,

Stability and Vibrations

523

then the point

P tends to the singular point for →∞t , having – in this case – an

asymptotically stable focus (Fig. 23.10b).

Fig. 23.10 Representation of the solution for complex eigenvalues of real part α:

(a)

0α >

, instable focus; (b)

0α <

, stable focus

= 0α , then the roots are purely imaginary and the solutions are

11 22

cos , sinCtCtξβξβ

(23.1.25')

Eliminating the time

t between these relations, we get the trajectories of the

representative point in the form

ξξ

(23.1.25'')

hence a family of ellipses. The singular point is a centre, being stable (Fig. 23.11).

Fig. 23.11 Representation of the solution for purely imaginary eigenvalues

around a stable centre

The notions of point of centre type, point of focus type, point of saddle type etc. have

been introduced by H. Poincaré in the study of the behaviour of the integral curves in

the neighbourhood of singular points (Poincaré, H., 1952).

Let us apply the above theory to the case of the mathematical pendulum of equation

(see Sect. 7.1.3.1)

MECHANICAL SYSTEMS, CLASSICAL MODELS

524

+==

sin 0,

θω θ ω



(23.1.26)

l being the length of the pendulum, and g the gravity acceleration; the position of the

particle in motion is given by the angle

= ()tθθ . With the notations

121

, d /dxxxtθ== , we obtain the system of differential equations of first order

==−

,sin

;

(23.1.27)

retaining only the linear terms, we can write

==−

(23.1.27')

The characteristic equation

−

=+=

−−

λω

ωλ

has only purely imaginary roots

=±

1,2

iλω, the singular point = 0θ being a centre of

stable equilibrium.

To study the position of equilibrium

=θπ, we make the change of variable

=+θπϕ, being led to the differential equations

−=

sin 0ϕω ϕ ;

(23.1.26')

the equivalent system of differential equations is

,sin

(23.1.28)

leading to the linear form

(23.1.28')

The characteristic equation

−

=−=

−

λω

ωλ

has real roots of opposite signs

=±

1,2

λω, the singular point being a saddle point;

hence, the equilibrium is instable, the known results being thus verified.

Stability and Vibrations

525

23.1.1.5 Stability of the Equilibrium of an Autonomous Discrete Mechanical

System with

n Degrees of Freedom, in Linear Approximation

Let be, in general, an autonomous system of differential equation

( , ,..., ), 1,2,...,

Xxx x i n

(23.1.29)

for which the point

( , ,..., )

αα α corresponds to a position of equilibrium; the

system of equations

( , ,..., ) 0, 1,2,...,

Xinαα α ,

(23.1.29')

specifies the set of these points. Starting from one of these points, the origin

(0,0,...,0)

becomes a position of equilibrium by the translation

, 1,2,...,

iii

xx i nα→+ = . We

can assume, in general, that the system of differential equations (23.1.29) has a position

of equilibrium at the origin, this one being a singular point. An expansion into a

Maclaurin series leads to

=+ =

∑12 12

( , ,..., ) ( , ,..., ), 1,2,...,

iijji

Xxx x ax Rxx x i n,

(23.1.30)

where

, ,...,

RR R

are remainders (powers of higher order of the variables

, ,...,

xx x) and where , , 1,2,...,

aij n= , are constants.

In a linear approximation, we consider the system (called the system of the first

approximation too)

∑

,1,2,...,

ij j

ax i n

(23.1.31)

Let us search solutions of the form

== >

∑

e , 1,2,...., , 0

ii i

xu i n u

;

(23.1.32)

introducing in (23.1.31), we get

∑

,1,2,...,

ij j i

au u i nλ ,

(23.1.33)

after simplifying with

≠e0

tλ

, or

()

−==

∑

0, 1,2,...,

ij ij j

aui nλδ ,

(23.1.33')

MECHANICAL SYSTEMS, CLASSICAL MODELS

526

where

δ is the symbol of Kronecker. Because at least one of the solutions

u is

non-zero, the determinant of the coefficients of the system of linear algebraic equations

(23.1.33') must vanish, so that

[

]

=−=() det 0

ij ij

Daλλδ,

(23.1.34)

obtaining thus for

λ an algebraic equation of n th degree

−

++++=

... 0

aa a aλλ λ ,

(23.1.35)

which has the roots

, 1,2,...,

inλ = .

The equation (23.1.34) is the characteristic (secular) equation of the matrix of

coefficients

[

]

a ;

(23.1.36)

introducing the roots

λ , called eigenvalues, in the system (23.1.33), we find n sets of

solutions

u , which form the eigenvectors. We introduce also the column matrices

(column vectors)

⎡

⎤⎡⎤

⎢

⎥⎢⎥

⎢

⎥⎢⎥

⎢

⎥⎢⎥

⎢

⎥⎢⎥

⎢

⎥⎢⎥

⎣

⎦⎣⎦

(23.1.36')

and the system of differential equations can be written in the form

(23.1.31')

its solutions being given by

=xue

tλ

(23.1.32')

The eigenvectors are given by the equation

,λ=≠0Au u u

(23.1.33'')

and the eigenvalues by the equation

[

]

=−=A() det 0D λλδ ,

(23.1.34')

where

δ is the unit matrix.

One must make a study of the eigenvalues, to can decide on the stability or instability

character of the position of equilibrium.

Stability and Vibrations

527

Let us suppose firstly that all the roots are simple; to each root

λ corresponds an

eigenvector

and a solution e , 1,2,...,

=u ; a linear combination of these

solutions

∑

(23.1.37)

where

, 1,2,...,

Ck n= , are arbitrary constants, is – as well – a solution of the system

of linear differential equations (23.1.31).

To show that (23.1.37) is the general solution of the mentioned system, one must

show firstly that the eigenvectors

, 1,2,...,

kn=u , form a system of linear

independent vectors. Let be

∑

0u ;

multiplying at the left by the matrix

and taking into account the equation (23.1.33''),

written for the index

k , we obtain

kk k

cλ

∑

0u .

Eliminating

c between the last two relations, we get

()

kkk

cλλ

−=

∑

0u .

Multiplying further, at the left, this last relation by the matrix

A and taking into account

the equation (23.1.33''), we can eliminate

c between the equation just obtained and the

previous one; proceeding – further – analogously, we can eliminate all the constants

c ,

obtaining, finally,

()()( )

12 1

...

nn n nn

cλλλλ λλ

−

−− − =0u ,

wherefrom

= 0

c , all the brackets being non-zero. Because all constants

c are

equivalent, it follows that

=====

121

... 0

ccc c , the eigenvectors uu u

, ,...,

being thus linearly independent. Putting

= 0t in (23.1.37), we can write

∑

c ;

(23.1.37')

hence, assuming the initial conditions

=xx

(0) , in a Cauchy problem, we can

determine, univocally, the constants

, 1,2,...,

ck n= , because the vectors u

are

linearly independent and form a basis in an

n -dimensional space. Thus, the generality

of the solution (23.1.37) is proved.

MECHANICAL SYSTEMS, CLASSICAL MODELS

528

If one of these roots, let be

λ , is double, then one can show that, besides the

solution

e, e

λλ

uu is a solution too; further, one can show that to a root

λ with a

multiplicity of order

m , corresponds a solution of the form

()

−−

++ ++ u

01 22 11

... e

mm t

kk k k k

CCtCt Ct

(23.1.37'')

The formulae (23.1.37) and (23.1.37') lead to important conclusions concerning the

problem enounced above. Thus:

(i) If all the roots of the equation (23.1.35) are real and negative or are complex

conjugate with a negative real part (

Re 0, 1,2,...,

knλ <=

), then appear factors of

the form

−

tα

and

ecos( ), , 0

βααβ

−

−>

, respectively, so that

→∞

=xlim ( )

t 0 ; the

trajectory of the representative point remains in the neighbourhood of the origin to

which it tends, so that this one is position of asymptotically stable equilibrium.

If some of the roots are simple and purely imaginary (

Re 0, 1,2,...,

knλ ≤= ),

then appear also terms of the form

−cos( )tβα, which do not vanish for →∞t , but

remain bounded for any

t ; the position of equilibrium is simply stable.

(ii) If at least one root is real and positive or if at least two complex conjugate roots

have their real part positive or if at least two complex conjugate roots purely imaginary

are multiple (at least double), then appear terms of the form

−e,e cos( )

αα

βα,

cos( ), , 0ttβααβ−>, which are not bounded for →∞t ; the position of

equilibrium is instable.

Figuratively, let be the complex plane of the roots

λ ; if all the roots are at the left

side of the imaginary axis, then the equilibrium is asymptotically stable, if on the

imaginary axis too are one or several distinct roots, then the equilibrium is simply

stable, while if all the roots are at the right side of the imaginary axis or if on the

imaginary axis there are multiply roots, then the equilibrium is instable.

Let us consider, e.g., the case of a damped linear oscillator, of equation (see Sect.

8.2.2.6)

20, 0xxxμω μ++= >  ,

(23.1.38)

with the notations

2/, /km kmμω

′

, where m is the mass, k is an elastic

coefficient and

′

k is a coefficient of viscous dumping. We can write the equation in the

form of the system

==−−

212

xxx

ωμ;

(23.1.38')

the characteristic equation is

−

=+ +=

−−−

λμλω

ωμλ

Stability and Vibrations

529

wherefrom

=− ± −

1,2

λμμω. The roots are real and negative (if

μω

) or

complex conjugate with the real part negative (if

μω) or we have a negative

double root (

=μω); obviously, the position of equilibrium is asymptotically stable,

the results obtained in Sect. 8.2.2.6 being thus verified.

If we have

< 0μ (self-sustained motions) in the equation (23.1.38), then one can

make an analogous study, finding the same roots, which have a positive real part; the

position of equilibrium is instable, corresponding to the results in Sect. 8.2.2.7.

23.1.1.6 Asymptotic Stability Criteria for the Systems of Linear Differential

Equations

We have seen above that the solution of the system of linear differential equations

(23.1.31) is asymptotically stable at the origin if and only if all the roots of the

characteristic equation have the real part negative; hence, the problem is put to find the

conditions which must be fulfilled by the coefficients of the polynomial function

−

=+ ++ +

() ...

Paa aaλλλ λ,

(23.1.35')

so that its zeros do verify the mentioned property.

We assume that

0a and multiply the equation by −1 if <

0a . Let be

0, 1,2,...,

kmλ <= , the real zeros and let be i, 0

kkk

αβα±<,

=−1,2,...,( )/2knm, the complex conjugate zeros, which are all at the left of the

imaginary axis; we can write

()( )( )

()

()/2

222

() i i

kkkkk

kkk

λλλλαβλαβ

λλ λ αλα β

−

= − −− −+

=− −++

∏∏

(23.1.35'')

Because all the terms at the right side of the expression (23.2.35'') are positive, it

results that all the coefficients of the polynomial function (23.1.35') must be positive

(and non-zero,

0, 0,1,2,...,

aj n>= , the polynomial being non-lacunar); this is a

necessary condition that its zeros have a negative real part.

In 1875, Routh gave an algorithm by means of which, using only the coefficient of

the polynomial

()

Px, one can show if all the considered zeros have their real part

negative; in 1895, Hurwitz stated, independently, an analogous algorithm, expressed in

a more convenient form, by means of some determinants, called Hurwitz’s

determinants, obtained as principal determinants from Hurwitz’s matrix (Routh, E.J.,

1892)

MECHANICAL SYSTEMS, CLASSICAL MODELS

530

−

⎡⎤

⎢⎥

⎣⎦

13 7 21

246 22

13 23

24 24

... ... 0

0 5 ... ... 0

0 ... ... 0

... ... ... ... ... ... ... ...

... ... ... ... ... ... ...

aaaa a

aaa a

(23.1.39)

where

= 0

a everywhere one has >pn or < 0p , in the form

−

== = ==H

112 3 24 1

, , ,..., det

aaa

aaaaa

ΔΔ Δ Δ Δ

(23.1.39')

We can thus state

Theorem 23.1.3 (Routh–Hurwitz criterion). If all Hurwitz’s determinants

corresponding to the polynomial

()

P λ , non-lacunary and with all coefficients

positive, are positive

0, 0,..., 0

ΔΔ Δ>> >,

(23.1.39'')

then and only then all the zeros of this polynomial have their real part negative and the

system of differential equations (23.1.31) has an asymptotically stable position of

equilibrium at the origin.

In particular, if

= 2n , then it results

=> = = >

11 22112

0, 0aaaaΔΔΔ ,

so that it is necessary and sufficient that the three coefficients be positive.

= 3n , then we obtain

== =−>=>

112 12 3 332

,0,0

aaaaaa

ΔΔ ΔΔ;

besides the positivity of the coefficients, the necessary and sufficient condition

−>

12 3

0aa aa

(23.1.40)

must be fulfilled too.

= 4n , then we have

Stability and Vibrations

531

11 212 3

3243214443

0, 0,

0, 0;

aaaaa

aaa

aaa a aa a

ΔΔ

ΔΔΔΔ

=> = − >

==−>=>

as one can easy see, the condition

0Δ can be fulfilled only if the condition

0Δ is also fulfilled. Hence, besides the positivity of the coefficients, only the

necessary and sufficient condition

()

−−>

312 3 14

0aaa aa aa

(23.1.40')

must be added.

For instance, corresponding to what has been said before, the position of equilibrium

is asymptotically stable for the equation (23.1.38), because all the coefficients of the

characteristic equation (of second degree) are positive.

The Routh–Hurwitz criterion is easy to apply if the characteristic equation has

numerical coefficients; but if these coefficients contain also literal parameters, then the

problem becomes complicated from a practical point of view. It is useful to consider

also the conditions established by Liénard and Chipart in 1914, where the number of

inequalities is reduced to a half with respect to the conditions (23.1.39''). As a matter of

fact, these conditions come from the observations made on Hurwitz’s determinants,

assuming – further – the positivity of the coefficients of the characteristic equation. One

sees easily from (23.1.39') that

> 0

Δ leads to

−

Δ . One can show also that the

condition

−

Δ includes also the condition

−

Δ , as it has been seen in the

preceding particular case; then,

−

Δ includes the condition

−

Δ a.s.o. We

can state

Theorem 23.1.4 (Liénard–Chipart criterion). If Hurwitz’s determinants, corresponding

to the polynomial

()

P λ , non-lacunary and with all coefficients positive, verify the

conditions

−

−−

>>>

0, 0, 0, ...

ΔΔΔ ,

(23.1.39''')

then and only then all zeros of this polynomial have the real part negative and the

system of differential equations (23.1.31) admit an asymptotically stable position of

equilibrium at the origin.

Let us write the polynomial

()

P λ in the form

()

=−

∏

()

Paλλλ

(23.1.35''')