Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

MECHANICAL SYSTEMS, CLASSICAL MODELS

422

it means that the point

123

(,, )qqq cannot move on any curve in

Λ , but only along

some curves which – at any point of them – are normal to the vector

123

(,, )aaa at the

respective point. As we have seen in Sect. 3.2.2.6 (formula (3.2.30'')), the necessary and

sufficient conditions of holonomy is

⋅=

123 123

(,, )curl(,, ) 0aaa aaa .

(22.1.14')

As it has been shown by Carathéodory, if we have

⋅≠

123 123

(,, )curl(,, ) 0aaa aaa in

a given domain, then any two points of this domain can be linked by an admissible

curve (even if the constraint relation (22.1.14) takes place).

In the general case of the constraint relations (22.1.13'), A. M. Lipschitz has

introduced a linear transformation in any point of the space (;)qt , obtaining a

necessary and sufficient condition of holonomy of the form

δ−δ=dd 0Mq Mq ,

(22.1.15)

for any displacements in the

−()sm-dimensional hyperplane; this condition is

equivalent to the conditions expressed in the Theorem 3.2.2 of Frobenius (see Sect.

3.2.2.6). We are thus led to the necessary and sufficient conditions

()

δ−δ=

∑

dd 0

kj kj

aq aq .

(22.1.15')

Returning, in particular, to the constraint relation (22.1.14), we obtain the necessary

and sufficient conditions

δ+δ+δ−δ−δ−δ=

11 22 33 11 22 33

dddddd 0aq aq aq aq aq aq

or, in a developed form,

()()

()

∂∂ ∂∂

⎛⎞ ⎛⎞

− δ −δ − δ −δ

⎜⎟ ⎜⎟

∂∂ ∂∂

⎝⎠ ⎝⎠

∂∂

⎛⎞

+− δ−δ =

⎜⎟

∂∂

⎝⎠

23 31

32 23 13 31

32 13

21 12

dd dd

dd0,

aa aa

qq qq qq qq

qq qq

for any

δδδ

123

,,qqq

and

123

d,d,dqqq

which equate to zero the Pfaffian (22.1.14);

this condition can be written also in the form

[

]

⋅δ × =

123 123 123

curl( , , ) ( , , ) d( , , ) 0aaa qqq qqq .

But the displacements

δ(

123

,,)qqq and

123

d( , , )qqq satisfy the conditions

⋅δ = ⋅ =

123 123 123 123

(,, ) (,, ) 0,(,, )d(,, ) 0aaa qqq aaa qqq

Dynamics of Non-holonomic Mechanical Systems

423

being normal to the vector

123

(,, )aaa ; hence, the vector δ( ×

123 123

,,)d(,,)qqq qqq is

collinear with the vector

123

(,, )aaa , obtaining again the condition (22.1.14').

In case of a disc which rolls on a horizontal plane, e.g., one writes the constraint

relations (22.1.3''); we mention that the bilinear covariants

δ− δ − δ+ δsin d sin d , cos d cos dRR RRψψϕ ψϕψ ψψϕ ψϕψ

can vanish only if

==sin 0,cos 0ψψ, the corresponding constraints being thus

non-holonomic.

We say that a function

( , , ,..., )

fq q q q is a first integral of the system (22.1.13) if

for any

d ,d , d ,...,d

qqq q which satisfy this system we have

∂∂∂ ∂

=++++=

∂∂∂ ∂

d d d d ... d 0

fff f

fq qq q

qqq q

hence if =

d0f . Obviously, each integral of the system of equations (22.1.13) allows to

reduce by a unity the number of co-ordinates which determine the position of the repre-

sentative point; as well, if the number of the independent co-ordinates may be reduced due

to the existence of a functional relation =

0f between the co-ordinates

, ,...,

qq q and

the time

q , then the function f is an integral of the system of equations (22.1.13). Hence,

the problem to determine the number of co-ordinates and the number of constraints of a

mechanical system

S is reduced to the problem of finding all the functional independent

integrals of the system (22.2.13). Finally, the system of constraint relations (22.1.13) is

equivalent to

≤mm holonomic constraints and to

−mm

non-holonomic

(non-integrable) constraints; hence, the representative point (the mechanical system

S ) is

specified by

−sm generalized co-ordinates and has −+smm degrees of freedom.

22.1.2.2 Quasi-co-ordinates. Relations of Transposition

The velocity of the representative point corresponding to a given configuration of the

mechanical system

S at a certain moment can be specified by means of the

generalized velocities or by the kinematical characteristics (22.1.4), introduced in Sect.

22.1.1.2, where we take

1,2,...,is

. These characteristics are total derivatives with

respect to time only if

∂

−= = =

∂∂ ∂

0, , , , 1,2,...,

ik i

ijk s

qq q

 ,

(22.1.16)

case in which the generalized co-ordinates can be obtained by a simple integration;

otherwise, the determination of the generalized co-ordinates needs the integration of a

system of linear differential equations, which is – in general – a difficult problem. We

introduce thus the quantities

∫

d , 1,2,...,

ti sπω ,

(22.1.17)

MECHANICAL SYSTEMS, CLASSICAL MODELS

424

which have a certain analogy with the co-ordinates, but are not functions of the position

of the mechanical system

S ; therefore, these quantities are called quasi-co-ordinates

(if

qω  , then it results =

qπ ) and form a corresponding representative space

′



We can write

== + =

ddd d,1,2,...,

ii ijji

tq ti sπω α α ,

(22.1.18)

too.

Taking into account (22.1.4'), we also have

=+ =

d d d , 1,2,...,

kki k

qtksβπ β ,

(22.1.18')

where

=δ =− =

, , , 1,2,...,

ij i

ki jk k ki

jk sαβ β α β .

(22.1.18'')

By introducing the kinematic characteristics (and the quasi-co-ordinates), we can

identify

m of them with the left member of the constraint relations (22.1.1')

=+ =

, 1,2,...,

kkj k

aq a k mω 

(22.1.19)

the last relations being thus written in the form

==0, 1,2,...,

kmω .

(22.1.20)

Thus, by using quasi-co-ordinates, one can establish a generalized form of Lagrange’s

equations.

The representative point

P of co-ordinates

, ,...,

qq qdescribes, during the motion

of the mechanical system

S , a curve

, in the space of configurations

Λ , while the

point

Π of quasi-co-ordinates

, ,...,

ππ π describes a curve Γ in an analogous

space

′



of the quasi-co-ordinates; both curves describe – completely – the motion, so

that there is not any difference between the generalized co-ordinates and the

quasi-co-ordinates. However, to any point

P corresponds only one position of the

mechanical system

S , but to a point

can correspond any position of this system.

We notice thus that a correspondence between a point

Π and an arbitrary position of

the mechanical system

S can be established only if, after travelling through a closed

curve

Γ , the mechanical system would return to the same position.

Let be

Δ= =

∫

d , 1,2,...,

ti sπω

(22.1.21)

the variations of the quasi-co-ordinates corresponding to the travelling through a closed

curve

by the representative point

; we notice thus that to a contour

in the space

Dynamics of Non-holonomic Mechanical Systems

425

of configurations

Λ corresponds – in general – an open contour Γ in the space of

quasi-co-ordinates

′



, because the kinematic characteristics are not integrable.

Using the virtual generalized displacements, we can write

δ= δ =, 1,2,...,

iijj

qi sπα ,

(22.1.22)

δ= δ =, 1,2,...,

kki

qksβπ .

(22.1.22')

Applying the operator

to the relations (22.1.22), it results

∂

δ= δ+ δ+ δ

∂

dd d d

iijj jijj

qqqtq

πα α ;

as well, by applying the operator

δ to the relations (22.1.18), we get

∂

δ=δ+ δ + δ

∂∂

dd d d

iijj j

qqq qt

πα .

Subtracting the two relations one of the other, we can write

()

dd dd d d.

ik i

iiijjj jij j

qq qq qt

qq q

ππα α

∂

⎛⎞⎛⎞

δ−δ = δ−δ + − δ+ − δ

⎜⎟⎜⎟

∂∂ ∂

⎝⎠⎝⎠



Taking into account (22.1.18'), (22.1.22') and denoting

∂

⎛⎞

=− =

⎜⎟

∂∂

⎝⎠

∂

⎛⎞⎛⎞

=− +− =

⎜⎟⎜⎟

∂∂ ∂

⎝⎠⎝⎠

, , , 1,2,..., ,

, , 1,2,..., ,

lm kl

ik i

jm ij jm

ilm s

im s

qq q

γββ

γββαβ

(22.1.23)

the relations of transposition read

()

d d d d d d , 1,2,..., .

mmm

iiijjj

lm l

qq ti sππα γππγπδ−δ = δ−δ + δ + δ =

(22.1.23)

The permutability relations of the operators

d and δ applied to the generalized

co-ordinates (

δ=δdd

qq) lead to the relations of transposition (the transitivity

relations of K. Heun)

d d d d , 1,2,...,

mmm

lm l

ti sππγππγπδ−δ = δ + δ = ;

(22.1.24)

in the particular case in which

(we have

too) and = 0

α ,

=, 1,2,...,ij s, hence in the case in which the kinematic characteristics do not depend

explicitly on time, we obtain

= 0

γ , while the relations (22.1.24) read

MECHANICAL SYSTEMS, CLASSICAL MODELS

426

d d d , 1,2,..., .

lm l

isππγππδ−δ = δ =

(22.1.24')

The obvious relations

, (!) 0, , , 1,2,..., ,

iii

lm ml ll

ilm sγγγ=− = =

(22.1.23'')

take place. Replacing in (22.1.24') the symbol of variation

δ by the differential d , it

results

==d d 0, 1,2,...,

lm l

isγππ ,

(22.1.25)

putting thus in evidence the gyroscopic nature of the non-holonomic terms in the

equations of a non-holonomic mechanical system. We mention that the relations

(22.1.24) and (22.1.24') allow to determine, easily, the coefficients

γ and

γ .

As an example of kinematic characteristics frequently used, we mention the

components of the angular velocity

ω of a rigid solid along the principal axes of

inertia, expressed by means of Euler’s angles (generalized co-ordinates) and by means

of their derivatives with respect to time (generalized velocities), given by the relations

(5.2.35). The variations (22.1.21) of the quasi-co-ordinates are

()

Δ= = +

Δ= = − +

Δ= = +

∫∫

dcosdsinsind,

d sin d sin cos d ,

ddcosd;

πω ϕθθϕψ

πω ϕθψ

(22.1.26)

we get thus

()

()( )

Δ=δ−δ =δ −δ

+δ − δ +δ − δ

Δ=δ−δ =δ −δ

+δ − δ + δ − δ

Δ=δ−δ =δ −δ

dd d d sincos

dd cossin dd sin,

dd d d sinsin

dd coscos dd cos,

dd d d sin.

ππϕψψϕθϕ

θψ θψ θ ϕ θϕ θϕ ϕ

ππψϕψϕθϕ

θψ θψ θ ϕ θϕ θϕ ϕ

ππψθψθθ

(22.1.26')

Corresponding to the relation (5.2.35), we will have (

123

, , qqqψθϕ===

;

0, 1,2,3

iα ==

)

===

==−=

===

11 12 13

21 22 23

31 32 33

sinsin,cos,0,

sin cos , sin , 0,

cos , 0, 1;

α θ ϕα ϕα

αθϕα ϕα

αθαα

as well, the relations (14.1.15) allow to write (

0, 1,2, 3

kβ ==)

Dynamics of Non-holonomic Mechanical Systems

427

11 12 13

21 22 23

31 32 33

sin cosec , cos cosec , 0,

cos , sin , 0,

sin cot , cos cot , 1.

βϕθβ ϕθβ

βϕβ ϕβ

βϕθβ ϕθβ

===

==−=

=− =− =

The first formula (22.1.23) leads to =∈

lm iml

γ , where ∈

iml

is Ricci’s symbol (2.1.29).

In case of a regular precession, defined by

00 0000

, , , , , constmt mtψψθθϕϕψθϕ=+ = =+ = ,

(22.1.27)

the trajectory of the representative point in the space of configuration is a straight line

in the plane

θθ

. In the space of quasi-co-ordinates we have

()

[]

()

[]

()

=− + −

000

sin cos cos ,

sin sin sin ,

cos ,

mnt

πθ ϕϕ

πθϕϕ

πθ

(22.1.27')

the trajectory of the point

being a spiral on a circular cylinder; this last

representation is useful for the study of the motion of the angular velocity vector

ω .

22.1.2.3 Non-holonomic Spaces. Vrănceanu’s Theorems

Being situated in the space of configurations

Λ , let be a holonomic and catastatic

(hence, scleronomic) mechanical system, for which the kinetic energy is of the form

()

ij i j

Tgqqq;

(22.1.28)

we organize this space as a Riemannian space, defined by the element of arc

d()dd

ij i j

sgqqq.

(22.1.28')

Corresponding to the Theorem 20.1.12 of Jacobi (see Sect. 22.1.4.2), being given a

discrete mechanical system

S , subjected to holonomic and scleronomic ideal

constraints, acted upon by no one force, the trajectory of the point

P in the space

Λ ,

endowed with the metrics (22.1.28'), is a geodesic of this space.

Let be

, 1,2,...,

vj s= , the components of a vector with the origin at the point P ,

in the space

Λ . The formulae

⎧⎫

⎪⎪

=− =

⎨⎬

⎪⎪

⎩⎭

d d , 1,2,...,

vvqjs

(22.1.29)

where one uses Christoffel’s symbol of the second kind, define the parallel

displacement in Levi-Civita’s sense of the vector (of components)

v with the origin at

MECHANICAL SYSTEMS, CLASSICAL MODELS

428

the point (

, ,...,

qq q), the vector displaced being + d

vv with the origin at the

point (

++ +

1122

d , d ,..., d

qqqqqq). In this case, the geodesics of the space

are

the curves along which the tangent vector is displaced by parallelism; these curves are

called autoparallel curves too. We can state

Theorem 22.1.1 The trajectory of the representative point P in the space

endowed with the metrics (22.2.28'), corresponding to the motion of a discrete

mechanical system

S , subjected to holonomic and scleronomic, ideal constraints and

acted by no one force is an autoparallel curve of this space.

One obtains thus a generalization of the principle of inertia, the straight lines in the

space

E being just its autoparallel curves.

Let us consider now, in the Riemannian space

Λ , a Pfaff system

===d d 0, 1,2,...,

sqk sλ

;

(22.1.30)

one defines thus a system of orthogonal congruencies if the relations

ij ij

gλλ

(22.1.30')

are satisfied. In this case

()()

()

=+++

21 2

d d d ... d

ss s s,

(22.1.31)

the metrics of the space

Λ being thus expressed in the form of a sum of squares.

Solving the system (22.1.30) with respect to

q , we obtain

==d d , 1,2,...,

qsj sμ ,

(22.1.32)

where

μ is the normalized algebraic complement of the element

λ , so that

=δ

ki i

λμ . The system of congruencies is thus definite by the differential system

=== =

... , 1,2,...,

μμ

(22.1.33)

through each point of

Λ passing one curve of each of the s families;

, ,...,

kk k

μμ μ

are the components of the tangent vector at a point of the corresponding curve which

passes through that point. Multiplying both members of the relation (22.1.30') by

μμ

and summing, we get

=δ

kkl

g μμ ;

(22.1.34)

hence, the vectors

{

}

, ,...,

kk k

μμ μ

are unitary and orthogonal two by two.

Dynamics of Non-holonomic Mechanical Systems

429

Being given a vector

=,1,2,...,

Vi s, in

Λ , by Levi-Civita’s parallel displacement

one obtains the vector

+ d

VV, with

⎧⎫

⎪⎪

=− =

⎨⎬

⎪⎪

⎩⎭

ddd

ijj

kjkk

VVqVq

γ ,

(22.1.35)

where

iimninimnmn

jjj

klklk lkk

mn mn

γλμμλμλμμμμ

∂

⎧⎫ ⎧⎫

∂

=− − =− +

⎨⎬ ⎨⎬

∂∂

⎩⎭ ⎩⎭

(22.1.35')

are Ricci’s coefficients of rotation.

We assume that, in

Λ , one gives a Pfaff’s system

=== =

d d ... d 0

ss s ,

(22.1.36)

which is not integrable; this system defines a non-holonomic space

−sm

Λ . A

displacement

q is called interior to this space if

==dd0

αα

for

= 1,2,...,mα ; more general, a vector is called interior to the non-holonomic space

−sm

Λ if its components , 1,2,...,Vm

α = , are all equal to zero. In this space too we

introduce a parallel displacement characterized by the formulae (22.1.35), (22.1.35'),

stating (Vrănceanu, Gh., 1936)

Theorem 22.1.2 (Gh. Vrănceanu). To a non-holonomic space

−sm

, defined by the

metrics (22.1.28') and by the equations (22.1.36), one can univocally associate a

parallel displacement of the interior vectors, along the interior paths, maintaining the

lengths.

Let be the vectors

, 1,2,...,

uis

λ ,

(22.1.37)

with

0, 1,2,...,um

α== ; we can write

∑

,1,2,...,

uj s

μ ,

(22.1.37')

where

u are the components of the vector tangent to the curve on the congruencies

{

}

λ .

The curve is autoparallel if the relations

=dd

uusγ hold for = d/d

ust; hence,

the autoparallel curves in the space

−sm

Λ verify the equations

==++

∑

, 1, 2,...,

jk k

jk m

uu i m m s

γ .

(22.1.38)

MECHANICAL SYSTEMS, CLASSICAL MODELS

430

We obtain thus a differential system (22.1.37'), (22.1.38) of the first order of

−2sm

equations with

−2sm unknown functions , 1,2,...,

qj s= , and , 1,

ui m=+

+ 2,...,ms

. A curve is definite if a point and a vector interior to the space

−sm

Λ are

given. If the equations (22.1.36) represent the constraints of a non-holonomic

mechanical system

S , then the equations (22.1.37'), (22.1.38) are equivalent to

Lagrange’s equations in the quasi-co-ordinates

s , which define the trajectories in the

absence of the given forces. We can thus state

Theorem 22.1.3 (Gh. Vrănceanu). The trajectory of the representative point P in the

space

−sm

Λ defined by the metrics (22.1.28') and the conditions (22.1.36),

corresponding to the motion of a discrete mechanical system

S subjected to

non-holonomic and catastatic ideal constraints, non-acted upon by given forces, is an

autoparallel curve of this space.

22.2 Lagrange’s Equations. Other Equations of Motion

We considered, in Sect. 17.1.2, the motion of a rigid solid which slides without

friction on a fixed plane, in particular the motion of a heavy homogeneous rigid solid of

rotation, of a heavy gyroscope or of a cylindrical homogeneous rigid solid; as well, the

motion without sliding of a sphere on a fixed plane, in particular that of a heavy

homogeneous one on a fixed horizontal plane, has been considered. We mention also

the results concerning the motion without sliding of a heavy circular disc on a fixed

horizontal plane. These problems have been studied by means of the Newtonian

equations of classical mechanics. In this order of ideas we consider the motion of an

arbitrary rigid solid on a fixed surface too.

After introducing Lagrange’s equations, one studies the above mentioned problems

in the frame of Lagrangian mechanics. One presents then some other equations of

motion.

22.2.1 Motion of a Rigid Solid on a Fixed Surface

In what follows, we study first of all the motion of a rigid solid on a fixed horizontal

plane, using Newtonian equations of classical mechanics; as well, we consider the

motion of a sphere on a perfect rough fixed surface. In what concerns the kinematics of

motion, we use the results obtained in Sect. 22.1.1.3.

22.2.1.1 Motion of a Rigid Solid on a Fixed Horizontal Plane

Let be a rigid solid which moves on a horizontal plane

, with support at three

points. Two of the supports slide freely (without friction) on a plane, while the third one

is a knife edge (a small wheel with a sharpened rim), rigidly connected to the solid in

motion; one assumes that the contact point

of the knife can move freely in the plane

along it, but not along a normal to its direction. This problem has been studied in

1911 by Chaplygin and solved by quadratures; therefore it will be called Chaplygin’s

problem. Later, it was considered in detail, in a particular case, by Carathéodory, in

1933, the respective mechanical system being called a sleigh. Other results are due to

Wagner.

Dynamics of Non-holonomic Mechanical Systems

431

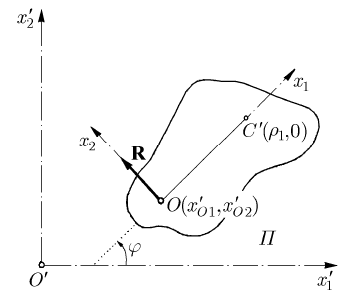
We consider the fixed frame of reference

′′′

Oxx and the movable frame of reference

Ox x , rigidly connected to the sleigh in the plane Π ; the

Ox -axis is along the

direction of the knife, the point

being the theoretical point of contact. The position

of the movable frame is specified by the co-ordinates

′′

xx of the point O and by

the angle

ϕ made by the

-axis with the

′′

Ox -axis (Fig. 22.10); thus, the problem is

reduced to the determination of the functions

(), 1,2

Ok Ok

xxtk

′′

==, and = ()tϕϕ .

But it is more convenient to choose as unknowns the components

′

v and

′

v of the

velocity along the axes of the movable frame of reference, as well as the angular

velocity of rotation

=ωϕ of the rigid solid about a vertical axis. We denote by

I the

moment of inertia of the rigid solid with respect to a vertical axis which passes through

the mass centre

C (of position vector

with respect to O ) of the rigid solid of mass

M . The constraint force R , applied at O , is directed along the

Ox -axis. For the sake

of simplicity, we assume that

0ρ , the centre of mass being projected at the point

′

(,)COρ , on the

′′

Ox -axis.

Fig. 22.10 Motion of a rigid solid with supports at three points

The theorem of momentum (14.1.60) reads

()

[

]

′′

+× +×+× × =

vv R



ωω

(22.2.1)

We have (

== =

12 3

0,ωω ωω)

()

′′

−−=

′′

++ =

Mv v R

ωωρ

ωρ



(22.2.1')

in projection on the axes of the movable frame. As well, the theorem of moment of

momentum with respect to a vertical axis passing through

leads to

=−

IRωρ



(22.2.2)