Teodorescu P.P. Mechanical Systems, Classical Models Volume II: Mechanics of Discrete and Continuous Systems
Подождите немного. Документ загружается.


Assuming that at the contact of the two spheres intervene internal forces, hence
internal percussions, only along the direction of the centres (we neglect the friction
forces and the corresponding percussions), we can use the results in Sect.. 2.1.2
(especially the formulae (17.2.9) (for
1
v
′
and
2
v
′
), to which we associate the relations
11
uu
′′ ′
= ,
22
uu
′′ ′
= . In this case,
22
111
Vvu
′′ ′′ ′
=+,
22
222
Vvu
′′ ′′ ′
=+,
(17.2.12)
the angles made by the velocities
1
′′
V and
2
′′
V with the line of centres being given by
121 1
1
1
1
1211 222
()sin
tan
( ) cos (1 ) cos
mmV
u
v
mkmV kmV
B
C
BB
′
′
+
==
′′
′′
−++
,
122 2
2
2
2
11 1 2 1 2 2
()sin
tan
(1 ) cos ( ) cos
mmV
u
v
kmV m km V
B
C
BB
′
′
+
==
′′
′′
++−
,
(17.2.12')
respectively.
As well, the formulae (17.2.9) lead to
1211 222
1
12 1
( ) cos (1 ) cos
()cos
mkmV kmV
V
mm
BB
C
′′
−++
′′
=
+
,
11 1 2 1 2 2
2
12 2
(1 ) cos ( ) cos
()cos
kmV m km V
V
mm
BB
C
′′
++−
′′
=
+
;
(17.2.12'')
using (17.2.10), the loss of kinetic energy reads
()
()
2
02
1122
1
() 1 cos cos
2
TkmVV%BB
′′
=− −
,
12
111
mm m
=+
.
(17.2.12''')
Fig. 17.17 Collision of a sphere with a fixed wall
In particular, let us consider a limit case in which one of the spheres is replaced by a
fixed wall
P, to which a sphere S strikes at the point P with a velocity
′′′
=+Vvu
,
which makes an angle B with the normal component
′
v (Fig. 17.17).
Neglecting the friction, the component
′
u of magnitude sinuV B
′′
= is not
modified; the component
′
v
of magnitude cosvV B
′′
= changes of direction after
collision, becoming
′′
v of magnitude vkv
′′ ′
= (corresponding to the relation (17.2.8),
where we make
22
′′′
==
vv0, the wall being fixed). We find thus
MECHANICAL SYSTEMS, CLASSICAL MODELS
504

222
cos
sin cos
cos
VV k kV V
B
BB
C
′′ ′ ′ ′
=+=≤
,
1
tan tan
k
CB= ,
(17.2.13)
where C is the angle made by the velocity
′′
V with the normal to the plane P (see the
analogous relations (13.1.21'), (13.1.21'') too; because
1k < , it results
CB≥
. Hence,
by the collision of a sphere
S with a fixed wall P, the velocity decreases in magnitude,
while the velocity vector moves away from the normal to the wall. In case of an elastic
collision, we have
1k = ; one obtains VV
′′ ′
= and CB= , finding again Huygens’s
laws of reflection of the light photon which hits obliquely a mirror (the velocity
maintains its magnitude, while the angle of reflection is equal to the angle of incidence).
Another mathematical model has been built up by I. ğăposu in 1991; he supposed
that, besides (17.2.8), one has
21
21
uu
k
uu
′′ ′′
−
=
′′
−
(17.2.8')
too. He obtained thus
1211 222
1
1211 222
()sin(1)sin
tan
( ) cos (1 ) cos
mkmV kmV
mkmV kmV
BB
C
BB
′′
++−
=
′′
−++
,
11 1 2 1 2 2
2
11 1 2 1 2 2
(1 ) sin ( ) sin
tan
(1 ) cos ( ) cos
kmV m km V
kmV m km V
BB
C
BB
′′
−++
=
′′
++−
,
(17.2.14)
results identical with the classical ones for
1k = (elastic collision). The velocities after
collision are
1211 222
1
12 1
( ) cos (1 ) cos
()cos
mkmV kmV
V
mm
BB
C
′′
−++
′′
=
+
1211 222
12 1
( ) sin (1 ) sin
()sin
mkmV kmV
mm
BB
C
′′
++−
=
+
,
11 1 2 1 2 2
2
12 2
(1 ) cos ( ) cos
()cos
kmV m km V
V
mm
BB
C
′′
++−
′′
=
+
11 1 2 1 2 2
12 2
(1 ) sin ( ) sin
()sin
kmV m km V
mm
BB
C
′′
−++
=
+
,
(17.2.14')
their components being given by
11 1
cosvV C
′′ ′′
=
,
22 2
cosvV C
′′ ′′
=
,
11 1
sinuV C
′′ ′′
=
,
22 2
sinuV C
′′ ′′
=
. The loss of kinetic energy becomes
[]
0222
12 12 12
1
() (1) 2 cos( )
2
TkmvvvvBB
′′ ′′
Δ=− +− −
,
(17.2.14'')
vanishing in the elastic case (
1k = ), as in the classical model.
17 Dynamics of Systems of Rigid Solids
505
One can show that the components of the velocities normal to the line of centres
remain constant, as in the classical case, if
12
uu
′′
= , that is if the velocities of the two
spheres have the same inclination on the above mentioned line.
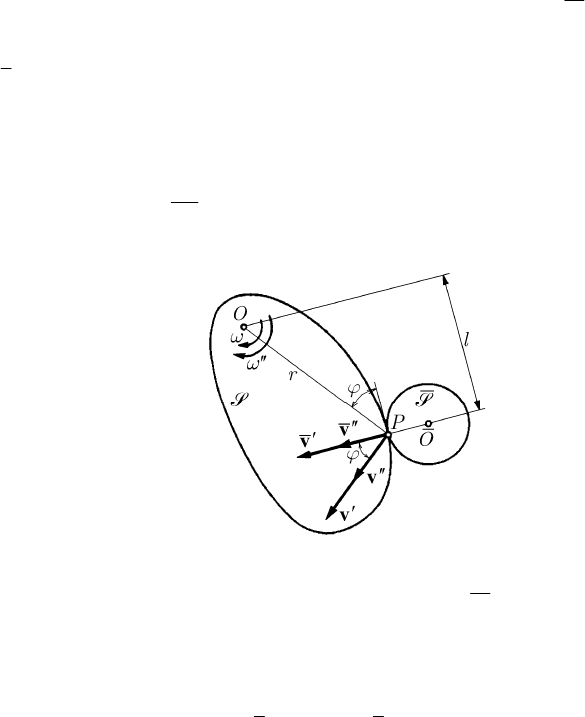
17.2.1.5 Collision of a Sphere with a Rigid Solid in Rotation About a Fixed Axis
Let be a rigid solid
S, which is in rotation about a fixed axis, having a moment of
inertia
I with respect to it; we suppose that this solid is hit by a sphere S of mass m, in
a plane perpendicular to the axis (which contains the pole
O of the axis and the centre
O of the sphere), along the common normal at the point P (Fig. 17.18). We denote by
′
v and
′′
v the velocities of the point P before and after collision, respectively, with
respect to a fixed frame of reference with the pole at
O; the magnitudes of these
velocities, which make the angle
K with the normal at the point P, will be vrX
′′
=
,
vrX
′′ ′′
=
, with rOP= , where
′
ω and
′′
ω are the angular velocity vectors, before
and after collision, respectively.
Fig. 17.18 Collision of a sphere with a rigid solid in rotation about a fixed axis
In case of the mechanical system formed by
S and
S
appear only the external
constraint percussions at the points of the rotation axis; the moment of the percussions
with respect to this axis vanishes, so that we can write a conservative theorem of the
moment of momentum in the form
ImvlI mvlXX
′′ ′′′′
+=+
,
(17.2.15)
where
l is the distance from the point O to the common normal at the point P. We
notice that
cos cosvrlKX KX
′′ ′
==
, cos cosvrlKX KX
′′ ′′ ′′
==
are the components of the velocities
′
v and
′′
v , respectively, along this normal. Taking
into account the study made in Sect. 17.2.1.2, we introduce the coefficient of restitution
MECHANICAL SYSTEMS, CLASSICAL MODELS
506
(to fix the ideas, we assume that cosvv K
′′
>
; otherwise, both the signs of the
denominator and of the numerator change)
cos
cos
vv
lv
k
vv v l
K
X
KX
′′ ′′
′′ ′′
−
−
==
′′ ′ ′
−−
.
(17.2.16)
The relations (17.2.15), (17.2.16) lead to
()
()
2
1
I
vv k v l
Iml
X
′′ ′ ′ ′
=−+ −
+
,
()
()
2
1
ml
kvl
Iml
XX X
′′ ′ ′ ′
=++ −
+
.
(17.2.17)
As well, the loss of the kinetic energy will be given by
()
()
()
2
0
2
2
1
1
2
Im
Tkvl
Iml
%X
′′
=− −
+
.
(17.2.18)
The elastic collision (
1k =
) takes place without loss of kinetic energy; in exchange,
the natural collision (
01k<<
) takes place with loss of kinetic energy, which is
maximal in case of a plastic collision (
0k =
), being given by
()
()
()
2
0
2
2
Im
Tvl
Iml
%X
′′
=−
+
.
(17.2.18')
17.2.1.6 Collision of Two Arbitrary Rigid Solids
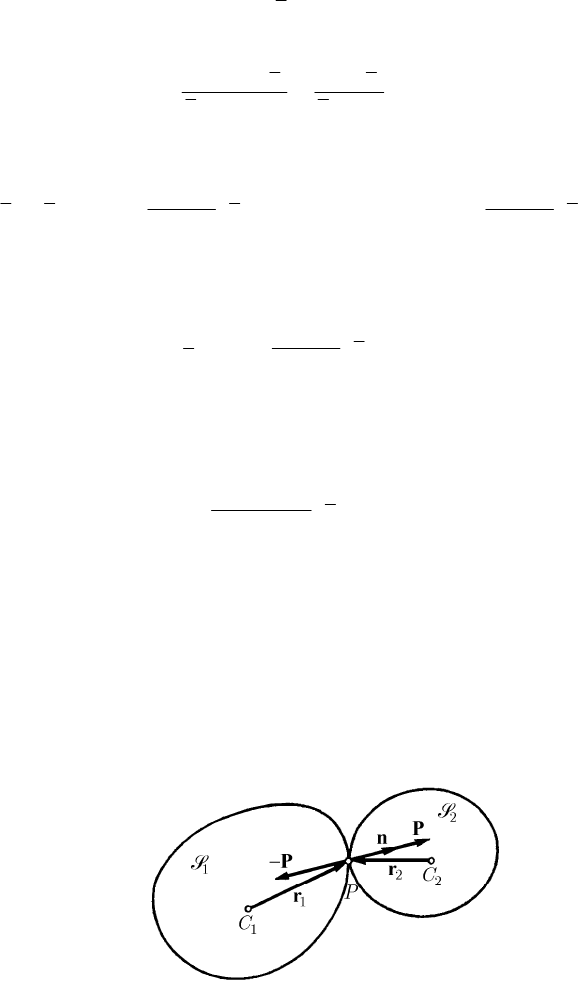
Let us consider now two rigid solids
1
S and
2
S of masses
1
M and
2
M and mass
centres
1
C and
2
C , respectively, which are in collision at the moment
0
t , the point of
impact being
P (Fig. 17.19). We denote by
1
C
′
v
and
2
C
′
v
and
1
C
′′
v
and
2
C
′′
v
, the
velocities of the mass centres before and after collision, respectively, with respect to a
given fixed frame of reference
′
R
; in this case, the theorem of motion of the mass
centre (the formula (13.1.24'')), applied to each rigid solid, gives
Fig. 17.19 Collision of two arbitrary rigid solids
()
11
1
CC
M
′′ ′
−=−vv P,
()
22
2
CC
M
′′ ′
−=vv P,
(17.2.19)
17 Dynamics of Systems of Rigid Solids
507
where P is the percussion, applied at the point P, by which the rigid solid
1
S acts upon
the rigid solid
2
S . As well, if
1
C
′
K
,
2
C
′
K
and
1
C
′′
K
,
2
C
′′
K
, are the angular momenta of
the two solids with respect to the corresponding mass centres, in the movable frames
1
R and
2
R , rigidly linked to the respective solids, with the poles at these centres,
before and after collision, respectively, then we can write the theorem of moment of
momentum (the formula (13.1.25)) with respect to each centre of mass, in the
mentioned frames, in the form
11
1
CC
′′ ′
−=−×
KK rP,
22
2
CC
′′ ′
−=×
KKrP,
(17.2.19')
where
11
CP=
J
JJJG
r ,
22
CP=
J
JJJG
r ; we obtain thus four vector equations (17.1.25), (17.1.25')
(12 scalar equations) for the five vector unknowns
1
′′
v ,
2
′′
v ,
1
C
′′
K ,
2
C
′′
K and P (15
scalar unknowns).
We assume that at the contact point
P do not appear constraint percussions, the
solids
1
S and
2
S being perfectly smooth, and we can write
P=Pn, 0P > , (17.2.20)
where
n is the unit vector of the common normal at the point P, with the sense from the
solid
1
S to the solid
2
S ; thus, the number of the scalar unknowns is reduced to 13.
Otherwise, it is necessary to introduce supplementary hypotheses concerning the
phenomenon of friction (we can introduce, e.g., a Coulombian sliding friction).
The velocities of the points
1
P
and
2
P
of the solids
1
S and
2
S , respectively, which
coincide with the point
P at the theoretic moment of impact, are expressed, with respect
to the fixed frame of reference
′
R
, in the form
11
11
PC
=+×vv rω
,
22
22
PC
=+×vv rω
,
(17.2.21)
where
1
ω and
2
ω are the angular velocities of the frames
1
R and
2
R , respectively,
with respect to the frame
′
R
. The relative velocity of the point
1
P with respect to the
point
2
P will, obviously, be
()
−⋅vvn
12
PP
, hence a velocity of compression,
corresponding to the respective phase (the interval of time
[
)
0
,tt
′
); the respective
velocity vanishes at the end of the phase and we may write
()
12
00
0
PP
−⋅=vvn, so
that the common velocity at the theoretic moment
0
t will be
12
00 0
PP
=⋅=⋅vvnvn
.
(17.2.22)
As well, the equations (17.2.19), (17.2.19') read
()
11
0
1
c
CC
MP
′
−=−vv n,
()
22
0
2
c
CC
MP
′
−=vv n,
(17.2.23)
()
11
0
1
c
CC
P
′
−=−×KK r n,
()
22
0
2
c
CC
P
′
−=×KK r n.
(17.2.23')
MECHANICAL SYSTEMS, CLASSICAL MODELS
508
These four vector equations, together with the scalar equation (17.2.22), where we take
into account (17.2.21), allow to determine the velocities
1
0
C
v ,
2
0
C
v and the angular
momenta
1
0
C
K ,
2
0
C
K , at the end of the phase of compression; in these relations, we take
into account that the angular momenta
1
C
′
K , and
2
C
′
K are expressed as functions of the
rotation angular velocity vectors
1
′
ω
and
2
′
ω
, respectively, so that the number of the
unknown vectors is not great.
The velocity
0
v of the point P at the end of the compression phase (the moment
0
t )
is given by (13.2.22), the corresponding percussion being
()()
11 22
00
12
c
CC CC
PM M
′′
=−⋅=−⋅vvn vvn.
(17.2.24)
By introducing the coefficient of restitution
k, the percussion corresponding to the
restitution phase will be given by
rc
k=PP, so that
()
1
cr c
k=+=+PP P P or
()
1 kP=+
c
Pn, (17.2.25)
the percussion
P being entirely determined. Replacing in (17.2.19), (17.2.19'), the
problem can be completely solved.
One can show that, in case of an elastic collision (
1k =
), the kinetic energy is
conserved (
0
() 0TΔ=
).
17.2.1.7 Theorems of Extremum
Let be a discrete system
S of particles
i
P , of masses
i
m , driven by the velocities
i
′
v
and
i
′′
v before and after collision, respectively, and acted upon by the given and
constraint percussions
i
P and
Ri
P , 1,2,...,ip= , respectively. These particles can be
rigid solids too, modelled as particles, for which we assume that the rotation angular
velocities have not jumps in the interval of percussion. We can write the theorem of
momentum in the form
()
ii i i
Ri
m
′′ ′
−=+vv PP, 1,2,...,ip= ,
(17.2.26)
for each particle; effecting a scalar product of these equations by the arbitrary vectors
i
w ,
1,2,...,ip=
, and summing, we get
()
()
11
pp
ii i i i i
Ri
ii
m
==
′′ ′
−⋅= + ⋅
¦¦
vvw PP w.
(17.2.26')
We notice that this relation is of the form of the principle of virtual velocities (the
formula (13.1.57')).
The mechanical system
S can be subjected to constraints which are maintained
during the application of the percussions, can appear suddenly or can disappear in this
interval of time (as it has been shown in Sect. 17.2.1.1). If
i
w are velocities which
satisfy the constraint relations, then the relation
17 Dynamics of Systems of Rigid Solids
509

1
0
p
i
Ri
i
=
⋅=
¦
Pw
(17.2.27)
corresponding to the relation which defines the ideal constraints, takes place.
If we take into account (17.2.27) and assume that
1
0
p
ii
i
=
′′
⋅=
¦
Pv
(17.2.28)
for
ii
′′
=
wv, then it results
()
1
0
p
ii i i
i
m
=
′′ ′ ′′
−⋅=
¦
vvv .
We can write
()
() ()
2
22
111
11
22
ppp
ii i i i i i i i i
iii
TT mv v m m
===
′ ′′ ′ ′′ ′′ ′ ′′ ′ ′′
−= − = − − − ⋅
¦¦¦
vv vvv.
Introducing the kinetic energy of the lost velocities, we get
()
0
0
0TT% =>
(17.2.28')
and we can state
Theorem 17.2.1 (Carnot, I) If upon a discrete mechanical system S does not act any
given percussive force, then the sudden apparition of a constraint leads to a loss of
kinetic energy.
This theorem can be put in connection with the Theorem 13.1.5.
If we assume that
ii
′
=
wv and that
1
0
p
ii
i
=
′
⋅=
¦
Pv ,
(17.2.29)
then it results
()
1
0
p
ii i i
i
m
=
′′ ′ ′
−⋅=
¦
vvv ,
where we took into account (17.2.27). We can write
()
() ()
2
22
111
11
22
ppp
ii i i i i i i i i
iii
TT mv v m m
===
′′ ′ ′′ ′ ′′ ′ ′′ ′ ′
−= − = − + − ⋅
¦¦¦
vv vvv,
so that
MECHANICAL SYSTEMS, CLASSICAL MODELS
510
()
0
0
0TT% =>,
(17.2.29')
and we may state
Theorem 17.2.2 (Carnot, II) An internal “explosion” in a non-deformable discrete
mechanical system
S leads to a “tearing” of its rigidity, with an increase of the kinetic
energy.
As a matter of fact, by “explosion” we intend the action of an internal percussive
force, which leads to an increase of the distance between the particles, the mechanical
system becoming deformable (the distances between two arbitrary particles do no more
remain constant in time). This result can be put in connection with the Theorem 13.1.6.
Let be a discrete mechanical system
S at rest with an inertial frame of reference; we
assume that one applies percussive forces upon some of the particles
i
P , so that these
particles have prescribed velocities
i
′′
v . Let us consider, as well, a possible motion,
which satisfies the constraints of the system, the considered particles having the
velocities
i
v . Let us denote
iii
′′
=−
wvv and let us suppose that
ii
′′
=
vv; in this
case
1
0
p
ii
i
=
⋅=
¦
Pw ,
(17.2.30)
because
i
=w0 for the particles mentioned above, while
i
=P0 for the other
particles. Observing that the relation (17.2.27) takes, as well, place and assuming that
i
′
=
v0, the relation (17.2.26') reads
()
1
0
p
ii i i
i
m
=
′′ ′′
⋅−=
¦
vvv
and we have
()
() ()
2
22
111
11
22
ppp
ii i iii ii ii
iii
TT mv v m m
===
′′ ′′ ′′ ′′ ′′ ′
−= − = − − ⋅ −
¦¦¦
vv vvv,
so that
()
0
0
0TTΔ=>,
()
0
TTT
′′
Δ=−,
()
2
0
1
1
2
p
ii i
i
Tm
=
′′
=−
¦
vv;
(17.2.30')
we can thus state
Theorem 17.2.3 (Kelvin) If a discrete mechanical system S, at rest with respect to an
inertial frame of reference, at the initial moment, is put in motion by percussive forces
which act upon some of its particles, so that to these particles are impressed prescribed
velocities, then the corresponding kinetic energy is smaller than the kinetic energy of
any possible motion which satisfies the constraints of the system, the considered
particles having the same prescribed velocities.
17 Dynamics of Systems of Rigid Solids
511
Let
i
′
v an
i
′′
v be the velocities of the particles of a mechanical system
S
, with the
general significance previously given (the velocities before and after the application of
the percussive forces, respectively, assuming that the system is subjected to certain
constraints); in case of the existence of supplementary constraints, consistent with the
motion of the system
S before the collision, the application of the same percussive
forces leads to the velocities
i
v after the percussion interval. For
ii
=wv takes place
a relation of the form (17.2.27) in both situations; indeed, in the second case intervene
only supplementary constraint forces. The relation (17.2.26') leads to
()
11
pp
ii i i i i
ii
m
==
′′ ′
−⋅= ⋅
¦¦
vvv Pv,
()
11
pp
ii i i i i
ii
m
==
′
−⋅= ⋅
¦¦
vvv Pv;
subtracting the two relations one from the other, we obtain
()
1
0
p
ii i i
i
m
=
′′
−⋅=
¦
vvv .
(17.2.31)
We can write
()
() ()
2
22
111
11
22
ppp
ii i i i i i i i i
iii
TT mv v m m
===
′′ ′′ ′′ ′′
−= − = − + − ⋅
¦¦¦
vv vvv,
so that
()
0
0
0TTΔ=>,
() ()
0
0
TTΔ=−Δ,
(17.2.31')
with the notations in (17.2.30'); we state
Theorem 17.2.4 (Bertrand) The kinetic energy corresponding to the application of
some percussive forces upon a discrete mechanical system
S, in motion with respect to
an inertial frame of reference, is greater than the kinetic energy corresponding to the
application of the same percussive forces upon the same initial motion, assuming that
supplementary constraints, consistent with the mentioned motion, have been
introduced.
17.2.2 Motion of a Rigid Solid Subjected to the Action of a Percussive
Force
In what follows we consider the motion of a free or constraint rigid solid (with a fixed
axis or with a fixed point) subjected to the action of a percussive force or suddenly
fixed; the results thus obtained will be applied to the ballistic pendulum.
17.2.2.1 Motion of a Rigid Solid with a Fixed Axis Subjected to the Action
of a Percussive Force. Centre of Percussion
Let us consider a rigid solid
S with a fixed axis % (specified by the fixed points O
′
and
1
O , situated at a distance l one of the other). We choose a fixed frame of reference
MECHANICAL SYSTEMS, CLASSICAL MODELS
512
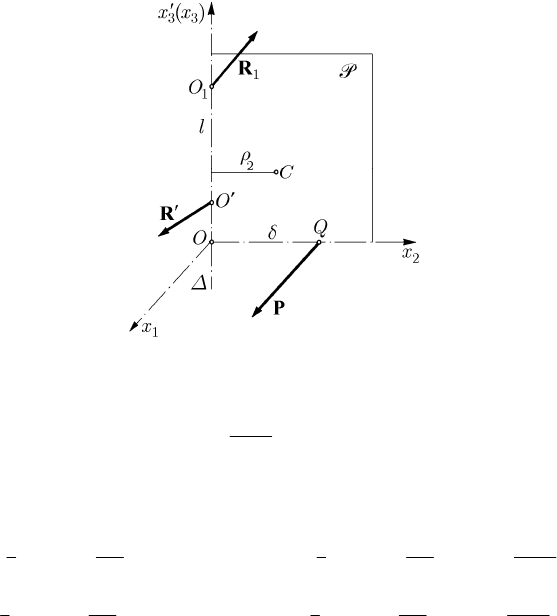
′
R
with the
3
Ox
′′
-axis along
1
OO
′
and a movable frame R with the pole O on the
fixed axis (in general,
O distinct from O
′
) and with the
3
Ox -axis along
1
OO too (Fig.
17.20). We obtain thus the jump relations (17.2.5), (17.2.5'), corresponding to the
theorems of momentum and moment of momentum. We find thus the jump of the
angular velocity
Fig. 17.20 Motion of a rigid solid with a fixed axis subjected
to the action of a percussive force
3
0
33
()
O
M
I
XΔ= ,
(17.2.32)
as well as the components of the constraint percussions
23
11
23
33
1
OO
I
RMM
lI
§·
=− −
¨¸
©¹
,
23 3
11 2
23
33 33
1
O
OO
M
I
RR M M M
lI I
S
§·
′
=− + − −
¨¸
©¹
,
31
12
13
33
1
OO
I
RM M
lI
§·
=−
¨¸
©¹
,
31 3
22 1
13
33 33
1
O
OO
M
I
RR M M M
lI I
S
§·
′
=− − − +
¨¸
©¹
.
(17.2.32')
The other two components remain non-determinate, because one can know only their
sum (
313 3
RR R
′
+=); the rigid solid is statically indeterminate from this point of
view.
Let be the case in which upon the rigid solid
S acts only one percussive force,
which leads to the percussion
P (
ii
RP= , 1, 2, 3i = ). The problem is put to find the
conditions in which the constraint percussions at
O and
1
O vanish (jerks do not appear
in the axis of rotation); we must have
1
0
i
i
RR
′
==, 1, 2, 3i = . In the first case, it
results
3
0P = , so that the percussion must be normal to the axis of rotation. We
choose the
1
Ox -axis parallel to P, so that to have
2
0P = , hence
1
PP= . To simplify
17 Dynamics of Systems of Rigid Solids
513
