Teodorescu P.P. Mechanical Systems, Classical Models Volume II: Mechanics of Discrete and Continuous Systems

Подождите немного. Документ загружается.

15 Dynamics of the Rigid Solid with a Fixed Point

sphere, and the curve

C , invariably linked to the spherical figure mentioned above;

the motion of the spherical figure

F is obtained by rolling without sliding of the curve

C over the curve

C .

15.1.1.2 Kinetical Considerations

In case of the rigid solid with a fixed point we have

′

v0, so that the velocity of the

mass centre

C is given by (see the results in Sect. 14.1.1.5)

′

=×

v ω

(15.1.5)

and the momentum of the rigid solid will be expressed in the form

′

=×H ω

(15.1.6)

We notice that

constρ==

, the centre C describing a curve situated on the sphere

()

0,ρ ; in this case, the magnitudes

′

v and

′

H are in direct proportion to ρ.

As well, we obtain the moment of momentum

′

′′

KKIω ;

(15.1.7)

making

OC≡ , we notice that this formula is identical with the formula (14.1.26'),

which takes place in the case of a free rigid solid. This result was to be expected,

because we have seen that the general motion of a free rigid solid can be studied in two

steps: (i) the motion of the mass centre

C as a free particle at which is concentrated the

whole mass

M of the rigid solid; (ii) the motion (rotation) of the rigid solid about the

centre

C (considered as a fixed point). Hence, if we take OC≡ , then our study is

useful for the second step of the mentioned general problem too. In this case,

= 0

hence

′

v0 and

′

=H0, while the formula (15.1.7) is reduced to the formula

(14.1.26').

The kinetic energy is given by

()

′′

=⋅ =⋅

KIωωω,

(15.1.8)

and we can use all the considerations in Sect. 14.1.1.6; if

OC≡ , then we can write

()

′′

=⋅ =⋅

KIωωω.

(15.1.8')

Besides the torsor

{

}

RM of the given forces, we introduce the constraint force

R , applied at the fixed point O; we notice that

=M0 (Fig.15.3a). In this case, the

elementary work of the given external forces is given by

283

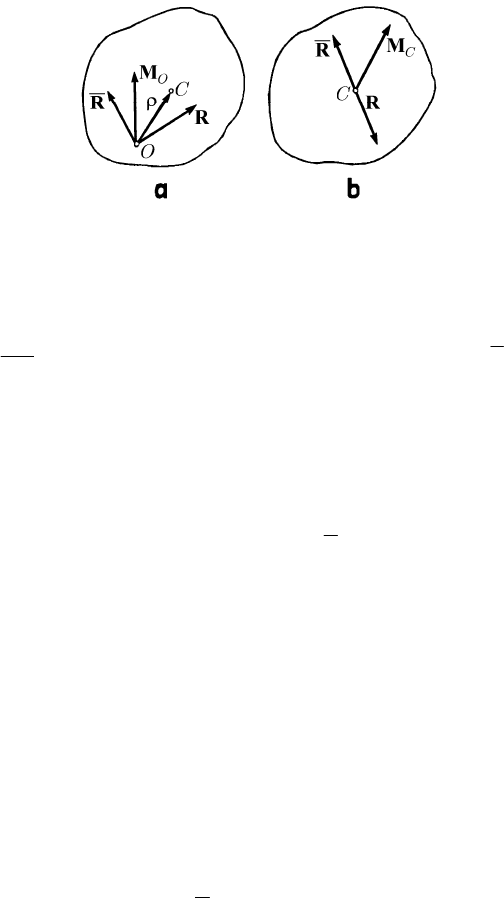
MECHANICAL SYSTEMS, CLASSICAL MODELS

′

=⋅

M ω ,

(15.1.9)

while the elementary work of the external constraint forces vanishes; as well, the power

of the given forces is of the form

′

=⋅

M ω .

(15.1.9')

Fig. 15.3 The rigid solid with a fixed point acted upon by arbitrary external forces;

the case O distinct from C (a) and the case

OC≡

(b)

The vector equations of motion (14.1.60), (14.1.60') become (see Sect. 14.1.2.2 too)

()

[]

CCC

MM M M

′

′′′

= = +× = ×+× × = +



avv RRωωρωωρ

, (15.1.10)

()

OOO

+× =



IIMωω ω ,

(15.1.11)

where

t∂∂

′′



vv is the derivative of the velocity

′

v with respect to time, in the

frame of reference

R. In components, along the axes of the non-inertial frame R we

can write

[

]

()

jji i

Ci ijk k

Ma M R Rωρ ωω ρ

′

=∈ + =+



, 1, 2, 3i = ,

(15.1.10')

()

kl ik l ijk l Oi

IMδω ωω+∈ =



, 1, 2, 3i = ;

(15.1.11')

with respect to the principal axes of inertia, we obtain Euler’s equations (taken again by

Lagrange, Poisson, Poinsot, P. Saint-Guilhem, R.B. Hayward, J.C. Maxwell, G.

Schmidt, P.V. Harlamov etc.)

()

11 3 2 23

III Mωωω+− =



()

22 1 3 31

III Mωωω+− =



()

33 2 1 12

III Mωωω+− =



(15.1.11'')

where

123

III≥≥ are the principal moments of inertia relative to the pole O.

Assuming that

OC≡

, the equation (15.1.11) becomes (Fig. 15.3,b)

+=RR0,

(15.1.10'')

284

15 Dynamics of the Rigid Solid with a Fixed Point

as in the static case, while the equation (15.1.11) takes the form (14.1.48).

We can express the kinetic energy also in the form

()

222 2

11 22 33

TIII I

ωωω ω

′

=++=,

(15.1.8'')

where we have reported to the principal axes of inertia and to the instantaneous axis of

rotation

Δ, respectively (along the direction of the vector ω).

The theorem of kinetic energy (14.1.66) reads

′

=⋅M ω

(15.1.12)

and if the fixed point is just the mass centre, then we have

′

=⋅M ω

(15.1.12')

In components, along the principal axes of inertia, it results

()

111 222 333 1 2 3

123

OO O

II I MMMωω ωω ωω ω ω ω++ =++



(15.1.12'')

while if

OC≡ , then we become the formula (14.1.49').

Sometimes it is useful that the non-inertial frame of reference

R with the pole at O

have a rotation Ω with respect to the rigid solid (not being rigidly connected to it);

obviously, in this case the equations of motion given by the general theorems have a

more intricate form, as it was mentioned in Sect. 14.1.1.4.

Using Euler’s angles and the relations (5.2.35), we can express the kinetic energy

(15.1.8'') in the form

()()

sin sin cos sin cos sin

TI I

ψθϕθϕ ψθϕθϕ

⎡

′

=++−

⎣



()

cosI ψθϕ

⎤

⎦



(15.1.13)

too. If the

O3-axis is a kinetic axis of symmetry of the rigid solid (the ellipsoid of

inertia is of rotation), then we have

IIJ== (important case in applications) and

we obtain the remarkable formula

()

22 2

sin cos

TJ I

ψθθ ψθϕ

⎡⎤

′

=+++

⎣⎦







(15.1.13')

We notice that, in this case,

//0TT∂∂ψ∂∂ϕ==. If the ellipsoid of inertia is a

sphere (

123

IIII===), then we get

()

22 2 2

2cos

TI I

ψθϕ ψϕθ ω

⋅

′

=+++ =





(15.1.13'')

285

MECHANICAL SYSTEMS, CLASSICAL MODELS

in conformity to the formula (15.1.4).

Taking into account the results in Sect. 14.1.1.6 (e.g., the formula (14.1.31)), we can

write

ddd

′′ ′

=⋅=⋅KKωω .

(15.1.14)

Observing that the ellipsoid of inertia is the locus of the points

P for which the position

vector





is given by (see the formulae (14.1.32) and (15.1.8''))

′





ω ,

(15.1.15)

we obtain

′

⋅=





K ; we can thus state that the moment of momentum

′

along the normal

OQ from O to the plane Π (

Q Π∈

), tangent to the ellipsoid of

inertia (see Fig. 3.9 too). The distance from the point

O to the plane Π is given by

vers

hOQOP OP K

′′

⋅

′

==⋅ =⋅=

′

′′





Taking into account (15.1.8), it results

′

(15.1.16)

The vector

J associated to the moment of inertia tensor

I is thus defined in the

form

vers

′

J ω

(15.1.17)

and is situated along the same normal

OQ. In conformity to the formula (14.1.34'),

JOQ K= ; the locus of the extremity P

′

of the vector

(we take

KRM= ,

so that

′

be the inverse of the point Q with respect to a sphere of centre O and of

radius

R; Fig. 3.9) is the ellipsoid of gyration. We obtain thus a graphic method for the

determination of the moment of momentum

′

15.1.1.3 General Methods of Computation. Case of the Heavy Rigid Solid

To determine the motion of the rigid solid with a fixed point

O, subjected to the action

of given forces of torsor

{

}

RM , as well as to the constraint force

(Fig. 15.3,a),

knowing its position at a given moment, we have at our disposal the vector equations of

286

15 Dynamics of the Rigid Solid with a Fixed Point

motion (15.1.10), (15.1.11) (or the equivalent equations) and the relations (5.2.35),

hence two vector equations and three scalar ones for the two vector functions

()t=ωω and ()t=RR and the three scalar functions ()tψψ= , ()tθθ= and

()tϕϕ= .

In general,

()

123

,, , , , ;

RR tψθϕω ω ω= and

123

(,,, , , ;)

Oi Oi

MM tψθϕω ω ω= ,

1,2, 3i = . The six unknown scalar functions (Euler’s angles ()tψψ= , ()tθθ= ,

()tϕϕ= and the components ()

tωω= , 1,2, 3i = , of the angular velocity vector

of the rigid solid) are determined by the system of differential equations (15.1.11''),

written in the normal form



()

[]

12323

MMII

ωωω== +−





()

[]

23131

MMII

ωωω== +−





()

[]

31212

MMII

ωωω== +−



(15.1.18)

and by the system of equations (14.1.53''); the initial conditions (at the moment

tt= )

of Cauchy type will be of the form

()

tψψ=

()

tθθ=

()

tϕϕ=

()

tωω=

1,2, 3

i =

(15.1.19)

Starting from the Theorem 14.1.12, we can state a theorem of existence and uniqueness

of the solution.

It is interesting to see that the formulation of the problem would be more intricate

(equations (14.1.55)–(14.1.55'')) by choosing the centre of mass

C as pole of the

movable frame of reference

If we determine the position of the rigid solid and the angular velocity

ω, then the

equation (15.1.10) allows to express the constraint force in the form

()

[]

M=− + × + × ×



RR ω

ωω

(15.1.20)

OC≡

, then the constraint force is

=−RR,

(15.1.20')

hence the same as in the static case (Fig. 15.3,b).

Introducing the direction cosines

α , 1,2, 3i = , of the

′

-axis with respect to the

axes of the frame of reference

R (one can pass to Euler’s angles by means of the

relations (5.2.36)), the components of the torsor of the given forces are of the form

()

123123

,,,,,;

RR tαααωωω= and

()

123123

,,,,,;

Oi Oi

MM tαααωωω= ; the six

unknown functions

()

tαα= and ()

tωω= , 1, 2, 3i = , are determined by

Poisson’s system of geometric equations (14.1.54), written in the normal form, and by

the system of dynamic equations (15.1.18). Associating the initial conditions

287

MECHANICAL SYSTEMS, CLASSICAL MODELS

()

tαα= , 1, 2, 3i = ,

(15.1.19')

to the initial conditions (15.1.19) (with which they must be compatible), we can state,

analogously, a theorem of existence and uniqueness.

Fig. 15.4 The rigid solid with a fixed point acted upon by its own weight G

An important particular case is that in which the rigid solid is acted only by its own

weight

MMg

′

==−Gg i at the centre of mass C (Fig. 15.4). The equations

(15.1.11'') take the form

() ( )

11 3 2 23 32 23

III Mgωωωραρα+− = −



() ( )

22 1 3 31 13 31

III Mgωωωραρα+− = −



() ( )

33 2 1 12 21 12

III Mgωωωραρα+− = −



(15.1.21)

If the fixed point is just the mass centre, then Euler’s equations (15.1.21) read

() () ()

()

123

132

CCC

IIIωωω+− =



() () ()

()

231

213

CCC

IIIωωω+− =



() () ()

()

312

321

CCC

IIIωωω+− =



(15.1.21')

and form a system of homogeneous equations.

The latter case can be seen as a second step in the study of the motion of the free

rigid solid subjected only to the action of its own weight; the first step is represented by

the study of the motion of the mass centre, the trajectory of which is a parabola (in

particular, the local vertical; see Sect. 14.1.1.8 too).

15.1.1.4 Jacobi’s Multiplier

To determine the first integrals of the systems of differential equations considered

above, a particularly important rôle is played by Jacobi’s multiplier. We will present the

corresponding theory for a system of differential equations

288

15 Dynamics of the Rigid Solid with a Fixed Point

()

12 1

, ,..., ;

xXxx xt

−



1,2,..., 1jn=−

(15.1.22)

As it was shown in Chap. 6, Sect. 1.2.2 and in Sect. 11.1.1.6, if the functions

X and

Xx∂∂, , 1,2,..., 1jk n=−, are defined and continuous in a neighbourhood V of

the point

()

00 0

01 2 1

;,,...,

txx x

−

, then there exists a neighbourhood ⊂VV in which

the solution of the system of differential equations (15.1.22) is obtained by means of the

first integrals

()

12 1

, ,..., ;

xx x t C

−

= , const

C = , 1,2,..., 1jn=−.

(15.1.23)

We can set up at the most

1n − independent first integrals; we say that the first

integrals

{

}

, 1,2,..., 1

kn=− form a fundamental system of first integrals if they are

of class

C with respect to all variables (

x , 1,2,..., 1kn=−, and t), the

corresponding functional determinant being non-zero.

()

12 1

, ,...,

det 0

, ,...,

xx x

∂

−

⎡⎤

≠

⎢⎥

⎣⎦

(15.1.23')

The initial conditions of Cauchy type allow the determination of the constants

C ,

1,2,..., 1jn=−, the solution

()

12 1

;,,...,

xxtCC C

−

= ,

1,2,..., 1jn=−

(15.1.22')

thus obtained being unique.

In a study of the problem of first integrals it is convenient to denote

tx= (t has no

more a privileged position), the system of differential equations (15.1.22) being written

in the form (

X = )

...

XX X

===

(15.1.24)

Thus, a function

()

, ,...,

fxx x= is a first integral of the system (15.1.22) if

dd0

∂

∑

(15.1.25)

Taking into account (15.1.24), we can write this condition in the form

=D ,

∂

∑

D .

(15.1.25')

289

MECHANICAL SYSTEMS, CLASSICAL MODELS

We notice that the system (15.1.24) is just the characteristic differential system

associated to the equation with partial derivatives of first order (15.1.25'), written by

means of the differential operator

D ; hence, we may state

Theorem 15.1.1 The relation (15.1.25') represents the necessary and sufficient

condition so that the function

f be a first integral of the system (15.1.24).

We can write (the function

f depends on the functions

, 1,2,...,km= ,

1mn≤−)

11 1 1

dd d

nm m n

jk k j

fx f x

∂∂

∂∂ ∂ ∂

== = =

∑∑ ∑ ∑

as well as

∂

∑

DD,

(15.1.25'')

=D ,

1,2,...,km=

; it results

Theorem 15.1.2 Any function f which depends on 1mn≤− first integrals

1,2,...,km= , of the system of differential equations (15.1.24) is a first integral of this

system.

If the rank of the matrix

()

, , ,...,

, ,...,

ff f

xx x

∂

⎡

⎤

⎢

⎥

⎣

⎦

1m + , 1mn<−, then the first integral f is independent of the first integrals

1,2,...,km= . Let us suppose now that the relations 0

=D take place for the

functions

X ≠ , 1,2,... 1kn=−, the rank of the matrix

()

12 1

, ,...,

xx x

∂

−

⎡

⎤

⎢

⎥

⎣

⎦

being

1n − ; the 1n − first integrals

are, in this case, independent and form a

fundamental system of first integrals

{

}

,1,2,...,1

kn=−. We have 0

=D and

can state

Theorem 15.1.3 A fundamental system of first integrals of the system (15.1.24) being

given, any other first integral of this system is a function of the considered fundamental

system.

The corresponding functional determinant vanishes

()

12 1

,,,...,

det 0

, ,...,

ff f

xx x

∂

−

⎡⎤

⎢⎥

⎣⎦

(15.1.26)

290

15 Dynamics of the Rigid Solid with a Fixed Point

Developing Jacobi’s determinant

D after the first line, it results

∂

∑

()

DΔ

=− , 1,2,...,in= ,

(15.1.26')

where

D is the minor of the element /

x∂∂ of the first line, while

Δ is the

corresponding algebraic complement. The relations (15.1.25') and (15.1.26), (15.1.26')

are equivalent, both being the necessary and sufficient conditions so that the function

be a first integral of the differential system (15.1.24); hence, there exists a function

called Jacobi’s multiplier, so that

MXΔ = , 1,2,...,in= .

(15.1.27)

The relation

Mf D=D

(15.1.27')

takes place too.

Together with Jacobi, we consider the determinant

11 12 1

1,1 1,2 1,

nn nn

aa a

uu u

−− −

…

…………

…

∂

, const

a = ,

where

1,2,..., 1in=−, 1,2,...,jn= ; using the above notations, we can write

UaΔ

∑

wherefrom

UaΔ∂∂=

, 1,2,...,jn= . Noting that

depends on

x by means of

the quantities

u , 1,2,..., 1in=−, 1,2,...,kn= , kj≠ , we have

11 11

nn nn

jj j

ik i

jjj

ik ik k

ik ik

xuxuxx

∂Δ ∂Δ ∂Δ

∂

∂∂∂∂∂∂

−−

== ==

∑∑ ∑∑

, kj≠ , 1,2,...,jn= ,

so that

1111 111

nnnn nnn

jj j

ik k ik k

jjik ijk

xuxxuxx

∂Δ ∂Δ ∂Δ

∂∂

∂∂∂∂∂∂∂

−−

==== ===

∑∑∑∑ ∑∑∑

111

nnn

ij j

ik k

ijk

uuxx

∂Δ

∂

∂∂∂∂

−

===

⎛⎞

⎜⎟

⎝⎠

∑∑∑

111

nnn

jij j

ik k k

ijk

ua ua xx

∂

∂∂

∂∂ ∂∂ ∂∂

−

===

⎛⎞

⎜⎟

⎝⎠

∑∑∑

291

MECHANICAL SYSTEMS, CLASSICAL MODELS

kj≠

where we took the symmetric part of the parenthesis with respect to the indices

j and k

(the contribution of the corresponding antisymmetric part vanishes) and where we took

into account the expression of the algebraic complement

Δ . Let us consider now, in

the determinant

U, the minor of second order formed by the lines 1 and i and by the

columns

j and k, that is

ik k

au au

=−

The determinant considered above will be thus of the form

()

jij

ik k

UauauUU

′′′

=− +, jk≠ ,

where the algebraic complement

′

of this minor does not contain any of the elements

,, ,

jij

kik

aau u, while U

′′

may depend at the most linearly on these elements. In this

case,

jij

ik k

ua ua

∂∂

∂∂ ∂∂

′′′

+=−=

and one obtains Jacobi’s identity in the form

∂Δ

∂

∑

(15.1.28)

Taking into account (15.1.27), one gets the equation of Jacobi’s multiplier in the

form

()

∂

∑

(15.1.29)

or in the form

()

ln 0

∂

∂∂

∂

⎡⎤

⎢⎥

⎣⎦

∑

(15.1.29')

Writing the latter condition for two multipliers

M and

M and subtracting the two

relations thus obtained one from the other, we get

ln ln 0

xM M

∂

⎛⎞

⎜⎟

⎝⎠

∑

D .

292