Taylor M.E. Partial Differential Equations III: Nonlinear Equations

Подождите немного. Документ загружается.

418 16. Nonlinear Hyperbolic Equations

Now write

(1.28)

g.u/  g.w/ D G.u;w/.u  w/;

G.u;w/D



u C.1 /w



d;

and similarly

(1.29) L.u;D/ L.w; D/ D .u w/  M.u;w;D/:

Then (1.25) yields

(1.30)

D L.u;D/v C A.u; u

; ru

/v C R

;

where

(1.31) A.u; u

; ru

/v D v  M.u; u

;D/u

C G.u; u

incorporates the ﬁrst terms on the right sides of (1.26)and(1.27), and R

is the

sum of the rest of the terms in (1.26)and(1.27). Note that each term making up

has as a factor I J

, acting on either u

;g.u

/,orL.u

;D/u

. Thus there is

an estimate

(1.32) kR

.t/k

 C



.t/k



1 Cku

.t/k



."/

;

where

(1.33) r

."/ DkI  J

L.H

k1

kI  J

L.H

Now, estimating .d=dt/kv.t/k

via the obvious analogue of (1.8)–(1.15)

yields

(1.34)

kv.t/k

 C.t/kv.t/k

C S.t/;

with

(1.35) C.t/ D C



.t/k

; ku.t/k



;S.t/DkR

.t/k

Consequently, by Gronwall’s inequality, with K.t/ D

C./d,

(1.36) kv.t/k

 e

K.t/



kf  hk

S./e

K./

d



;

for t 2 Œ0; B/. A similar argument with time reversed covers t 2 .A; 0,andwe

have the following:

1. Quasi-linear, symmetric hyperbolic systems 419

Proposition 1.3. Fo r k>n=2C1, solutions to (1.1) satisfying (1.18) are unique.

They are limits of solutions u

to (1.4), and, for t 2 I ,

(1.37) ku.t/  u

.t/k

 K

.t/kI  J

L.H

k1

Note that if J

is deﬁned by (1.7)and'./ D 1 for jj1,wehavethe

operator norm estimate

(1.38) kI  J

L.H

k1

 C"

k1

Returning to properties of solutions of (1.1), we want to establish the following

small but nice improvement of (1.18):

Proposition 1.4. Given f 2 H

;k>n=2C 1, the solution u to (1.1) satisﬁes

(1.39) u 2 C.I;H

For the proof, note that (1.18) implies that u.t/ is a continuous function of t

with values in H

.M /,giventheweak topology. To establish (1.39), it sufﬁces

to demonstrate that the norm ku.t/k

is a continuous function of t. We estimate

the rate of change of ku.t/k

by a device similar to the analysis of (1.8). Un-

fortunately, it is not useful to look directly at .d=dt/kD

u.t/k

when j˛jDk,

since LD

u may not be in L

. To get around this, we throw in a factor of J

,and

for j˛jk look at

(1.40)

u.t/k

D 2



L.u;D/u;D





g.u/; D



As above, we have suppressed the dependence on .t; x/, for notational con-

venience. The last term on the right is easy to estimate; we write the ﬁrst

term as

(1.41) 2.D

L.u;D/u;D

u/ D 2.LD

u;D

u/ C 2.ŒD

;Lu;D

u/:

Here, for ﬁxed t; L.u;D/D

u 2 H

1

.M /, which can be paired with D

u 2

.M /. We still have the Moser-type estimate

(1.42) kŒD

;Luk

 C kA

.u/k

kuk

C C kA

.u/k

kuk

;

parallel to (1.13), which gives control over the last term in (1.41). We can write

the ﬁrst term on the right side of (1.41)as

(1.43)



.L C L



u;D



C 2



ŒJ

;LD

u;D



420 16. Nonlinear Hyperbolic Equations

The ﬁrst term is bounded just as in (1.10)–(1.11). As for the last term, we have

(1.44) ŒJ

;Lw D

ŒA

.u/; J

@

Now the nature of J

as a Friedrichs molliﬁer implies the estimate

(1.45) kŒA

@

 C kA

kwk

see Chap. 13,  1,Exercises1–3.

Consequently, we have a bound

(1.46)

u.t/k

 C



ku.t/k



ku.t/k

;

the right side being independent of " 2 .0; 1. This, together with the same anal-

ysis with time reversed, shows that kJ

u.t/k

D N

.t/ is Lipschitz continuous

in t, uniformly in ".AsJ

u.t/ ! u.t/ in H

-norm for each t 2 I , it follows that

ku.t/k

D N

.t/ D lim N

.t/ has this same Lipschitz continuity. Proposition

1.4 is proved.

Unlike the linear case, nonlinear hyperbolic equations need not have smooth

solutions for all t. We will give some examples at the end of this section. Here we

will show, following [Mj], that in a general context, the breakdown of a classical

solution must involve a blow-up of either sup

ju.t; x/j or sup

u.t; x/j.

Proposition 1.5. Suppose u 2 C



Œ0; T /; H

.M /



;k>n=2C 1 (n D dim M ),

and assume u solves the symmetric hyperbolic system (1.1)fort 2 .0; T /. Assume

also that

(1.47) ku.t/k

.M /

 K<1;

for t 2 Œ0; T /. Then there exists T

>T such that u extends to a solution to (1.1),

belonging to C



Œ0; T

/; H

.M /



We remark that, if A

.t; x; u/ and g.t; x; u/ are C

in a region R  M  ,

rather than in all of R  M  R

, we also require

(1.48) u.t; x/  

 ; for t 2 Œ0; T /:

Proof. This follows easily from the estimate (1.46). As noted above, with

.t/ DkJ

u.t/k

,wehaveN

.t/ ! N

.t/ Dku.t/k

pointwise as

" ! 0,and(1.46) takes the form dN

=dt  C

.t/N

.t/. If we write this in an

equivalent integral form:

(1.49) N

.t C /  N

.t/ C

tC

.s/N

.s/ ds;

1. Quasi-linear, symmetric hyperbolic systems 421

it is clear that we can pass to the limit " ! 0, obtaining the differential inequality

(1.50)

 C.ku.t/k

.t/

for the Lipschitz function N

.t/. Now Gronwall’s inequality implies that N

.t/

cannot blow up as t % T unless ku.t/k

does, so we are done.

One consequence of this is local existence of C

-solutions:

Corollary 1.6. If (1.1) is a symmetric hyperbolic system and f 2 C

.M /,then

there is a solution u 2 C

.I M/, for an interval I about 0.

Proof. Pick k>n=2C 1, and apply Theorem 1.2 (plus Proposition 1.4)togeta

solution u 2 C.I;H

.M //. We can also apply these results with f 2 H

.M /,

for ` arbitrarily large, together with uniqueness, to get u 2 C.J;H

.M //,

for some interval J about 0, but possibly J is smaller than I .Butthenwe

can use Proposition 1.5 (for both forward and backward time) to obtain u 2

C.I;H

.M //. This holds, for ﬁxed I , and for arbitrarily large `.Fromthisit

easily follows that u 2 C

.I  M/.

We make some complementary remarks on results that can be obtained from

the estimates derived in this section. In particular, the arguments above hold when

.t; x; u/ and g.t; x; u/ have only H

-regularity in the variables .x; u/, as long

as k>n=2C 1. This is of interest even in the linear case, so we record the

following conclusion:

Proposition 1.7. Given A

.t; x/ and g.t; x/ in C.I;H

.M //; k >

n C 1;

D A



, the initial-value problem

(1.51)

.t; x/ @

u C g.t; x/; u.0/ D f 2 H

.M /;

has a unique solution in C.I;H

.M //.

In some approaches to quasi-linear equations, this result is established ﬁrst and

used as a tool to solve (1.1), via an iteration of the form

(1.52)

C1

.t; x; u



C1

C g.t; x; u



/; u

C1

.0/ D f;

beginning, say, with u

.t/ D f . Then one’s task is to show that fu



g converges,

at least on some interval I about t D 0, to a solution to (1.1). For details on this

approach, see [Mj1]; see also Exercises 3–6 below.

The approach used to prove Theorem 1.2 has connections with numerical

methods used to ﬁnd approximate solutions to (1.1). The approximation (1.4)

422 16. Nonlinear Hyperbolic Equations

is a special case of what are sometimes called Galerkin methods. Estimates

established above, particularly Proposition 1.3, thus provide justiﬁcation for some

classes of Galerkin methods.

For nonlinear hyperbolic equations, short-time, smooth solutions might not

extend to solutions deﬁned and smooth for all t . We mention two simple examples

of equations whose classical solutions break down in ﬁnite time. First consider

(1.53)

D u

; u.0; x/ D 1:

The solution is

(1.54) u.t; x/ D

1  t

;

for t<1, which blows up as t % 1.

The second example is

(1.55) u

C uu

D 0; u.0; x/ D e

x

Writing the equation as

(1.56)



C u



u D 0;

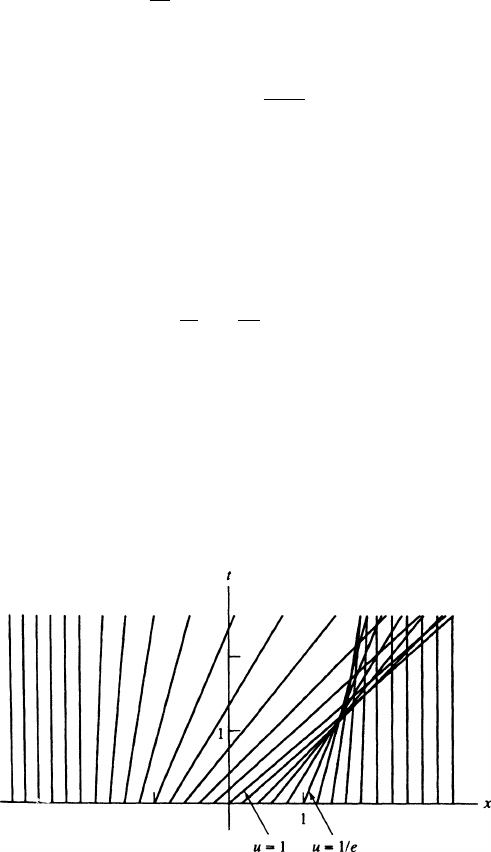
we see that u.t; x/ is constant on straight lines through .x; 0/, with slope

u.0; x/

1

,inthe.x; t/-plane, as illustrated in Fig. 1.1. The line through .0; 0/

has slope 1, and that through .1; 0/ has slope e; these lines must intersect, and by

that time the classical solution must break down. In this second example, u.t; x/

does not become unbounded, but it is clear that sup

.t; x/j does. As we will

discuss further in  7, this provides an example of the formation of a shock wave.

A detailed study of breakdown mechanisms is given in [Al].

FIGURE 1.1 Crossing Characteristics

Exercises 423

Exercises

1. Establish the results of this section when M is any compact Riemannian manifold,

by the following route. Let fX

W 1  j  N g be a ﬁnite collection of smooth

vector ﬁelds that span the tangent space T

M for each x. With J D .j

;:::;j

/,set

D X

X

;setjJ jDk.AlsosetX

;

D I; j;j D 0. Then use the square norm

kuk

jJ jk

Also, let J

D e

"

. To establish an analogue of (1.15), it will be useful to have

ŒX

 bounded in OPS

k1

1;0

.M /; for jJ jDk; " 2 .0; 1:

2. Consider a completely nonlinear system

(1.57)

D F.t;x;u; r

u/; u.0/ D f:

Suppose u takesvaluesinR

. Form a ﬁrst-order system for .v

;:::;v

/ D

.u;@

u;:::;@

u/:

(1.58)

D F.t;x;v/;

`D1

F /.t; x; v/ @

C .@

F /.t; x; v/; 1  j  n;

with initial data

v.0/ D .f; @

f;:::;@

f/:

We say (1.57) is symmetric hyperbolic if each @

F is a symmetric K  K matrix.

Apply methods of this section to (1.58), and then show that (1.57) has a unique

solution u 2 C.I;H

.M //,givenf 2 H

.M /; k > n=2 C 2.

Exercises 3–6 sketch how one can use a slight extension of Proposition 1.7 to show

that the iterative method (1.52) yields u



converging for jtj smalltoasolutiontothe

quasi-linear PDE (1.1). Assume f 2 H

.M /; k > n=2 C 1.

3. Extend Proposition 1.7,bytakingf 2 H

,andg 2 C.I;H

/,for` 2 Œ0; k (while

keeping A

2 C.I;H

/ and k>n=2C 1), and obtaining u 2 C.I;H

4. Granted Proposition 1.7, show that fu



g is bounded in C.I;H

.M //, after possibly

shrinking I .(Hint: Produce an estimate of the form

C1

.t/k



kf k



./ d

exp





.s/ ds



;

where '



.s/ D '.ku



.s/k

/ and



.s/ D .ku



.s/k

/. Then apply Gronwall’s

inequality.)

5. Derive an estimate of the form

C1

.t/  u



.t/k

k1

 Ajtj sup

s2Œ0;t



.s/  u

1

.s/k

k1

;

424 16. Nonlinear Hyperbolic Equations

for t 2 I , and deduce that fu



g is Cauchy in C.I;H

k1

.M //, after possibly further

shrinking I .(Hint: With w D u

C1

 u



, look at a linear hyperbolic equation for w

and apply the extension of Proposition 1.7 to it, with ` D k  1:)

6. Deduce that fu



g has a limit u 2 C.I;H

k1

.M // \ L

.I; H

.M //, solving (1.1).

7. Suppose u

and u

are sufﬁciently smooth solutions to (1.1), with initial data

.0/ D f

. Assume (1.1) is symmetric hyperbolic. Produce a linear, symmetric

hyperbolic equation satisﬁed by u

 u

.Iff

D f

on an open set O  M , deduce

that u

D u

on a certain subset of R  M , thus obtaining a ﬁnite propagation

speed result, as a consequence of the ﬁnite propagation speed for solutions to linear

hyperbolic systems, established via (5.26)–(5.34) of Chap. 6.

8. Obtain a smooth solution to (1.1) on a neighborhood of f0gM in R  M when

f 2 C

.M / and M is any open subset of R

.(Hint:Togetasolutionto(1.1)

on a neighborhood of .0; x

/, identify some neighborhood of x

in M with an open

set in T

and modify (1.1) to a PDE for functions on R  T

. Make use of ﬁnite

propagation speed to solve the problem.)

9. Let T



be the largest positive number such that (1.55) has a smooth solution for

0  t<T



. Show that, in this example,

ku.t/k

1=3

.R/

 K<1; for 0  t<T



(Hint:Fors D T



 t % 0, consider similarities of the graph of x 7! u.t; x/ D y

with the graph of x Dy

 sy:)

10. Show that the rays in Fig.1.1 are given by

ˆ.x; t/ D



x C te

x



;

and deduce that T



in Exercise 9 is given by



11. Consider a semilinear, hyperbolic system

(1.59)

D Lu C g.u/; u.0/ D f:

Paralleling the results of Proposition 1.5, show that solutions in the space



I;H

.M /



, k>n=2, persist as long as one has a bound

(1.60) ku.t/k

.M /

 K<1;t2 I:

In Exercises 12–14, we consider the semilinear system (1.59), under the following

hypothesis:

(1.61) g.0/ D 0; jg

.u/jC:

For simplicity, take M D T

12. Let u

be a solution to an approximating equation, of the form

(1.62)

D J

C J

g.J

/; u

.0/ D f:

2. Symmetrizable hyperbolic systems 425

Show that

 C ku

;

kru

 C kru

Deduce that, for any ">0,(1.62) has a solution, deﬁned for all t 2 R, and, for any

compact I  R,wehave

bounded in L



I;H

.M /



\ Lip



I;L

.M /



13. Deduce that, passing to a subsequence u

, we have a limit point u 2 L

loc

.R;H

.M //

\ Lip

loc

.R;L

.M //, such that

! u in C



I;L

.M /



in norm, for all compact I  R; hence g.J

/ ! g.u/ in C



R;L

.M /



,andu

solves (1.59). Examine the issue of uniqueness.

Remark: This result appears in [JMR]. The proof there uses the iterative method (1.52).

14. If dim M D 1, combine the results of Exercises 11 and 13 to produce a global smooth

solution to (1.59), under the hypothesis (1.61), given f 2 C

.M / and g smooth.

Remark:IfdimM is large, the global smoothness of u is open. For some results, see

[BW].

2. Symmetrizable hyperbolic systems

The results of the previous section extend to the case

(2.1) A

.t; x; u/

j D1

.t; x; u/@

u C g.t; x; u/; u.0/ D f;

where, as in (1.3), all A

are symmetric, and furthermore

(2.2) A

.t; x; u/  cI > 0:

We have the following:

Proposition 2.1. Given f 2 H

.M /; k > n=2C1, the existence and uniqueness

results of  1 continue to hold for (2.1).

We obtain the solution u to (2.1) as a limit of solutions u

(2.3) A

.t;x;J

D J

C g

; u

.0/ D f;

where L

and g

are as in (1.5)–(1.6). We need to parallel the estimates of  1,

particularly (1.8)–(1.15). The key is to replace the L

-inner products by

426 16. Nonlinear Hyperbolic Equations

(2.4)



w; A

.t/w



.t/ D A

.t;x;J

which by hypothesis (2.2) will deﬁne equivalent L

norms. We have

(2.5)



.t/D



D 2



.@u

=@t/; A

.t/D





.t/D



Here and below, the L

-inner product is understood. The ﬁrst term on the right

side of (2.5) can be written as

(2.6) 2





C 2



ŒD

@



in the ﬁrst of these terms, we replace A

.@u

=@t/ by the right side of (2.3), and

estimate the resulting expression by the same method as was applied to the right

side of (1.8)in 1. The commutator ŒD

 is amenable to a Moser-type es-

timate parallel to (1.12); then substitute for @u

=@t; A

1

times the right side of

(2.3), and the last term in (2.6) is easily estimated. It remains to treat the last term

in (2.5). We have

(2.7) A

.t/ D

.t;x;J

.t; x//;

hence

(2.8) kA

.t/k

 C



.t/k

; kJ

.t/k



Of course, k@u

=@tk

can be estimated by ku

.t/k

, due to (2.3). Conse-

quently, we obtain an estimate parallel to (1.15), namely

(2.9)

j˛jk

/  C



.t/k



1 CkJ

.t/k



From here, the rest of the parallel with  1 is clear.

The class of systems (2.1), with all A

D A



and A

 cI > 0, is an extension

of the class of symmetric hyperbolic systems. We call a system

(2.10)

j D1

.t; x; u/@

u C g.t; x; u/; u.0/ D f;

a symmetrizable hyperbolic system provided there exist A

.t; x; u/, positive-

deﬁnite, such that A

.t; x; u/B

.t; x; u/ D A

.t; x; u/ are all symmetric. Then

applying A

.t; x; u/ to (2.10) yields an equation of the form (2.1) (with different g

and f ), so the existence and uniqueness results of  1 hold. The factor A

.t; x; u/

is called a symmetrizer.

2. Symmetrizable hyperbolic systems 427

An important example of such a situation is provided by the equations of

compressible ﬂuid ﬂow:

(2.11)

v C



grad p D 0;

@

 C  div v D 0:

Here v is the velocity ﬁeld of a ﬂuid of density  D .t; x/. We consider the

model in which p is assumed to be a function of . In this situation one says the

ﬂow is isentropic. A particular example is

(2.12) p./ D A



;

with A>0;1< <2;forair, D 1:4 is a good approximation. One calls

(2.12)anequation of state. Further discussion of how (2.11) arises will be given

in  5.

The system (2.11) is not a symmetric hyperbolic system as it stands. However,

one can multiply the two equations by b./ D =p

./ and 

1

, respectively,

obtaining

(2.13)



b./ 0

0

1







D



b./r

grad

div 

1







Now (2.13) is a symmetric hyperbolic system of the form (2.1). Recall that

(2.14) .div v; f /

D.v; grad f/

Thus the results of  1 apply to the equation (2.11) for compressible ﬂuid ﬂow, as

long as  is bounded away from zero.

Another popular form of the equations for compressible ﬂuid ﬂow is obtained

by rewriting (2.11) as a system for .p; v/;using(2.12), one has

(2.15)

p C .p/ div v D 0;

v C .p/ grad p D 0;

where .p/ D 1=.p/ D .A=p/

1=

. This is also symmetrizable. Multiplying

these two equations by .p/

1

and .p/, respectively, we can rewrite the sys-

tem as



.p/

1

0.p/







D



.p/

1

div

grad .p/r





See Exercises 3–4 below for another approach to symmetrizing (2.11).