Sundararaja D. The Discrete Fourier Transform. Theory, Algorithms and Applications
Подождите немного. Документ загружается.

264
The Continuous-Time Fourier Series
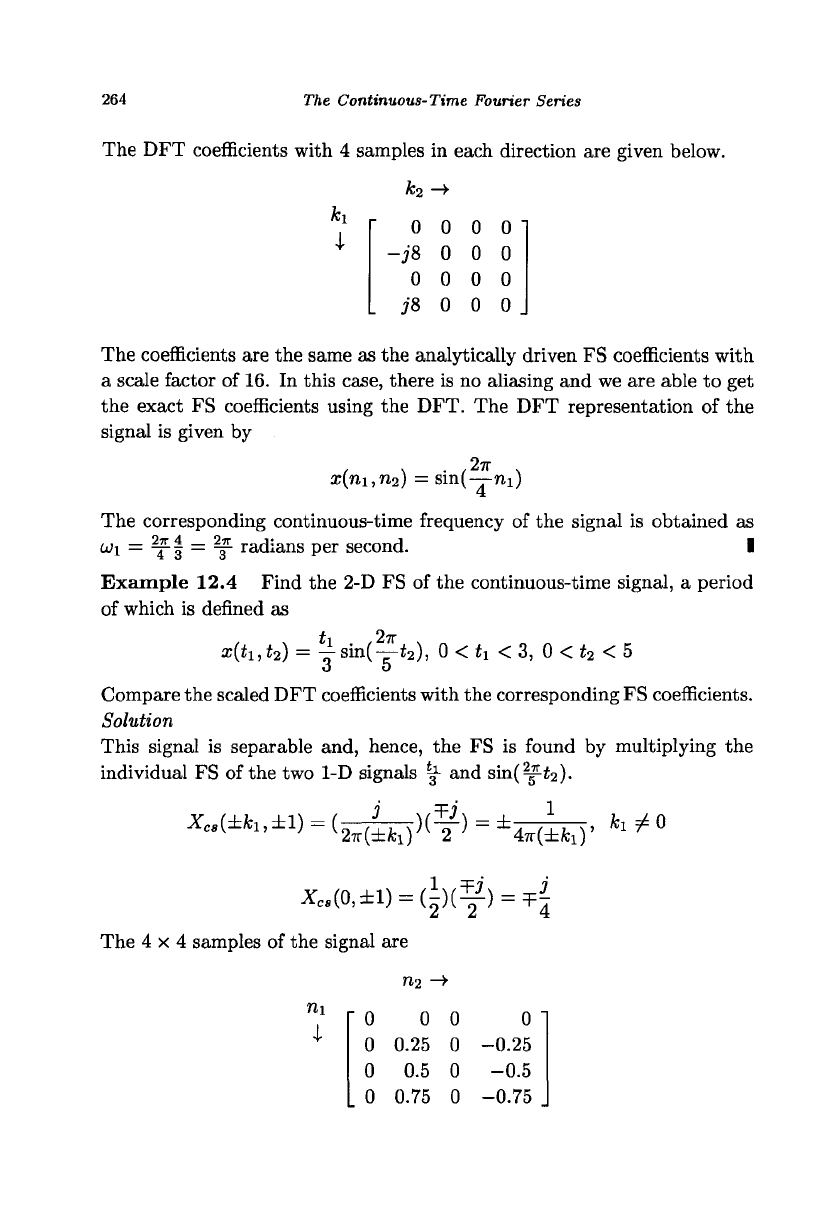
The DFT coefficients with 4 samples in each direction are given below.
k
2
->•
0 0 0 0
-38 0 0 0
0 0 0 0
j8 0 0 0
The coefficients are the same as the analytically driven FS coefficients with
a scale factor of 16. In this case, there is no aliasing and we are able to get
the exact FS coefficients using the DFT. The DFT representation of the
signal is given by
x(ni,n
2
) = sin(—ni)
The corresponding continuous-time frequency of the signal is obtained as
ui = ^| = Tp radians per second. I
Example 12.4 Find the 2-D FS of the continuous-time signal, a period
of which is defined as
x(t
u
t
2
) = ^-sin(-^
2
), 0 < h < 3, 0 < t
2
< 5
3 5
Compare the scaled DFT coefficients with the corresponding FS coefficients.
Solution
This signal is separable and, hence, the FS is found by multiplying the
individual FS of the two 1-D signals ^ and sin(^-t
2
).
1
.Y
c
.(±ki,±l) = (
I )(^-) = ±-
27r(±fci)
A
2 ' 47r(±*i)'
fei^O
*
c
.(0,±l) = (i)(^) = TJ
The 4x4 samples of the signal are
"1 r
I
n
2
->
0 0 0 0
0 0.25 0 -0.25
0 0.5 0 -0.5
0 0.75 0 -0.75
The 2-D Continuous- Time Fourier Series
265
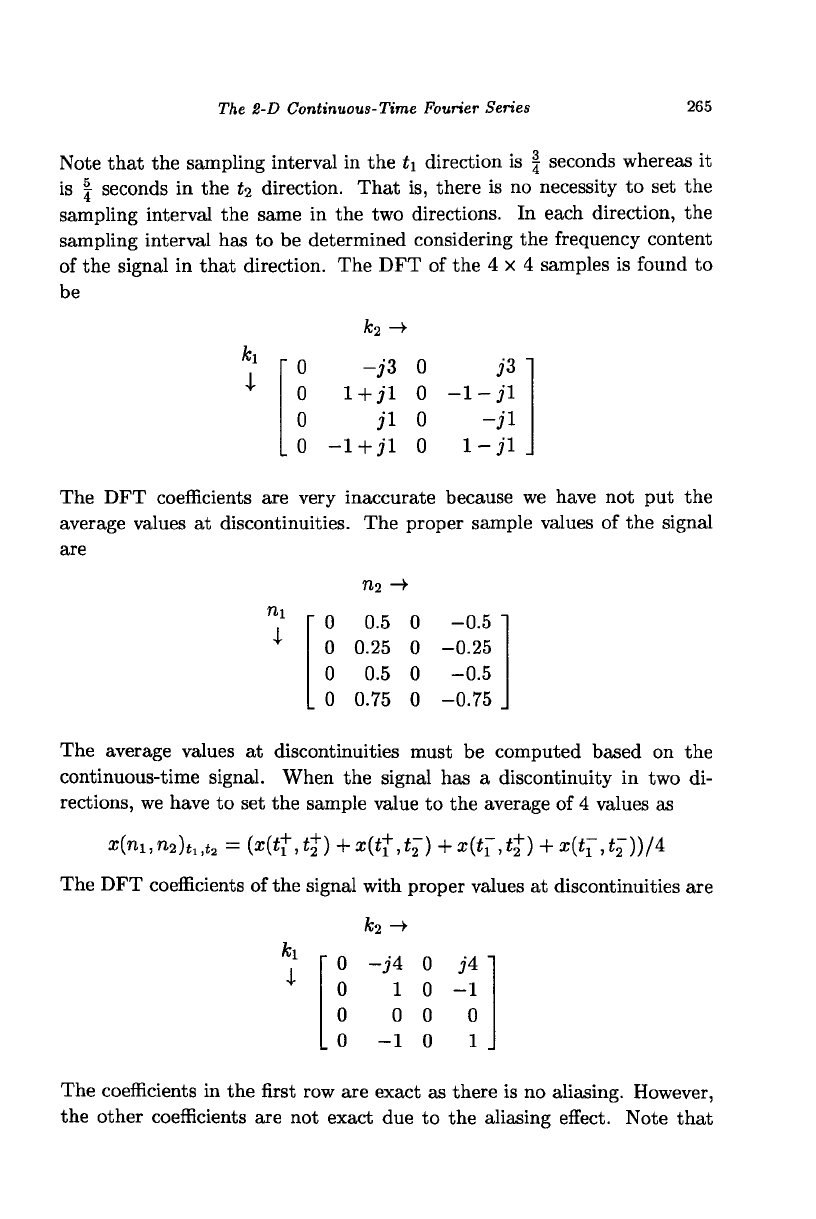
Note that the sampling interval in the t\ direction is § seconds whereas it
is | seconds in the ti direction. That is, there is no necessity to set the
sampling interval the same in the two directions. In each direction, the
sampling interval has to be determined considering the frequency content
of the signal in that direction. The DFT of the 4 x 4 samples is found to
be
k
2
->•
*i
4-
0
0
0
0
-j3 0
l
+
jl 0
jl 0
-l+jl 0
J'3
-1-jl
-n
i-ji
The DFT coefficients are very inaccurate because we have not put the
average values at discontinuities. The proper sample values of the signal
are
ri2 ->•
Til
1
" 0
0
0
. 0
0.5
0.25
0.5
0.75
0
0
0
0
-0.5
-0.25
-0.5
-0.75
The average values at discontinuities must be computed based on the
continuous-time signal. When the signal has a discontinuity in two di-
rections, we have to set the sample value to the average of 4 values as
x(ni,m)t
llta
= (x(tt,4) + x(tt,1£)+x(ti,4) + x{ti,t2))/4:
The DFT coefficients of the signal with proper values at discontinuities are
k
2
-t
fci
I
0
0
0
0
-j4 0
1 0
0 0
-1 0
jA
-1
0
1
The coefficients in the first row are exact as there is no aliasing. However,
the other coefficients are not exact due to the aliasing effect. Note that
266
The Continuous-Time Fourier Series
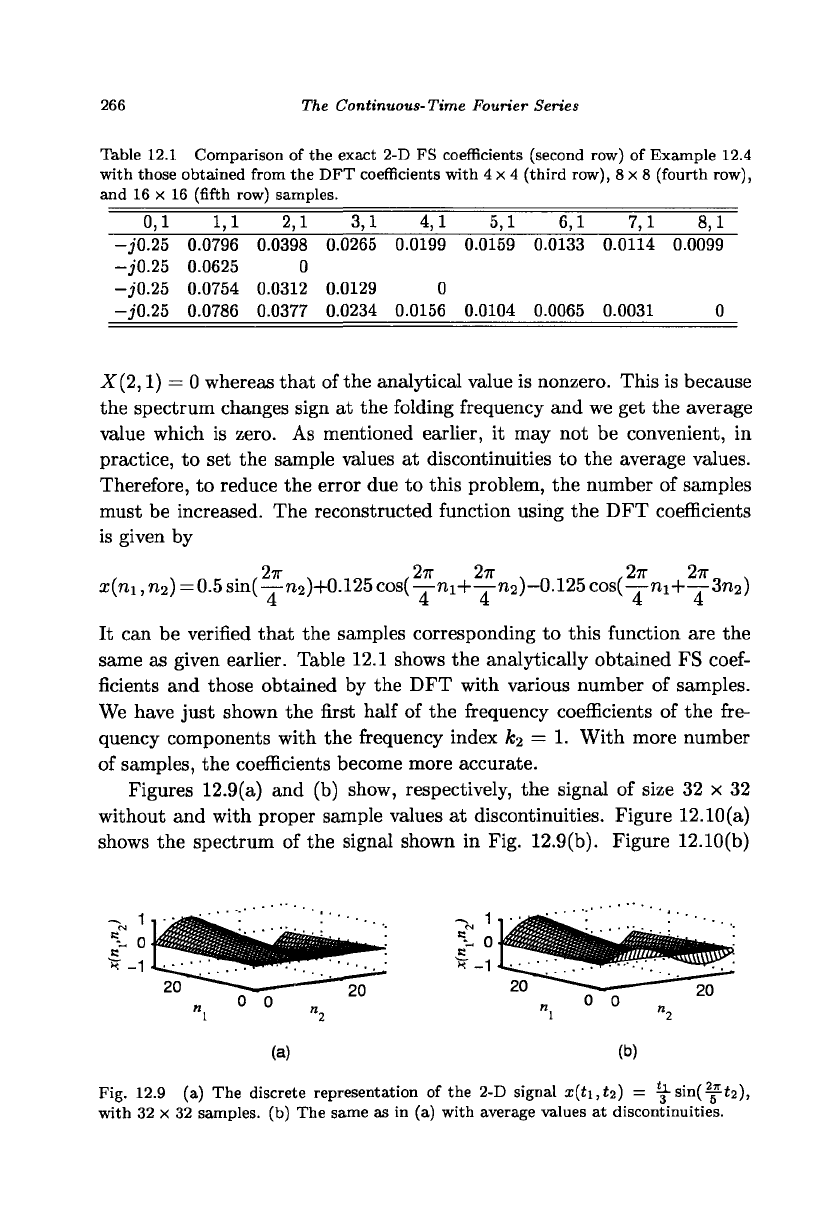
Table 12.1 Comparison of the exact 2-D FS coefficients (second row) of Example 12.4
with those obtained from the DFT coefficients with 4x4 (third row), 8x8 (fourth row),
and 16
X
16 (fifth row) samples.
0,1
1,1
2,1
3,1 4,1
5,1
6,1
7,1
8,1
-jO.25 0.0796
-jO.25 0.0625
-jO.25 0.0754
-jO.25 0.0786
0.0398
0
0.0312
0.0377
0.0265 0.0199 0.0159 0.0133 0.0114 0.0099
0.0129
0.0234
0
0.0156 0.0104 0.0065 0.0031
X(2,1) = 0 whereas that of the analytical value is nonzero. This is because
the spectrum changes sign at the folding frequency and we get the average
value which is zero. As mentioned earlier, it may not be convenient, in
practice, to set the sample values at discontinuities to the average values.
Therefore, to reduce the error due to this problem, the number of samples
must be increased. The reconstructed function using the DFT coefficients
is given by
2w 2TT 2W 2TT 2TT
a;(ni,n
2
)=0.5sin(-—n2)+0.125cos(—-ni+—-n2)-0.125cos(—-niH—r^
n
2)
v
' 4 4 4 4 4
It can be verified that the samples corresponding to this function are the
same as given earlier. Table 12.1 shows the analytically obtained FS
coef-
ficients and those obtained by the DFT with various number of samples.
We have just shown the first half of the frequency coefficients of the fre-
quency components with the frequency index fc
2
= 1. With more number
of samples, the coefficients become more accurate.
Figures 12.9(a) and (b) show, respectively, the signal of size 32 x 32
without and with proper sample values at discontinuities. Figure 12.10(a)
shows the spectrum of the signal shown in Fig. 12.9(b). Figure 12.10(b)
(a) (b)
Fig. 12.9 (a) The discrete representation of the 2-D signal x{t\,t2) = ^-sin(^
L
t2),
with 32 x 32 samples, (b) The same as in (a) with average values at discontinuities.
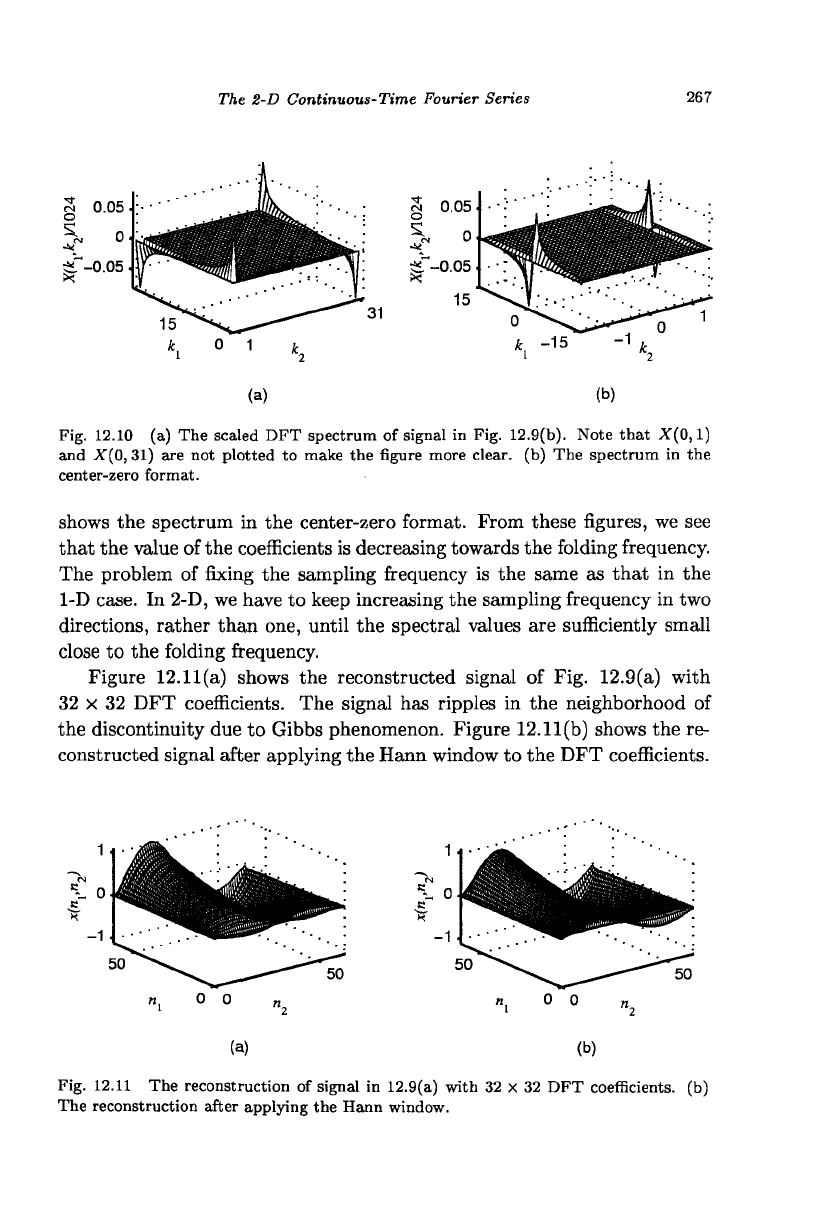
The 2-D Continuous-Time Fourier Series
267
(a)
(b)
Fig. 12.10 (a) The scaled DFT spectrum of signal in Fig. 12.9(b). Note that X(0,1)
and X(0,31) are not plotted to make the figure more clear, (b) The spectrum in the
center-zero format.
shows the spectrum in the center-zero format. From these figures, we see
that the value of the coefficients is decreasing towards the folding frequency.
The problem of fixing the sampling frequency is the same as that in the
1-D case. In 2-D, we have to keep increasing the sampling frequency in two
directions, rather than one, until the spectral values are sufficiently small
close to the folding frequency.
Figure 12.11(a) shows the reconstructed signal of Fig. 12.9(a) with
32 x 32 DFT coefficients. The signal has ripples in the neighborhood of
the discontinuity due to Gibbs phenomenon. Figure 12.11(b) shows the re-
constructed signal after applying the Hann window to the DFT coefficients.
(a)
(b)
Fig. 12.11 The reconstruction of signal in 12.9(a) with 32 x 32 DFT coefficients, (b)
The reconstruction after applying the Hann window.
268 The Continuous-Time Fourier Series
As expected, the ripples are reduced at the cost of reducing the rise time.
Note that, for 2-D signals, we apply the 1-D window in each direction. For
this example, we applied the window only in the ki direction as there is no
truncation of the spectrum in the other direction. I
12.3 Summary
• In this chapter, we studied the trigonometric and complex expo-
nential forms of the FS. The failure of the FS to provide uniform
convergence in the vicinity of a discontinuity was discussed. It was
shown how the FS coefficients are approximated by the DFT coeffi-
cients.
The errors arising in the resulting procedure were analyzed.
• Fourier analysis is the representation of a signal in terms of sinu-
soids.
As the function being the same, the DFT and the FS are
closely related and the latter can be approximated to a desired ac-
curacy by the DFT coefficients with proper choice of the number
of samples taken over an integral number of periods of the time-
domain continuous-time signal.
References
(1) Guillemin, E. A. (1952) The Mathematics of Circuit Analysis, John
Wiley, New York.
(2) Cadzow, J. A. and Van Landingham, H. F. (1985) Signals, Systems,
and Transforms, Prentice-Hall, New Jersey.
Exercises
12.1 Find the FS representation, x(t) =
cos
3
1.
12.2 Find the FS representation, x(t) = sin
4
t.
12.3 Square wave with even symmetry
Deduce the FS representation from the result of Example 12.2.
A f <t<T

Exercises
269
* 12.4 Sawtooth wave
Find the FS representation analytically.
x{t) = { ±t 0<t<T
Compute the DFT with 4, 8, and, 16 samples and compare the scaled DFT
and the FS coefficients with A = 1 and T = 1.
12.5 Triangular wave
Find the FS representation analytically.
M
- J 7T* 0 < i < f
XW
~ \ 2A(1 - f) \ < t < T
Compute the DFT with 4, 8, and, 16 samples and compare the scaled DFT
and the FS coefficients with A
—
1 and T = 1.
12.6 Half-wave rectified sine wave
Find the FS representation analytically.
*«>
=
{o
Asin(^i) 0<i<f
J<t<T
Compute the DFT with 4, 8, and, 16 samples and compare the scaled DFT
and the FS coefficients with A = 1 and T = 1.
12.7 Using the results of Exercise 12.6, deduce the FS representation of
half-wave rectified cosine wave.
x(t)
= {
Acos(^t) 0<i<^and^<t<T
0 ?<*<f
12.8 Using the results of Exercise 12.6, deduce the FS representation of
full-wave rectified sine wave
x(t) = { A\ sin(^i)| 0 < t < T
12.9 Using the results of Exercise 12.7, deduce the FS representation of
full-wave rectified cosine wave
x(t) = {
A\cos{^-t)\
0<t<T
270
The Continuous-Time Fourier Series
12.10 Square curve
Find the FS representation analytically.
x(t) = { t
2
0<t<T
Compute the DFT with 4, 8, and, 16 samples and compare the scaled DFT
and the FS coefficients with T = 1.
* 12.11 Even symmetric pulse train
Find the FS representation analytically.
A 0<t<w
x{t) = { 0 w <t<T-w
A T-w<t<T
Compute the DFT with 4, 8, and, 16 samples and compare the scaled DFT
and the FS coefficients with A
—
1, T = 1, and w — |.
12.12 Pulse train
Find the FS representation analytically.
x(t) = |
A 0<t<2w
0 2w < t < T
Compute the DFT with 4, 8, and, 16 samples and compare the scaled DFT
and the FS coefficients with A = 1,T — 1, and w = |.
12.13 Half inverted cosine wave
Find the FS representation analytically.
/jt
, f -Acosi^t) 0<t < f
X(i) =
l0 f<t<T
Compute the DFT with 4, 8, and, 16 samples and compare the scaled DFT
and the FS coefficients with A = 1 and T = 1.
12.14 Using the results of Exercise
12.13,
deduce the FS representation of
two inverted half cosine waves
<t)
=
{
-AcosC^-t) 0<<<£
Acos(^f) %<t<T

Exercises 271
12.15 Find the complex FS coefficients.
27T 7T 27T
/(*i,*
2
) = cos(—h + - +
—2*
2
),
0 < h < 7, 0 < h < 5
7 3 5
12.16 Find the FS representation analytically.
f(t
u
t
2
) = 2tit2, 0 < *i < 3, 0 < *
2
< 4
Compare the first 4x4 scaled DFT coefficients computed using 32 x 32
samples with the FS coefficients.
12.17 Find the FS representation analytically.
f(h,t
2
) =
*i*2,
0 < *i < 2, 0 < *
2
< 3
Compare the first 4x4 scaled DFT coefficients computed using 32 x 32
samples with the FS coefficients.
* 12.18 Find the FS representation analytically.
/(*i,*
2
) = *i+*
2
, 0<*i <3, 0<*
2
<2
Compare the scaled DFT coefficients using 4 x 4, 8 x 8, 16 x 16 samples
with the FS coefficients.

Chapter 13
The Continuous-Time Fourier
Transform
The FT is the frequency-domain representation of a continuous-time ape-
riodic signal in terms of sinusoids with continuum of frequencies. Both the
DFT and the FT do the same function, that is providing the sinusoidal rep-
resentation of signals. However, the DFT analyzes a periodic version of a
finite discrete signal whereas the FT analyzes an aperiodic continuous-time
signal. Therefore, the essential differences to be considered in approxi-
mating the samples of the FT by those of the DFT are: (i) the integral
evaluated in the case of the FT is approximated by a numerical integration
procedure, (ii) the limit of the integration in DFT is finite whereas it is
infinity in the case of FT, and (iii) a finite set of coefficients is used in the
DFT and coefficients at continuum of frequencies are used in the case of
the FT.
In Sec. 13.1, we start with the fact that FT is a limiting case of the FS,
present the relation between the DFT and the FT, and conclude with an
example of approximating the samples of the FT by those of the DFT. In
Sec.
13.2, the approximation of the 2-D FT by the DFT is described.
13.1 The 1-D Continuous-Time Fourier Transform
The FT as a limiting case of the FS
The FT is the same as the FS with the period of the waveform approach-
ing infinity. Consider the FS of the pulse train shown in Fig. 13.1 with
increasing periods. Keeping the pulse width the same but doubling the
period, the magnitudes are reduced by a factor of two and there are double
273
