Stacey F.D., Davis P.M. Physics of the Earth

Подождите немного. Документ загружается.

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C18.3D

–

307

– [294–313] 13.3.2008 11:43AM

The physics of  is discussed in Section 19.3. Since

it remains finite at V !0, by Eq. (18.52) q

¼0.

This is true however the infinite pressure condi-

tion is approached so that the corresponding adia-

batic derivative, q

, also vanishes and therefore,

by Eq. (E.8), C

!0atV !0. With the conditions



> 0, q

¼0, C

¼0, Eq. (E.4) gives

> 1 þ 

: (18:53)

Now we can appeal to a relationship between 

and K

that is discussed in Section 19.3. An early

theory by Slater (1939) made the simplifying

assumption that for all elastic moduli, X,

dlnX/dV had the same value (Poisson’s ratio, ,

independent of pressure), in which case

Eq. (19.32) becomes



¼ K

=2  1=6: (18:54)

This is known as Slater’s gamma and the sub-

script

distinguishes it from other expressions

for . Under normal conditions  increases with

pressure and 

overestimates ,butatP !1

Slater’s assumption becomes valid (Section 18.8)

and therefore



¼ K

=2  1=6: (18:55)

Although Eq. (18.54) is unsatisfactory, Eq. (18.55)

is secure for reasons considered below. With

Eq. (18.53) this means

> 5=3; 

> 2=3: (18:56)

This is the thermodynamic bound on K

referred to in Sections 18.3 and 18.4. The coupling

to 

has proved somewhat contentious, so we

examine how rigorous and general it is. Equa-

tion (18.53) is inescapable, being thermodynami-

cally rigorous and applicable to all materials.

The remaining step is an appeal to Eq. (18.55).

When Eq. (18.44), which is an algebraic identity

if K

remains positive, is applied to Eq. (19.39),

one of the standard relationships for , it yields

Eq. (18.55) independently of any assumption about

the parameter f.Equationsfor of the acoustic

type (Eq. (19.33)), derived from Gr ¨uneisen’s mode

definition of  (Eq. (19.18)), also reduce to

Eq. (18.55), if  and K are related by an equation

such as Eq. (18.67), and this is another way of

invoking Slater’s assumption, used above, that

Poisson’s ratio is independent of pressure. Note

that these bounds on K

and g

refer to these

quantities as equation of state parameters of ordi-

nary materials in the observed pressure range and

not to extreme pressure states that could be

reached only via dramatic phase transitions.

There is scope for further constraints of this

kind. With Eq. (18.55), Eq. (E.4) gives



¼½ð1=Þð@ ln K

=@TÞ



P!1

¼ðK

 5=3Þ=2; (18:57)

and Eq. (E.17) reduces to

½ð1=Þð@K

=@TÞ



P!1

¼ðK

 5=3ÞðK

þ 5=3Þ=4:

(18:58)

In principle it appears that these equations sug-

gest the possibility of a more restrictive version

of Keane’s rule (Eq. (18.38)) but this remains to be

proved.

18.7 Finite strain of a composite

material

Section 10.4 introduces the problem of elasticity

of a granular material with constituents having

different elasticities. For small pressure incre-

ments the mismatch of elasticities causes grain

boundary stresses, so that the applied pressure is

partly supported by deviatoric stresses. The

result is a composite bulk modulus higher than

if all the grains were individually compressed

hydrostatically. In this situation the effective

modulus is approximated by K

VRH

(Eq. (10.13)).

If the deviatoric stresses relax (by grain deforma-

tion), because they are very prolonged or are too

great to be supported by the material strength,

then all grains are subjected to the same hydro-

static pressure and the smaller Reuss modulus,

(Eq. (10.12)) applies. We need to consider the

difference between K

and K

VRH

in the Earth

because seismic waves impose small stresses,

and use the unrelaxed modulus, K

VRH

, but in a

homogeneous layer with a wide pressure range

the variation in density with depth is descri-

bed by the relaxed modulus, K

. Use of the

Williamson–Adams equation (Section 17.5)

18.7 FINITE STRAIN OF A COMPOSITE MATERIAL 307

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C18.3D

–

308

– [294–313] 13.3.2008 11:43AM

usually relates density variation to the seismi-

cally observed modulus and neglects the differ-

ence. We examine the significance of this to the

interpretation of lower mantle properties. It has

no relevance to the core, which is not granular.

It should be noted that we consider here proper-

ties at pressures sufficient to ensure complete

closure of pores. The pressure dependence of

rock properties arising from porosity at low pres-

sure is a separate question.

Table 18.1 gives results of calculations for a

mineral mix that approximates the lower man-

tle. It uses 290 K laboratory observations of K and

for silicate perovskite, subscripted pv, and

magnesiowustite, subscripted mw. We see that

at zero pressure K

and K

VRH

differ by almost 2%.

Properties of the same mix at pressures corre-

sponding to the bottom and top of the lower

mantle are calculated by applying the reciprocal

K-primed equation (Eqs. (18.45) to (18.47)) assum-

ing that K

¼2.4 for each mineral separately.

This is the best estimate of the lower mantle

value. While we have no assurance that it applies

to the individual minerals, the error in assuming

it cannot be significant. As seen in the table,

the difference between K

and K

VRH

decreases

strongly with pressure, becoming less than 0.2%

at the bottom of the mantle.

In Earth model data, the difference between

and K

VRH

is observationally indistinguishable

from a temperature gradient departing from an

adiabat. As in Section 17.5, we can write

d=dP ¼ð@=@PÞ

þð@=@TÞ

½dT=dP ð@T=@PÞ



(18:59)

with

dT=dP ¼ð1 þ Þð@T=@PÞ

¼ð1 þ ÞT=K

; (18:60)

where (1 þ) is the factor by which the tem-

perature gradient differs from the adiabat.

With  ¼(1/)(@/@T)

, Eq. (18.59) becomes

d=dP ¼ð=K

Þ½1  T: (18:61)

Values of (T) over the depth of the lower mantle

are 0.0507 to 0.0361, so the K differences in

Table 18.1 would appear equivalent to  ¼0.17

to 0.05. Over the depth of the lower mantle this

integrates to a temperature deficit of 90 K relative

to the adiabat. It is doubtfully significant, but the

Earth model PREM is consistent with a temper-

ature excess of about 100 K over this range, so on

this basis the true excess is estimated to be 200 K.

In the interpretation of lower mantle proper-

ties the difference between K

and K

VRH

is insuf-

ficient to be sure that it is seen, but the

difference between their pressure derivatives,

and K

VRH

, is more significant. When a mixture

of minerals with different bulk moduli is com-

pressed the less compressible constituents

become increasing volume fractions of the

whole. Thus their higher bulk moduli contribute

increasingly to the modulus of the composite

and there is a contribution to K

¼dK/dP arising

from the varying volume fractions, in addition to

the effect of K

for each constituent. In the case of

we can differentiate Eq. (10.12) with respect to

P, noting that dV

/dP ¼V

, etc., so that

ðK

þ 1Þ=K

¼ðV

=VÞðK

þ 1Þ=K

þðV

=VÞðK

þ 1Þ=K

þ; (18:62)

Table 18.1 Relaxed and unrelaxed bulk

moduli, K

, K

VRH

and their pressure

derivatives, for a mineral mix simulating

the lower mantle, with 80% perovskite and

20% magnesiowustite by volume at P ¼0.

Each mineral is assumed to obey Eq. (18.24)

with K

and K

as listed and K

¼2.4. Values

are calculated for a 290 K isotherm at P ¼0

and at pressures corresponding to the top

and bottom of the lower mantle

P (GPa) 0 23.8 3 135.75

264 350.14 704.89

162 251.65 605.69

(/

)

1 1.0811 1.3456

(/

)

1 1.1235 1.4781

/V 0.2 0.1939 0.1854

(GPa) 234.47 325.44 684.12

(GPa) 243.60 331.04 686.50

VRH

(GPa) 239.04 328. 24 685.31

(1 K

VRH

) 0.019 118 0.008 530 0.001 736

3.8 3.469 2.993

4.1 3.531 2.969

4.166 3.582 3.003

3.899 3.499 2.992

VRH

4.032 3.540 2.997

308 FINITE STRAIN AND HIGH-PRESSURE EQUATIONS OF STATE

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C18.3D

–

309

– [294–313] 13.3.2008 11:43AM

giving the values of K

in Table 18.1. It is notice-

able that K

is significantly higher than might be

expected intuitively from the K

values for the

individual minerals. In the case of K

we must

differentiate in the same way, allowing the vol-

ume fractions to vary, because we are interested

in the variation of K

over a wide pressure range.

This gives

¼ðV

=VÞK

þðV

=VÞK

þþK

 1;

(18:63)

and K

VRH

is the average of K

and K

As seen in Table 18.1, the K

bias is most

noticeable at P ¼0. Thus it is in the extrapolation

of the lower mantle equation of state to zero

pressure that greatest care is required in match-

ing the equation to properties of a plausible min-

eral mix. If the equation of state uses P() data

then K

and K

are required, but if seismological

values of K are fitted then the appropriate value

of K

is K

VRH

. The lower mantle equation of state

fit in Appendix F uses K data and the value of K

(4.2) is K

for extrapolation on the geotherm at a

temperature estimated to be 1700 K. With the

temperature dependence estimated by Stacey

and Davis (2004) this gives K

¼4.0 at 290 K, in

agreement with K

VRH

in Table 18.1.

18.8 Rigidity modulus at high

pressure

Unlike compression, pure shear deformation

causes no temperature rise. Adiabatic and isother-

mal values of rigidity modulus, , are identical

and there are no relationships for  correspond-

ing to Eqs. (E.1) or (18.1). From a theoretical per-

spective  is more difficult to deal with than is K

and a different approach is required. But in seis-

mology  is as well observed as is K,soweneeda

fundamental understanding of it to make full use

of the available data. The approach presented

here is based on what is termed second-order

elasticity theory. It is a conventional elasticity

theory in the sense that shear strains are treated

as infinitesimal (we do not have to consider finite

shear strains), but a finite hydrostatic compres-

sion is superimposed. Then elastic strain energy

must be calculated to second order in shear strain

to derive a valid expression for .

At low pressure, close to r ¼a in Fig. 18.4, the

potential function is parabolic, that is the depar-

ture of  from the minimum value is proportional

to (r a)

and K is determined by 

¼d

/dr

.But,

as Eq. (18.19) shows, the complete exp-

ression involves also 

and this vanishes only at

r ¼a, which is the zero pressure condition by

Eq. (18.18). The same principle applies to the cal-

culation of , but with a reversed sign for the 

term. To see how this arises, consider an atomic

close-packed structure, with planes of atoms

linked in arrays of equilateral triangles. The

bonds connecting three such atoms are illus-

trated in Fig. 18.6, with the bond length changes

caused by shear of the structure, displacing atom

CtoC

. The A–C

and B–C

bond lengths become

; r

ﬃﬃﬃ



 s



1=2

¼r



ð3=8Þs

þ;

(18:64)

where r

is the equilibrium bond length at arbi-

trary ambient pressure and not the zero pressure

length, a. It is essential to the calculation to

retain the s

term in this equation. The energy

of each bond can be written as a Taylor expan-

sion about r

ðrÞðr

Þ¼

ðr

Þðr  r



ðr

Þðr  r

þ;

(18:65)

FI G U R E 18.6 Changes in bond lengths between atoms

that are arranged in an equilateral triangle in an

unstrained crystal as a shear strain is imposed.

18.8 RIGIDITY MODULUS AT HIGH PRESSURE 309

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C18.3D

–

310

– [294–313] 13.3.2008 11:43AM

drawing attention to existence of the 

term in

the expression for bond energy. If P 6¼0, that is

r 6¼a, 

(r) does not vanish. The strain energy, E

of the bonds represented in Fig. 18.6, that is the

sum of the energies of the A–C

and B–C

bonds

less the A–C and B–C energies, is then given by

substitution of r

, r

for r in Eq. (18.65),

¼ðr

Þþðr

Þ2ðr

¼

ðr

Þðr

 r

Þþ

ðr

Þðr

 r



ðr

Þðr

 r



ðr

Þðr

 r



ðr

Þþ



ðr



: (18:66)

Since the shear strain energy is (1/2)s

, we see

that 

(and therefore P) appears in the expres-

sion for . But this should not be surprising

because it also appears in the expression for K

(Eq. (18.19)).

The 

term in Eq. (18.66) would be missing if

the s

term were omitted from Eq. (18.64). This is

why we refer to the analysis as second-order

elasticity theory. It is this 

term, and the corres-

ponding term in Eq. (18.19), that give the pres-

sure dependence to the ratio /K. The two terms

in Eq. (18.66) appear with the same sign, but in

Eq. (18.19) they have opposite signs. This means

that the pressure terms have opposite effects for

the two moduli, increasing K but decreasing 

and therefore the ratio / K. A calculation by

Falzone and Stacey (1980) for all strain orienta-

tions and bond directions in a face-centred cubic

crystal showed that this conclusion applies to all

cases. It is quite general. Thus, we can write both

K and  as linear combinations of 

and 

, with

the 

term proportional to P by Eq. (18.18) and

appearing with opposite signs in the two cases.

Elimination of 

from these equations gives a

linear relationship connecting , K and P,

 ¼AK þBP. Using the zero and infinite pressure

conditions to fix A and B, we have the most useful

form of the –K–P equation,



















: (18:67)

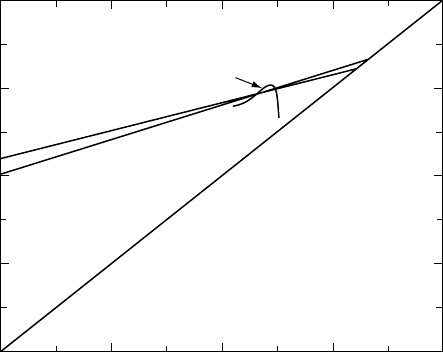
Equation (18.67) provides an explanation for

the high value of Poisson’s ratio, ,thatislow/K,

of the inner core. A common inference that the

inner core is close to a fluid state is quite wrong.

The value of n is just what is expected for solid

iron at a pressure of 300 þGPa. But the inner core

itself does not serve as a convincing test of the

validity of Eq. (18.67).  is not well observed for

the inner core and the gradient of /K vs P/K in the

PREM model is positive, not negative as Eq. (18.67)

requires (Fig. 18.7). The reason for this is consid-

ered below. But a mildly surprising check on

Eq. (18.67) is provided by lower mantle data,

which fit the equation extremely well. With

parentheses to indicate standard deviations of

the final digits, the PREM tabulation gives

=K ¼ 0:631ð1Þ0:899ð6ÞP=K: (18:68)

There are two interesting implications of this

result. (i) Equation (18.67) is derived with the

assumption that bond forces are central and it is

Lower mantle

(PREM)

Laboratory

iron

(Isaak and

Masuda, 1995)

0.2

0.1

0.3

0.4

0.6

Inner core

(PREM)

Infinite pressure

estimates

0.10

0.2 0.3

0.4

300 K

900

μ /K

FI G U R E 18.7 Lower mantle and core data fitted to

the –K–P equation (Eq. (18.67)).

310 FINITE STRAIN AND HIGH-PRESSURE EQUATIONS OF STATE

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C18.3D

–

311

– [294–313] 13.3.2008 11:43AM

not immediately obvious that it should apply

to the lower mantle, for which non-central

forces (intrinsic rigidity of angles between

bonds) must be significant. (ii) The standard devi-

ations of the coefficients in Eq. (18.68) are smaller

than would be expected from the accuracy of

PREM. Considering the second point first, a fit of

Eq. (18.67) to the lower mantle range of the ak135

model (see Fig. 17.9) gives even smaller standard

deviations but with coefficients differing from

those in Eq. (18.68) by 10 standard deviations.

The lower mantle is sufficiently heterogeneous

for different data sets and analyses to give notice-

ably different coefficients, but the variations in 

and K follow Eq. (18.67) extremely well for each of

them. Thus we can be confident of the validity of

the form of this equation, although the coeffi-

cients are not as well determined as Eq. (18.68)

suggests. So, what is the role of non-central forces,

which are not recognized in the derivation,

above, of Eq. (18.67)? The conclusion must be

that they are not independent of the central

forces but are different manifestations of the

same forces.

In the case of the inner core, with very low

/K, the central force assumption is more easily

justified than in the case of the mantle, so we

consider the implication of the misfit of the

PREM gradient in Fig. 18.7. Although we pre-

sume  to be diminished by anelasticity at the

very high homologous temperature, T/T

, of the

inner core, this does not explain the anomalous

gradient, d/dP, which we attribute to an artifact

of the Earth modelling. This gradient appears

anomalously high, but a more obviously anom-

alous feature of PREM is the very low dK/dP in the

inner core. At core pressures both solid and

liquid must have close-packed atomic structures,

disallowing a significant difference in dK/dP

for the inner and outer cores. The well-observed

(P-wave) modulus is  ¼K þ(4/3), which is not

anomalous, and the anomalies in both d/dP and

dK/dP can be adjusted to satisfactory agreement

with fundamental theory without a changing

d/dP. Both gradients can be adjusted in a com-

pensating way without affecting the radial pro-

file of  or the average values of  and K.

With this conclusion, Fig. 18.7 gives us crucial

information about the equation of state for the

core. We cannot extrapolate the core data past

P/K  0.35 (at which /K would be zero). Thus

(P/K)

< 0.35 and so, by Eq. (18.44), K

> 1/

0.35 ¼2.8. But (P/K)

must exceed the inner core

value, 0.25, so we know that K

< 4.0. Stacey

and Davis (2004) concluded that, for the core, K

is very close to 3.0. In Section 18.9 we consider

how this relates to the use of derivative equations

of state, especially Eq. (18.45), for the core.

Now we draw a conclusion from Eq. (18.67)

that is necessary to the infinite pressure extra-

polation of the Gr ¨uneisen parameter, as in

Eq. (18.55). Differentiating Eq. (18.67), we have

dlnð=KÞ

dlnP

¼

ð=KÞ













1  K



(18:69)

At P !1,(1K

P/K) !0 by Eq. (18.44), so /K

becomes independent of P. Since constant /K

was assumed in Slater’s derivation of Eq. (18.54),

the derivation becomes valid in the P !1 limit

and, even though Eq. (18.54) is unsatisfactory,

Eq. (18.55) is well founded and Eq. (18.56) follows.

18.9 A comment on application to

the Earth’s deep interior

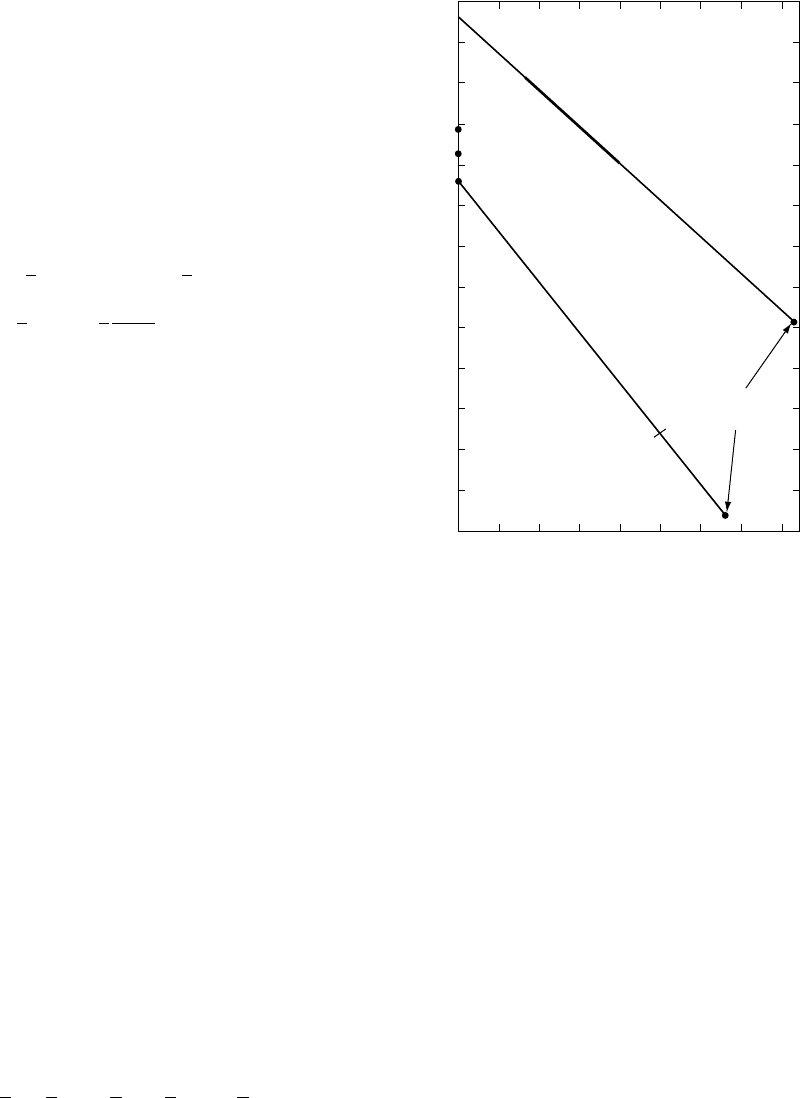
Figure 18.7 draws attention to the fact that, on

the P/K scale, the core is sufficiently close to the

infinite pressure limit to restrict K

to a limited

plausible range. The same conclusion is reached

in another way from Fig. 18.8. This presents the

PREM outer core data on a 1/K

vs P/K plot, show-

ing its approach to the infinite pressure limit

given by Eq. (18.44). Plotted in this way, the

core appears much closer to the P ¼1condition

than to P ¼0. The lines through the data are

alternative plots of Eq. (18.45), which also show

as straight lines on this figure and which inter-

sect Eq. (18.44) at P !1. Stacey and Davis (2004)

found that these alternatives bounded the plau-

sible range of core fits, but favoured the one with

1/K

 0.2, which gives K

¼3.0. That Figs. 18.7

and 18.8 are both consistent with the same value

of K

means that Eq. (18.45) is consistent with

Eq. (18.67). There are only three finite strain

equations that can accommodate the value of

18.9 APPLICATION TO THE EARTH’S DEEP INTERIOR 311

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C18.3D

–

312

– [294–313] 13.3.2008 11:43AM

fixed in this way, Eqs. (18.28), (18.39) and

(18.45). Although they appear very different

they are in fact quite similar in form, which

means that the choice between them is not

very important. Equation (18.45) and its integral

forms were used to calculate the lower mantle

and core properties listed in Appendix F, along-

side details of the PREM model that was used as

the starting point for the calculations.

A particular reason for care in selecting an

equation of state is that derivative properties, K

and even more so K

, differ greatly between

equations and they are needed for calculation

of thermal properties (Chapter 19). Realistic val-

ues of parameters, such as the Gr ¨uneisen param-

eter (Section 19.3), can be calculated only by

using a finite strain equation for which these

derivatives are satisfactory, and a glance at

Fig. 18.5 shows that deep in the lower mantle

the equations are very different, even though

they are all fits to the PREM data. The differences

are even greater in the core. However, it must be

recognized that none of these equations is more

than an empirical approximation. We have

no rigorous theory and can only make a choice

from the available equations on the basis of

tests and constraints, such as those outlined in

Sections 18.6 and 18.8, and convenience of use.

For geophysical applications there are advan-

tages to the use of P/K as the pressure parameter,

as in Eqs. (18.45) to (18.47), rather than P/K

which is more conventional. One is that P and K

are both tabulated in Earth models, so that P/K

can be treated as an observed quantity. Another

is that properties such as the Gr ¨uneisen para-

meter and the ratio /K reach finite limits as

P !1in much the same way as does P/K and so

relate more naturally to it. Stacey and Davis

(2004) give a more extended discussion of this

problem and used Eqs. (18.46) and (18.47) for the

equation of state fits to the lower mantle and

core listed in Appendix F.

Equation-of-state fits to the lower mantle

require a cautionary note. They implicitly

assume that the material is uniform, not only

in mineral structure but in phase structure,

including electronic structures of individual

minerals. An electronic phase transition in iron

ions at lower mantle pressures is now well rec-

ognized and it modifies physical properties,

including elasticity and acoustic velocities (Lin

et al., 2006). A theoretical overview of the prob-

lem is presented by Sturhahn et al. (2005), who

point out that, at the high temperatures of the

lower mantle, the transition is smeared out over

a wide pressure range, probably most of the

lower mantle. The transition involves a realign-

ment of the spins of the 3d electrons that are

responsible for the magnetic properties of iron.

At low pressure the available spins in each atom

0.4

0.3

0.2

0.1

0 0.1 0.40.30.2

/ K

1/K

′

PREM

Infinite pressure limit

FI G U R E 18.8 PREM data for the outer

core on a 1/K

vs P/K plot, showing two

fits to Eq. (18.45) and the infinite pressure

limit (Eq. (18.44)).

312 FINITE STRAIN AND HIGH-PRESSURE EQUATIONS OF STATE

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C18.3D

–

313

– [294–313] 13.3.2008 11:43AM

are aligned parallel, giving the atoms magnetic

moments. This is referred to as the high spin

state. At pressures above about 60 GPa, the spins

become paired to cancel the magnetic moments

in a low spin state. The effect has been studied in

greatest detail in (Mg,Fe)O, but also affects perov-

skite. Such a transition, in which increases in

density and bulk modulus are spread over a

wide pressure range, is difficult to distinguish

seismologically from the behaviour of a constant

phase structure with modified properties, such as

a high value of dK/dP. The full implications for the

lower mantle have yet to be worked out; this

problem is discussed also in Section 17.5.

We have emphasized that equations of state

are different for different materials, or different

phases of the same material. Thus the properties

of iron under inner core conditions do not

extrapolate to the properties of laboratory iron,

which has a different structure. The same limi-

tation applies to comparison of the outer core

with laboratory measurements on liquid iron.

The liquids have different structures, each

being a dislocated version of the solid with

which it is in equilibrium, although the change

in liquid structure near to a solid–solid phase

transition is smeared out over a range in pres-

sure (and varies with temperature). This was

demonstrated by Sanloup et al. (2000) for liquid

iron close to the solid – transition. Thus we

cannot use laboratory observations on liquid

iron to constrain the equation of state of the

outer core. The values of K

and K

are different.

But for the core we have a value for K

, obtained

as described in Section 18.8, and, as seen in

Fig. 18.8, this is nearer to the core value of K

than is K

. K

is a stronger constraint on the

equation of state of the core than is K

18.9 APPLICATION TO THE EARTH’S DEEP INTERIOR 313

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C19.3D

–

314

– [314–336] 13.3.2008 2:45PM

Thermal properties

19.1 Preamble

Convection is an underlying theme for this chap-

ter, the following four and Chapters 12 and 13.

With the probable exception of the inner core,

the entire Earth is convecting and this must

always have been so. Many of the topics that geo-

physicists study, including earthquakes, tectonics

and the geomagnetic field, are consequences of

convection, thermally driven in the mantle but at

least partly driven by a process of compositional

separation in the core. The Earth is a thermo-

dynamic engine, generating mechanical energy

in the process of transferring heat from the hot

interior to the surface. The sources of heat are

considered in Chapter 21 and the thermodynamic

efficiency and resulting mechanical power in

Chapter 22. The calculations rely on estimates of

the thermal properties considered in this chapter,

especially the Gr ¨uneisen parameter.

There must be a general correspondence

between local high temperatures and low seis-

mic wave speeds in the mantle and this is sought

by the technique of seismic tomography

(Section 17.7), but the pattern of convection is

not so simple as to make this straightforward,

except for the observation of high wave speeds in

the cool subducting slabs. Superimposed compo-

sitional variations confuse the picture. One

approach is to compare the P- and S-wave speeds

and for this purpose we need to know the tem-

perature dependences of the wave speeds. It

appears that, at least in the deepest part of

the mantle, temperature and composition are

correlated. The effects of composition on seismic

wave speeds at lower mantle pressure are not

well documented; the immediate task is to pro-

duce reliable information on the temperature

effect.

The strongest control on estimates of core

temperatures is the observation that the inner

core boundary (ICB) marks a transition from

liquid to solid iron alloy. This has prompted

numerous experiments as well as theories to

determine the melting point of iron at very

high pressures. Experimentally this is very diffi-

cult and the observations that we have rely on

extrapolations to ICB pressure from somewhat

lower pressures. There are also theoretical diffi-

culties. The solid phase of iron at core pressures

is not the familiar low pressure body-centred

cubic () form, or even the face-centred ()

form to which iron transforms between 1192 K

and 1617 K (and is approximated by the structure

of stainless steel) but hexagonal close-packed

"-iron. The theory of simple melting (Section 19.4)

can be applied to any of these phases if they are

well removed from the triple points on the melt-

ing curve that mark the solid–solid phase tran-

sitions, but the theory cannot be extrapolated

through the triple points. There is also doubt

about the melting point depression by light sol-

utes in the core. We estimate the ICB temper-

ature as 5000 K, acknowledging that it could be

in error by at least 500 K.

At the high temperatures of the Earth’s inte-

rior, thermal properties are approximated by

classical theory. In this context ‘high’ means

above the characteristic Debye temperatures,

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C19.3D

–

315

– [314–336] 13.3.2008 2:45PM



, of minerals that (for insulators) mark a tran-

sition in specific heat from strong temperature

dependence at low T to almost temperature inde-

pendent high T behaviour. Values of 

for com-

mon minerals at zero pressure are listed by

Anderson and Isaak (1995); almost all fall within

the range 200 K–1000 K, with those important to

the mantle at the upper end of this range. Except

for a thin surface layer, T /

> 1 throughout the

Earth, but that is not true for laboratory condi-

tions. Unless measurements are made at high

temperatures, laboratory data on most thermal

properties can be applied to the Earth only by

extrapolations involving some understanding of

the quantum phenomena that become obvious

at very low temperatures.

The quantum t heory of specific heat began

with Einstein’s theory o f the energy of a har-

monic oscillator, meaning one of the vibra-

tional modes of a crystal. This is also the

starting point for a fundamental un derstanding

of thermal expansion. Expansion coefficient

and specific heat are closely linked and the theo-

retical connection is the Gr ¨uneisen parameter, .

This is a dimensionless combination of four

familiar properties, as in Eq. (18.2), repeated

here:

 ¼ K

=C

¼ K

=C

: (19:1)

 can also be defined as the ratio of thermal

pressure to thermal energy.

Throughout the Earth the numerical value of 

is in the range 1.0 to 1.5. It is much more nearly

constant than  or K

. It is virtually independent

of temperature at high T (at constant volume)

and its modest pressure dependence is a subject

of several theories that have converged to rea-

sonable agreement. The use of  has become

central to studies of the thermal physics of the

Earth because it provides a control on calcula-

tions that is otherwise unavailable. In the Earth

/ is well observed and C

is clearly understood,

but the only useful values of  are obtained from

 by Eq. (19.1). Normally  is used as a parameter

in its own right and not just as a proxy for ;

thermodynamic equations can be expressed in

terms of either, but whichever is used the

numerical values are obtained from . The

concept of  originated in solid state physics

but it rarely rates even a passing mention in

texts on solid state or thermal physics. This is a

reason for giving it close attention in Section 19.3.

The principal use of  in geophysics is in the

estimation of adiabatic temperature gradients

and the mechanical power of convection. It is

important also in the extrapolation of deep

Earth properties to zero pressure (Section 18.9)

and to melting theory (Section 19.4).

Many thermodynamic identities are relation-

ships between thermal and elastic properties

(thermoelasticity), and those most useful to geo-

physics are listed in Appendix E. They are basic

theoretical tools, the algebraic rules of the sub-

ject. We often make simplifying approximations

and these require some care. By starting with

the identities in Appendix E, we can judge the

adequacy of these approximations. One is the

assumption of constant specific heat, C

. Since

derivatives of C

appear in many of the identi-

ties, this is obviously a convenient simplifica-

tion, but we need to consider how satisfactory

it is. It is applicable only to insulators; the elec-

tron contribution to the specific heat of the core

does not behave in the same way as the lattice

component and it accounts for about 1/3 of the

total. For the mantle the assumption that

(@lnC

/@lnV)

is negligible is much better

than the assumption C

(@lnC

/@lnV)

0. This

is a useful point because in geophysics we are

generally more interested in adiabatic properties

than in isothermal ones. As can be seen in

Table E.3, particular care is needed with deriva-

tives of  because C

appears in the entries for

@ at constant P or V and it is divided by the

small quantity (T), making it an important

term. For constant S, however, this problem

does not arise.

The central theme of this chapter is the cal-

culation of thermal properties of the Earth, start-

ing with seismological data. The essential link

between elastic and thermal properties is the

uneisen parameter (Eq. (19.1)). By using it, we

are able to apply the detailed information about

the Earth’s interior derived from seismology to

the study of its thermal behaviour, especially

convection and the thermodynamic basis of

tectonics.

19 . 1 P R EA M B LE 315

//FS2/CUP/3-PAGINATION/SDE/2-PROOFS/3B2/9780521873628C19.3D

–

316

– [314–336] 13.3.2008 2:45PM

19.2 Specific heat

It is a popular supposition that the Earth is

hot inside because of the heat released by radio-

activity. Thus, it may come as a surprise to real-

ize that the total radiogenic heat release in the

life of the Earth is much less than either the heat

loss over this time or the present stored heat.

Heat capacity plays an essential role in the dis-

cussion of the global energy budget (Chapter 21)

as well as appearing as a thermodynamic param-

eter in the equations used to study deep Earth

physics.

As mentioned in Section 19.1, the interior of

the Earth is hot enough for the thermal vibra-

tions of atoms to be treated classically to a useful

approximation. Ignoring for the moment the

effect of conduction electrons in the metallic

core, this means that the thermal energy (of an

insulator) varies linearly with temperature, T.

For a solid with N atoms it is 3NkT (minus a

constant, as in Eq. 19.13, below), where k is

Boltzmann’s constant. We often refer to moles

and, for a substance with n atoms per molecule,

the thermal energy can be written as 3nRT per

mole, where R ¼N

k is the gas constant and N

Avogadro’s number. But many geological materi-

als are compounds or mixtures for which a mole

is not a convenient concept and thermal energy

is better expressed in terms of mass and mean

atomic weight,

m. Then the classical thermal

energy, 3RT=

m per gram atom, is

Classical E

Thermal

¼ 24 943 T=m Jkg

1

: (19:2)

Note that the mole is a unit identified with the

gram, but this equation gives energy per kilo-

gram. For the mantle we estimate

m  22 and

for the outer core

m ¼ 44:53 with the composi-

tion in Table 2.5, or 50.16 for the inner core.

Specific heat, the temperature derivative of

thermal energy, has a corresponding classical

value if heating or cooling occurs at constant

volume, V,

Classical C

¼ð@E

Thermal

=@TÞ

¼ 24 943=m JK

1

: (19:3)

is the specific heat occurring most often in

theoretical discussions but is not directly

measurable because, except for gases, heating

cannot occur at constant volume. In the normal

situation of heating or cooling at constant pres-

sure, P, there is an additional factor arising from

the energy required for thermal expansion and

the specific heat, C

, is related to C

by one of the

standard identities in Appendix E,

¼ð@E

Thermal

=@TÞ

¼ C

ð1 þ TÞ: (19:4)

Equation (19.4) is an identity, valid for all materi-

als in all situations, and is not restricted to the

classical (high temperature) regime, as are

Eqs. (19.2) and (19.3). In the Earth, C

exceeds C

by 3% to 10%. Values of C

are listed in the ther-

mal model in Appendix G.

Equation (19.3) is the Dulong–Petit law of

specific heat, which arose from early recognition

that heat is energy of atomic motion. But, even

within the assumption of classical physics there

is a small correction. The mean kinetic energy

per atom at temperature T is

kT for each of the

three independent directions of motion (x,y,z),

and the Dulong–Petit assumption is that the

average potential energy of stretched and com-

pressed atomic bonds is equal to the average

kinetic energy. This is the principle of equiparti-

tion, which would apply if the bonds were per-

fectly harmonic, giving sinusoidal oscillations.

But atomic bonds are anharmonic, as illustrated

in Fig. 18.4. There is a small anharmonic correc-

tion to C

, discussed in Section 19.8.

When we consider the thermal properties of

Earth materials at laboratory temperature, the

classical assumption breaks down. It is no longer

satisfactory to think in terms of thermal energies

of individual vibrating atoms, but, rather, the

energies of vibrational modes of a crystal lattice

as a whole. The atoms do not move independ-

ently; there are standing waves with a wide

range of wavelengths and corresponding frequen-

cies. These modes are not excited to any arbitrary

level of vibration, as assumed in the classical

theory, but are restricted by quantum principles,

so that a mode of frequency  may have any of a

series of discrete energies (n þ

)h,wheren is an

integer and h is Planck’s constant. This is the basis

of the quantum theory of specific heat. The theory

may appear to be of limited relevance to the

Earth, almost all of which is at a sufficiently

316 THERMAL PROPERTIES