Simon Benninga. Financial Modelling 3-rd edition
Подождите немного. Документ загружается.


578 Chapter 21
This protective put or portfolio insurance strategy guarantees that you
will lose no more than $6 on your share of General Pills stock. If the
stock’s price at the end of the year is more than $50, you will simply let
the put expire without exercising it. However, if the stock’s price at the
end of the year is less than $50, you will exercise the put and collect $50.
It is as if you had purchased an insurance policy on the stock with a $6
deductible.
Of course this protection doesn’t come for free: Instead of investing
$56 in your single share of stock, you have invested $58.38. You could
have deposited the additional $2.38 in the bank and earned interest of 8
percent
*
$2.38 = $0.19 in the course of the year; alternatively, you could
have used the $2.38 to buy more shares.
21.2 Portfolio Insurance on More Complicated Assets
In the example, we have implemented a portfolio insurance strategy by
purchasing a put whose underlying asset exactly corresponds to our
share portfolio. But this technique may not always be possible:
•
It could be that there is no traded put option on the shares we wish to
insure.
•
It could also happen that we want to purchase portfolio insurance on
a more complicated basket of assets, such as a portfolio of shares. Puts
on portfolios do exist (for example, there are traded puts on the S&P
100 and S&P 500 portfolios), but there are no traded puts on most
portfolios.
It is here that the Black-Scholes option-pricing model comes to our
aid. From this formula it follows that a put option on a stock (from here
on, “stock” will be used to refer to a portfolio of stocks as well as a single
stock) is simply a portfolio consisting of a short position in the stock and
a long position in the risk-free asset, with both positions being adjusted
continuously. For example, consider the Black-Scholes formula for a put
with expiration date T = 1 and exercise price X. At time t, 0 ≤ t < 1, the
put has value
P SNd Xe Nd
tt
rt
=− − + −
−−
() ()
()
1
1
2
where

579 Portfolio Insurance
d
S
X
rt
t
dd t
t
1
2
21
1
1
1=
(
)
++ −
−
=− −
ln /2)(
,
()
σ
σ
σ
where 1 − t is time remaining to maturity and S
t
is the price of the stock
at time t.
Thus buying a put is equivalent to investing Xe
−r(1−t)
N(−d
2
) in a risk-
free bond that matures at time 1 and investing −S
t
N(−d
1
) in the stock.
Since the investment in the stock is negative, a put is equivalent to a
short position in the stock and a long position in the risk-free asset.
The total investment required to buy one share of the stock plus a put
on the stock is S
t
+ P
t
. Writing this out and substituting in the Black-
Scholes put formula gives
Total investment, protective put =+
=− − +
−−
SP
SSNd Xe N
tt
tt
rt
()
()
1
1
(()
[()] ()
() (
()
()
−
=−−+ −
=+ −
−−
−−
d
SNdXe Nd
SN d Xe N d
t
rt
t
rt
2
1
1
2
1
1
2
1
))
where the last equality uses the fact that for the standard normal distri-
bution N(x) + N(−x) = 1. An even more useful way of looking at this
problem is to regard the total investment S
t
+ P
t
at time t as a portfolio
of a stock and a bond; we can then ask what is the proportion ω
t
of this
portfolio invested in the stock at time t. Rewriting the preceding formula
in terms of portfolio proportions gives
Proportion invested in stock =
=
+−
−−
ω
t
t
t
rt
SN d
SN d Xe N d
()
() (
()
1
1
1
2
))
Proportion invested in risk-free asset =−
=
−
−−
1
1
2
ω
t
rt
t
Xe N d
SN
()
()
(() ( )
()
dXe Nd
rt
1
1
2
+−
−−
In summary, if you want to buy a specifi c portfolio of assets and an
insurance policy guaranteeing that at t = 1 your total investment will not
be worth less than X, then at each point in time, t, you should invest a
proportion ω
t
of your wealth in the specifi c portfolio you have chosen,
and a proportion 1 − ω
t
in riskless, pure-discount bonds that mature at

580 Chapter 21
t = 1. The Black-Scholes put-pricing formula can be used to determine
these proportions.
21.3 An Example
Suppose you decide to invest $1,000 in General Pills (GP) stock (cur-
rently selling at $56) and in protective puts on the shares with an exercise
price of $50 and an expiration date one year from now. This method
insures that your dollar value per share at the end of one year will be no
less than $50. Suppose that there is no traded put on GP, so that you will
have to create your own put by investing in the share and in riskless
discount bonds. The riskless rate of interest is 8 percent, and the standard
deviation of GP’s log return is 30 percent. We will construct a series of
portfolios that implements this strategy on a week-by-week basis.
1
21.3.1 Week 0
At the beginning of week 0, the initial investment in shares of GP
should be
ω
0
=
+
=
∗
+
=
SNd
SP
01
00
56 0 7865
56 2 38
75 45
() .
.
.percent
with the remaining proportion, 1 − ω
0
= 24.55 percent, invested in riskless
discount bonds maturing in one year. If traded European puts on GP
existed and if these puts had an exercise price of 50 with an exercise date
one year from now, these would be trading at $2.38. Your strategy would
consist of buying 17.13 shares of GP (cost = $959.23) and 17.13 puts (cost
= $40.77). Buying $754.40 of shares and $245.60 of bonds exactly dupli-
cates the initial investment in 17.13 shares and 17.13 puts. This equiva-
lence is guaranteed by Black and Scholes. The calculations of the option
price and the appropriate portfolio proportions are shown in the follow-
ing spreadsheet picture:
1. There’s an implicit contradiction here between the fi nite updating strategy and the
continuous rebalancing that underlies the Black-Scholes model. This is discussed at the
end of this section.

581 Portfolio Insurance
We now turn to the end of week 0. You started at t = 0 with an initial
investment of $1,000; now suppose that by the end of the fi rst week the
price of GP shares had increased to $60. Then your portfolio value at the
beginning and end of the fi rst week would look like this:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
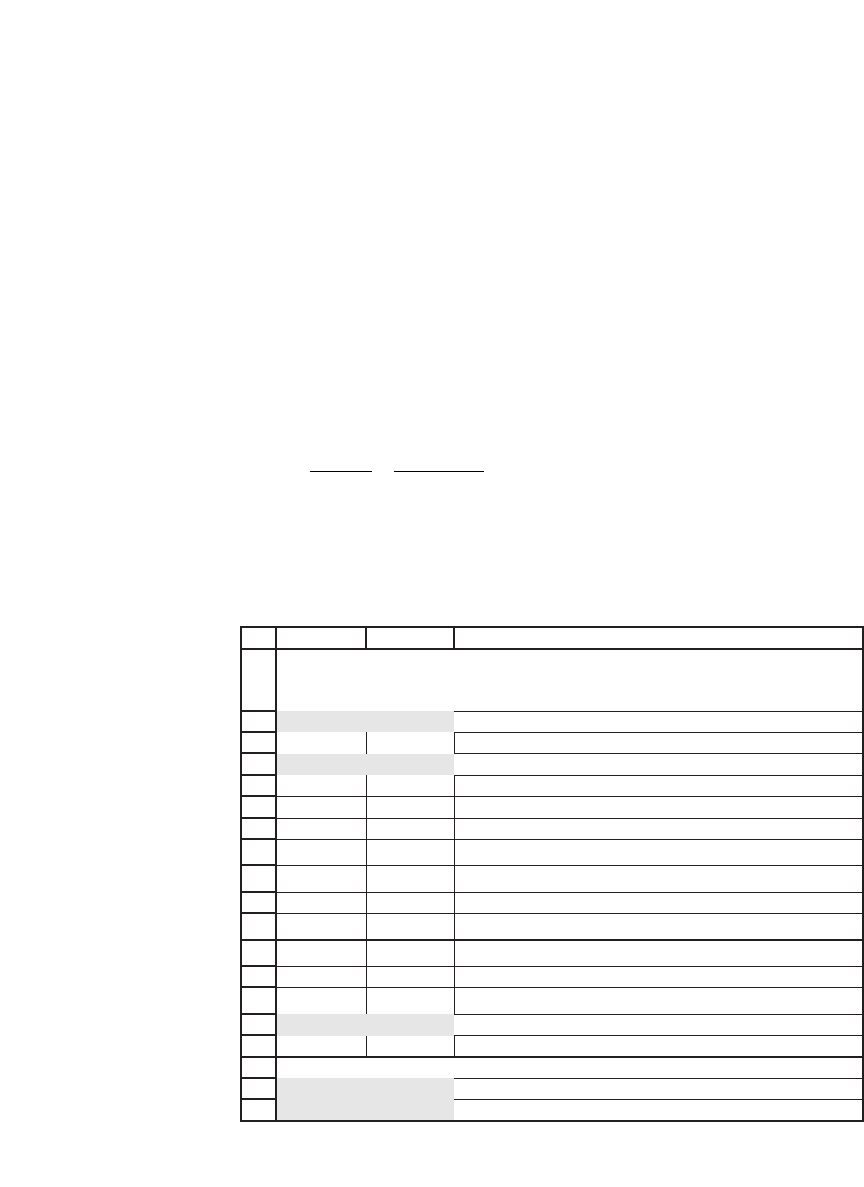
AB C
S56Stock price
X 50 Exercise price
T 1 Time remaining
r 8.00% Risk-free rate of interest
Sigma 30% Stock volatility
d
1
0.7944 <-- (LN(S/X)+(r+0.5*sigma^2)*T)/(sigma*SQRT(T))
d
2
0.4944
<-- d
1
-Sigma*SQRT(T)
N(d
1
)
0.7865
<-- Uses formula NormSDist(d
1
)
N(d
2
)
0.6895
<-- Uses formula NormSDist(d
2
)
Call price 12.22
<-- S*N(d
1
)-X*exp(-r*T)*N(d
2
)
Put price 2.38 <-- call price - S + X*Exp(-r*T): by Put-Call parity
Calculating the portfolio insurance proportions
Omega 75.45% <-- =B2*B11/(B2+B15), proportion in shares
1-omega 24.55% <-- =1-B18, proportion in bonds
Black-Scholes Option-Pricing Formula
Applied to General Pills Put
Portfolio value, beginning of week Portfolio value, end of week
Week
Stock price
at begin. of
week
Stock price
at end of
week
1
d
,
beginning
of week
Omega,
beginning
of week
Stocks Bonds
Portfolio
value
Stocks Bonds
Portfolio
value
0 56.00 60.00 0.7944 0.7545 754.50 245.50 1000.00 808.39 245.88 1054.27
Note that the value of your bonds at the end of the week is known in
advance; this value grows by a factor of 1.00154 = e
(1/52)0.08
per week.
2
The
2. Throughout we assume that the interest rate does not change. As an approximation to
reality, this assumption is acceptable in this situation (meaning that the effect of interest
rate changes is much smaller than the effect of changes in the stock price). If we wished
to account for interest-rate changes, then this would add another source of uncertainty
to the model.
582 Chapter 21
value of your shares, however, depends on the rate of growth in the price
of GP stock. During week 0, this rate of growth was 60/56 = 1.0714.
21.3.2 Week 1
At the beginning of week 1 you rebalance your portfolio, increasing the
proportion of equity and decreasing the amount invested in the bonds.
The new portfolio proportions refl ect the time to maturity (time after
one week is t = 1/52 = 0.0192), the stock price at the beginning of the
week ($60), and the total portfolio value at the beginning of the week
($1,054.27). As the next spreadsheet picture shows, you should now
rebalance your portfolio, investing
ω
0.0192
=
+
=
∗
+
=
SN d
SP
() .
.
.
1
60 0 8476
60 1 63
82 53 percent
in shares of GP and the remainder, 17.47 percent in bonds. Here $1.63
is the Black-Scholes cost of a European put, calculated with the correct
parameters:
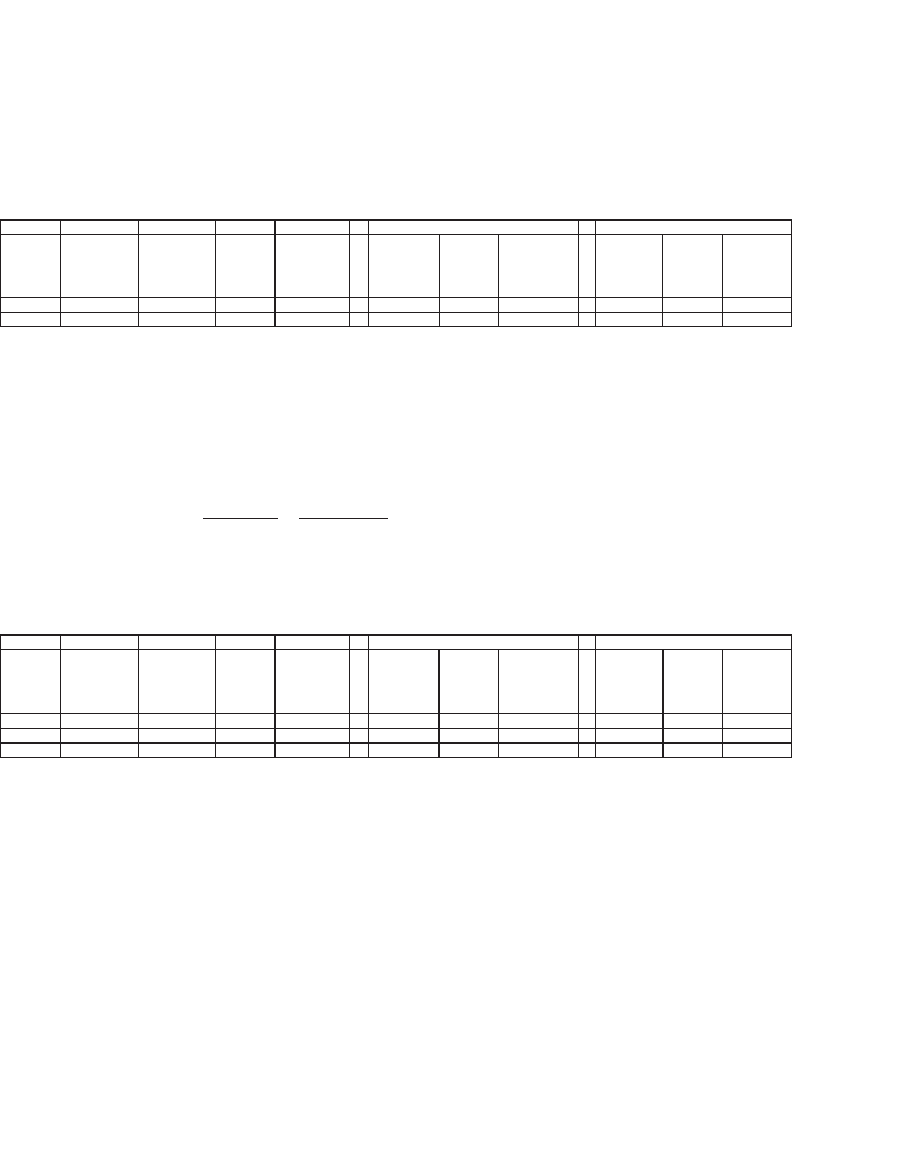
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
AB C
S60Stock price
X 50 Exercise price
T 0.9808 <-- =1-1/52 , time remaining
r 8.00% Risk-free rate of interest
Sigma 30% Stock volatility
d
1
1.0263 <-- (LN(S/X)+(r+0.5*sigma^2)*T)/(sigma*SQRT(T))
d
2
0.7292
<-- d
1
-Sigma*SQRT(T)
N(d
1
)
0.8476
<-- Uses formula NormSDist(d
1
)
N(d
2
)
0.7671
<-- Uses formula NormSDist(d
2
)
Call price 15.40
<-- S*N(d
1
)-X*exp(-r*T)*N(d
2
)
Put price 1.63 <-- call price - S + X*Exp(-r*T): by Put-Call parity
Calculating the portfolio insurance proportions
Omega 82.53% <-- =B2*B11/(B2+B15), proportion in shares
1-omega 17.47% <-- =1-B18, proportion in bonds
Black-Scholes Option-Pricing Formula
Applied to General Pills Put
583 Portfolio Insurance
Suppose that at the end of week 1, the price of a share of GP tumbled
to 52. Your position now would look like this:
Portfolio value, beginning of week Portfolio value, end of week
Week
Stock price
at begin. of
week
Stock price
at end of
week
d
1
,
beginning
of week
Omega,
beginning
of week
Stocks Bonds
Portfolio
value
Stocks Bonds
Portfolio
value
0 56.00 60.00 0.7944 0.7545 754.50 245.50 1000.00 808.39 245.88 1054.27
1 60.00 52.00 1.0263 0.8253 870.06 184.22 1054.27 754.05 184.50 938.55
2 52.00 ??? 0.5419 0.6635 622.73 315.82 938.55 ??? 316.30 ???
21.3.3 Week 2
Since another week has passed, the proportion of your investment in GP
should now be
ω
0.0385
=
∗
+
=
∗
+
=
SNd
SP
() .
.
.
1
52 0 7061
52 3 33
66 35 percent
This gives
Portfolio value, beginning of week Portfolio value, end of week
Week
Stock price
at begin. of
week
Stock price
at end of
week
d
1
,
beginning
of week
Omega,
beginning
of week
Stocks Bonds
Portfolio
value
Stocks Bonds
Portfolio
value
0 56.00 60.00 0.7944 0.7545 754.50 245.50 1000.00 808.39 245.88 1054.27
1 60.00 52.00 1.0263 0.8253 870.06 184.22 1054.27 754.05 184.50 938.55
The uncertainty will be resolved only when we know the value of the
shares at the end of the week.
Of course this example is somewhat misleading because the Black-
Scholes model assumes that portfolio proportions are continuously
adjusted, whereas we have waited a whole week to readjust our pro-
portions. In the background lurks a pious hope that fi nite (but short)
adjustment intervals will approximate the Black-Scholes continuous-
readjustment scheme. (Since we are only human, we can’t in fact make
continuous adjustments to our portfolio. Moreover, since readjustment
of the portfolio involves transaction costs to the investor, only fi nite
adjustment is possible.)
584 Chapter 21
21.4 Some Properties of Portfolio Insurance
The preceding example illustrates some of the typical properties of port-
folio insurance. Three important properties are the following:
property
1 When the stock price is above the exercise price X, then the
proportion ω invested in the risky asset is greater than 50 percent.
Proof The proof of this property requires a little manipulation of our
formula for ω. Rewrite ω as
ω=
SN d
SN d Xe N d
Xe N d
SN d
rt
rt
()
() ( )
()
()
()
()
1
1
1
2
1
2
1
1
1
+−
=
+
−
−−
−−
We will show that when S ≥ X, the denominator of ω is < 2, which will
prove the proposition: First note that when S ≥ X, X/S ≤ 1. Next note
that e
−r(1−t)
< 1 for all 0 ≤ t ≤ 1. Finally, examine the expression
Nd
Nd
Ntd
Nd
NtSXrt
()
()
()
()
(. ( ) ( )
−
=
−−
=
−− + −
[]
2
1
1
1
1
05 1 1
σ
σσ(1−ln /
tt
NtSXrtt
))
(. ( ) ( ) ))05 1
1
σ1− σ(1−++−
[]
<
ln /
This proves the property.
property 2 When the stock’s price increases, the proportion ω invested
in the stock increases and vice versa.
Proof To see this property, it is enough to see that when S increases,
the value of the put decreases and N(−d
1
) decreases. Rewrite the original
defi nition of ω as
ω=
−−
()
[]
+
=
−−
[]
+
SNd
SP
Nd
PS
11
1
11
()
/
Thus, when S increases, the denominator of ω decreases and the numera-
tor increases, which proves Property 2.
property 3 As t → 1, one of two things happens: If S
t
> X, then ω
t
→ 1.
If S
t
< X, then ω
t
→ 0.
Proof To see this property, note that when S
t
> X and t → 1, N(d
1
) →
1 and N(−d
1
) → 0; thus for this case ω
t
→ 1. Conversely, when S
t
< X and
585 Portfolio Insurance
t → 1, N(d
1
) → 0 and N(−d
1
) → 1 and thus ω
t
→ 0. (Strictly speaking
these statements are only true as “probability limits”—see Billingsley,
1968. What about the case when, as t → 1, S
t
/X → 1? In this case ω
t
→
1
/
2
. However, the probability of this occurring is zero.)
21.5 What Do Portfolio Insurance Strategies Look Like? A Simulation
What do portfolio insurance strategies look like? In this section we con-
sider this question by simulating such a strategy. Throughout we assume
that the interest rate is constant and that the stock price is lognormally
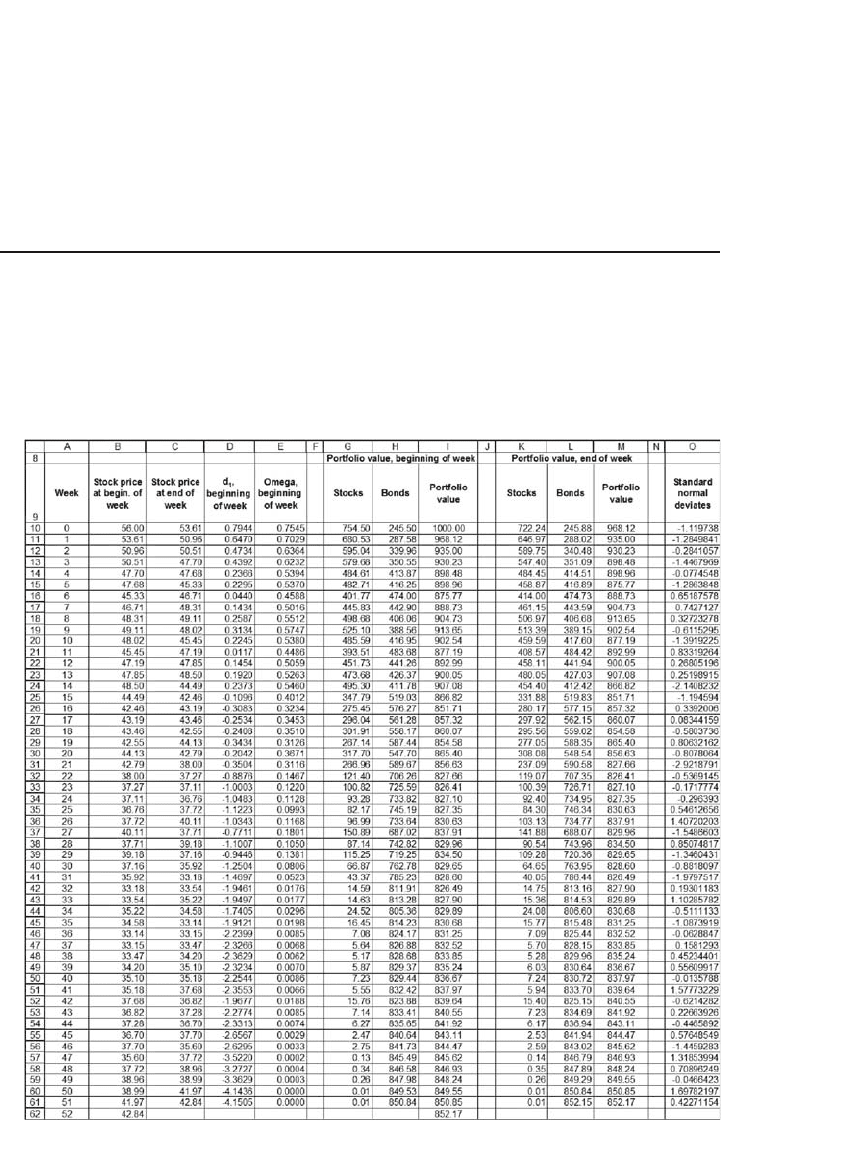
distributed. Here is a sample from the output of a simulation:
586 Chapter 21
All the columns of the simulation have already been explained except
column O: This column contains a series of random numbers drawn from
a standard normal distribution. The price of the stock at the end of each
week is determined by these random numbers. In this example the stock
price of 56.87 at the end of week 0 is—as explained in Chapter 18—deter-
mined by the lognormal assumption
SS e
tt
tZt
=∗
−
+
1
μσ
ΔΔ
,
which for this
particular case becomes
56 59 50
015
1
52
0 30 0 183745
1
52
.
...
=∗
∗+ ∗
e
. As explained in
Chapter 18, these standard normal deviates were created with the Excel
command Tools|Data Analysis|Random Number Generation.
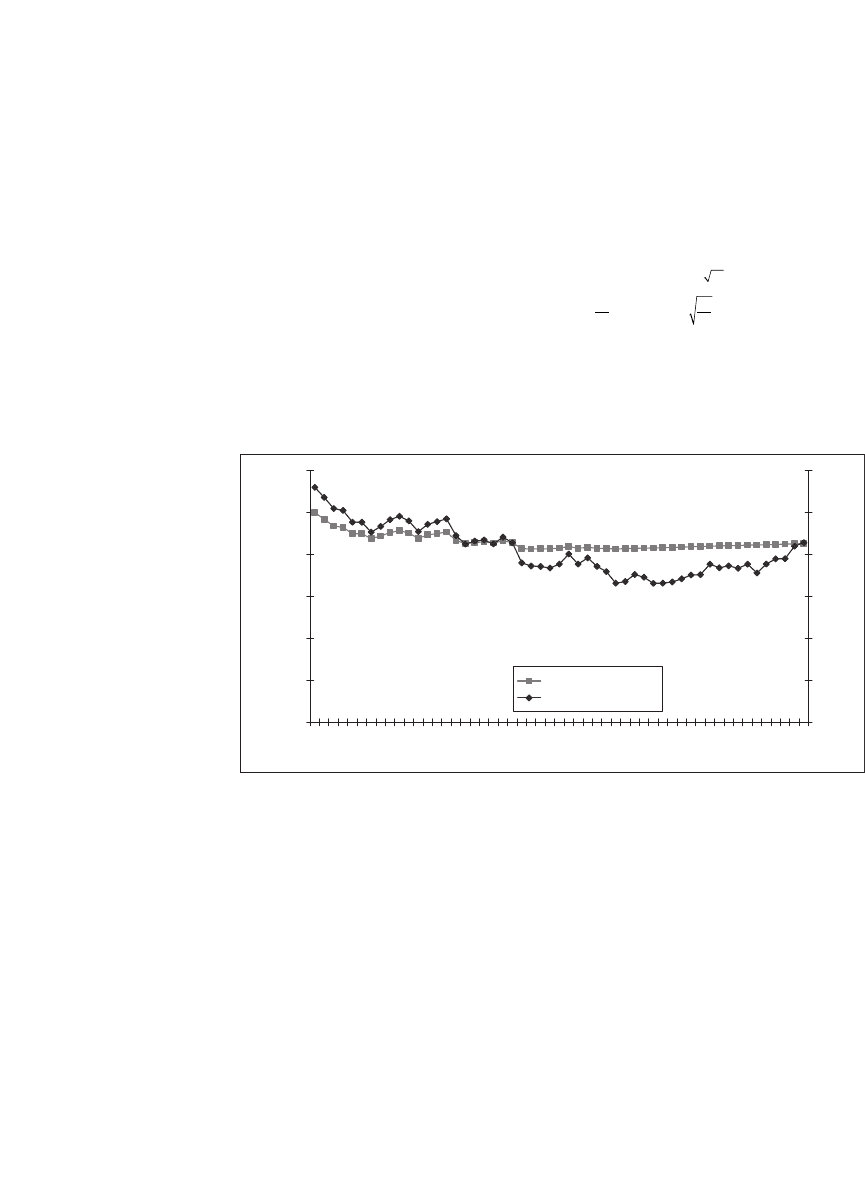
When graphed, the results look like this:
Portfolio Insurance Simulation
0
200
400
600
800
1,000
1,200
0 2 4 6 8 10121416182022242628303234363840424446485052
Week
Insured portfolio value
0
10
20
30
40
50
60
Stock price
Insured portfolio
Stock price
In this particular graph the stock price (graphed on the right axis)
declined over the year, so that the portfolio insurance strategy pays off
more than a straight stock strategy would have. In effect, we have used
the puts that are implicit in this strategy.
In this example, the stock price declines below $50 per share. As we
pointed out in section 21.3, at date 0 the portfolio insurance strategy
involved the equivalent of purchasing 17.13 shares of the stock and a put
on each of these shares. If our simulation were continuously updated, the
portfolio value would never dip below $856.50 (= 17.13 * $50). In this
case, because the updating of the portfolio positions is weekly and not
continuous, the terminal value of the portfolio is close to $856. As sug-
gested by Property 3 of section 21.4, by the end of the year, the portfolio
587 Portfolio Insurance
insurance strategy is wholly invested in bonds. By week 47 there is no
investment in stocks whatsoever.
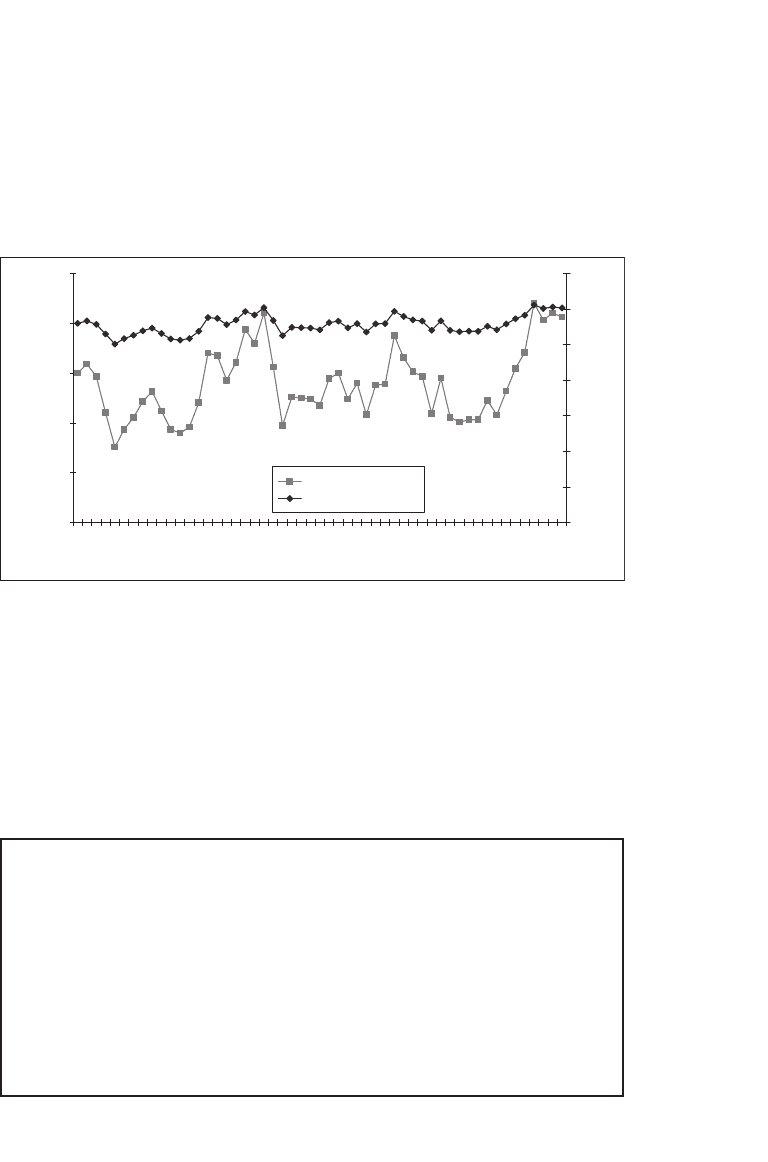
We can rerun the simulation and graph the output, tracking both the
stock price and the total portfolio value over the course of the year:
Portfolio Insurance Simulation
850
900
950
1,000
1,050
1,100
0 2 4 6 8 10 12 14 16 18 20 22 24 26 28 30 32 34 36 38 40 42 44 46 48 50 52
Week
Insured portfolio value
0
10
20
30
40
50
60
70
Stock price
Insured portfolio
Stock price
In this example, by the end of the year the portfolio insurance strategy
will be wholly invested in stocks (again this result follows from Property
3 of section 21.4). In this example the portfolio insurance proves more
expensive than an uninsured investment—ex post, we would have been
better off not following an insurance policy.
We can use the Tools|Record Macro feature of Excel to produce a
macro that automates the simulation procedure.
Sub Simulation()
‘ Simulation Macro
‘ key assigned: [Ctrl]+a
‘
Application.SendKeys (“{Enter}”)
Application.Run “ATPVBAEN.XLA!Random”, _
ActiveSheet.Range(“$O$11:$O$61”), , _
, 2, , 0, 1
End Sub
