Raabe J. Hydro power - the design, use, and function of hydromechanical, hydraulic, and electrical еquipment

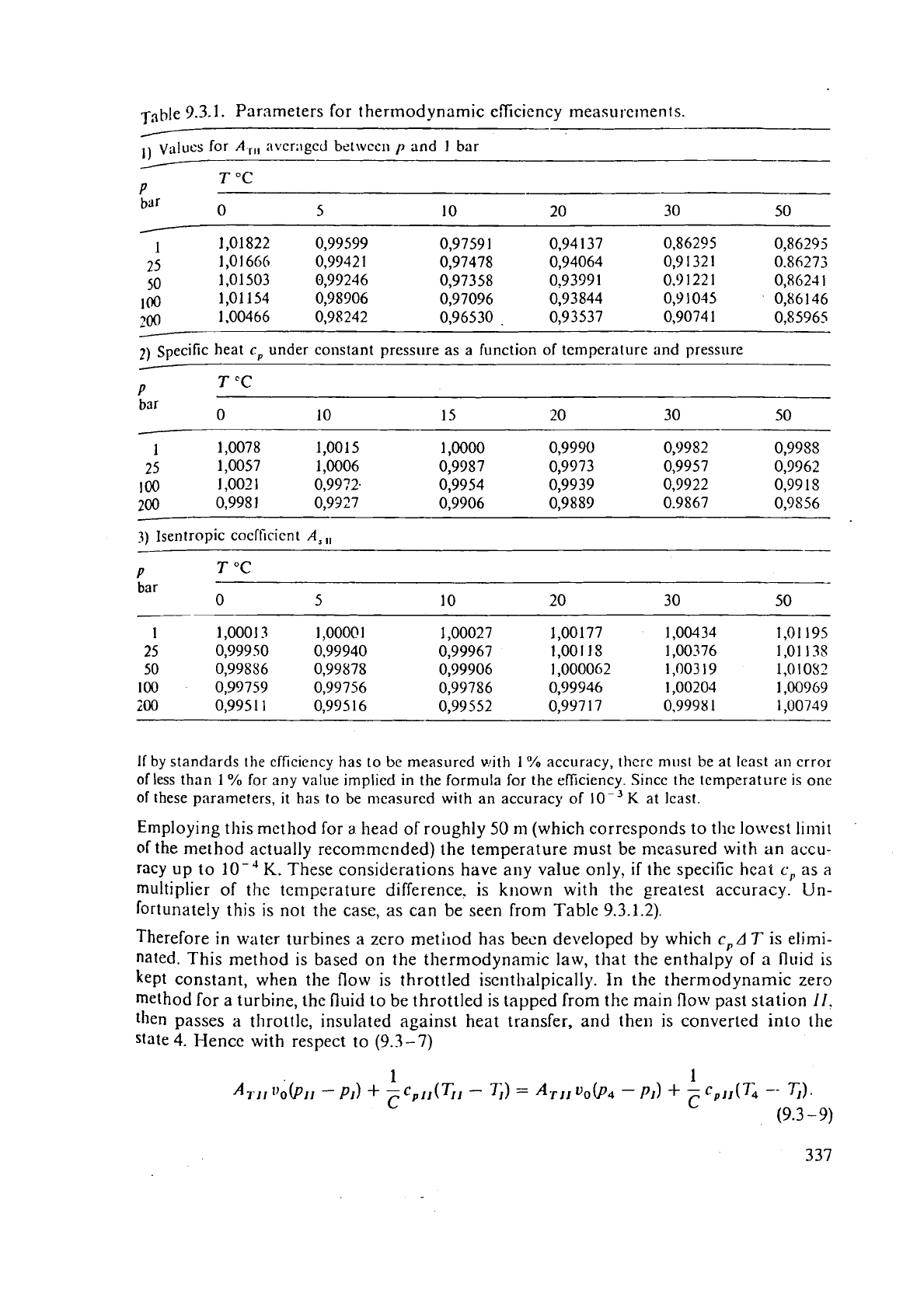
Table

9.3.1.

Parameters for thermodynamic efficiency measurxxnen ts.

/

Values for

A

,,,

nvcr;~ged betwccn

p

and

1

bar

/

P

T

"C

bar

0 5 10 20 30 50

2)

specific heat

c,

under

constant pressure as

a

function of temperature and pressllre

-

P

T

'C

bar

0 10 15 20 30 50

1

1,0078

1,0015 1,0000

0,9990

0,51982 0,9958

25

1,0057

1,0006 0,9987

0,9973 0,9957 0,9962

100

1,002

1

0,99?2. 0,9954

0,9939 0,9922

0,99

1

S

200

0,998

1

0,9327 0,9906

0,9889 0.9867

0,9S56

-

3)

Isentropic cocfficicnt

A,

,,

P

bar

If

by

standards

the

efficiency

has to be measured

with

1

%

accuracy, thcrc must be

at

least

an

crror

of

less than

1

%

for any value implied

in

the formula for the efliciency. Sincc the temperature

is

one

of

these parameters,

it

has to be measured

with

an accuracy of

K

at

Icast.

Employing this method for

a

head of roughly

50

nl (which corresponds to the lowest limit

of

the method actually recornmcnded) the temperature must be n~easured with an accu-

racy

up to

lo-'

K.

These considerations have any value only, if the specific heat

c,

as

a

multiplier of the temperature difference. is known with the greatest accuracy. Un-

fortunately this is not the case, as can be seen from Table 9.3.1.2).

Therefore in water turbines a zero

method has becn developed by which

c,

A

T

is elimi-

nated. This method is based on the thermodynamic law, that the enthalpy of a fluid is

kept constant, when the flow is throttled

iscnthalpically.

In

the thermodynamic zero

method for a turbine, the

fluid to be throttled is tapped from the main flow past station

11.

then

passes a throttle, insulated against heat transfer, and then is converted into the

state

4.

Hence with respect to

(9.3

-

7)

7'11~

trick

of

tllc

/cro

~iictllocf consists

ill

throttling.so

tli;it

tl~c te~npcraturc.

'r,

(past the

tllrottlc)

;irltl

1;

in

ilic

csIi;~~ist

~011ncc'tion hcco~lit' eq11;ll by adcqr~atc ;:~ljustn~cnt of

the

2

tllrottlc.

l'llu.;

thc

crl~Ii:llpy tliffcrct~cc bctwceri tl~c prcssurc

and

the

cxliaust COlincction

of

the machinc I~ccomc~

$

By

this tllc nct hcnd llic 1ot;ll cnthalpy diCfcscnce gives

It slioulJ bc nicntionetl that

in

an ex;lct tcst, the very slight variation of gravity acccleratioll

g

vs

;iltit~~dc ant1 latitutlv sllo111d be considcrcd

[8.123].

Ry

making

equril the cross section

'4,

and

.4,

and

arrailgirig the same velocity distrib",

tions in them, continuity eliminates the term due to

c,

and

c,

in (9.3-11). Thus

the

measurement of

the

enthalpy ;is the work done per unit mass of the real machine

is

reduced to

!he

nie:~sursn~ent of

a

pressure difference

p,

-

p,.

The problem arises, from ~vhat point of the cross scction

11

the flow should be taken. With the fluid

tcmpcrature oS:i.hat point

in

scction

1

it sliould be compared?

It

should be

a

point with the same

mass-averaged v;11ucs of

C

illld

T

in both the cross sections.

Such

3

point can be obtained only from

tests over rhc cross szc!ion for encli Flow regime. Obviously thc st:~tiou for the mass-averaged tern-

pcra!arc tiiffcrs frorn thilt duc to the muss-avcraged kinetic energy. Since the temperature term

is

decisive

tlris must be

preferred.

To avoid disturbnnccs of mcnsurcment by oscillations of tlie intake tappi~ig, the support of this

strcarnlinetl

bod!.

shoulci bc sufficiently stiff. Positioning too near to the wall is not advisable for the

follo\ving rci~soi:?;.

rls

previously shown, this lnethod is restricted to heads above

50

m. Since the

miscd Co\v ni;ichinc

predominates

in this range, attentiori should be directed to the fact, that most

OS

the losscs hcrc arc causcd by leakngu

anrl

disk friction and thcn transmitted to the relatively small

Icalcage llow, thus bcing cscessively hented compared with the bulk of tlie main flow passing the

runner.

Because of

thc low thcrrnal diffusivity of water, this heated liquid layer close to the draft tube

w

cannot be expected to mix

\vitll

the adjacent main flow down to station

I.

Fortunately many

d

tubes, especi;!lly thosc for vertical sets have a bend. Owing to thc sccondary flow in this bend (n

for once 11sl:ful). tlic \!.rtrmcr OlitcI layers arc mixed with the main flow.

If

tIi~

head

y

1-1

Lills

short of

50

m,

the

internal energy term of

(9.3-

1)

cannot be measur

accurately

eno!.lgh.

Thus

the conventional method must be applied zccording to

whi

the specific head

(now

restricted to the mechanical energy terms)

Y

=

yH

=

A(y/g

+

c2/2

+

ylz)il

requires the measurernents of the flow-averaged values

p/~,

c2/2 and

yh

in the

cro

sections

I1

and

I.

9.3.3.

Tl~ermodynamic

measurement

of

internal efficiency

In the case of

a

turbine, the internal efficiency represents the energy converted into

bla

work in relation to the energy offered by the fluid, both referred to unit mass of th

The difference of both the energies implies the losses, converted into heat and tran

to

tl:e fluid.

-

These

are:-The hydraulic flow losses in the admissio~i part (distributor, suction pipe) the rot

the

diffuser. including also the so-called exchange losses bet\veen rotor and stationary part

(d

distributor. suction pipe) in

consequence

of the closed flow loops

at

the ends of the rotor

v

335

51,rolld and huh c.spcci:illy ivhcrl \\lorking undcr off-dcsign conditions. Lastly

ii

comprises thc lossrs

of

d,,<~

fl-ictioll

and

Ica

k;lgr

(volunict ric loss) in thc rotor.

The

cncrgy offcrcd by the hcnd in the case of

a

turbinc corresponds to thc difirencc of

cnthalpy in an ideal nlncliine without these losses and without the lie;~t

flow

conr?ec?ed

l,crewith

A,,).

entropy

increment

'd.~'

is combined with

a

heat

dq

transferred irreversibly into the

fluid fro111 outside according to

ds

>

dq/T

(9.3-

13)

~t~i~tly speaking. this relation is valid

first

for a heat

flow

crossing thc system bounclnry of the

m3cliinc.

Now,

we

knon,

that the heat transfer through the walls of a hydraulic machine is negligibly

S,,,~I]. The heat, flowing into the fluid of a real machine, as

a

black box, from outside can

bc

inrcrprctcd as follows: First thc internal loss 3s an irrcvcrsible work is donc

by

kirtuc of viscous and

turb~lcncc-condirioncd stress undcr the strain ratc of thc fluid. This dissipation gcncrates hciit.

wllich can be considered 3s being afterwards

transferred

from outside into thc systcm (machine).

Col~trary to multistage thermal turbo engines, this heat revovery cannot be used in the following

~tages, as thcy usually don't exist in

a

water turbine.

~hus the real ~nachinc can be understood as a machine with heat flow from outside the

spstelll into

it.

Then tile entropy varies in the case of

a

real machine.

The

thcrmodynamic

relation for the increment of the enthalpy as a furlction of that of the entropy

ds

is given

by

[9.861

di

=

(d

ilds),

tls

+

(o7i/dp),

dp.

For an ideal machine, the increment of entropy vanishes. Consequently

Idcnce the finite difference of total enthalpy of an ideal machine

with

the so-called isentropic coefficient (Table 9.3.1.3)

A,,,

=

(l/n,)

cai/(?ll>,.

(9.3

--

1

7)

Togetller with

A

if

from (9.3- 11) the internal efficiency reads for

a

turbine

(T)

and a pump

(0

(Table 9.9.3),

1

=

AA;

qip

=

Aifs/Aif. (9.3-

18)

ll-ic di\lergence of isobars in

Fig.

9.3.1

a

reveals that

for

a certain increment of entropy, the intcrnal

cficiency

of

a pump is lower than that of

a

turbine operating with the same pressure difference

PI/

-

PI-

From (9.3-

i8),

(9.3-

16), (9.3-

11)

it is seen, that the efficiency is only given by the

measurement of

the differential pressures

p,

-

p,,

-

p,, when the energy diffel-ences

r:

-

r:,

rh

-

c.:

are imagined as small or compensated

by

adequately setting

A,,,

A,,

A,.

Thus any

flow

measurement

is

eliminated.

.

9.3.4.

Convcntion;rl

measuremeilt

of

internal

efficiency

This so-called conventional measurement of

the

internal efficiency has to be applied,

when

the head falls short of

50

m.

Here the measurement

of

flow

Q,

especially on the

Prototype is

a

rather time-consuming and error-charged procedure, which makes this

Fig.

9.3.1.

Working proccss of real machine with losscs.

a)

Real proccss

(---),

and ideal process

ds

=

0

of !luicl machine on thc enthalpy(i), entropy(.s)-diagram between isobars pl,,pl.

T

turbine,

P

pump. Diverscnc:: of isobars causcs

a

better efficiency in turbine proccss than in pump process

for

a givcn incrcasc

in

entropy.

b)

Torqi~e reaction dynamometer with elimination of shaft friction

when

nlcasuring the intcrnal torque

Ad,

=

Mi

by weight-induced load

F.

c) Schcme of power loss compo-

nents in an

inipcller pump from the shaft power

PC.,,

to the net hydradic

power

input

Pb.

Pm

n~echrtnicnl po\\er loss,

Pd,

power loss by disk friction,

Po

po\ilt.r loss by leakage flow,

c,

hydraulic

loss

in suction linc, impeller, diffuser and by exchange losses between stationary part and impeller,

intcrnal input,

P,

peripheral input. In

a

turbine transformation of power

is

rcvcrsed.

method infcrior to thc tllermodynamic method, (provided the head allows its applica-

tion~.

As

methods for flow measurement may be mentioned:

a)

In

the prctotype: bleasurement of the velocity profile by flow

metering,

srilt solution

method,

in

closed ducts alio by salt velocity method, Gibson watcr hammer method

[9.91

ro

9.94j.

ultrasonic met hod by Klrc~pp,

Boettclrcr

L9.951.

5)

In

the

modcl

test:

Venturi

metcr, weighi~lg machine, measuring tank, calibrated wei

flu\.:

metcrin~.

Prandtl

probe or similar probe, inductive method. Laser velocimeter,

h

film

probz, hot wire probe, semiconductor probe. Hence the gross(in)output

6

=

QQ

Y

=

~Qgtl.

The internal out(in)put results from the speed

w,

usually measured

by

induction, and

t

torque

?il.

measured either on the prototype

by

means of strain gauges or on the

m

by

means

of

a

torque reaction dynamometer (of the hydraulic, electrodynamic or

tional type).

TIie

needed internal torque

Mi

can be obtained by strain gauges

spring-suspended, oil- or air-cushioned stator of the reaction torque meter also

contai

the shalt bearings (Fig.

9.3.1

b). Hence the internal out(in)put

q

=

OM,.

Thus the internal csEciency (negative sign

pump,

positive turbine)

qi

=

(e/e)T1.

Even

if

the conventional method is more time consuming, it may be more exact than

a

measurement on the prototype, when the measurement is carried out on

a

340

In3

&ined niodelh of

300

to 500 mni nonlinal diameter

C,

by means of

a

hydrost:t;ic, ilir-.

or

oil-bca~~ing-r~~o~~~ltcri

torque reaction dynamometer for torque IneasuI.cnlent and a

,veigl~i~~g lnachine or a tink for measurement of the flow. Hcnce accurilcy of

rl

on

lnodel

is

T

0,5

%,

including, e.g. maximum relative errors

of

flow and head up to

2

.

10

3.

TherCfOrc in future the model test will be preferred more and Inore as the decisive and

selccti~e procedure for setting an adequate design of

a

certain turbine. The prototype test

Hr;l~

be degraded to a control for the results predicted by the model test. Because

of

effi-

ciency scale effects the efficiency of the

smaller model, opernting under a snialler Reynolds

than the prototype becomes smaller than the efficiency of the latter.

Hence to predict the efficiency of a prototype

on

the basis of

nod el

tests, efficiency scaling formulas

are

recluired. Such formillas have been eithcr found by experience on models and prototypes

by

means of rather simplc rules postulated and improved since the eve of this century, or on

the

basis of pipe loss formu1:is by

Can~er-or

[9.85],

Moorly

[9.96],

PJcidercr

[3.11-1.

~~Iirr-c~t

19.971,

~lrtrorl

[9.98; 9.991,

Ostrr\~alder

[9.70;

9.100;

9.1011,

Riitschi

[9.102],

.bJiihlrr~~nitr,

[9.103], and

Fa).

i9.99; 9.1041.

A

special working group of the

1AHII

headed by professor

Oster\t'~lldcr.

[9.70] in thc past

17

years has collected much test material from industry.

A

compilation of all the findings wss

by

Osr~r\tlnlder

[9.70; 9.1051. An essential contribt!tion was also made by

Srrschclerzkv

[g.106] \lfllo rlssipcd thc losses to an equivalellt technical roughness typical for the machined surraccs

of

elements found in hydro turbomachines.

9.3.5.

The scale effect of internal efficiency

According to experience, the hydraulic loss depends on the Reynolds number and the

relative roughness of the duct walls.

-

Roughness: From pipe test we know, that the friction cocficient in the turbulent range

depends decisively on the relative sand roughness of the pipe wall

[5.27].

An application

of these test results to the ducts of hydraulic machines requires at first

a

substitution for

the

pipe diameter of the hydraulic diamcter of the duct's passage defined by

dl,

=

3

x

cross sectional arealperiphcry. Then it must be remembered, that thc pipe test

is

boundary layer, as the decisive carrier of loss in a

structural member of a fluid machine,

e.g.

the rotor, is one, developing along a starting

length. Thus at an arbitrary station within the duct considered, the boundary layer

thickness is surely below the hydraulic diameter

d,.

The latter is the value to which the

roughness elevation is related in pipc tests.

Morcover application of sand roughness test results is not correct, since the structure of wall

roughness there differs from that of sand

roilghness. In gcneral the

technical

roughness is morc

pointcd than the sand roilghncss and depends on the kind and the orientation of surface finish.

Hence relying further on

thc test results of sand roughness, requires the definition of an equivalent

sand roughness due to

a

certain measured technical roughness.

Srrscheletzky

made some eflorts to

do this

[9.106]

by means of penetrating decpcr into thc structure of a roughness and the

flow

about

it. Hints at roughness on hydro turbomacl~ines are given by

Grein

19.31.

An

older roughness modcl assumed, that any roughness has no influence on the loss,

if

the highest

roughness

elevation on a surface is submerged in the so-called laminar sublayer. The thickness of

this layer, having no turbulence-conditioned cross fluctuations of velocity, ob~iously was overesti-

mated.

Recent measurements in

thc flow laminae closc to the

pipc

wall by

Hartner

[8.136] by means of a

Laser

iinernolncter within a liquid having the same refraction index as the adjaccnt planely ground

~lcxiglass block, formirlg thc pipe wall, have indicatcd, that thcsc cross fluctuations of velocity have

unexpectedly

high

amplitudes

at a very small distance from thc wall.

T:IIc

~C;\SOI~

for

this

ptieno~iic~io~i is

givcn

by

thc

rncch;inism

of

tl~rhulcnt

flo~v.

u.1iic.h

opcr;ltcs

thn,

l~i~y

:tccortlirig

to

S~r:;c./tc-l~~/:~.

['3.10(,].

Particles

in

thc

nciyhho\~rliood

of

thc

w;tll

with

its

hig],

strail,

riitc

try

to

esc;~pc

illto

rcyions

with

lower

str;~il~

ratc. .This Icacl:; o\vi~ig to cc~nscrv:itiori

of

nl,,,

,,

a

~nc>venlcrlt towards

thc

will1

of

othcr

fluid

clc~iicnts

with

higlicr

velocity.

'l.hus

th~

fluid

butwecn

roughness

clcvntion

is

illways s~imulated

by

Iluct\~;ltions.

Thc inlluencc of roughliess is of highest interest for the mnnuhcturcr, who wants to know

to what degree any particular surktcc finish of tl~c ducts, e.g. grinding, has cllccts on

th;

efficiency.

-

HeJl~o/tl,s number. Here the influence might be limited to that on ducts with hydrauli-

cally smooth walls. Now follows a review of the elementary effect on the basis of loss in

a straight pipe. An elimination of the pressure drop in

a

pipe from relations

(5.4-

13) and

(5.4.-

15)

gives with

r

=

R

=

(112 the loss cocfficient of the pipe as a function of the

wall

shear stress

r

=

s,,,

whcre c,,, is the mean velocity of the cross section. Since the

flow

close to the wall is always

laminar

r,,

=

~v(Gc/~3y),,,, where

y

is normal to the wall.

Jn the relevant turbulent regime now considered the velocity distribution across

the

boundary layer, rhickriess 6, may be approximated by

c

=

c0(y/6)""', whe~e c, is the

vsluci

ty

at

the outer edge of the boundary layer. Hcnce

SciZy

=

[c,:011(S)]

(y/S)""

'.

In

conscquellce of the approximative velocity distribution, this derivntlve tends to infinity

at

tne \vall

(y

=

0). Assuming a laminar sublayer with constant strain rate

c?cidy

and

depth yo (yo

<<

6),

the wall shear stress may be expressed by

For simplicity the transition laminar turbulent is put

asidc. In

a

first order approximation

the boundary layer thickness

b

of the face considered, is assumed

to

be proportional

to

the nominal diameter of the machine

D.

But this is done only in context with 1n6, sine

the ratio

yo/d

may be assumed to vary only very little as a function of

D.

Doing so

in

(9.3-23)

and then inserting this in (9.3-22) yields

According to experience

thc velocity distribution across thc boundary layer becomes

more

"f~ill" with increasing Reynolds number now defined by

Re

=

c,

Dlv.

(9.3

-

25)

Hence the ratio c,/c,, decreases slightly vsRe. Due to this also nl rises vsRe. After

Schliclltirrg

[5.4]

for a pipe whose Reynolds number Re' is defined by the pipe diameter,

,,,

?rows from

In

=

6 to

m

=

10,

when Re' increases from Re1=

4

-

lo3

to

Re1=

3240.10!

Then the exponent

1

-

l/m changes from 0,832 to 0,9. Also

6/y,

may increase slightly

vs

Re.

However, this is strongly competlsated by the decrease of I/nr vs

Re.

ra~ging from

0.166 to 0.1. For b!v,

=

10, the product (Ilm) (6/v,)'-'I" decrc3ses from 1,29 at

Re'a

1

-

lo3

to

0.79

at

Re'

=

3240

.

lo3.

From this it is seen that tllc pipe loss coefficient

ds

creases

vs

the Reynolds number. Substituting the machine duct considered by

a

strai$l

2 2

pipe and remembering cilj(Z

H)

=

(c,,~c,,,~,) c, d(2

gll)

=

A'

KC^,^^

to depend

on

the

design

KC,,^

and on the load factor

A

=

c,~/c,,,, the loss ratio of the

partied$

element considered (may

be

the whole machine) can be expressed

as

49

For

a

certain mncliine or part of

a

machine the ratio

Llrl,

of duct len_rlh to hydrai~lic

diilrnctCr is

~VCII.

;ilso

KC,,,^,,

is given and constant

(if

scnlc cffccts of tlie bep arc put

,,idc). llcncc [he loss ratio dcpcnds only on the load Fdctor

/1

=

QIQ,,

and

j.

according

to

(9.3-24).

Fro111 this

it

is secn that at a certiiin load factor the loss

11;.

decreases vs

Re.

S3nle psoblcn~s arise whcn the above is applied to a real machine. The latter has some

sections

such as the draft tube, tl~at do not have remarkable scale effect. IIence only

a

P

ortion

'(I'

of tlie internal loss of the machine is subjected to scale effects. The portion

'rl'

of

scnle-subjected loss deperlds on the design.

A

high head Francis turbine with its

relati~~ly s~nall throat vclocity coeflicient

Kc,,,,

has at least at bep a rather large

'u'.

fir:

generally

adopted insensibility of the diff~lser loss

coefficient

against scale effc'cts night resuit,

fronl

a

gro\\'th

of

thc dissipation-linked turbulcncc level

in

the

draft tube proportional to its size, and

from

its

ratlicr

invariable inlet velocity

c,,

in

consequence of thc econonlicly restricted submergence

of

any

limiting

loaction turbine design.

On

the other hand

'a'

depends also on the load factor.

A

low head Kaplan turbine may

ha\le a small value of

'n'

sincc the draft tube loss

[,KC,:,

may amount to 0'07 at full load.

However, at

20

O/o

of this load, this loss is rcduced to

1,4

%,

Lvhereas the runner loss, before

2%

beorlles now

10

%,

so that

'a'

being 0,22 under full load

is

now increased

to

O,88.

Moreover

also

the

bcp

and

its

velocity coefficients

may

depend on

the

Reynolds n~rmber.

Further-

more

the

neglect oTRcynolds

-

dependent transition points and s:lpcrimposed rouglincss effccts ]nay

c~mplicatc

the

whole. Contrary to

pipc

flow,

the

boundary at

the

essential ele~ncnts

of

Ilqdro

rurbornnchines

is

subjected

to

scale-conditioned pressure gradients of the

main

flo,~~. Finally. rota-

tion,

wall

curvature

and secondary flow

in

fluid machines, makes the loss generation

thcre

diffcreiit

from

that

in

a

straight

pipc.

-

Practice oriented scaling due to Reynolds number without roughness effects: the

rollowing relation holds between the internal loss on the Model

(M)

and

the prototype

(P)

with a fluid of kinematic \k.cosity

112

D

ti

=

a,'

(

,\f/Dp)

(vp/~,\~)]""

+

1

-

0.

(9.3

-

27)

The

value

'VI

=

ViI'

-

'li~

(9.3

-

28)

is

called cfficicncy scaling or eficicncy valorization.

The

number

'a'

depends on the type of machine and its load factor. For Kaplan turbines

within 0,4

<

Q,'Q,,

c

2,2,

Oster\vulcler

assumed [9.100]

0

=

09

-

0,273

(Q/Q,,),,.

(9.3

-

29)

The same author proposed the exponent

'n'

to be in the case of a Kaplan turbine

n

=

0,893

log,,

Re

(9.3

-

30)

with

a

Reynolds number for Kaplan turbines defined by

Re

=

bv,

Llv

where

L

is the chord of a mean cylindrical section,

\v,

the

undisturbed throughflow

velocity giver1 by

w,

=

(w,

+

1v,)/2, where

w,

and

w,

are the relative vzlocities

at

inlet

edge

2

and outlet edge

1

of the runner vane.

According to an older proposal of

Ackeret

[9.97],

a

=

0,5,

n

=

5.

This was occasionally

Improved by

Nutton

[9.98;

9.991.

For Francis turbines

Ostrrrvnlder

has found

by

teSts

[9.107]

a

=

1

-

0,082

Q',

,

.,/(I

-

rlD)

(9.3

-

32)

in

wllich

Q',

,

op

is thc unit flow at bep,

q,

the

draft tube efficiency.

9.3.6.

Scvcrnl

ci'ficicnccs

and

their n~easorerncnt

In

gcncr;~l the ~ncasurc~nent of the intcrnnl inpt11 (output)

of a prototype (Tull size

machitx)

has

lo start from the measurement of the indicilted clcctric oulpl~t (inpr~t) of the

generator

(motor)

I:.,

as frutn

e.,

=

U,,.,

i,,.,

cos

9,

in which

UCjt,

is the effcctivt. voltage,

i,,,.

the effective current, cos

cp

the pewer factor.

The Tollowing losses have to be added (si~btractzd) to (from)

y,,

to obtain

e:

1

j

The electric alternator (motor) lcss under load

P,l,l,,,cl

containing a resistance and

reactance term.

2)

The mccl~:~nical

10s:;

of the alternator (motor)

e,,,

,,,

due to ventilation, disk friction and

due

to

its share of bciil-ing loss

(if

separable).

3j

The rnechi~nicnl loss of tlic hydraulic machine

en,

dlit. to its shaft seal

~,,,,,,,

and its

share or bearing loss

f?,,,,

$,,,.

Both the l~lachincs

ma!,

have different man

be

mcasured only simultalieously.

Thcref~re

tests i~ccds

21i

nrgreement such as a) the on

load

and

dtle

to the ventilntion of the gen

tional to the load on

it

and the latter propo

n

machine with vertical shalt, the thrust bearing load due to the tt~rbine consists 011 the onc

ban

of the

\\;eight

of its runncr

and

its shaft portion, and on the other hand of the hydraulic axial

thrus

c)

The vcntilation loss of thc

generator

rotor can

be

obtaincd by scaling up rnotlel tests.

Thus with the lower sig~l valid for the pump, the internal output (input)

becomes

E

=

P,I

+

Pl/tlosr

el

f

hrrn

*

en.

With lllc gross (nct) input (output.)

P,

=

qQ

yH,

the internal elTiciency for the prototy

is

given

as

(Fig.

9.3.!

c)

jji

=

(811;)

*

.

The coupling (out)input

P,,,,

is defined

p,",,,

=

4

T

8"

The loss by

disk

friction

G,

and leakage flow

PQ

may be obtained from

a

model test

a rotor, whose inlet and outlet cross section have been blanked off, where the friction

both the blanking sheets and bearings has been eliminated by separate tests. Hence

aft

;in adcquate scaling according to

4,.

=

const w3 D5(wD2/\~)T115, the hydraulic or perip

era1 (out)irlput of the prototype

p,=EfP,/+PQ.

P,

dif'fers from

P,

by

the hydraulic losses

P,

occuring in the wetted passages of the machi

such as distributor (diffuser), rotor, draft tube (suction pipe), and exchange of angu!

momentum between roior and adjacent stati~nary parts of the machine.

P.=P,TP,.

-

8,

c,

P,,

and

PQ

can be obtained

by

consuming

(Q)

measurements. Sirlcc t

especially within the rotor and

betwe

344

,roublcso~nc, thc

lilat

two re1;ltions can

Lx

urcd for the co~np~lt:ltion of the h~.dr;ll~li~ loss

Pr.

liencc the so-called pcriphcral or hytlraulic cfficicncy (Fig.

9.3.!

c)

,-hz

latter

is

rcqi~ircd for thc

Ilrrlcritrrr

equntic~~i, (Cap.

5.3).

Thc rnech;~nic;il efficiency f~r

,be

jmagi~1ilry cilse of

n

separate electric nwchine is defined

by

rhis value describes the qitality of the bcarings and the shaft seal. Thc disk friction loss

its efficiency results frorn

sinliIarly the loss and efficiency due to leakage flow

Q,,

The

valuc

I],,

can

be

estimated

or

obtained fro~n

he:^^

in& ai~d

shaft

se;il

1~5th.

It

ranges

b~t\vrcn

0,995

to

0,98.

l'hc

internal cfficicncy can

be

measured

by

the

conventional

or

tllc

rllcrmomcts~c ~ncihod

above),

the

di5k

friction cfficicncy and

the

volumc[ric

efficiency

can

be

~lleast~red

:I\

dcscribcd

above

or calcr~lated according

to

Cap.

5.5

For

the

linkage

of

P,,

and

FQ

uscd hcrc.

s:e

Cap

5.5

For the design of

a

machine

by

Elder's equatio~~ the peripheral efficicncy

11,

is rcqt~ired.

Since

lnodel tests on hydraulic loss are rarely round and co~nplicated,

rl,,

can be c,~lculated

[ram

ill,

Irb

and

II;,

as follows

9.4.

Experimental

techniques

9.4.1.

Instrumentation

for

steady

flow

9.4.1.1.

Rlanorncters: Funda~nentals: Manonlctcrs are uscd for the measurement of the

static pressure, which is

striclly speaking

a

pressure at the wall v.*hich guidcs a fluid.

It

is

measured by a pressure difference between the desired and

n

known pressure. Usually

the

latter is that of the surrounding atmosphere. Therefore each measurcnlcnt of

a

pressurc needs a simulta!~eous reading of the reference pressure.

-

Liquids ~nostly used in manometers: Usually the measurcd prcssurc is

in

eq~~ilibrium

with the height difference of

a

liquid colu~n~~. Mercury, \\later and carbontetrachloride are

used,

the latter especially for accurate measuren~ents in air tests. Air tests are Inore easily

carried

out and therefore often used at least as preliminary tests.

-

Types of manometers:

a)

U

type n7:tnolneter. .Phe t\vo branches should be

of

equal, constant and not toe small

diameter.

Thus eliminating errors due to surface tension.

b)

Inclined tubc manometer.

A

very sensitive instrument.

f)

Betz

manometer.

The

vertical branch with

a

very small tube is located co;~xially in the

interior of

a

much larger vessel, closed

at

its top,

on

the level of which acts the ilnknoivn

Pressure. The narrow branch contains

a

float which moves with its vertical scale along

an

optical projection device.

Weight manometer. For high pressore.

The

pressure acts

on

a

floating piston, which

i;;

nc;~rly frictionli::is.

Its

I;lscc is !,;~l;tiicctl by c;~libr;l.lctl ~vcights

on

the pistori. F-:linli~~~ti~,,

of

~rictioii

I>\

\il)r;~tt>~-x

~i~i~tio~i or rot;~tio~i of pist{)n.

C)

hl;~~ic~nictcr I;)r .ir~nt~lt;t~lcot~s nic;~~~rsc~~~cnL at

Iiliilly

st;~lions. Ilcre c;lcIl stiltion

is

conncciccl

I,:

i~

I~~C;I\LII-~II_C

ti1I~

\~ijl~

;I

I;trgc

VCSSC~

011

111~

~CVC~

of \vliicIi

~IC~S

the rcfcrellce

prcssu re.

-

('onvcrsion of prcss~~se illto

;it1

cIcctric sig1l:lt:

111

~c~lcr;~! this

voids

time loss

and

clinninutcs Iium;~n ohscrv;~tion crrors.

It

also l'i~cili~:~tes the

usc

of such an elcctric output

as

llle inpi~t of

;I

conlpi~lcr.

-

Type of co~~vcrsinn:

;I)

1'110~0

ccll:

.l'hc

c~.ll

is

lixcrl

on

:I

c;~rricr. whicll ~novcs

with

constant velocity from

a

point

of

ccrt;~in c.lcv;ltiol~

Joun

:iIo~ig

tlic

ni;~~ionictcr ti~bc. When

the

nicniscus of the liquid cc)lulnn

is

rc:~c.hc.ti. tl~c

lis!i~

Iicanl

c,rx:b~ing

lhu

ILI~C

is

\vc;~kcnctl by absorption bcfc~rc

rc;~ching

the photo

cell.

7-hc11 coun!i~lg

.;t;tl-tt;.

It

cntls

\vlicn

the

cirrricr p:~sscs

:I

Ycro mark of licigl~t

1c.vt.l.

17)

C;rp;~citor:

7'his

i.,

Iiscil

on

;I

niovi11y

c;~rricr-

simil;rr

10

;I).

!\t

thc

riiolr!cnt.

the

cylinrlricul capacitor

movcs

alnng

thc

iiquicl

colurii~~. ampliti~tlc or frcclucncy

of

;I

givcn clcctric;~l oscillation undergo

a

change.

cj

Resistor:

If

tlie m;lnolnctcr

licli~icl

is

;In

clcctric conductnr,

it

can be conncctcd to one pole ofa

b;lttcry. Thc otltcr

[~olc

is

con~lcctcd

via

n

straight

stiff

tIii11

?\.ire i~tt:iclicd to tlic tubc and dipping

in

thc

n~anoriictcr IiquiJ

at

its

oplxlsite

end.

The c!cc.tl.ic;\I rcsi~t;~ticc

of

tli~

non submcrgcd part

of

this

\virc

:lr~tl

thc

ci!rrc~~t

restilting hcrcol' ir:dic;~tc.;

1111'

Icn~lh

ol'

!hc n1:rnomctcr column.

dt

L)cform;~iion

bj

prcbburc: Thib c;t~l

Ilc

i~sccl

by

piciro clirartz cl-yst:ll or by

u

disk

with

n

micro strain

gallgc.

-

Problems involtrecl in nlzasusc~nent of pressure:

:II

I_)ampi~iy

nT

oscilliitio~ls.

A

narrow throat at the inlet of

the

n1:lnometer tube

may

be

lij~fl~l.

51

Guiding walls

\\.it11

non-stnllsti

flow

as

n

presecluisite for ;illy prcssure n:easurement:

S

lc;~siircment

oi

prcssiirc within

il

l7u~v ficltl recli~ises the usc of

a

mc:~suring probe so that

i?ow

sep;ira:ion is not caused ancl gcner.:~Ily so that

thc

rnain flow is tlisturbcd as little as

possible.

1-his is attained by ~nakir~g thc streamlined carrier ol'tlic pressure tappings small

i~r

comparison

\\.i;ll

the size of thc duct cvherc t!lc ~ncasurcmcnt is made. Furtllcrmore the

sur-hcc of this pr-ol?e ntxr the t:~ppinzs has to be ;is far

as

possible in tlie tangential

d~rcction

of

the

110~:

to be

measured.

,

3

,.

Si~ch

a

streamlined body contains usually four tappinss in its cylindrical port, distributd

u11ifol.rnly around the circumference of the probe. These four tappings asc interconnected.

This gives a cross section-averaged pressure

along the periphery, used for

measurement. To

n~ini~nize the size of tlie probe, these static pressure tappings are located

as near as possible

to

the curved axial symmetric head of the probe.

Due to

cur\;~ture of

the

strciimlines

in

this rcgion the static prcssure at the surfzce falls somewhat

short of its clcs~red

magnitude

in the undisturbed flow. This can be compensntcd either by a sufficient

d~rraocc

of

the

tappings from probe 1ie;id or b) thc artificial impact prcssure on the front facc ofad

:t\i.~lly

movable

rlns

(see Fig

9.4.1

c).

Thc correct position of rhc ring depends on the ~eynol

numbcr

of

tlic

flon

[9.72;

9.741.

-

Limits for the si7e of

the

probe and its tappings: The diameter of thc probe

can

reduced down to

5

rnm. This takes account of the capillarity effects of too small holes,

t

limitation of me;~surinp time and the limits of resistance i11 the tubes connecting

t

tappings wit11 tlie n~nnonieter.

A

prcssure port or tapping should be as small

as

possi

However with rcspcct to surface tension and pipe friction it should not be less

t

0.3

mln in di;~nictt.r.

\\'hen

the ports arc con~iecfed with micro semiconductor probes

t

diameter

of

the prbbe's head is limited by the actual smallest diameter of

2

mm

of

su

Raabe J. Hydro power - the design, use, and function of hydromechanical, hydraulic, and electrical еquipment

Подождите немного. Документ загружается.