Pump Handbook by Igor J. Karassik, Joseph P. Messina, Paul Cooper, Charles C. Heald

Подождите немного. Документ загружается.

9.14.2 NUCLEAR PUMP SEISMIC QUALIFICATIONS 9.311

DOCUMENTATION ____________________________________________________

Refer to IEEE 344 for a list of documents that might be required for dynamic qualification

of the pump-motor assembly (by test or analysis) and for general guidance in preparing

the pump assembly purchase specification.

FURTHER READING __________________________________________________

U.S. Nuclear Regulatory Commission guides (available from NRC, Washing-

ton, D.C.).

Regulatory Guide 1.100, “Seismic Qualification of Electrical Equipment for Nuclear Power

Plants.”

Regulatory Guide 1.61, “Damping Values for Seismic Design of Nuclear Power Plants.”

Regulatory Guide 1.70, “Standard Format and Content of Safety Analysis Reports of

Nuclear Power Plants.”

Regulatory Guide 1.89, “Qualification of Class 1E Equipment for Nuclear Power Plants.”

Regulatory Guide 1.92, “Combining Modal Responses and Spatial Components in Seismic

Response Analysis.”

Industry Standards (Available from the Institute of Electrical and Electronics

Engineers, New York.)

IEEE 323, “Qualifying Class 1E Equipment for Nuclear Power Generating Stations.”

IEEE 344, “IEEE Recommended Practices for Seismic Qualification of Class 1E Equip-

ment for Nuclear Power Generating Stations.”

Other Another source that may be helpful comes from the American Society of

Civil Engineers.

American Society of Civil Engineers. “Structural Analysis and Design of Nuclear Plant

Facilities.” Manuals and Reports on Engineering Practices, No. 58, New York, 1980.

ROBERT H. GLANVILLE

KEN W. TAYLOR

9.313

SECTION 9.15

METERING

METERING OR PROPORTIONING _______________________________________

Conventional reciprocating pumps can be adapted to function as metering or proportion-

ing devices in the transfer of liquids. The principal adaptation is the addition of a means

of varying the pumping rate and predicting what that rate will be, which makes the mod-

ified units suitable for use as final control elements in continuous-flow processes. Metering

pumps are often employed where two or more liquids must be proportioned or where mix-

ture ratios must be controlled.These effects are achieved by changing the displacement per

stroke (by moving the crankpin by special linkages or by partial stroking) or by changing

the stroking speed through the use of variable-speed transmissions or electric motors.

Accurate cyclic volume compensation can also be performed using electronic calibration.

Four basic types of positive displacement reciprocating pumps, and several variations,

are used for this service: packed plunger pumps, pumps with a mechanically actuated

diaphragm, pumps with a hydraulically actuated diaphragm, and pumps with hydrauli-

cally actuated pistons.

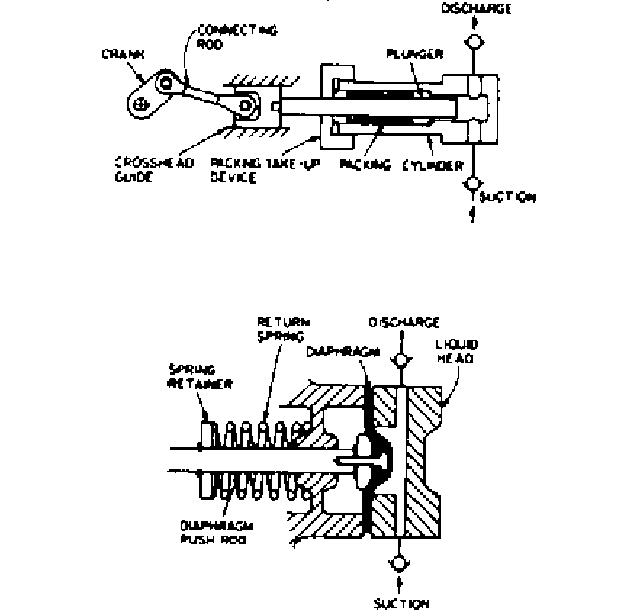
Packed Plunger The packed plunger pump is the most commonly used type because of

its relatively simple design and wide range of pressure capability. It is an adaptation of

the conventional reciprocating transfer pump (Figure 1). Its advantages are

1. Relatively low cost

2. Pressure capability to 50,000 lb/in

(345 MPa) gage

3. Mechanical simplicity

4. Wide capacity range, from a few cubic centimeters per hour to 20 gpm (4.5 m

/h)

5. High accuracy, better than 1% over a 15:1 range

6. Only slightly affected by changes in discharge pressure

9.314 CHAPTER NINE

FIGURE 1 Packed plunger pump

FIGURE 2 Mechanically actuated diaphragm pump

Its disadvantages are

1. Packing leakage, making it unsuitable for corrosive or dangerous chemicals

2. Packing and plunger wear and the resulting need for gland adjustment

3. Inability to pump abrasive slurries or chemicals that crystallize

Mechanically Actuated Diaphragm The mechanically actuated diaphragm pump is

commonly used for low-pressure service where freedom from leakage is important. This

pump utilizes an unsupported diaphragm, which is moved in the discharge direction by

a cam and returned by a spring (Figure 2). Its advantages are

1. Relatively low cost

2. Minimum maintenance at 6- to 12-month intervals

3. Zero chemical leakage

4. Ability to pump slurries and corrosive chemicals

Its disadvantages are

9.15 METERING 9.315

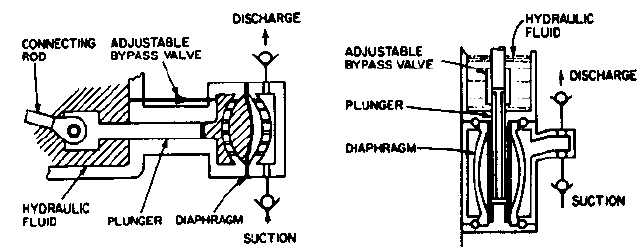
FIGURE 3 Hydraulically actuated diaphragm pump

with flat, circular diaphragm

FIGURE 4 Hydraulically actuated diaphragm pump

with tubular diaphragm

1. Discharge pressure limitation of 125 to 150 lb/in

(860 to 1030 kPa) gage

2. Accuracy in 5% range and as much as 10% zero shift with change from minimum to

maximum discharge pressure

3. Capacity limit of 12 to 15 gph (0.045 to 0.057 m

/h)

Hydraulically Actuated Diaphragm The hydraulically actuated diaphragm pump is a

hybrid design that provides the principal advantages of the other types.A packed plunger

is used to pulse hydraulic oil against the back side of the diaphragm. The reciprocating

action thus imparted to the diaphragm causes it to pump in the normal manner without

being subjected to high pressure differences. A flat diaphragm design is shown in Figure

3 and a tubular diaphragm design in Figure 4.

The advantages of the hydraulically actuated diaphragm pump are

1. Pressure capability to 5000 lb/in

(34.5 MPa) gage

2. Capacities to 20 gpm (4.5 m

/h)

3. Minimum maintenance

4. Zero chemical leakage

5. Ability to pump slurries and corrosive chemicals

6. Accuracy around 1% over 10:1 range

Its disadvantages are

1. Subject to predictable zero shift of 3 to 5% per 1000 lb/in

(6.9 MPa) gage

2. Higher cost

Hydraulically Actuated Pistons This type of design is used when high accuracy at rel-

atively low pressure is required. Figure 5 shows the complete pumping and metering unit,

whereas Figure 6 shows a sectioned view of the metering unit. The pumping unit shown

in Figure 6 can be a gear or vane type, driven by an electric motor. This is integral to a

dual-measuring unit (Figure 5), mounted on top of the pumping unit. Alternatively, a cen-

trifugal pump located remotely in the product storage tank can provide the pumping func-

tion. The pumping unit is run at a constant speed of about 850 rpm. A bypass valve in the

pumping unit varies the output from 0 to 100% in response to a control valve located

downstream of the meter.

The metering unit shown in Figure 5 is a dual unit providing two separate measured

outputs. Each meter unit has two pistons and three chambers. Scotch yokes that drive a

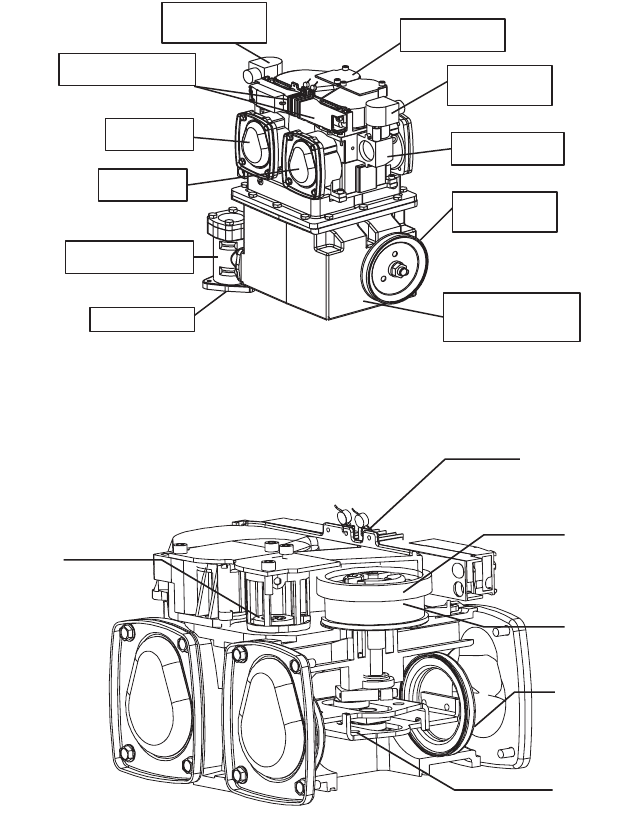
9.316 CHAPTER NINE

Pulse Transmitter

Check Valves

Flow Control

Valve #1

Flow Control

Valve #2

Motor Drive

Pulley

Strainer/Filter

Discharge #1

Gear Pumping

Unit with bypass

Meter #1

Meter#2

Suction Inlet

FIGURE 5 “Duplex” metering pump (Dresser-Wayne)

Pulse

Transmitter

Magnet

Wheel

Timing

Valve

Piston

Cups

Piston

Yokes

Check

Valve

FIGURE 6 “Duplex” meter (Dresser-Wayne)

rotary valve that ports the fluid in and out of the measuring chambers connect the pistons.

A shaft encoder and calibration unit is integral to the meter to provide accurate adjust-

ment of the pumped volume together with batch and running volume totals. The advan-

tages are

1. High accuracy (0.25%) across complete flow range to 25 gpm (6 m

/h)

2. Compact, totally integrated multi-function design.

9.15 METERING 9.317

3. Minimum maintenance every 1.3

—

1.5 million gallons (5000

—

6000 m

)

4. Low initial and ownership cost

Its disadvantages are

1. Pressure limitation of 100 lb/in

(700 kPa).

2. Maximum flow rate of approximately 25 gpm (6 m

/h)

3. Only suitable for low viscosity noncorrosive products.

CAPACITY CONTROLS ________________________________________________

There are five methods commonly used to adjust the capacity of metering pumps. The

choice of which method to use is determined by the application for which the pump is

intended.

Manually Adjustable While Stopped This control is generally found on packed plunger

pumps of conventional design. Capacity changes are effected by moving the crankpin in

or out of the crank arm while the pump is not in motion. This is the least expensive

method and is used where frequent changes in pump displacement are not required.

Manually Adjustable While Running This feature is most frequently found on

mechanically actuated diaphragm pumps, where it is accomplished by limiting the return

stroke of the diaphragm with a micrometer screw. On packed plunger pumps, stroke

adjustment while the pump is running is relatively complicated. Stroke length is set by

some type of adjustable pivot, compound linkage, or tilting plate, which is manually posi-

tioned by turning a calibrated screw. On hydraulically actuated diaphragm pumps, rela-

tively simple control is provided by manually adjustable valving that changes the amount

of the intermediate liquid bypassed at each stroke.

Pneumatic Meeting pumps used in continuous processes must be controlled automat-

ically. In pneumatic systems, the standard 3- to 15-lb/in

(21- to 103-kPa) gage air signal

is utilized to actuate air cylinders of diaphragms directly connected to the stroke-adjusting

mechanism.

Electric On electric control systems, stroke adjustment is through electric servos that

actuate the mechanical stroke-adjusting mechanism. These accept standard electronic

control signals.

Variable Speed This method of adjusting capacity is achieved by driving a reciprocat-

ing pump with a variable-speed prime mover. Because it is necessary to reduce stroke rate

to reduce delivery, discharge pulses are widely spaced when the pump is turning slowly.

Surge chambers or holdup tanks are used when this factor is objectionable.

In control situations involving pH and chlorinization, two variables exist at once; for

example, flow rate and chemical demand. This is easily handled by a metering pump dri-

ven by a variable-speed prime mover. Flow rate can be adjusted by changing the speed of

the pump, and chemical demand by changing its displacement.

CALIBRATION _______________________________________________________

Calibration, which is a fine adjustment of capacity, can be performed in the same way as

the capacity controls previously discussed by using a fine stroke adjustment mechanism.

It can better be achieved using electronics linked to the pumped volume display. A shaft

encoder is connected to the meter that outputs pulses proportional to the volume pumped.

9.318 CHAPTER NINE

The number of pulses is selected relative to the display resolution required. If th of a

liter were required on a nominal cyclic volume of 1 liter, 105 pulses would be provided per

revolution. The pulses are fed into counting and processing electronics.The electronics are

put in calibrate mode and a fixed measure pumped into a calibrated vessel. The electron-

ics counts the number of pulses received for the known volume pumped, compares that to

the number of pulses it should have received, and calculates a calibration factor for that

meter. This factor is stored in nonvolatile memory, and used by counter to “discard” extra

pulses when the pump is in normal delivery mode. The 105 pulses per revolution is used

to make sure that there are always extra pulses to discard allowing a ;5% manufacturing

variation on the nominal cyclic volume.The calibrating electronics can also store batch and

lifetime volumes pumped totals, and supply this information to a local or remote display.

SERVICE AND MAINTENANCE _________________________________________

Proportioning pumps utilizing manual capacity controls can be installed, serviced, and

operated by plant personnel. Pneumatic capacity controls are more sophisticated, but after

their construction and function are understood, maintenance and service should become

routine. Electric capacity controls require a basic understanding of electric circuits for

installation, operation, and routine service. Modular construction allows repair service by

replacement of component groups, thus diminishing the task of trouble-shooting.

All proportioning pumps utilize suction and discharge check valves. These require reg-

ular maintenance and service because they are used frequently, encounter corrosion, and

must be kept in good working order to ensure accurate pump delivery. Service periods are

greatly dependent on liquids pumped, pump operating speed, and daily running time.

Usual service periods run from 30 days to 6 months or longer. Check valves are designed

to facilitate service with a minimum of downtime, thus allowing replacement of wearing

parts at minimum expense. Good design allows this service to be accomplished without

breaking pipe connections to the pump.

Packed plunger pumps require periodic adjustments of the packing takeup device to

compensate for packing and plunger wear. Packing has to be replaced periodically, and reg-

ular lubrication of bearings and wear points is required.

The lubricating oil in speed-reduction gearing, either separate integral units or built

in, must be changed at six-month intervals.

Diaphragm pumps usually require replacement of the diaphragm as part of routine

service at six-month intervals. On hydraulically actuated diaphragm pumps, the

hydraulic fluid must also be changed.

Pneumatic and electric controls generally present no special maintenance problems.

The frequency of routine cleaning and lubrication is dependent on environmental condi-

tions and should be consistent with general plant maintenance procedures.

INSTALLATION_______________________________________________________

Proper installation of metering pumps is very important if reliable pump operation is to

be obtained.

NPSH must be kept as high as possible, and the manufacturer’s recommendations as

to pipe size and length, strainers, relief valves, and bypasses must be observed.

MATERIALS OF CONSTRUCTION _______________________________________

With the tremendous variety of liquid chemicals used in industry today, an all-inclusive

guide to suitability of materials for pump construction is virtually impossible. For the

great majority of common chemicals, pump manufacturers publish data on materials of

100

9.15 METERING 9.319

construction. In general, packed plunger and hydraulically actuated diaphragm pumps

are available as standard construction in mild steel, cast or ductile iron, stainless steel,

and plastic. Mechanically actuated diaphragm pumps are usually available as standard

construction in plastic and stainless steel. Almost any combination of materials can be fur-

nished on special order.

Diaphragms are available as standard construction in Teflon, chemically-resistant

elastomers, and stainless steel from various manufacturers.

See also Section 3.6, “Diaphragm Pumps,” and Section 3.5, “Displacement Pump Flow

Control.”

9.16.1

HYDRAULIC TRANSPORT

OF SOLIDS

KENNETH C. WILSON

ANDERS SELLGREN

9.321

SECTION 9.16

SOLIDS PUMPING

INTRODUCTION______________________________________________________

Applications of Slurry Transport

Vast tonnages are pumped every year in the form of

solid-liquid mixtures, known as slurries. The majority of applications are short-distance

in-plant installations, mainly in the minerals industry. A large plant may contain several

hundred centrifugal pumps handling various types of slurries.

The application that involves the largest quantities is the dredging industry, continually

maintaining navigation in harbors and rivers, altering coastlines and winning material for

landfill and construction purposes.As a single dredge may be required to maintain a through-

put of 7000 tons of slurry per hour or more, very large centrifugal pumps are used with

impeller diameters of over 100 in. (2.5 m). (See Wilson et al., 1997, and Subsection 9.16.2.)

The manufacture of fertilizer is another process involving massive slurry-transport

operations. In Florida phosphate matrix is recovered by huge draglines in open-pit mining

operations. It is then slurried, and pumped to the wash plants through pipelines with a

typical length of about 6 miles (10 km). The total drive capacity can be in excess of 13,400

hp (10 MW).

Recent decades have seen a great increase in the transport of waste materials, in slurry

form, to suitable deposit sites. Waste-disposal environmental problems require that wastes

be conveyed to dedicated and monitored disposal sites, either underground or on the sur-

face.This requirement can often be satisfied by backfilling mines (either deep or open-pit),

and slurry transport is the favored placing method.

Large slurry pumping operations are found in Alberta, Canada, where oil sands

obtained by open-pit mining operations are directly pumped for processing. The mixing

and exposure time during the slurry transportation has eliminated some of the processing

carried out at the processing plant, resulting in large savings. After the extraction opera-

tions are completed, the sand is used as backfill in areas previously mined.

9.322 CHAPTER NINE

Coarse-particle slurries with maximum particle sizes of 4 inches (100 mm) or more can

often be pumped cost-effectively by combining them with smaller particles, to produce a

broad and even particle size distribution (Sellgren & Addie, 1998).

Slurry transportation may play an important role in the development of integrated

mining systems of tomorrow. In mines there is sometimes a considerable inflow of ground-

water that has to be removed.When the mine dewatering installations are integrated with

a hydraulic hoisting system, the cost of power needed to pump out the groundwater can be

excluded from the cost of hoisting in making cost comparisons with other modes of trans-

porting the solids to the surface.The economic effectiveness of hydraulic hoisting, together

with hydraulic design considerations, have been discussed by Kostuik (1965) and Sellgren

et al. (1989) for both small shallow mines and large deep underground mines.

Long-distance slurry pipeline transport is a thoroughly tested and cost-effective mode

of transportation of ores, coal, and industrial minerals. For example, in Brazil up to 13 mil-

lion tons (12 million tonnes) of iron ore concentrate (0.15 mm) have been pumped per year

since 1977 from an inland mine to a pellet plant at the coast 250 miles (400 km) away. The

well-known Black Mesa pipeline in southwestern United States transports a partially

processed slurry coal (1.5 mm) about the same distance to an electrical generating station.

Long distance slurry pipelines involve heads of 75 atmospheres or more at each pump-

ing section, the pumps employed are therefore usually of the positive-displacement type

(see Subsection 9.16.3). Descriptions of long-distance pipelines and associated slurry

pumps can be found, for example, in Brown & Heywood (1991).

Principles of Slurry Flow Slurries are mixtures of solid particles and a liquid (typi-

cally water).The interaction of the solids and the liquid can produce a large variety of flow

behaviors. The major types will be outlined here and described in more detail in subse-

quent sections. The simplest type of slurry behavior occurs, for example, when silt or fine

sand is dredged. It is represented by the “equivalent fluid” model. This evaluates the fric-

tion loss (pressure gradient) in the pipeline on the basis of an equivalent fluid with the

density of the mixture and a friction factor near that of water at the same flow rate. This

simple case is not particularly common in practice. On one hand, there are slurries of finer

particles, e.g., natural clays or industrial materials like red mud. Typically, these are non-

Newtonian in nature, and require rheological techniques to evaluate the pressure gradi-

ent. These will be outlined in the following section on homogeneous slurries.

On the other hand, as particle size or density increases, then settling of particles

becomes significant. In turbulent flow the fluctuating velocity of the turbulent eddies (v¿)

acts against the particle settling velocity (terminal fall velocity v

). The importance of par-

ticle settling increases as the ratio v

/v¿ becomes larger. When settling is significant the

slurry is no longer homogeneous. It is particle-lean near the top of the pipe and particle-

rich near the bottom. In the lower area, contact between particles and the bottom of the

pipe add a granular (Coulombic) shear stress to that produced by the fluid, thus increas-

ing the pressure gradient (for a given flow rate). The solids contributing to this Coulombic

stress are known as “contact load” solids.The stratification ratio, representing the fraction

of total solids that travels as contact load, tends to increase with v

/v¿. The turbulent fluc-

tuating velocity v¿ varies with the mean flow velocity V

(flow rate divided by pD

/4 where

D is the internal pipe diameter). Thus, decreasing V

from an initially large value

increases the stratification ratio, and hence the solids effect on the pressure gradient. With

further reductions in V

the liquid effect on pressure gradient decreases, combining with

the increasing solids effect to produce a minimum in the pressure gradient (for constant

delivered solids concentration). This minimum, shown schematically on Figure 1, was

observed long ago by Blatch (1906).

Calculations for this type of pressure-gradient behavior are outlined in the next section

on settling slurries, as are other features of this type of flow. It is worth noting here that,

as V

is decreased below the minimum-gradient value, there comes a lesser velocity at

which particles at the bottom of the pipe cease moving and form a stationary deposit, also

shown on Figure 1. This deposit leads to a further increase in pressure gradient (and

hence in the power required) and may cause system instability. Beginning with Durand

(1951a, 1951b), deposition has been studied extensively (see the settling slurry section),

primarily so that it can be predicted and hence avoided in engineering design.

Pump Handbook by Igor J. Karassik, Joseph P. Messina, Paul Cooper, Charles C. Heald - 3rd edition

Подождите немного. Документ загружается.