Powers D.L. Boundary Value Problems and Partial Differential Equations
Подождите немного. Документ загружается.


478 Answers to Odd-Numbered Exercises
37. a. u =−
r
2
4
+c
1
ln(r) +c
2
;
b. u =−
(ln(r))
2
2
+c
1
ln(r) +c
2
.
39. V(x, y)
∼
=
a
0
=
1
a
a
0
f (x)dx if y > 5L (because e
−λ
1
.5L
∼
=
0).
41. The solution is θ(X, Y) = 1. The low Biot number, B = 0, means a very
large value for conductivity κ, so very little or no cooling takes place.
Chapter 5
Section 5.1
1.
∂
2
u
∂x
2
+
∂
2
u
∂y
2
=
1
c
2
∂
2
u
∂t
2
,0< x < a,0< y < b,0< t,
u(x, 0, t) = 0, u(x, b, t) = 0, 0 < x < a,0< t,
u(0, y, t) = 0, u(a, y, t) = 0, 0 < y < b,0< t,
u(x, y, 0) = f (x, y),
∂u
∂t
(x, y, 0) = g(x, y),0< x < a,0< y < b.
3.
∂
2
u
∂x
2
+
∂
2
u
∂y
2
+
∂
2
u
∂z
2
=
1
c
2
∂
2
u
∂t
2
.
Section 5.2
1.
c
0
∂
2
u
∂z
2
dz =
∂u
∂z
c
0
=0 by Eq. (12).
3. W
+(2h/bκ)(T
2
−W) = 0, 0 < x < a, W(0) = T
0
, W(a) = T
1
.
W(x) = T
2
+A cosh(µx) + B sinh(µx),whereµ
2
=2h/bκ,
A =T
0
−T
2
, B = (T
1
−T
2
−A cosh(µa))/ sinh(µa).
5. ∇
2
u =
1
k
∂u
∂t
,0< x < a,0< y < b,0< t,
∂u
∂x
(0, y, t) = 0, u(a, y, t) =T
0
,0< y < b,0< t,
u(x, 0, t) = T
0
,
∂u
∂y
(x, b, t) = 0, 0 < x < a,0< t,
u(x, y, 0) = f (x, y),0< x < a,0< y < b.
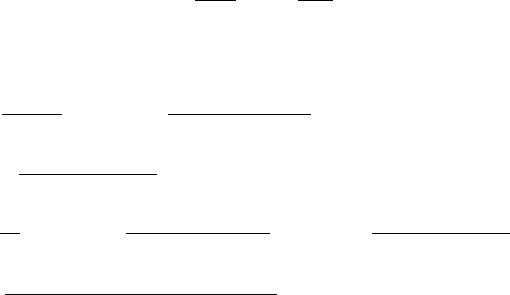
Chapter 5 479
Section 5.3
1. If a = b, the lowest eigenvalues are those with indices (m, n),inthisor-
der: (1, 1); (1, 2) = (2, 1); (2, 2); (3, 1) = (1, 3); (3, 2) = (2, 3); (1, 4) =
(4, 1); (3, 3).
3. Frequencies are λ
mn
c/2π (Hz), where λ
2
mn
are the eigenvalues found in
the text.
5. λ
2
mn
=(mπ/a)
2
+(nπ/b)
2
, for m = 0, 1, 2,..., n = 1, 2, 3,....
7. a. u(x, y, t) = 1.
For b and c the solution has the form
u(x, y, t) =
m,n
a
mn
cos
mπx
a
cos
nπy
b
exp
−λ
2
mn
kt
,
where λ
2
mn
=(mπ/a)
2
+(nπ/b)
2
and m and n run from 0 to ∞.
b. a
00
=
(a +b)
2
, a
m0
=−
2b(1 −cos(mπ))
m
2
π
2
,
a
0n
=−
2a(1 −cos(nπ))
n
2
π
2
, a
mn
=0otherwise;
c. a
00
=
ab
4
, a
m0
=−
ab(1 −cos(mπ))
m
2
π
2
, a
0n
=−
ab(1 −cos(nπ))
n
2
π
2
,
a
mn
=
4ab(1 −cos(nπ ))(1 −cos(mπ))
m
2
n
2
π
4
if m and n are greater than zero.
9. The choice of a positive constant for either X
/X or Y
/Y,underthe
boundary conditions in Eqs. (9) and (10), will lead to the trivial solution.
11. The nodal lines form a grid: u
mn
(x, y, t) = 0atx = 0, a/m,2a/m,...,a
and at y =0, b/n,2b/n,...,b.
Section 5.4
1. The partial differential equations are the same, the boundary conditions
become homogeneous, and in the initial conditions g(r,θ)is replaced by
g(r,θ)−v(r,θ).
3. In the heat problem, T
+λ
2
kT =0.Inthewaveproblem,T
+λ
2
c
2
T =0.
5. The boundary conditions Eqs. (10) and (11) would be replaced by
Q(0) =0, Q(π) = 0.
Solutions are Q(θ ) = sin(nθ), n = 1, 2,....

480 Answers to Odd-Numbered Exercises
7. Taking the hint and using the fact that ∇
2
φ =−λ
2
φ, the left-hand side
becomes
λ
2
k
−λ
2
m
R
φ
k
φ
m
while the right-hand side is zero, because of the boundary condition.
Section 5.5
1. λ
n
=α
n
/a,whereα
n
is the nth zero of the Bessel function J
0
.Thesolutions
are φ
n
(r) = J
0
(λ
n
r) or any constant multiple thereof.
3. This is just the chain rule.
5. Rolle’s theorem says that if a differentiable function is zero in two places,
its derivative is zero somewhere between. From Exercise 4 it is clear that
J
1
must be zero between consecutive zeros of J
0
. Check Fig. 7 and Table 1.
7. Use the second formula of Exercise 6, after replacing µ by µ + 1onboth
sides.
9. u(r) = T +(T
1
−T)I
0
(γ r)/I
0
(γ a).
Section 5.6
1. v(0, t)/T
0
∼
=
1.602 exp(−5.78τ)−1.065 exp(−30.5τ),whereτ =kt/a
2
.
3. v(r, t) =
∞
n=1
a
n
J
0
(λ
n
r) exp(−λ
2
n
kt), λ
n
=α
n
/a.UseEq.(13)andothersto
find a
n
=T
0
J
1
(α
n
/2)/α
n
J
2
1
(α
n
).
5. Integration leads to the equality
a
0
rφ
2
dr +λ
2
a
0
r
2
φ
2
dr =0.
The first integral is evaluated directly. The second must be integrated by
parts.
Section 5.7
1. Use
1
r
d
dr
r
d
dr
J
0
(λr)
=−λ
2
J
0
(λr).
3. The frequencies of vibration are λ
mn
c = α
mn
c/a.Thefivelowestvaluesof
α
mn
, in order, have subscripts (0, 1), (1, 1), (2, 1), (0, 2),and(3, 1).See
Table 1 in Section 5.6.
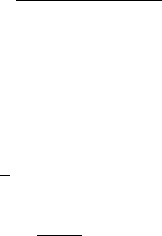
Chapter 5 481
5. φ(a,θ)=0andφ(r,θ)=φ(r,θ +2π).
7. Set J
m
(λ
mn
r) = φ
n
.Then(rφ
n
)
=−λ
mn
rφ
n
and (rφ
q
)
=−λ
mq
rφ
q
are the
equations satisfied by the functions in the integrand. Follow the proof in
Section 2.7.
9. Generally, radii for φ
0n
are r = λ
0m
/λ
0n
for m = 1, 2,...,n.Forn = 2,
r =2.405/5.520 =0.436 and 1.
Section 5.8
1. φ(x) = x
α
[AJ
p
(λx) +BY
p
(λx)], where α = (1 −n)/2, p =|α|.
3. For λ
2
=0, Z = A + Bz.
5. φ(ρ +ct) =
¯
F
o
(ρ +ct) +
¯
G
e
(ρ +ct),
ψ(ρ −ct) =
¯
F
o
(ρ −ct) −
¯
G
e
(ρ −ct),
where
¯
F
o
(x) is the odd periodic extension with period 2a of xf (x)/2and
¯
G
e
(x) is the even periodic extension with period 2a of
(x/2c)g(x)dx.
7. The weight function is ρ
2
and the interval is 0 to a.
9. v(x) = (b −x)(x − a)/(a + b)x
2
.
11. No. The idea is to find a solution of the partial differential equation that
depends on only one variable. That is impossible if f depends on both x
and y.
13. a
n
=−
b
a
v(x)X
n
(x)x
3
dx
b
a
X
2
n
(x)x
3
dx
.
Section 5.9
1. [k(k +1) −µ
2
]a
k+1
−[k(k −1) −µ
2
]a
k−1
=0, valid for k = 1, 2,....
3. P
5
=
1
8
63x
5
−70x
3
+15x
.
5. y = A ln
1 +x
1 −x
.
7. Differentiate Eq. (9) and add to it n times Eq. (8).
9. Leibniz’s rule states that
(uv)
(k)
=
k
r=0
k
r
u
(k−r)
v
(r)
.

482 Answers to Odd-Numbered Exercises
(A superscript k in parentheses means kth derivative.) The right-hand
side looks like the binomial theorem. In the case at hand, at most two
terms are not zero.
11. b
n
=
0(n odd),
−(−1)
n/2
(2n +1)
(n +2)(n −1)
1 ·3 ·5 ···(n −1)
2 ·4 ·6 ···n
(n even).
Section 5.10
1. The solution is as given in Eq. (5) with coefficients as shown in Eq. (7).
The integration yields (see Section 5.9)
b
0
=
1
2
; b
n
=0 for n even; b
1
=3/4and
b
n
=
(−1)
(n−1)/2
2 ·c
n
1 ·3 ·5 ···(n −2)
2 ·4 ·6 ···(n −1)
·
2n +1
n +1
, n = 3, 5, 7,....
3. u(φ, t) = T −
b
n
P
n
(cos(φ)) exp(−(µ
2
+n(n + 1))kt/R
2
),whereµ
2
=
γ
2
R
2
, n is odd, and b
n
is as at the end of Part B, with T
0
=T.
5. The eigenfunctions are as in Part C, except that n must be odd in order to
satisfy the boundary condition.
7. The nodal surfaces are: a sphere at ρ =0.634 and two naps of a cone given
by φ =0.304π and ρ = 0.696π .
Chapter 5 Miscellaneous Exercises
1. u(x, y, t) =
∞
m=1
a
m
sin(µ
m
y) exp
−µ
2
m
kt
+
∞
n=1
a
mn
cos(λ
n
x) sin(µ
m
y) exp
−
µ
2
m
+λ
2
n
kt
,
µ
m
=mπb, λ
n
=nπ/a,
a
m
=T
1 −cos(mπ)
mπ
, a
mn
=
4T
π
3
(cos(nπ)−1)(1 − cos(mπ))
n
2
m
.
3. u(a/2, b/2, t) =
∞
n=1
b
mn
sin
nπ
2
sin
mπ
2
exp
−
λ
2
n
+µ
2
m
kt
,
where λ
n
=nπ/a, µ
m
=mπ/b,and
b
mn
=
4T
π
2
(1 −cos(mπ ))(1 −cos(nπ))
mn
.

Chapter 5 483
The first three nonzero terms are, for a = b, those with (m, n) =
(1, 1), (1, 3) = (3, 1), (3, 3). All terms with an even index are 0.
u(a/2, a/2, t)
∼
=
16T
π
2
e
−2τ
−
2
3
e
−10τ
+
1
9
e
−18τ
,
where τ =ktπ
2
/a
2
.
5. u(r) =
a
2
−r
2
/2andu(r) =
∞
1
C
n
J
0
(λ
n
r), with C
n
=
2a
2
α
3
n
J
1
(α
n
)
.
7. w(x, t) = a
0
+
∞
n=1
a
n
cos(λ
n
x) exp
−λ
2
n
kt
,
v(y, t) =
b
m
sin(µ
m
y) exp
−µ
2
m
kt
,
where µ
m
=mπ/b, λ
n
=nπ/a, and initial conditions are
v(y, 0) =1, 0 < y < b; w(x, 0) =Tx/a, 0 < x < a.
9. J
0
(λr) exp(−λ
2
kt).
11. B
k
=b
k
/k(k + 1) for k = 1, 2,...; b
0
must be 0, and B
0
is arbitrary.
13.
1 −x
2
y
−
m
2
1 −x
2
y +µ
2
y = 0.
15. u(r, z) =
∞
n=1
a
n
sinh(λ
n
z)
sinh(λ
n
b)
J
0
(λ
n
r),
where λ
n
=
α
n
a
and a
n
=
2U
0
α
n
J
1
(α
n
)
.
17. u(r, z, t) = sin(µz)J
0
(λr) sin(νct) is a product solution if µ = mπ/b, λ =
α
n
/a,andν =
µ
2
+λ
2
. The frequencies of vibration are therefore νc
or
c
mπ
b
2
+
α
n
a
2
.
19. Each of the two terms satisfies ∇
2
φ =−(5π
2
)φ.Ony = 0andx = 1,
both terms are 0; on y = x they are obviously equal in value, opposite in
sign.
21. Each term satisfies ∇
2
φ =−(16π
2
/3)φ.
On y =0, φ =sin(2nπx) −sin(2nπx);
on y =
√
3x , φ =sin(4nπx) +0 −sin(2nπ ·2x);
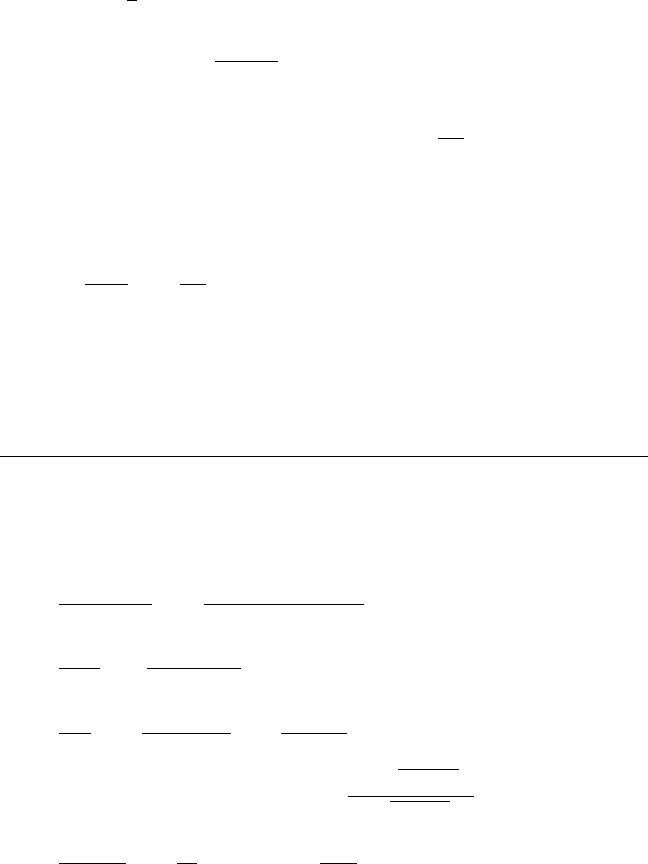
484 Answers to Odd-Numbered Exercises
on y =
√
3(1 −x), φ =sin(4nπ x) +sin(2nπ(1 −2x)) −sin 2nπ.
23. For a sextant, φ
n
= J
3n
(λr) sin(3nθ) and J
3n
(λ) = 0. Thus λ
1
= 6.380,
which is less than
16π
2
/3 =7.255.
25. b =−1.
27. Since y(x) = hJ
0
(kx)/J
0
(ka),wherek = ω/
√
gU, the solution cannot
have this form if J
0
(ka) = 0.
29. u(r, t) = R(t)T(t); R(r) = r
−m
J
m
(λr),wherem = (n − 2)/2; T(t) =
a cos(λct) +b sin(λct).
31. b =
D
r
L
2
D
z
R
2
, ρ =
UL
D
z
.
33. a
0
=12.77, a
1
=−4.88.
35. tan(λ) = Dλ/(D +λ
2
), λ = π (D = 0), 3.173 (D = 1), 4.132 (D = 10).
Chapter 6
Section 6.1
1. c.
s
2
+2ω
2
s(s
2
+4ω
2
)
;d.
ω cos(φ) −s sin(φ)
s
2
+ω
2
;
e.
e
2
s −2
;f.
2ω
2
s(s
2
+4ω
2
)
.
3. a.
e
−as
s
;b.
e
−as
−e
−bs
s
;c.
1 −e
−as
s
2
.
5. a. e
−t
sinh(t);b.e
−t
cos(t);c.e
−at
sin(
√
b
2
−a
2
t)
√
b
2
−a
2
.
7. a.
e
at
−e
bt
a −b
;b.
t
2a
sinh(at);d.
t
2
e
at
2
;
e. f (t) =
1, 0 < t < 1,
0, 1 < t.
9. a. [sin(ωt) − ωt cos(ωt)]/2ω
2
;
b. See Table 2;
c. See 7c;
d. [cos(ωt) − ωt sin(ωt)]/2.

Chapter 6 485
Section 6.2
1. a. e
2t
;b.e
−2t
;c.
3e
−t
−e
−3t
2
;d.
sin(3t)
3
.
3. a.
1 −e
−at
a
;b.t −sin(t);c.
sin(t) −
1
2
sin(2t)
3
;
d. (sin(2t) −2t cos(2t))/16; e. −
3
4
+
1
2
t +e
−t
−
1
4
e
−2t
;f.cosh(t) −1.
5. a.
e
2t
−e
−2t
4
;b.
1
2
sin(2t);
c.
3
2
+
i
√
2 −3
4
exp
−i
√
2t
−
i
√
2 −3
4
exp
i
√
2t
;d.4
1 −e
−t
.
7. a. 1 −cos(t);b.
e
t
−cos(ωt) +ω sin(ωt)
ω
2
+1
;c.t −sin(t).
Section 6.3
1. a. s =−
2n −1
2
π
2
, n = 1, 2,...;
b. s =±i
2n −1
2
π, n = 1, 2,...;
c. s =±inπ , n = 0, 1, 2,...;
d. s = iη,wheretanη =
−1
η
;
e. s = iη,wheretanη =
1
η
.
3. a.
sinh(
√
sx)
s
2
sinh(
√
s)
;b.
1
s
−
cosh
√
s(
1
2
−x )
s(s +1) cosh(
√
s/2)
.
5. a. u(x, t) = x +
∞
n=1
2sin(nπ x)
nπ cos(nπ)
exp
−n
2
π
2
t
;
b. u(x, t) is 1 minus the solution of Example 3.
Section 6.4
1. t +
x
2
2
.
3. v(x, t) =
4
π
2
∞
1
cos
(2n −1)(
1
2
−x )
sin((2n −1)π t)
(2n −1)
2
sin
2n−1
2
π
.
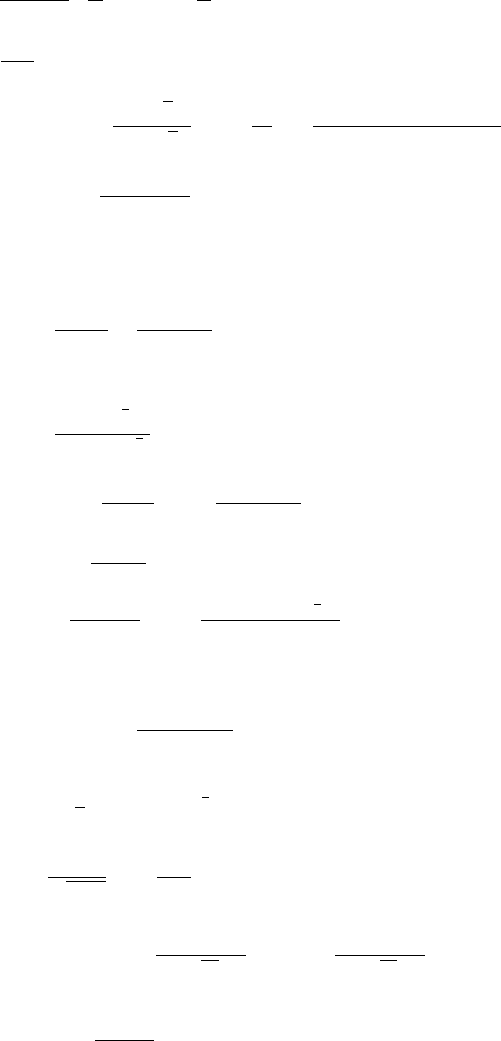
486 Answers to Odd-Numbered Exercises
5. a.
ω
ω
2
−π
2
1
π
sin(πt) −
1
ω
sin(ωt)
sin(πx);
b.
1
2π
2
sin(πt) − πt cos(πt)
sin(πx).
7. a. u(x, t) = x −
sin(
√
ax)
sin(
√
a)
e
−at
+
2a
π
∞
1
sin(nπx) exp(−n
2
π
2
t)
n(a −n
2
π
2
) cos(nπ)
;
b. The term −
x cos(nπx)
cos(nπ)
exp
−n
2
π
2
t
arises.
Chapter 6 Miscellaneous Exercises
1. U(s) =
T
0
γ
2
+s
+
γ
2
T
s(γ
2
+s)
,
u(x, t) = T
0
exp
−γ
2
t
+T
1 −exp
−γ
2
t
.
3. U(s) =
cosh(
√
sx)
s
2
cosh(
√
s)
,
u(x, t) = t −
1 −x
2
2
+
∞
n=1
2cos(ρ
n
x)
ρ
3
n
sin(ρ
n
)
exp
−ρ
2
n
t
,
where ρ
n
=
(2n−1)π
2
.
5. u(x, t) =
x(1 −x)
2
−
∞
n=1
4cos
ρ
n
(x −
1
2
)
ρ
n
sin(ρ
n
/2)
exp
−ρ
2
n
t
,
where ρ
n
=(2n −1)π .
7. u(x, t) = x +
∞
1
2sin(nπ x)
nπ cos(nπ)
exp
−n
2
π
2
t
.
9. U(x, s) =
1
s
1 −exp
−
√
sx
.
11. f (t) =
x
√
4πt
3
exp
−x
2
4t
.
13. u(x, t) =
∞
n=0
erfc
2n +1 −x
√
4t
−erfc
2n +1 +x
√
4t
.
15. F(s) = 2
∞
n=1
1
s
2
+n
2
.
Chapter 7 487
17. f (t) =
∞
−∞
1
2a
G
inπ
a
e
inπt/a
.
19. F(s) must be of the form F(s) =G(s)/H(s),whereG(s) is never infinite.
The solutions of H(s) = 0 must form an arithmetic sequence of purely
imaginary numbers, and H
(s) =0ifH(s) = 0.
21. F(s) =
(1 −e
−πs
)
2
s(1 −e
−2πs
)
=
1 −e
−πs
s(1 +e
−πs
)
.
23. F(s) =
1 +e
−πs
(s
2
+1)(1 − e
−πs
)
.
25. u(x, t) =
sin(ωx) sin(ωt)
sin(ω)
+
∞
n=1
(−1)
n+1
2ω
ω
2
−n
2
π
2
sin(nπx) sin(nπ t).
27. U(x, s) =
ω
s
2
+ω
2
e
mx
, m =
1
2
−
1
4
+s.
29. α
2
=
1
2
1
4
±
1
4
2
+ω
2
.Sinceα must be real, take the + sign.
Chapter 7
Section 7.1
1. 16(u
i+1
− 2u
i
j + u
i−1
) =−1, i = 1, 2, 3, u
0
= 0, u
4
= 1. Solution: u
1
=
11/32, u
2
=5/8, u
3
=27/32.
3. 16(u
i+1
− 2u
i
+ u
i−1
) − u
i
=−
1
2
i, i = 1, 2, 3, u
0
= 0, u
4
= 1. Solution:
u
1
=0.285, u
2
=0.556, u
3
=0.800.
5. 16(u
i+1
− 2u
i
+ u
i−1
) =
1
4
i, i = 0, 1, 2, 3, u
0
− 2(u
1
− u
−1
) = 1, u
4
= 0.
Solution: u
0
=0.422, u
1
=0.277, u
2
=0.148, u
3
=0.051.
7. n = 3: u
1
= 4.76, u
2
= 4.24; n = 4: u
1
= 6.65, u
2
= 9.14, u
3
= 5.92. The
actual solution,
u(x) =−
sin(
√
10x)
sin(
√
10)
,
has a maximum of about 50. The boundary value problem is nearly
singular.
