Pecharsky V.K., Zavalij P.Y. Fundamentals of Powder Diffraction and Structural Characterization of Materials

Подождите немного. Документ загружается.

652

Chapter 7

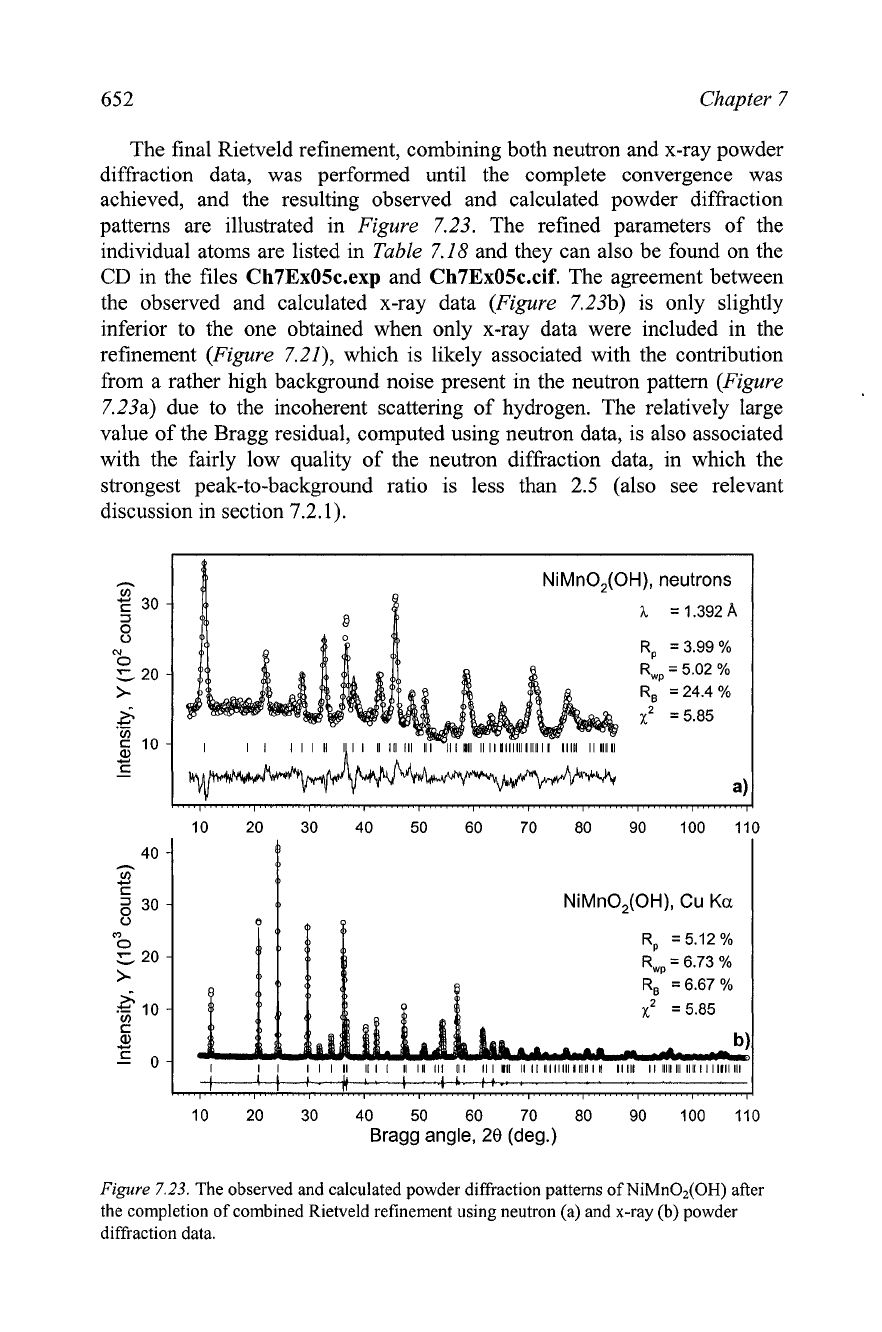
The final Rietveld refinement, combining both neutron and x-ray powder

diffraction data, was performed until the complete convergence was

achieved, and the resulting observed and calculated powder diffraction

patterns are illustrated in Figure 7.23. The refined parameters of the

individual atoms are listed in Table 7.18 and they can also be found on the

in the files

Ch7ExOSc.exp

and

Ch7ExOSc.cif.

The agreement between

the observed and calculated x-ray data (Figure 7.23b) is only slightly

inferior to the one obtained when only x-ray data were included in the

refinement (Figure

7.21), which is likely associated with the contribution

from a rather high background noise present in the neutron pattern (Figure

7.23a) due to the incoherent scattering of hydrogen. The relatively large

value of the Bragg residual, computed using neutron data, is also associated

with the fairly low quality of the neutron diffraction data, in which the

strongest peak-to-background ratio is less than

2.5

(also see relevant

discussion in section

7.2.1).

NiMnO,(OH),

neutrons

1.392

10 20 30 40 50 60 70 80 90 100 110

Bragg angle,

(deg.)

Figure

7.23.

The observed and calculated powder diffraction patterns of NiMn02(OH) after

the completion of combined Rietveld refinement using neutron (a) and x-ray (b) powder

diffraction data.

Crystal structure refinement

The population parameter of hydrogen has been refined to a value of

62(5)

and, therefore, the chemical composition of the material is

NiMn03H8, or NiMn03-6(OH)6, where

0.62(5). Hence, a fraction of the

Mn atoms should be in the 4+ oxidation states. The latter was confirmed by

the magnetic susceptibility measurements. The preferred orientation

parameters, refined for both preferred orientation axes,

i.e. [O 101 and [loo],

are 0.74 and 1.40, respectively, resulting in the texture factors ranging

between 0.52 and 2.10, which corresponds to the preferred orientation

magnitude of about

It appears that all pieces of this crystallographic puzzle are now in place

and they agree both with each other and with all available information.

These are: the chemical composition and the oxidation states of the metal

atoms; the crystal structure in general, including the distribution of Mn and

Ni atoms, and the amount and positions of hydrogen atoms; geometry, which

includes bond lengths, coordination polyhedra and hydrogen bonding; and

basic magnetic properties, which confirm a mixture of

Mn3' and Mn4+ in the

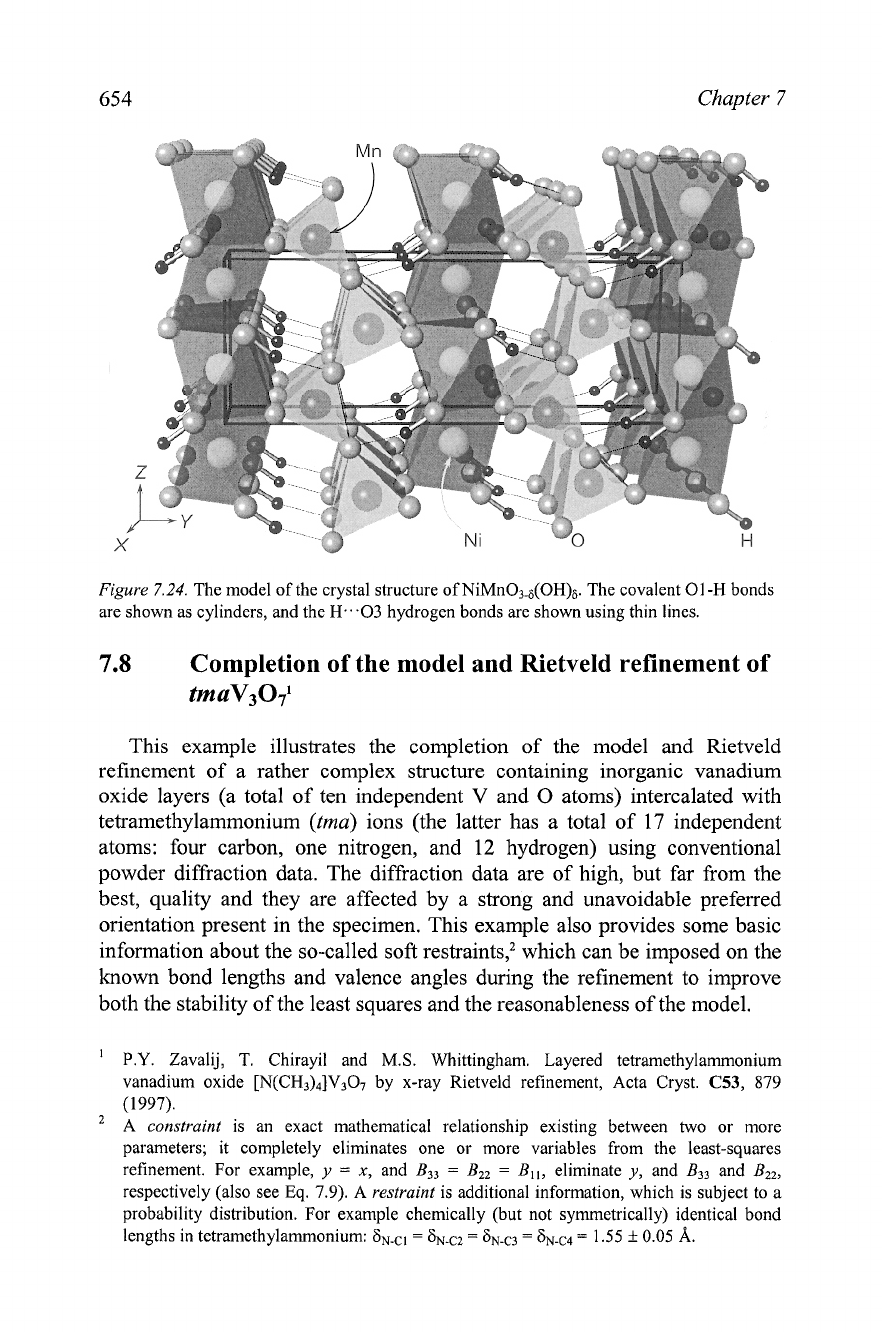
material. Thus, the fully determined and refined crystal structure of

NiMn03-6(OH)6 makes reasonable chemical and physical sense and its

complete model is illustrated in

Figure

7.24.

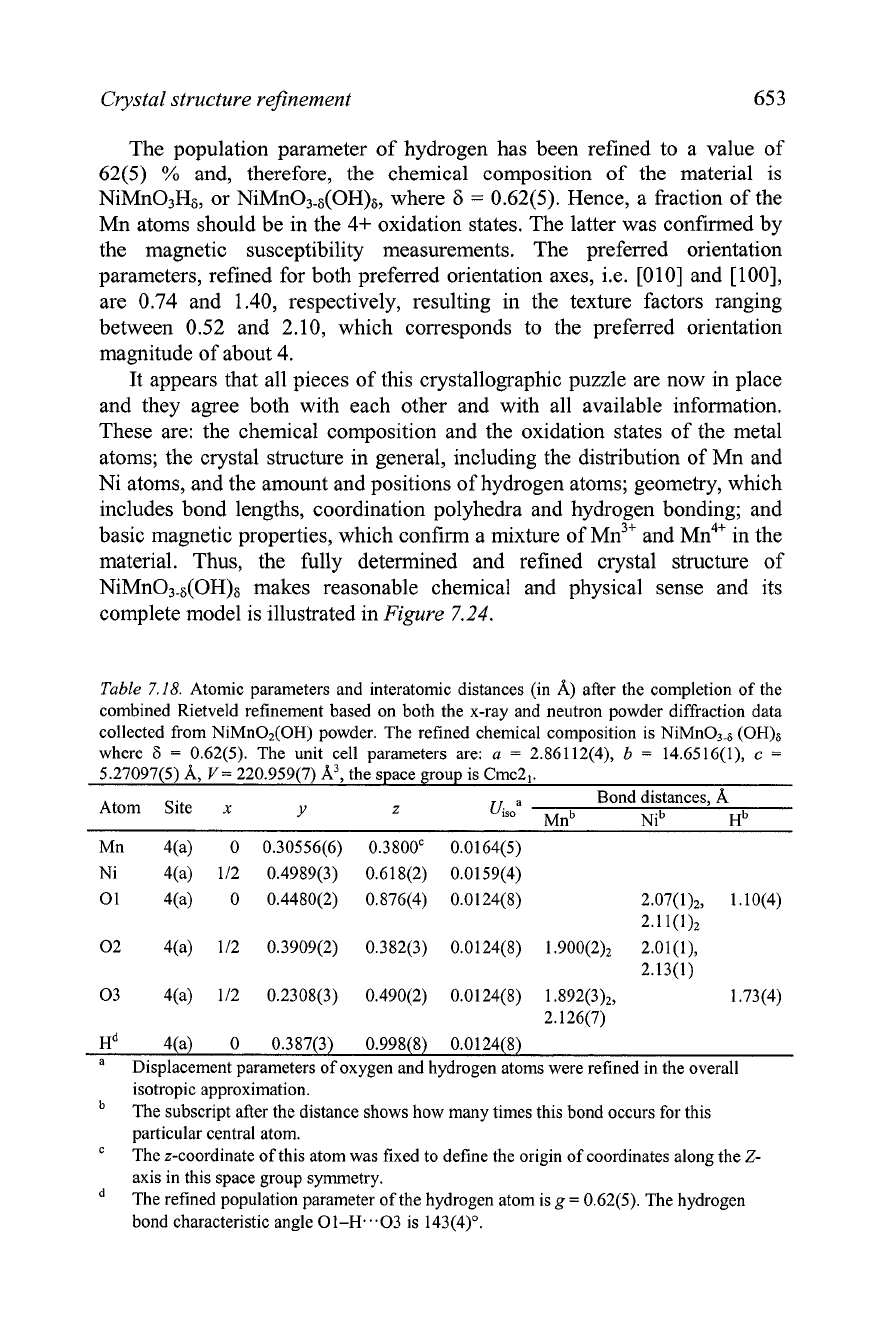
Table

7.18.

Atomic parameters and interatomic distances (in A) after the completion of the

combined Rietveld refinement based on both the x-ray and neutron powder diffraction data

collected from NiMn02(OH) powder. The refined chemical composition is NiMn03.6 (OH)6

where

0.62(5). The unit cell parameters are:

2.86112(4),

14.6516(1),

5.27097(5) A,

220.959(7) A3, the space group is Cmc2,.

Atom Site

Bond distances, 8,

Uisoa

Mnb

~i~

Mn 4(a) 0 0.30556(6) 0.3800' 00.0164(5)

Ni 4(a) 112 0.4989(3) 0.618(2) 0.0159(4)

01 4(a) 0 0.4480(2) 0.876(4) 0.0124(8) 2.07(1),, 1.10(4)

2.1 1(1)2

02 4(a) 112 0.3909(2) 0.382(3) 0.0124(8) 1.900(2)2 2.01(1),

2.13(1)

03 4(a) 112 0.2308(3) 0.490(2) 0.0124(8) 1.892(3),, 1.73(4)

2.126(7)

4(a) 0 0.387(3) 0.998(8) 0.0124(8)

Displacement parameters of oxygen and hydrogen atoms were refined in the overall

isotropic approximation.

The subscript after the distance shows how many times this bond occurs for this

particular central atom.

The z-coordinate of this atom was fixed to define the origin of coordinates along the

Z-

axis in this space group symmetry.

The refined population parameter of the hydrogen atom is

0.62(5). The hydrogen

bond characteristic angle Ol-H...03 is 143(4)O.

654

Chapter

Figure

7.24. The model of the crystal structure of NiMn03.8(OH)6. The covalent 01-H bonds

are shown as cylinders, and the H...03 hydrogen bonds are shown using thin lines.

7.8

Completion of the model and Rietveld refinement of

tmaV3071

This example illustrates the completion of the model and Rietveld

refinement of a rather complex structure containing inorganic vanadium

oxide layers (a total of ten independent

and

atoms) intercalated with

tetramethylammonium (tma) ions (the latter has a total of 17 independent

atoms: four carbon, one nitrogen, and

hydrogen) using conventional

powder diffraction data. The diffraction data are of high, but far from the

best, quality and they are affected by a strong and unavoidable preferred

orientation present in the specimen. This example also provides some basic

information about the so-called soft restraints: which can be imposed on the

known bond lengths and valence angles during the refinement to improve

both the stability of the least squares and the reasonableness of the model.

P.Y.

Zavalij,

Chirayil and M.S. Whittingham. Layered tetramethylarnmonium

vanadium oxide [N(CH3)4]V307 by x-ray Rietveld refinement, Acta Cryst.

C53,

879

(1997).

. .

constraint

is an exact mathematical relationship existing between two or more

parameters; it completely eliminates one or more variables from the least-squares

refinement. For example,

and B33

BZ2

BI1, eliminate

and B33 and B22,

respectively (also see

Eq.

7.9).

restraint

is additional information, which is subject to a

probability distribution. For example chemically (but not symmetrically) identical bond

lengths in tetramethylarnmonium: 8N-CI

6N-C3

tiNwC4

1.55

0.05

Crystal structure

refinement

655

The experimental pattern was collected with a 0.02' step from 7 to 50'

29 and a counting time of 60 seclstep, and from 50 to 69O 29 with a counting

time of 90 seclstep. The high Bragg angle intensities were scaled to the 60

seclstep counting time for all calculations. The following initial parameters

were used in the model completion and refinement:

The initial model of the crystal structure was taken from Table

6.40.

The default profile parameters were taken from the instrumental

parameter file

Scintag.prm

(see section 7.6).

The sample shift parameter

8.94 corresponds to the sample

displacement s

-0.195 mm, which was determined together with the

unit cell dimensions during the least squares refinement of lattice

parameters.

The space group is P2Jn and the unit cell dimensions are a

18.482, b

6.5526,

8.4297 8L,

91.103', as determined earlier.

The overall isotropic displacement parameter,

U,,,

0.015 8L2.

The starting model is found on the

in the files

Ch7Ex06a.exp

and

Ch7Ex06a.cif

together with all relevant structural parameters, and the

experimental powder pattern is located in the file

Ch7Ex06-CuKa.raw.

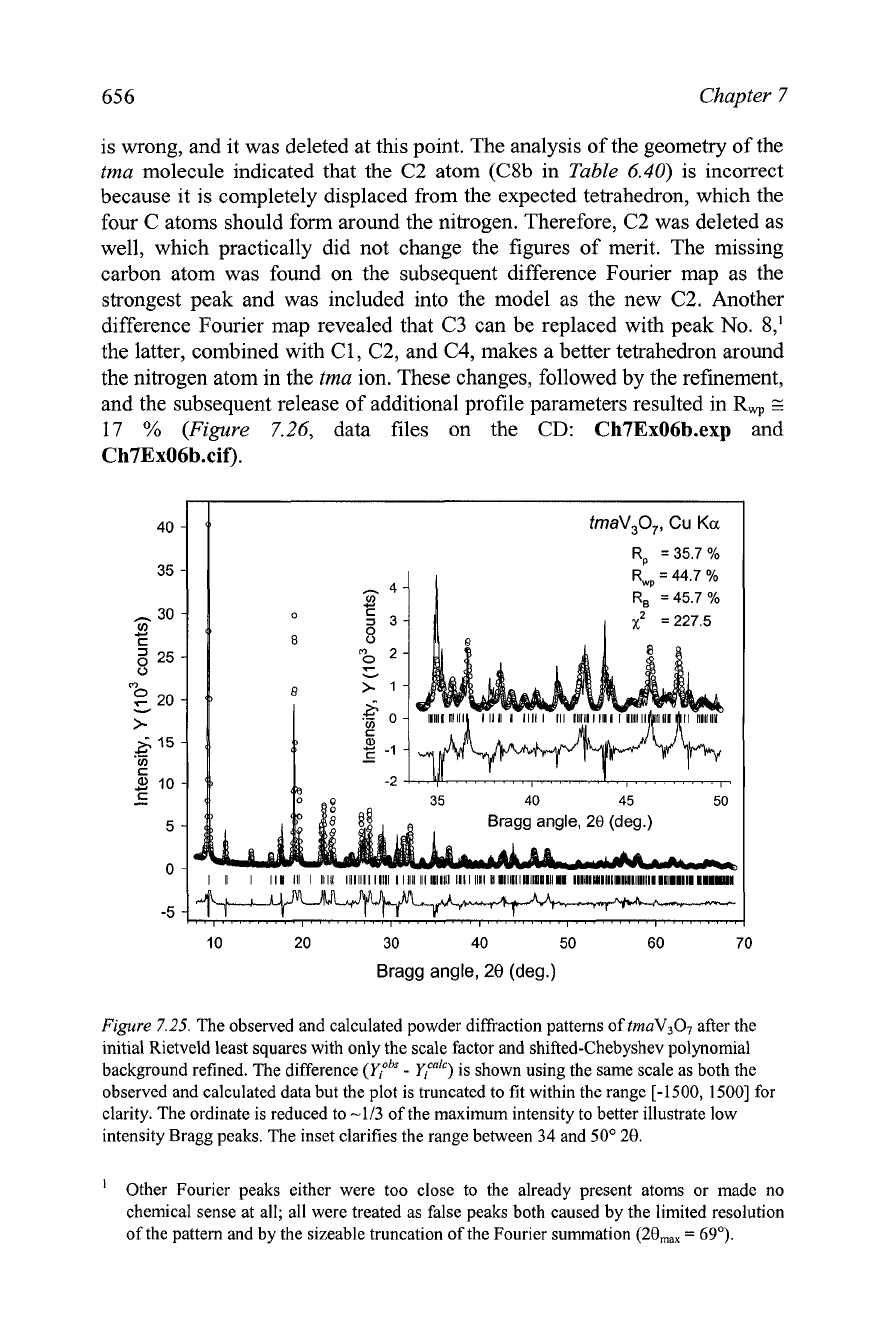
Initially, only the scale factor and 6 coefficients for a shifted-Chebyshev

polynomial approximation of the background were refined. The resulting

residuals, which are shown in the second row of Table 7.19, are higher than

one could expect for a nearly complete model (all non-hydrogen atoms were

thought to be located). As can be seen from the inset in Figure 7.25, both the

calculated intensities and peak shapes are far off from their observed values.

The intensity mismatch may be associated, to a certain extent, with a

considerable preferred orientation, which is expected from the highly

anisotropic shapes of the crystallites (see the inset in Figure 6.26) and nearly

guaranteed easy cleaving of the particles along the planes parallel to the

vanadate layers expanded by the tetramethylammonium. Therefore, the

subsequent refinement included the grain size broadening parameter

(X)

and

the preferred orientation along the [loo] axis, resulting in some improvement

of the fit.

The next refinement step included the unit cell dimensions and peak

asymmetry, noticeably lowering the residuals. At this point, the difference

Fourier map was calculated, which revealed a potential missing atom. The

coordinates of this peak were incorporated into the model as 08. This

addition, together with the refinement of the coordinates and individual

Ui,,

of all atoms, performed in order to perhaps detect erroneously positioned

atoms (one of the eight oxygen atoms is obviously false), substantially

improves the fit, lowering

from -30 to -20

The analysis of individual

Ui,,

(Table 7.20) shows that one of them is extremely high:

U,,,

0.6

8L2

for

07. This atom has a short distance to V1 (0.78

suggesting that its location

656

Chapter 7

is wrong, and it was deleted at this point. The analysis of the geometry of the

tma molecule indicated that the C2 atom (C8b in Table

6.40)

is incorrect

because it is completely displaced from the expected tetrahedron, which the

four C atoms should form around the nitrogen. Therefore, C2 was deleted as

well, which practically did not change the figures of merit. The missing

carbon atom was found on the subsequent difference Fourier map as the

strongest peak and was included into the model as the new C2. Another

difference Fourier map revealed that C3 can be replaced with peak No.

8,'

the latter, combined with C1, C2, and C4, makes a better tetrahedron around

the nitrogen atom in the tma ion. These changes, followed by the refinement,

and the subsequent release of additional profile parameters resulted in

(Figure 7.26, data files on the CD:

Ch7Ex06b.e~~

Ch7Ex06b.cif).

and

10 20 30 40 50 60 70

Bragg angle,

(deg.)

Figure

7.25.

The observed and calculated powder diffraction patterns of tmaV307 after the

initial Rietveld least squares with only the scale factor and shifted-Chebyshev polynomial

background refined. The difference

(Y:~"

yicnlc)

is shown using the same scale as both the

observed and calculated data but the plot is truncated to fit within the range [-1500, 15001 for

clarity. The ordinate is reduced to -113 of the maximum intensity to better illustrate low

intensity Bragg peaks. The inset clarifies the range between 34 and 50"

20.

Other Fourier peaks either were too close to the already present atoms or made no

chemical sense at all; all were treated as false peaks both caused by the limited resolution

of the pattern and by the sizeable truncation of the Fourier summation

(20,,,

69").

Crystal structure refinement

657

Table 7.19. The progress of Rietveld refinement of the crystal structure of tmaV307 from x-

ray powder diffraction data.

Refined parameters RD RWD

Initial (CD:

Ch7Ex06-CuKa.raw, Ch7Ex06a.exp,

and 60.7 68.6 99.4 535

Ch7Ex06a.cif)

Scale, shifted-Chebyshev polynomial background (Figure 7.25)

Plus grain broadening

(X)

and preferred orientation, PO, with

texture axis

001

Plus unit cell dimensions and asymmetry,

Added 08; coordinates and Uindi, included, Table 7.20

Deleted 07 and C2

Added C2 and replaced C3 from a difference Fourier map

(Figure 7.26) (CD:

Ch7Ex06b.e~~

and

Ch7Ex06b.cif)

Strain broadening

(Y),

asymmetry

(a),

and sample shift

Scale, background, and unit cell dimensions: first with porosity

(absorption)

and a2; and then with PO [loo],

sample shift

and overall Uiso

Excluded 8 to 12" 29; PO [Ol 01, then

X,,

Plus coordinates of V and

Plus coordinates of N and C

Plus PO axes ratio, individual Uiso(V), overall Uis,(0) and Uiso

(N, C) (Figure 7.27) (CD:

Ch7Ex06c.e~~

and

Ch7Ex06c.cif)

Plus soft restraints on C-N distances and C-N-C angles (Table

7.21) (CD:

Ch7ExO6d.e~~

and

Ch7ExO6d.cif)

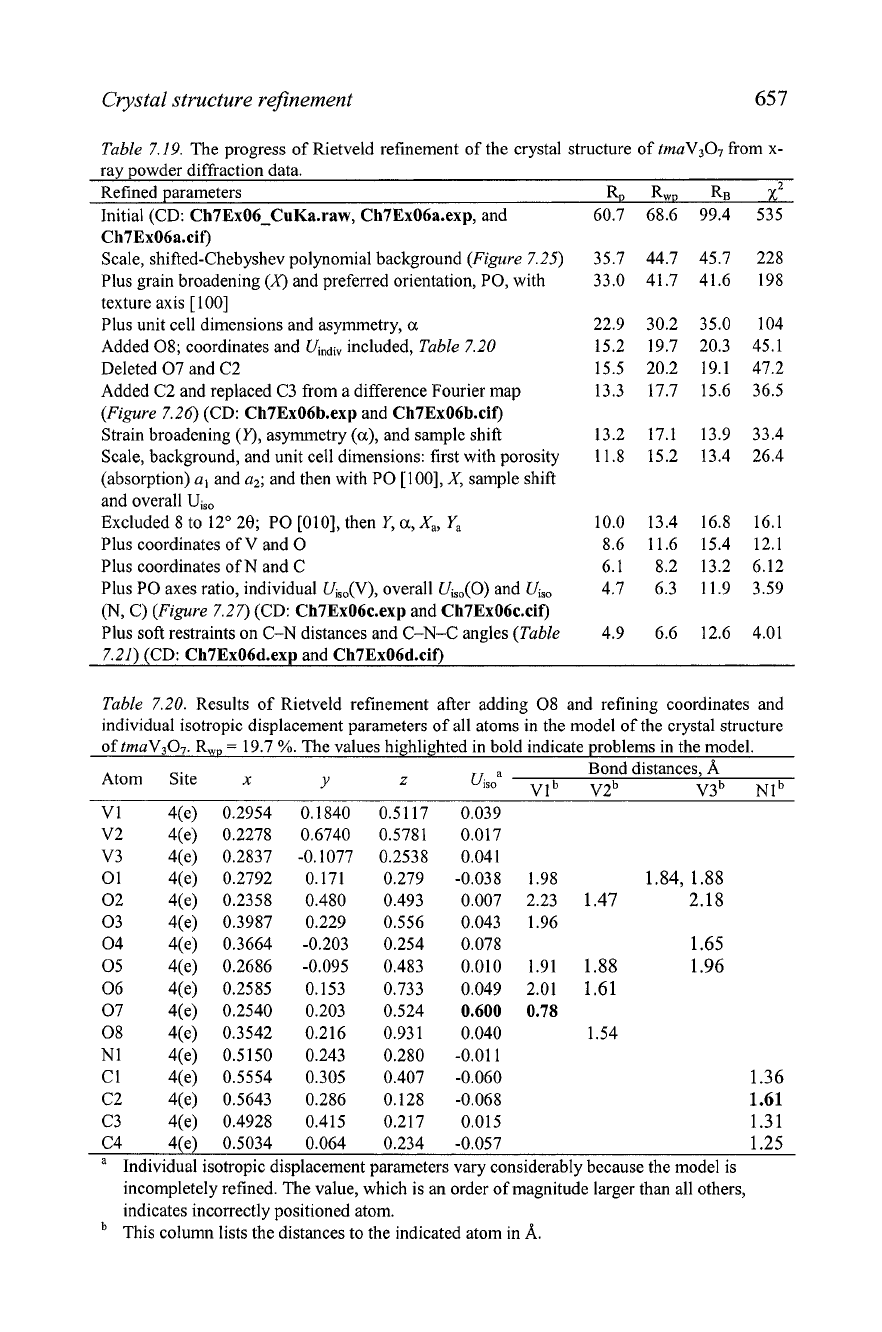
Table 7.20. Results of Rietveld refinement after adding 08 and refining coordinates and

individual isotropic displacement parameters of all atoms in the model of the crystal structure

of tmaV3O7. R,,,,

19.7

The values highlighted in bold indicate problems in the model.

Atom Site

Bond distances,

Ui~~a

V2b

~3' ~1~

VI 4(e) 0.2954 0.1840 0.5117 0.039

C4 4(e) 0.5034 0.064 0.234 -0.057 1.25

Individual isotropic displacement parameters vary considerably because the model is

incompletely refined. The value, which is an order of magnitude larger than all others,

indicates incorrectly positioned atom.

This column lists the distances to the indicated atom in

Chapter

tmaV,O,,

=13.3%

R,,

17.7

10 20 30 40 50 60 70

Bragg angle,

(deg.)

Figure

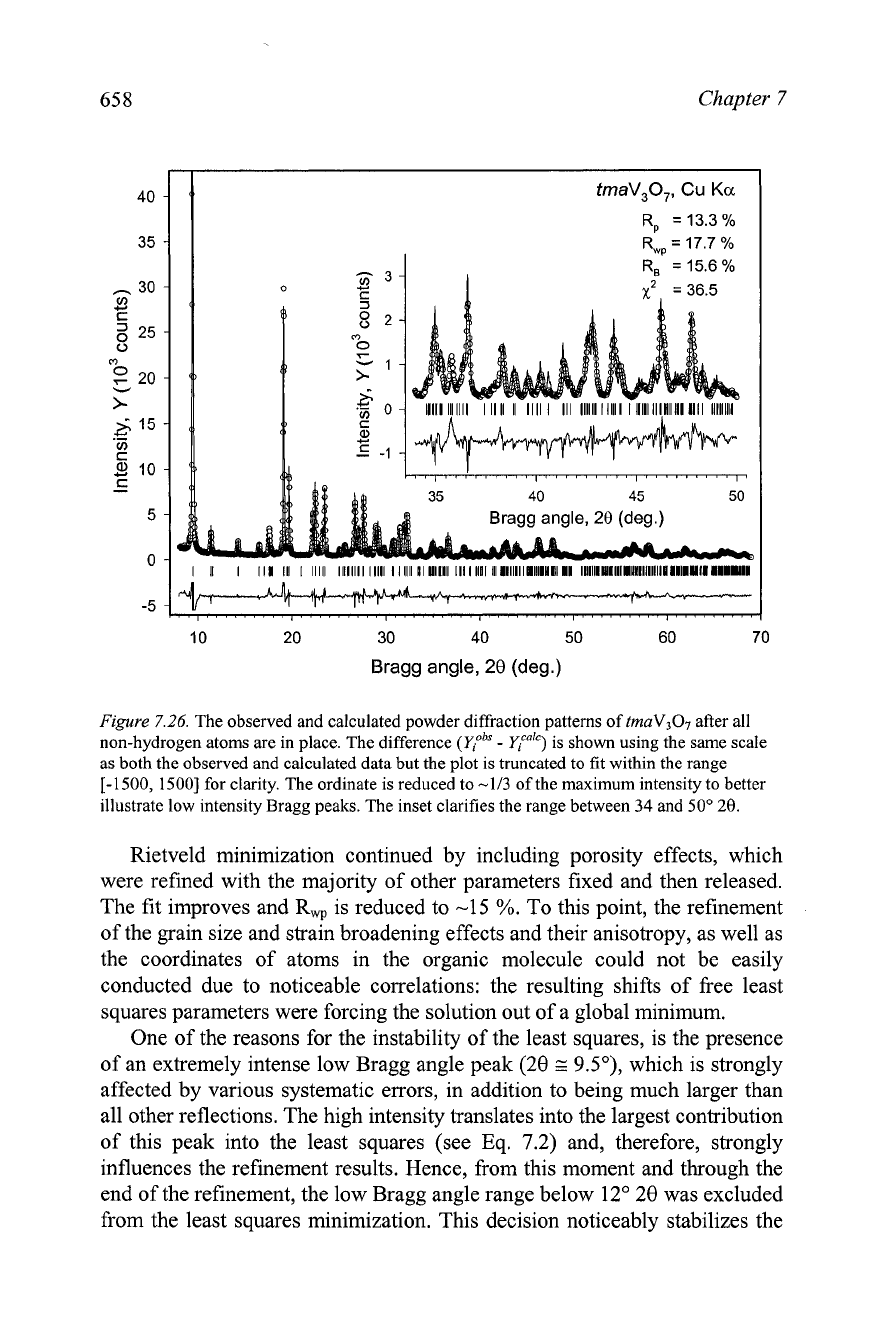
7.26.

The observed and calculated powder diffraction patterns of

tmaV307

after all

non-hydrogen atoms are in place. The difference

(qobs

ylCaIC)

is shown using the same scale

as both the observed and calculated data but the plot is truncated to fit within the range

1-1500,

15001 for clarity. The ordinate is reduced to

-113

of the maximum intensity to better

illustrate low intensity Bragg peaks. The inset clarifies the range between

and

SO0

20.

Rietveld minimization continued by including porosity effects, which

were refined with the majority of other parameters fixed and then released.

The fit improves and R, is reduced to -15

To this point, the refinement

of the grain size and strain broadening effects and their anisotropy, as well as

the coordinates of atoms in the organic molecule could not be easily

conducted due to noticeable correlations: the resulting shifts of free least

squares parameters were forcing the solution out of a global minimum.

One of the reasons for the instability of the least squares, is the presence

of an extremely intense low Bragg angle peak (28

9.5"), which is strongly

affected by various systematic errors, in addition to being much larger than

all other reflections. The high intensity translates into the largest contribution

of this peak into the least squares (see Eq. 7.2) and, therefore, strongly

influences the refinement results. Hence, from this moment and through the

end of the refinement, the low Bragg angle range below 12" 28 was excluded

from the least squares minimization. This decision noticeably stabilizes the

Crystal structure refinement

least squares, although it has little influence on all figures of merit, and the

latter is actually quite unexpected.'

Next, a second preferred orientation axis,

[OlO],

was included into the

minimization, which was based both on the model of the crystal structure

(the orientation of chains in the

vanadate layers) and on the observed shapes

of the crystallites (the plates are elongated in one direction). The refinement

was completed by subsequently releasing the coordinates of atoms forming

an inorganic framework (the

and

atoms) and then organic molecule (the

and

atoms), after which the individual Ui,, for the metal atoms (Vl-V3)

and overall

U,,,

for the oxygen atoms and the organic molecule (two

different overall parameters) together with all profile parameters except for

Wand

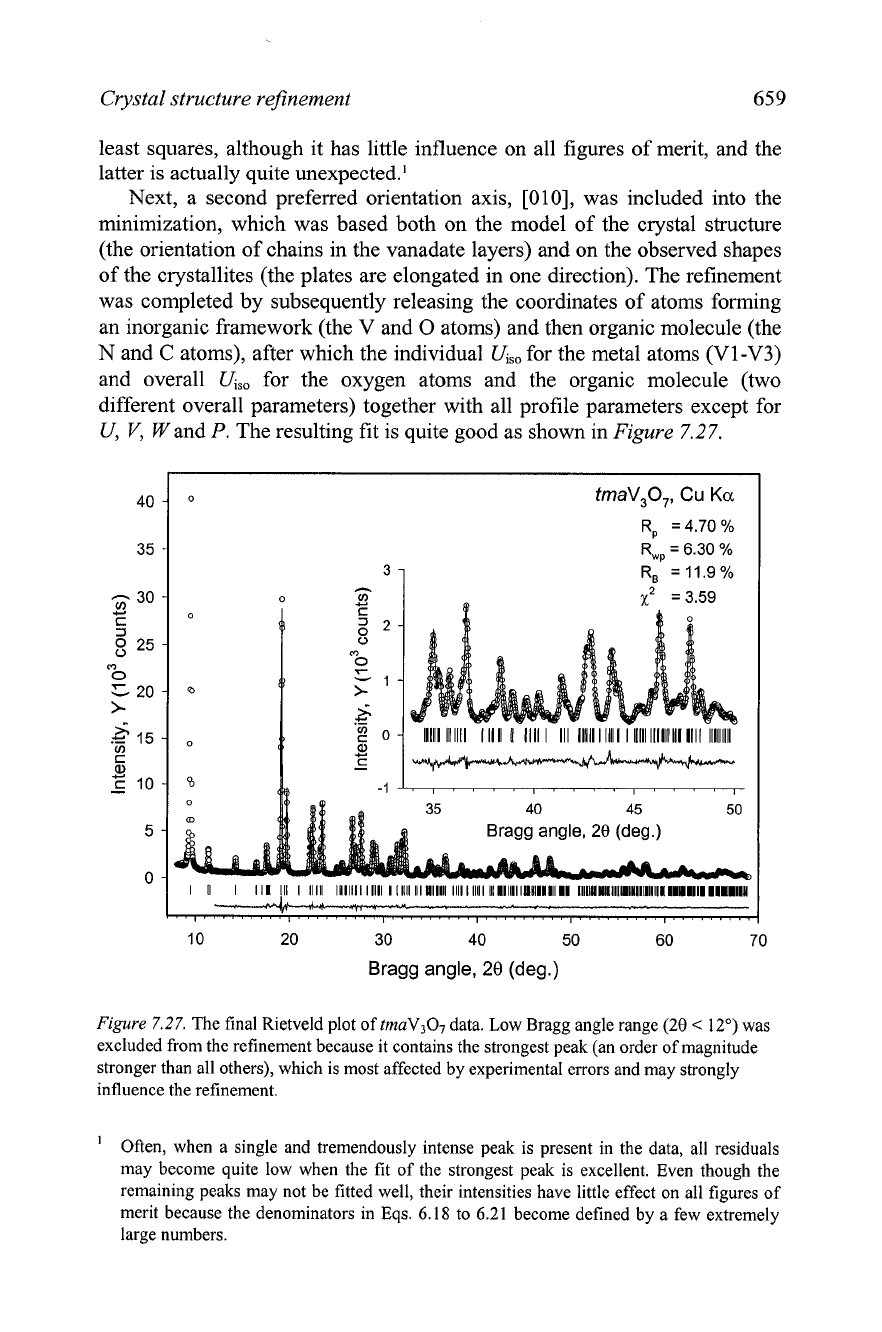
The resulting fit is quite good as shown in

Figure

7.27.

4.70

R,,

6.30

11.9%

Bragg angle,

(deg.)

II I

111

11111

1111111 11111

11111

111

111111 1111 111111 IU IIIIIIIIIIIIIIIII

lllllUlllllUlYllllllllllllNllll

IltlllU

10 20 30 40 50 60 70

Bragg angle,

(deg.)

Figure 7.27.

The final Rietveld plot of tmaV307 data. Low Bragg angle range

(20

12")

was

excluded from the refinement because it contains the strongest peak (an order of magnitude

stronger than all others), which is most affected by experimental errors and may strongly

influence the refinement.

Often, when a single and tremendously intense peak is present in the data, all residuals

may become quite low when the fit of the strongest peak is excellent. Even though the

remaining peaks may not be fitted well, their intensities have little effect on all figures of

merit because the denominators in Eqs.

6.18

6.21

become defined by a few extremely

large numbers.

660

Chapter

Despite the reasonable fit between the observed and calculated

intensities, the analysis of the interatomic distances and bond angles reveals

that the geometry of the tetramethylammonium ion, though acceptable

considering data quality, quantity,' and complexity, is slightly out of the

expected range. The geometry was improved by using the so-called soft

restraint^,^

which are realized in GSAS. The limits on the C-N bond lengths

were set to 1.500

0.015

and the values of C-N-C angles were restrained

to 109.5

1.5". The weights for bonds and angles were manually selected as

and 6, respectively, after testing their influence on the least squares.

Application of soft restraints slightly increases all residuals, as seen from a

comparison of the two last rows in

Table

7.19 but it substantially improves

the geometry of the organic molecule

(Table

7.21). Before the soft-restrained

refinement, the

C-N

bond lengths were in the 1.48 to 1.67

range with the

average bond length 1.56

The C-N-C angles varied from 100 to 127O,

and the spread from the ideal tetrahedral angle was quite large. After the

refinement with the soft restraints, the C-N bond lengths fall within the 1.50

to 1.55

range and bond angles are between 106 and 1 14O, both of which

are acceptable. It is worth noting that instead of soft restraints, a rigid-body

refinement of the

tma

molecule, whose geometry is well known, could also

be employed (the rigid body approach is yet another method of geometrical

restrictions, which is realized in GSAS).

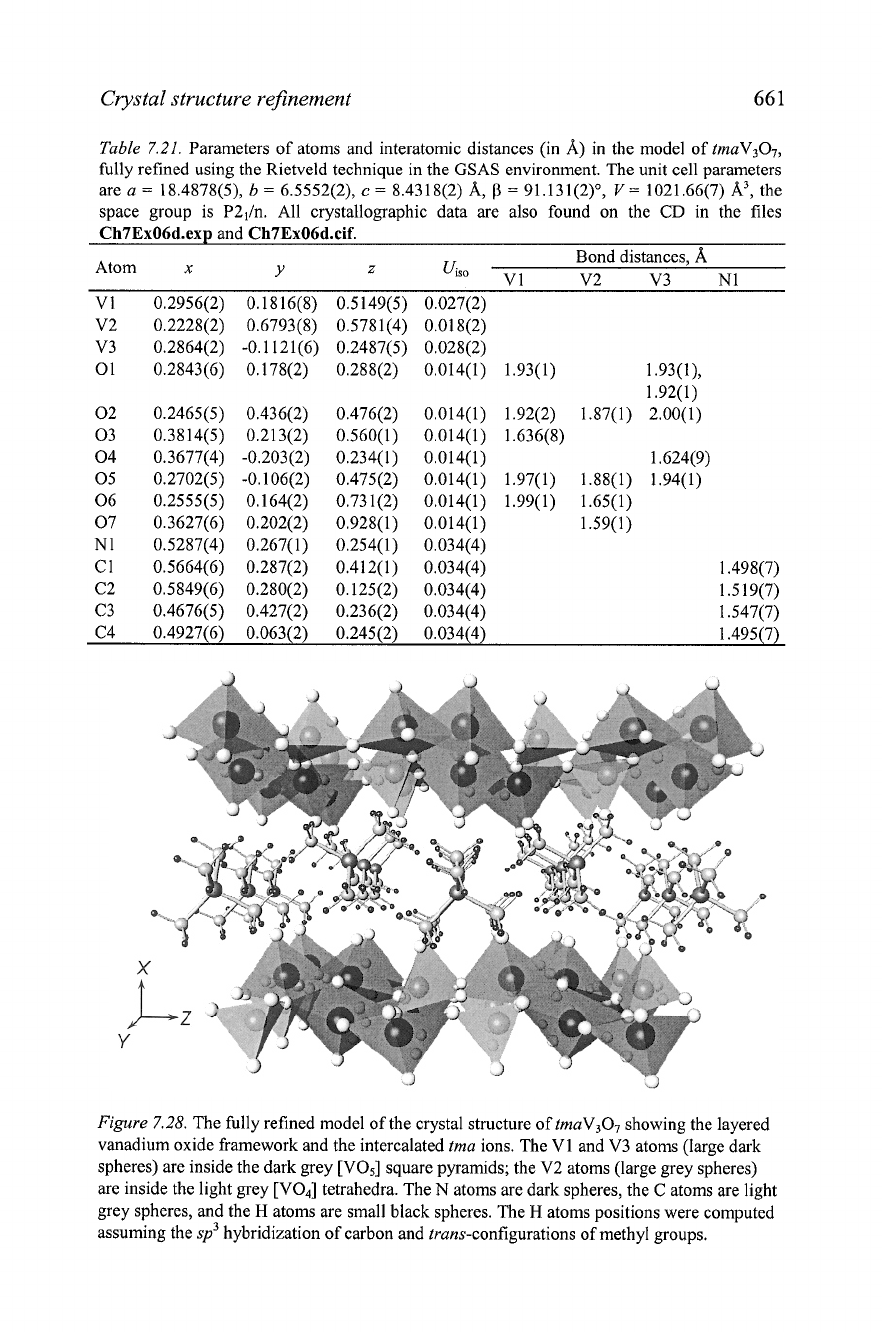
The final Rietveld refinement yields a reasonable structural model

(Figure

7.28), which fits nicely as a new member in the series of V307-based

structures with vanadium oxide layers differing only by the orientations of

the square pyramids and

tet~ahedra.~

specific feature of this refinement is a

strong preferred orientation along two axes, [loo] and [OlO], with

parameters

T~~~

0.76 and

T~~~

1.38 in a

to 1 ratio. The preferred

orientation multipliers range from 0.58 to 2.08, which results in a total

magnitude of about 4, similar to the case described in the previous section.

Due to the presence of the large number of lightly scattering atoms, intensity diffracted by

this powder specimen becomes extremely low above

70'

(sinO/h

0.37

A-').

In a

typical powder diffraction experiment, it is necessary to collect the data to sinO/h

0.5

A-I,

preferably to even higher values.

Soft restraints set limits on the bond distances andlor angles, which are known and are

desired to reach or keep during the least squares minimization. Soft restraints are not exact

(see the footnote on page

654),

and the specified geometry may never be achieved when

distances and angles are set to non-realistic values. The influence of soft restraints, when

compared to a straightforward profile fitting, is regulated by varying the so-called weight,

which can be increased to force the geometry closer to the desired values. The latter

generally considerably worsens the fit if actual bond angles and distances contradict

diffraction data.

P.Y.

Zavalij,

Zhang, and MS. Whittingham, Crystal structure of layered

bis(ethy1enediamine)nickel

hexavanadate as a new representative of the

V6Ol4

series. Acta

Cryst.

B55,953 (1999).

Crystal structure re$nement

Table

7.21.

Parameters of atoms and interatomic distances (in A) in the model of tmaV307,

fully refined using the Rietveld technique in the GSAS environment. The unit cell parameters

are

18.4878(5), b

6.5552(2),

8.4318(2) A,

91.131(2)O,

1021.66(7) A', the

space group is P2,In. All crystallographic data are also found on the

in the files

Ch7Ex06d.e~~

and

Ch7Ex06d.cif.

Atom

Bond distances,

V1 V2 V3 N1

Vl 0.2956(2) 0.1816(8) 0.5149(5) 0.027(2)

Figure

7.28.

The fully refined model of the crystal structure of tmaV307 showing the layered

vanadium oxide framework and the intercalated tma ions. The V1 and V3 atoms (large dark

spheres) are inside the dark grey [V05] square pyramids; the V2 atoms (large grey spheres)

are inside the light grey [VOJ tetrahedra. The

atoms are dark spheres, the C atoms are light

grey spheres, and the

atoms are small black spheres. The

atoms positions were computed

assuming the

sp3

hybridization of carbon and trans-configurations of methyl groups.