Pecharsky V.K., Zavalij P.Y. Fundamentals of Powder Diffraction and Structural Characterization of Materials
Подождите немного. Документ загружается.

Fundamentals of diffraction
20 1
The magnitude of the preferred orientation can be evaluated using the
following function:
which is unity in the case of random orientation, otherwise
J
>
1. When all
grains are perfectly aligned (single crystal) the function 2.84 becomes
infinity.
Only even orders are taken into account in Eqs. 2.83 and 2.84 due to the
presence of the inversion center in the diffraction pattern. The number of
harmonic coefficients
C
and terms
k(h)
varies depending on lattice symmetry
and desired harmonic order
L.
The low symmetry results in multiple terms
(triclinic has 5 terms for
L
=
2) and therefore, low orders 2 or 4 are usually
sufficient. High symmetry requires fewer terms (e.g. cubic has only 1 term
for
L
=
4), so higher orders may be required to adequately describe preferred
orientation. The spherical harmonics approach is realized in
GSAS.'
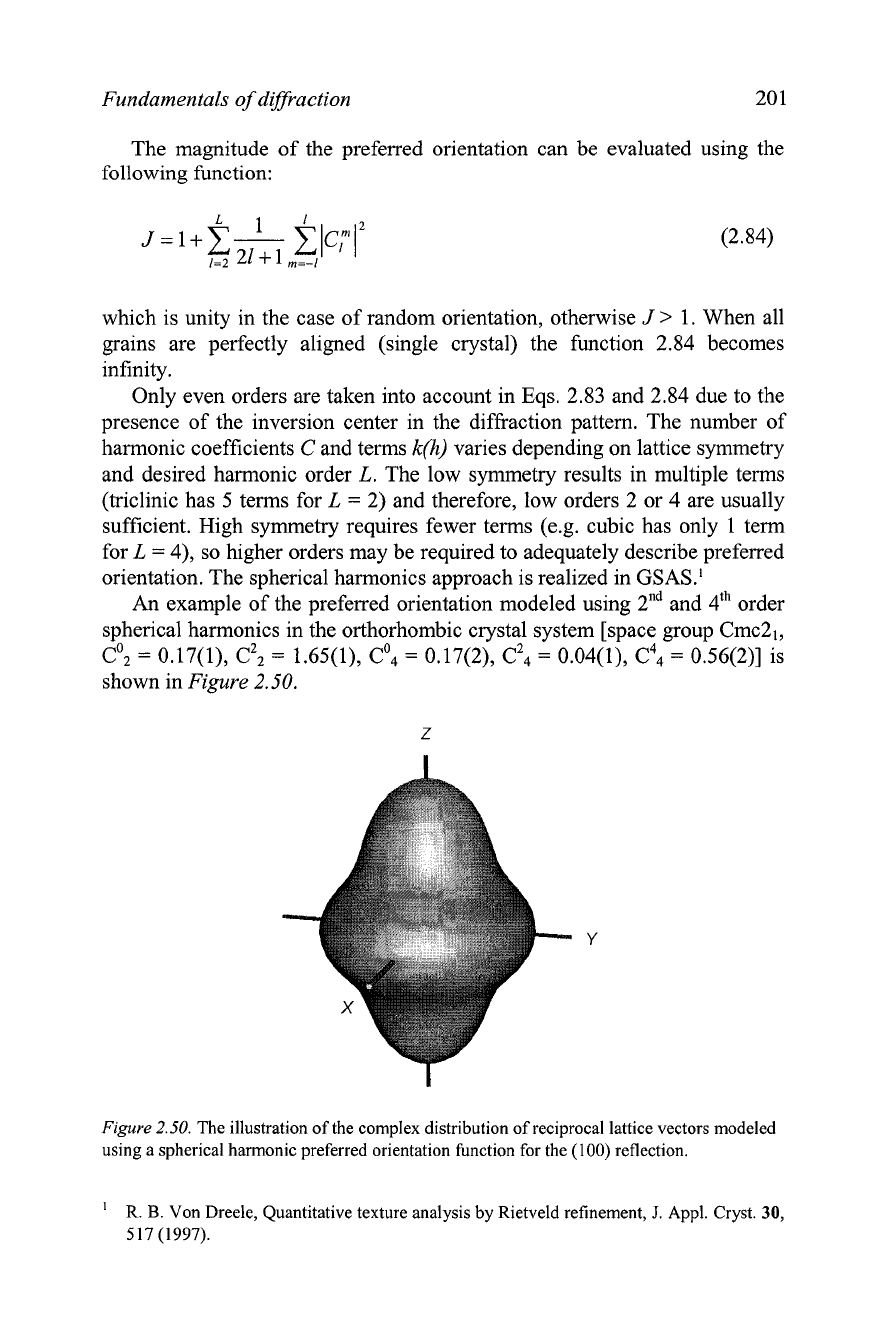
An
example of the preferred orientation modeled using 2nd and 4th order
spherical harmonics in the orthorhombic crystal system [space group Cmc2,,
co2
=
0.17(1),
c~~
=
1.65(1),
co4
=
0.17(2),
c~~
=
0.04(1),
c~~
=
0.56(2)] is
shown in
Figure 2.50.
Figure
2.50.
The illustration of the complex distribution of reciprocal lattice vectors modeled
using a spherical harmonic preferred orientation function for the (100) reflection.
'
R.
B. Von Dreele, Quantitative texture analysis by Rietveld refinement,
J.
Appl. Cryst.
30,
517 (1997).

202
Chapter
2
Here the surface represents the probability to find the reciprocal lattice
point (100) in the diffractometer coordinate system assuming Bragg-
Brentano focusing geometry. The Z-axis is perpendicular to the sample, and
X-
and Y-axes are located in the plane of the sample.
To date, the spherical harmonics approach is the most comprehensive
method developed to account for the preferred orientation effects but in
routine experiments it should be used with great care. The order of
expansion should be increased gradually and only as long as improvements
are obvious and the results make sense.
An
unnecessarily large number of
harmonic coefficients may give excellent agreement between the observed
and calculated diffraction patterns but incorrect structural, especially thermal
displacement parameters may result.
In
its full form, Eq. 2.82 may be used in
complex texture analysis, where powder diffraction data have been collected
not only as a function of Bragg angle 28 but also at different orientations
along
Debye rings and with tilting the sample.
2.10.7
Extinction factor
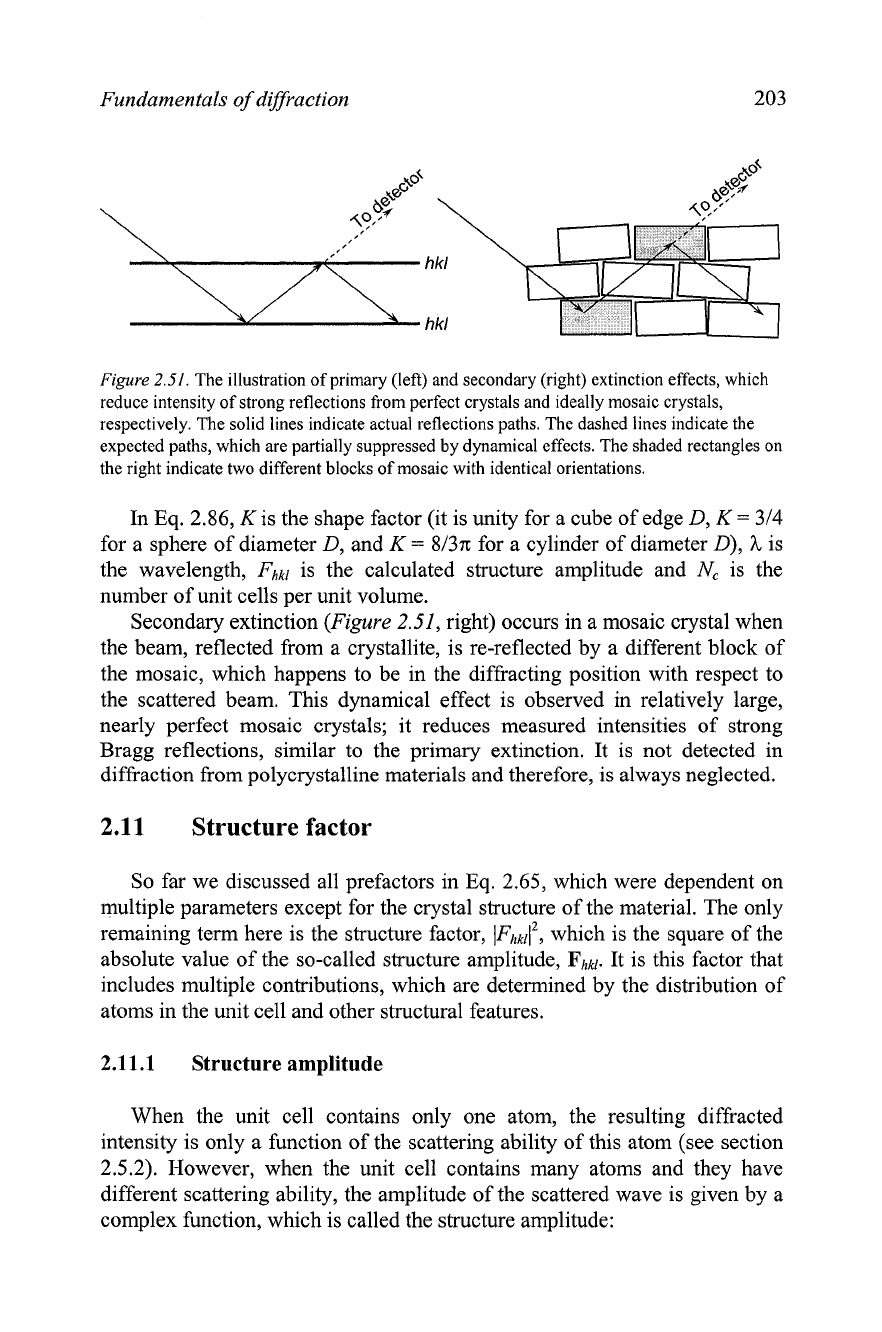
Extinction effects, which are dynamical in nature, may be noticeable in
diffraction from nearly perfect andlor large mosaic crystals. Two types of
extinction are generally recognized: primary, which occurs within the same
crystallite, and secondary, which originates from multiple crystallites.
Primary extinction is caused by back-reflection of the scattered wave into the
crystal and it decreases the measured scattered intensity
(Figure
2.51,
left).
Furthermore, the re-reflected wave is usually out of phase with the incident
wave and thus, the intensity of the latter is lowered due to destructive
interference. Therefore, primary extinction lowers the observed intensity of
very strong reflections from perfect crystals. Especially in powder
diffraction, primary extinction effects are often smaller than experimental
errors; however, when necessary they may be included in Eq. 2.65 as:'
E,,
=
E,
sin2
8
+
EL
cos2
8
where
EB
and
EL
are Bragg (28
=
7c)
and Laue
(28
=
0) components, both
defined as various functions of the extinction parameter,
x,
which is
normally
a
refined variable:
T.M.
Sabine,
R.B.
Von Dreele, and J.E. Jorgensen, Extinction in time-of-flight neutron
powder diffractometry, Acta Cryst.
A44,
374
(1988);
T.M.
Sabine, A reconciliation of
extinction theories, Acta Cryst.
A44,368 (1988).
Fundamentals of diffraction 203
Figure
2.51.
The illustration of primary (left) and secondary (right) extinction effects, which
reduce intensity of strong reflections from perfect crystals and ideally mosaic crystals,
respectively. The solid lines indicate actual reflections paths. The dashed lines indicate the
expected paths, which are partially suppressed by dynamical effects. The shaded rectangles on
the right indicate two different blocks of mosaic with identical orientations.
In
Eq. 2.86,
K
is the shape factor (it is unity for a cube of edge
D,
K
=
314
for a sphere of diameter
D,
and
K
=
813n for a cylinder of diameter
D),
h
is
the wavelength,
Fhkl
is the calculated structure amplitude and
N,
is the
number of unit cells per unit volume.
Secondary extinction (Figure
2.51,
right) occurs in a mosaic crystal when
the beam, reflected from a crystallite, is re-reflected by a different block of
the mosaic, which happens to be in the diffracting position with respect to
the scattered beam. This dynamical effect is observed in relatively large,
nearly perfect mosaic crystals; it reduces measured intensities of strong
Bragg reflections, similar to the primary extinction. It is not detected in
diffkaction from polycrystalline materials and therefore, is always neglected.
2.11
Structure factor
So far we discussed all prefactors in Eq. 2.65, which were dependent on
multiple parameters except for the crystal structure of the material. The only
remaining term here is the structure factor,
IF^^^^^,
which is the square of the
absolute value of the so-called structure amplitude, Fhkl. It is this factor that
includes multiple contributions, which are determined by the distribution of
atoms in the unit cell and other structural features.
2.1
1.1
Structure amplitude
When the unit cell contains only one atom, the resulting diffracted
intensity is only a function of the scattering ability of this atom (see section
2.5.2). However, when the unit cell contains many atoms and they have
different scattering ability, the amplitude of the scattered wave is given by a
complex function, which is called the structure amplitude:

204 Chapter
2
n
F(h)
=
gJtJ
(s)
fJ
(s) exp(27cih
.
xJ
)
j=1
where:
-
P(h)
is the structure amplitude of a Bragg reflection with indices hkl,
which are represented as vector
h
in three dimensions. The structure
amplitude itself is often shown in a vector form since it is a complex
number;
-
n
is the total number of atoms in the unit cell and
n
includes all
symmetrically equivalent atoms;
-
S
is sineh&;
-
2
is the population (or occupation) factor of the
jth
atom
(g'
=
1 for a fully
occupied site);
-
P
is the temperature factor, which describes thermal motions of the
jth
atom;
-
fJ(.s) is the atomic scattering factor describing interaction of the incident
wave with a specific type of an atom as a function of sinelh for x-rays or
electrons, and it is simply
fJ
(i.e. is independent of sinelh) for neutrons;
-
i=fi;
-
h-x'
is a scalar product of two vectors:
h
=
(h, k,
I)
and vector xj
=
(2,
y',
2).
The latter represents fractional coordinates of the
jt"
atom in the unit
cell:
Taking into account Eq. 2.88, the previous Eq. 2.87 can be written in the
expanded form as:
n
Fhkl
=
g't'
(s)
f
'
(s)
exp[2ni(hn'
+
ky
'
+
lz'
)I
(2.89)
j=1
2.1 1.2
Population factor
Considering a randomly chosen unit cell, each available position with a
specific coordinate triplet (2,
y',
d)
may be only occupied by one atom
(fractional population parameter
g'
=
1) or it may be left unoccupied
(g'
=
0).
On the other hand, even very small crystals contain a nearly infinite number
of unit cells (e.g. a crystal in the form of a cube with 1 pm side will contain

Fundamentals of diffraction 205
lo9 cubic unit cells with a
=
10
A)
and diffraction is observed fkom all of the
unit cells simultaneously. Hence, the resulting structure amplitude is
normalized to a certain mean unit cell, which represents the distribution of
atoms averaged over the entire volume of the studied sample.
In
the majority
of compounds, each crystallite is fully ordered and the content of every unit
cell may be assumed identical throughout the whole crystal, so the
population factor remains unity for every atom. Occasionally, population
factor(s) may be lower than one but greater than zero, and some of the
common reasons of why this occurs are briefly discussed below.
It is possible that in some of the unit cells atoms are missing. Thus,
instead of a complete occupancy, the corresponding site in an average unit
cell will contain only a fraction
(0
<
g'
<
1) of the
jth
atom.
In
cases like that,
it is said that the lattice has defects and
g'
smaller than 1 reflects a fraction of
the unit cells where a specific position is occupied. Obviously, a fraction of
the unit cells where the same position is empty complements
g'
to unity and
it is equal to 1
-
g'.
A
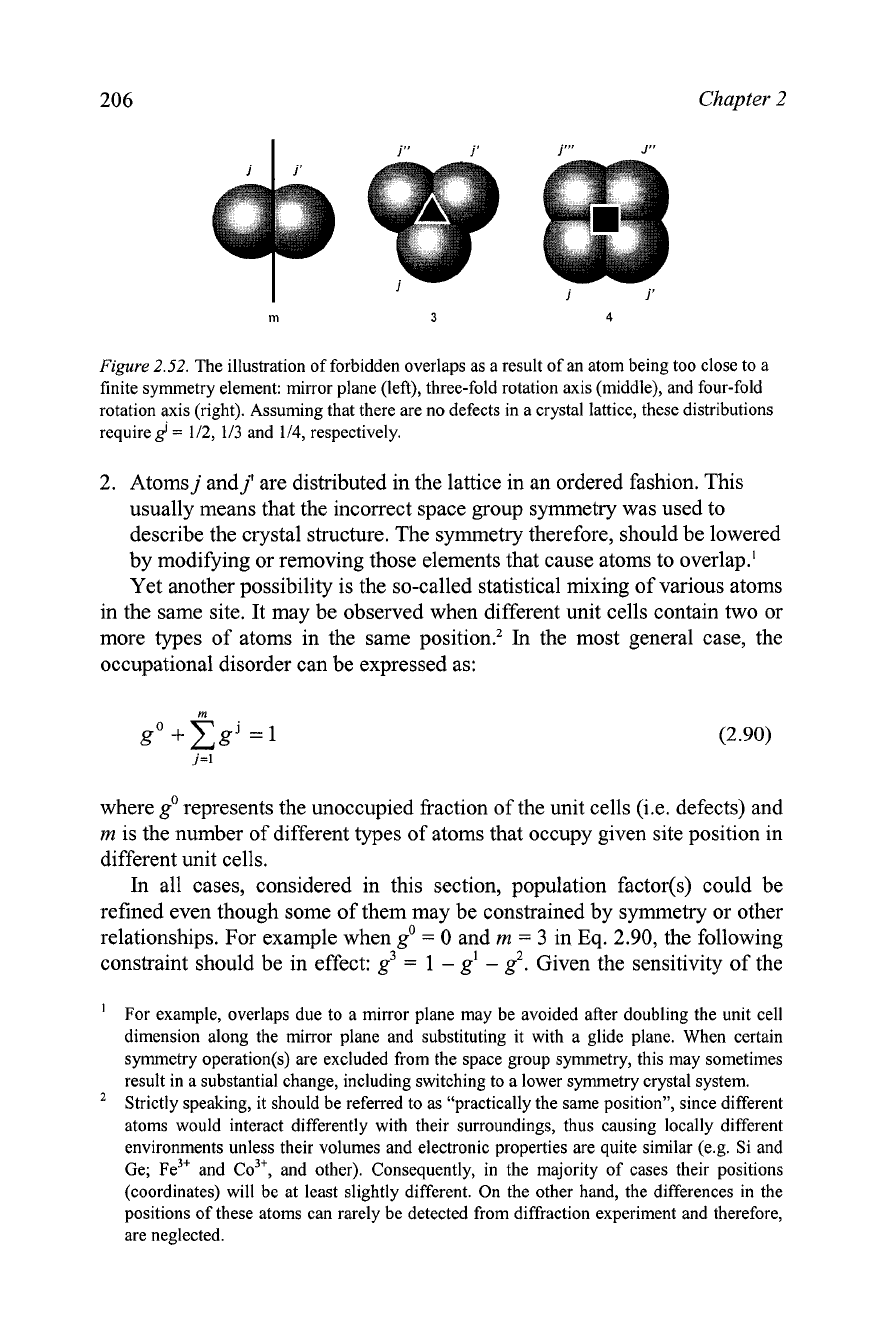
different situation arises when in an average unit cell atom
j
is located
close to a finite symmetry element, e.g. mirror plane.
A
mirror plane
produces atom j', symmetrically equivalent to j, so that the distance between
j'
and
j
becomes unreasonably small and core electrons of the two atoms
overlap but in reality they cannot be located at such close distance (Figure
2.52, left). This usually means that in some of the unit cells atom
j
is located
on one side of the mirror plane, while in the others it is positioned on the
opposite side of the same plane. If this is the case, then in the absence of
"conventional" defects, the population factors
g'
and
g"
are related as
g'
=
1
-
21, and
g'
=
g"
because of mirror symmetry.
In
general,
g'
=lln, where n is the multiplicity of the symmetry element
which causes the overlap of the corresponding atoms. When the culprits are
a mirror plane, a two fold rotation axis or a center of inversion,
n
=
2
and
g'
=
0.5. For a three fold rotation axis n
=
3
and
g'
=
113, and so on (Figure
2.52, middle and right). When defects are present in addition to the overlap,
g'
is no longer equal to
g"
and two or more population factors may become
independent.
The condition illustrated in Figure 2.52 usually happens when the real
symmetry of the unit cell (and the lattice) is lower than the symmetry of the
average unit cell as detected from
diffraction data. Hence, the following
should be taken into the consideration:
1. Distribution of atoms
j
and j' in the lattice is random, so the crystal is
partially disordered.
In
this case, the average cell may still be used to
describe the average crystal structure even when it has a higher symmetry
than the real unit cell.
Chapter
2
Figure
2.52.
The illustration of forbidden overlaps as a result of an atom being too close to a
finite symmetry element: mirror plane (left), three-fold rotation axis (middle), and four-fold
rotation axis (right). Assuming that there are no defects in a crystal lattice, these distributions
require
2
=
112,
113
and
114,
respectively.
2.
Atoms
j
and
j'
are distributed in the lattice in an ordered fashion. This
usually means that the incorrect space group symmetry was used to
describe the crystal structure. The symmetry therefore, should be lowered
by modifying or removing those elements that cause atoms to overlap.'
Yet another possibility is the so-called statistical mixing of various atoms
in the same site. It may be observed when different unit cells contain two or
more types of atoms in the same position.'
In
the most general case, the
occupational disorder can be expressed as:
where
go
represents the unoccupied fraction of the unit cells (i.e. defects) and
m
is the number of different types of atoms that occupy given site position in
different unit cells.
In
all cases, considered in this section, population factor(s) could be
refined even though some of them may be constrained by symmetry or other
relationships. For example when
go
=
0 and
m
=
3
in
Eq.
2.90, the following
constraint should be in effect:
g3
=
1
-
g1
-
g2.
Given the sensitivity of the
For example, overlaps due to a mirror plane may be avoided after doubling the unit cell
dimension along the mirror plane and substituting it with a glide plane. When certain
symmetry
operation(s) are excluded from the space group symmetry, this may sometimes
result in a substantial change, including switching to a lower symmetry crystal system.
Strictly speaking, it should be referred to as "practically the same position", since different
atoms would interact differently with their surroundings, thus causing locally different
environments unless their volumes and electronic properties are quite similar
(e.g. Si and
Ge; Fe3+ and co3+, and other). Consequently, in the majority of cases their positions
(coordinates) will be at least slightly different. On the other hand, the differences in the
positions of these atoms can rarely be detected from diffraction experiment and therefore,
are neglected.
Fundamentals
of
diffraction
207
population parameters to the potentially overestimated symmetry, it is
important to analyze their values, especially if they converge into simple
factions, e.g. 112, 113, and so on (see
Figure
2.52).
2.11.3
Temperature factor
At any temperature higher than absolute zero, certain frequencies in a
phonon spectrum of the crystal lattice are excited and as a result, atoms are
in a continuous oscillating motion about their equilibrium positions, which
are determined by coordinate triplets (x, y,
z).
To account for these
vibrations, the so-called temperature factor is introduced into the general
equation (Eq. 2.87) of the structure amplitude.
It is worth noting that according to a recommendation issued by the
International Union of Crystallography
(IUCr), the corresponding
parameters representing the temperature factor should be referred as "atomic
displacement parameters" instead of the commonly used "temperature
parameters" or "thermal parameters". This suggestion is based on the fact
that these parameters, when determined from x-ray diffraction experiment,
represent the combined total of several effects in addition to displacements
caused by thermal motion. They include deformation of the electron density
around the atom due to chemical bonding, improperly or not accounted
absorption, preferred orientation, porosity, and so on, even if they influence
the structure factor to a much lesser degree than thermal motion.
Oscillatory motions of atoms may be quite complex and as a result,
several different levels of approximations in the expression of the
temperature factor can be used.
In
the simplest form, the temperature factor
of the
jth
atom is represented as:
where
~j
is the displacement parameter of the
jt"
atom,
8
is the Bragg angle
at which a specific reflection
hkl
is observed and
h
is the wavelength. This is
the so-called isotropic approximation, which assumes equal probability of an
atom to deviate in any direction regardless of its environment,
i.e. atoms are
considered as diffuse spheres.
In
Eq. 2.91
where (U2)i is the root mean square deviation of the
jth
atom from its
equilibrium position (x, y,
z)
in A2.

208
Chapter
2
Considering Eq. 2.92, the isotropic displacement parameters are only
physical when they are positive (also see section
2.1
1.4).
Depending on the
nature of the material, they usually vary within relatively narrow ranges at
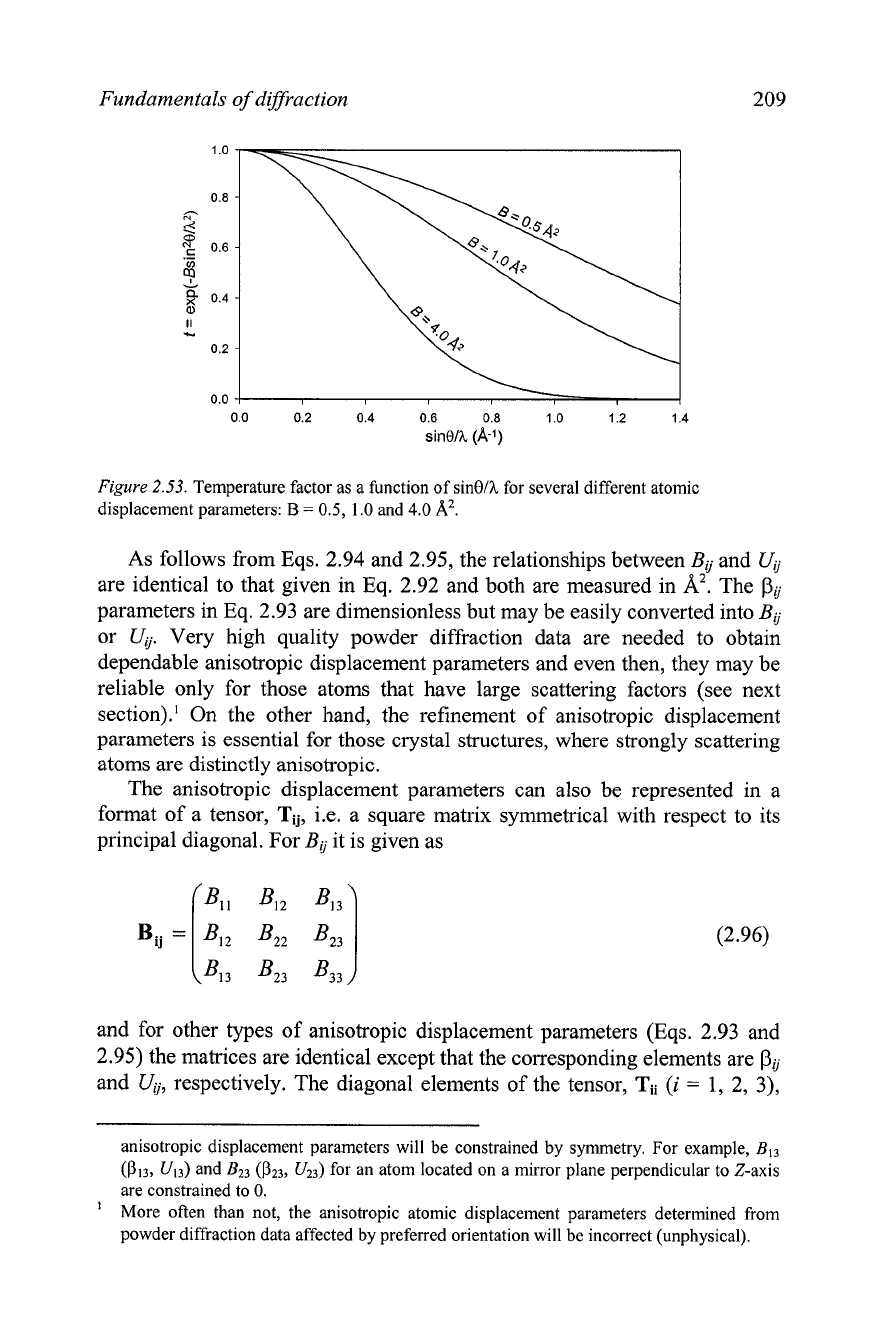
room temperature. For inorganic ionic crystals and intermetallic compounds
the typical range of
B's is -0.5 to -1 A2; for other inorganic and many
coordination compounds B varies from -1 to -3 A2, while for organic and
organometallic compounds and for solvent or other intercalated non-bonded
molecules or atoms this range extends from -3 to -10
A2
or higher. As can
be seen in
Figure
2.53, the high value of the atomic displacement parameter
results in a rapid decrease of the structure amplitude when the Bragg angle
increases (also see Eq. 2.87).
As mentioned at the beginning of this section, the temperature factor
absorbs other unaccounted or incorrectly accounted effects. The most critical
are absorption, porosity and other instrumental or sample effects (see
Chapter 3), which in a systematic way modify the diffracted intensity as a
function of the Bragg angle. As a result, the
B
parameters of all atoms may
become negative. If this is the case, then the absorption correction should be
re-evaluated and re-refined or the experiment should be repeated to
minimize the deleterious instrumental influence on the distribution of
intensities of Bragg peaks.
The next level of approximation accounts for the anisotropy of thermal
motions in a harmonic approximation and describes atoms as ellipsoids in
one of the three following forms, which are, in fact, equivalent to one
another:
where
pi,
,..pi,
,
B,',
.
.
.B;~
and
u/,
. .
.u:,
are the anisotropic atomic
displacement parameters (six parameters' per atom).
'
This is true for atoms located in the general site position (point symmetry
I),
where all six
parameters are independent from one another. In special positions, some or all of the
Fundamentals of diffraction 209
Figure
2.53.
Temperature factor as a function of sinelk for several different atomic
displacement parameters:
B
=
0.5,
1.0
and
4.0
A'.
As follows from Eqs.
2.94
and 2.95, the relationships between
BU
and
UU
are identical to that given in Eq. 2.92 and both are measured in
A2.
The
PU
parameters in Eq. 2.93 are dimensionless but may be easily converted into
Bij
or
uy.
Very high quality powder diffraction data are needed to obtain
dependable anisotropic displacement parameters and even then, they may be
reliable only for those atoms that have large scattering factors (see next
section).' On the other hand, the refinement of anisotropic displacement
parameters is essential for those crystal structures, where strongly scattering
atoms are distinctly anisotropic.
The anisotropic displacement parameters can also be represented in a
format of a tensor,
Tij, i.e. a square matrix symmetrical with respect to its
principal diagonal. For
By
it is given as
and for other types of anisotropic displacement parameters (Eqs. 2.93 and
2.95) the matrices are identical except that the corresponding elements are
PU
and
Ui,.,
respectively. The diagonal elements of the tensor, Tii (i
=
1,
2, 3),
anisotropic displacement parameters will be constrained by symmetry. For example, BI3
(PI3, UI3) and
BZ3
(P23, U23) for an atom located on a mirror plane perpendicular to Z-axis
are constrained to
0.
'
More often than not, the anisotropic atomic displacement parameters determined from
powder diffraction data affected by preferred orientation will be incorrect (unphysical).
210
Chapter
2
describe atomic displacements along three mutually perpendicular axes of
the ellipsoid. Thus, similar to the isotropic displacement parameter, they may
not be negative and should have reasonable values at room temperature as
established by the nature of the material. Generally, the ratios between
w.i,
should not exceed
3
to
5,
unless the large anisotropy can be explained.
All nine elements of the tensor
Tij
establish the orientation of the
ellipsoid in the coordinate basis of the crystal lattice. Hence, any or all non-
diagonal elements may be positive or negative but certain relationships
between them and the diagonal parameters should be observed as shown in
Eq.
2.97
for
Bij.
If any of these three relationships between the anisotropic
displacement parameters is violated, then the set of parameters has no
physical meaning.
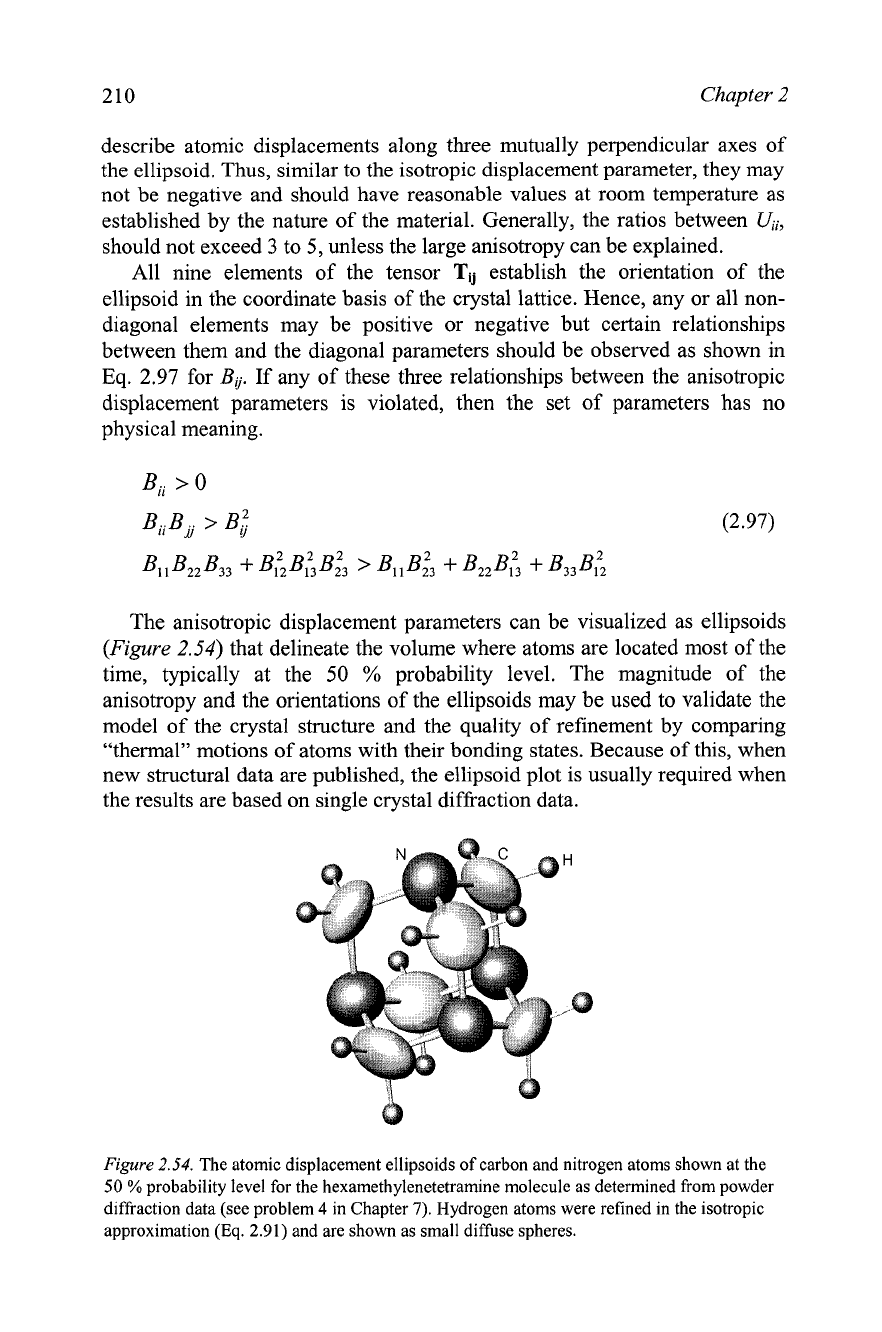
The anisotropic displacement parameters can be visualized as ellipsoids
(Figure
2.54)
that delineate the volume where atoms are located most of the
time, typically at the 50
%
probability level. The magnitude of the
anisotropy and the orientations of the ellipsoids may be used to validate the
model of the crystal structure and the quality of refinement by comparing
"thermal" motions of atoms with their bonding states. Because of this, when
new structural data are published, the ellipsoid plot is usually required when
the results are based on single crystal
diffkaction data.
Figure
2.54.
The atomic displacement ellipsoids of carbon and nitrogen atoms shown at the
50
%
probability level for the hexamethylenetetramine molecule as determined from powder
diffraction data (see problem
4
in Chapter
7).
Hydrogen atoms were refined in the isotropic
approximation
(Eq.
2.91)
and are shown as small diffuse spheres.
