Pecharsky V.K., Zavalij P.Y. Fundamentals of Powder Diffraction and Structural Characterization of Materials
Подождите немного. Документ загружается.


80
Chapter
I
First, we select a new basis in which the rotation axis intersects with the
origin of coordinates. This is equivalent to changing the coordinates of the
original vector from
X
to
x,
where
According to Eq. 1.39, the symmetry transformation in the new basis
results in vector
x',
where
After switching back to the original basis by applying negative
translation
-At
to the right hand part of Eq. 1.42 we obtain the coordinates of
the symmetrically equivalent vector
X'
in the original basis as
where
E
is unity matrix. Noting that (R
-
E)At
+
t
=
T,
i.e. it is the
translational part that reflects the shift of the rotation axis from the origin of
coordinates in addition to the conventional translational part, t, Eq. 1.43
becomes identical to Eq. 1.39. Thus, equations 1.38 and 1.39 are valid for
any crystallographic transformation in three dimensions.
It may be useful to briefly summarize the main properties of rotation
transformation matrices,
R,
some of which were already mentioned above:
-
In
conventional crystallography matrix elements may accept only the
following values:
-
R
is unimodular:
-
The inverse rotation matrix is the same as the direct matrix, i.e.
R-'
=
R
when the rotation order is
1
or 2, otherwise
R-'
represents rotation in the
opposite direction.
-
An
order of an axis represented by the operation R can be determined in
two steps:
1.
In
the case of an inversion axis, when det(R1)
=
-1,
the matrix should
be converted to a simple rotation by multiplying its elements by -1
:
Fundamentals
of
crystalline state
8
I
2.
The order of the axis
(N)
is determined fkom the number of
multiplications of the matrix
R
by itself that are required to obtain the
unity matrix,
E:
1.20.5
Augmented matrices
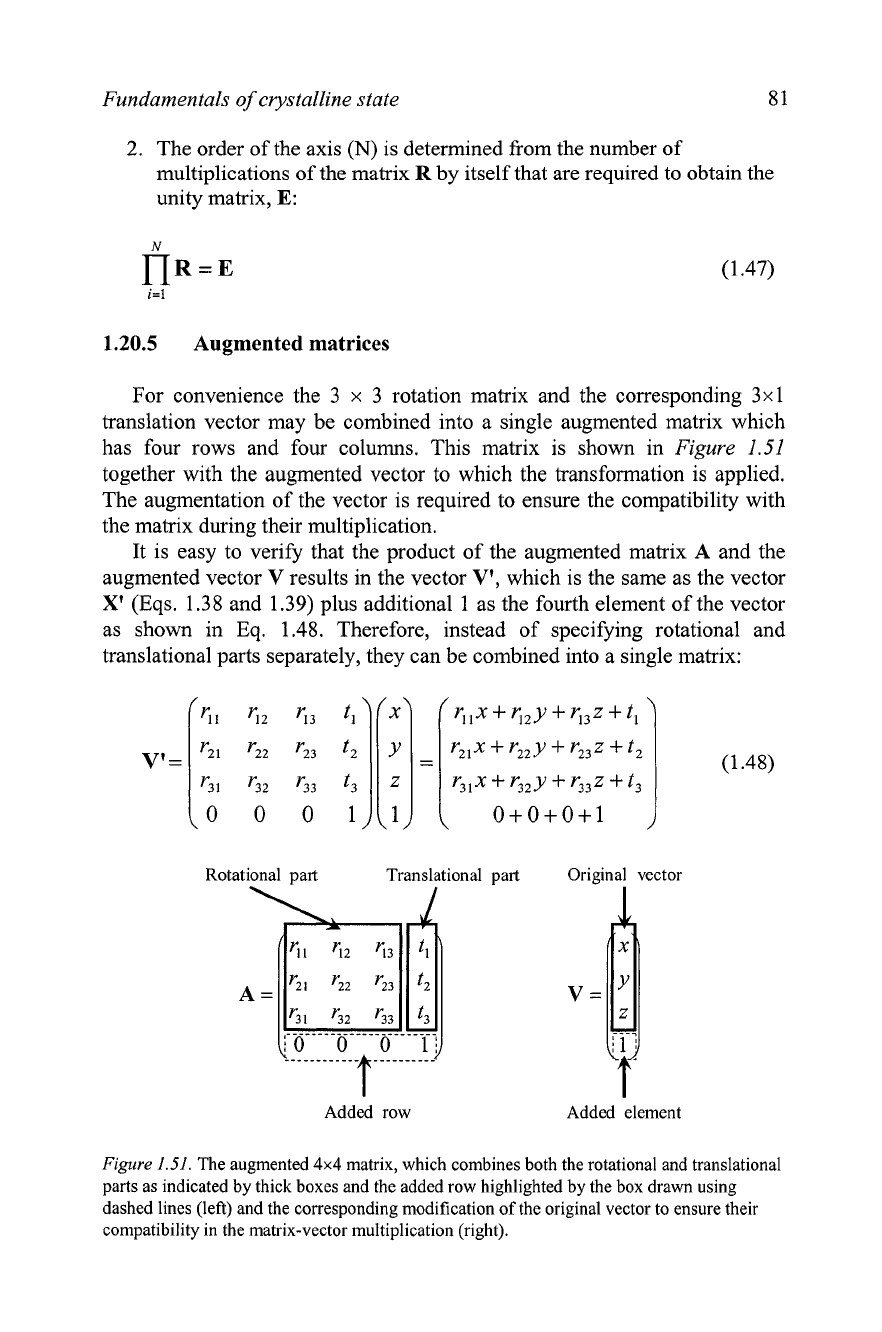
For convenience the
3
x
3 rotation matrix and the corresponding 3x1
translation vector may be combined into a single augmented matrix which
has four rows and four columns. This matrix is shown in
Figure
1.51
together with the augmented vector to which the transformation is applied.
The augmentation of the vector is required to ensure the compatibility with
the matrix during their multiplication.
It is easy to verify that the product of the augmented matrix
A
and the
augmented vector V results in the vector V1, which is the same as the vector
X1 (Eqs.
1.38
and 1.39) plus additional 1 as the fourth element of the vector
as shown in Eq.
1.48.
Therefore,
instead of specifying rotational and
translational parts separately, they can be combined into a single matrix:
Rotational
art
Translational uart
Original vector
Added row Added element
Figure
1.51.
The augmented
4x4
matrix, which combines both the rotational and translational
parts as indicated by thick boxes and the added row highlighted by the box drawn using
dashed lines (left) and the corresponding modification of the original vector to ensure their
compatibility in the matrix-vector multiplication (right).
82
Chapter
1
1.20.6
Algebraic representation of crystallographic symmetry
Considering conventional crystallographic symmetry, the elements
representing rotations, i.e.
rij,
are restricted to
1,
0,
or -1, and the elements
representing translations, i.e.
ti
in
Eq.
1.48, are restricted to
f
112,
f
113, +1/4,
f
116,
f
118 including their integer multiples, and
0.
In this way, all possible
transformations of atoms by symmetry operations are represented by the
multiplication of matrices and vectors.
Therefore, symmetrical transformations in the crystal are formalized as
algebraic (matrix-vector) operations
-
an extremely important feature used in
all crystallographic calculations in computer software. The partial list of
symmetry elements along with the corresponding augmented matrices that
are used to represent symmetry operations included in each symmetry
element is provided in
Table
1.19
and
Table
1.20.
For a complete list,
consult the International Tables for Crystallography, vol.
A.
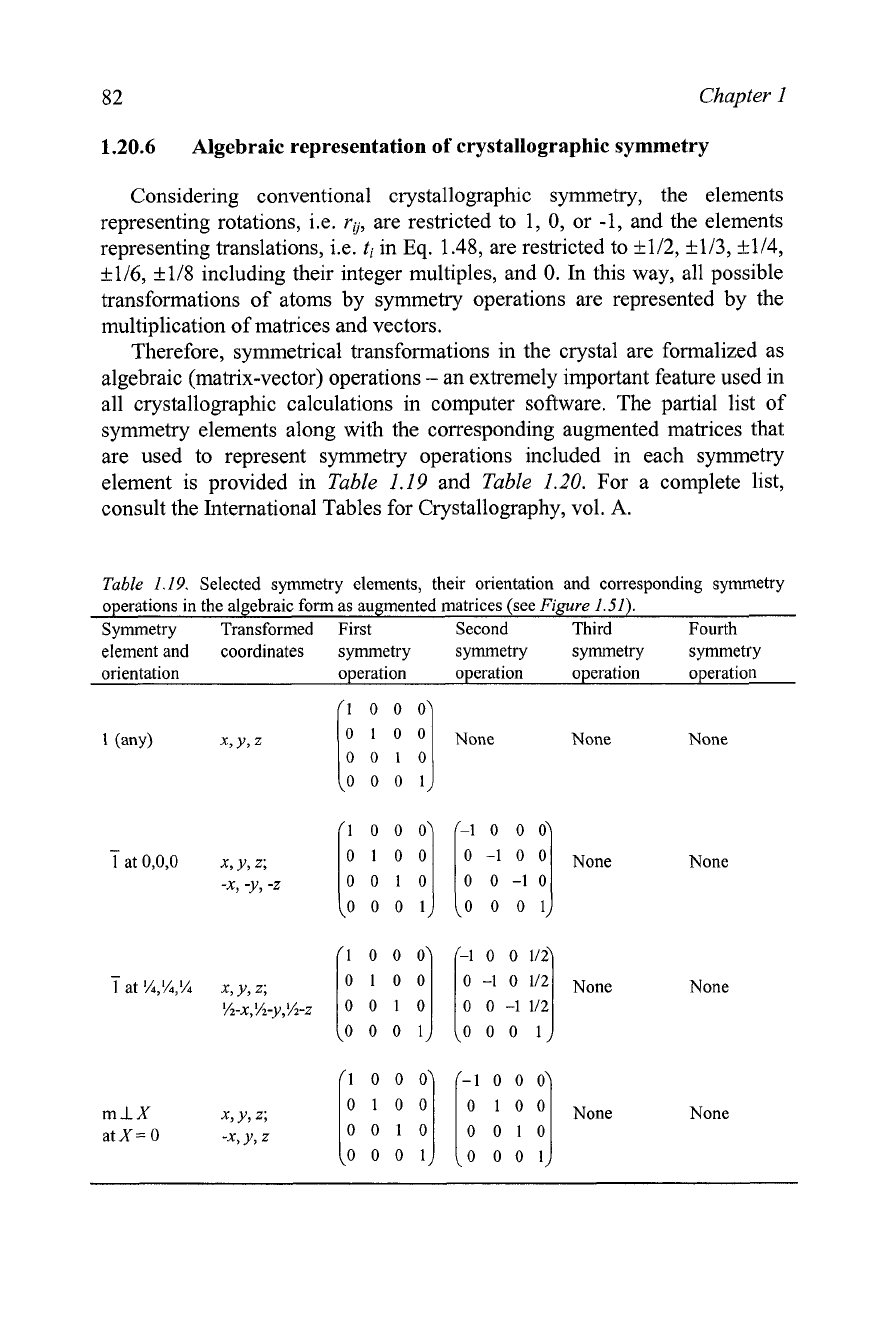
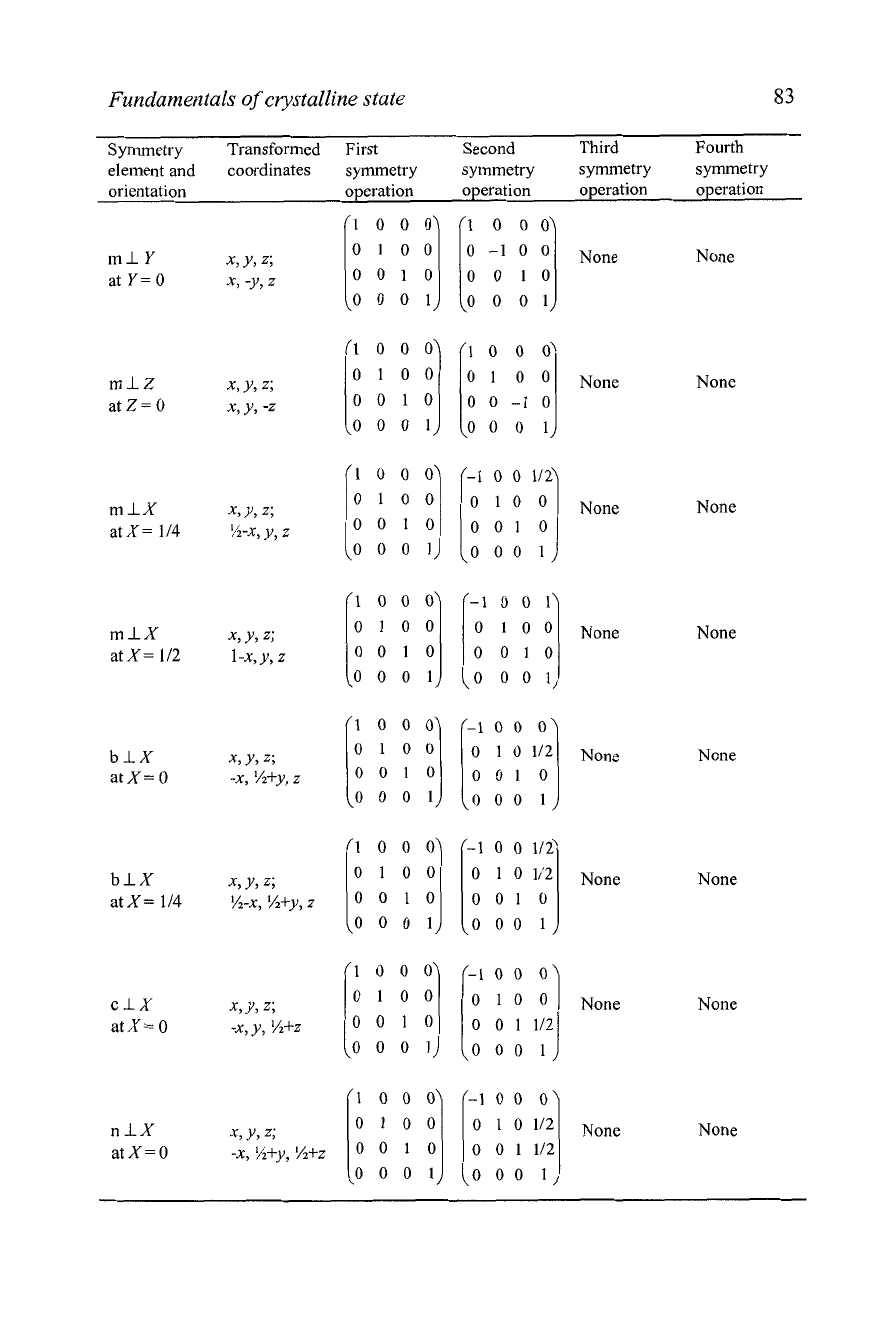
Table
1.19.
Selected symmetry elements, their orientation and corresponding symmetry
operations in the algebraic form as augmented matrices (see
Figure
1.51).
Symmetry Transformed First Second Third Fourth
element and coordinates symmetry symmetry symmetry symmetry
orientation operation operation operation operation
None None
-
1
at
0,0,0
x,
y,
z;
None
-X,
-y,
-z
1000 -100112
-
1
at
%,%,%
x,
y, z;
1:
:
:
1:
.
1
None
None
1/2-x,1/2-Y,l/z-z
0 0
1
0 0 0
-1
112
None
Fundamentals
of
crystalline state
8
3
Symmetry Transformed First Second Third Fourth
elementand coordinates symmetry symmetry symmetry symmetry
orientation operation operation operation operation
None
None
None
None
None
None
None
None
84
Chapter
I
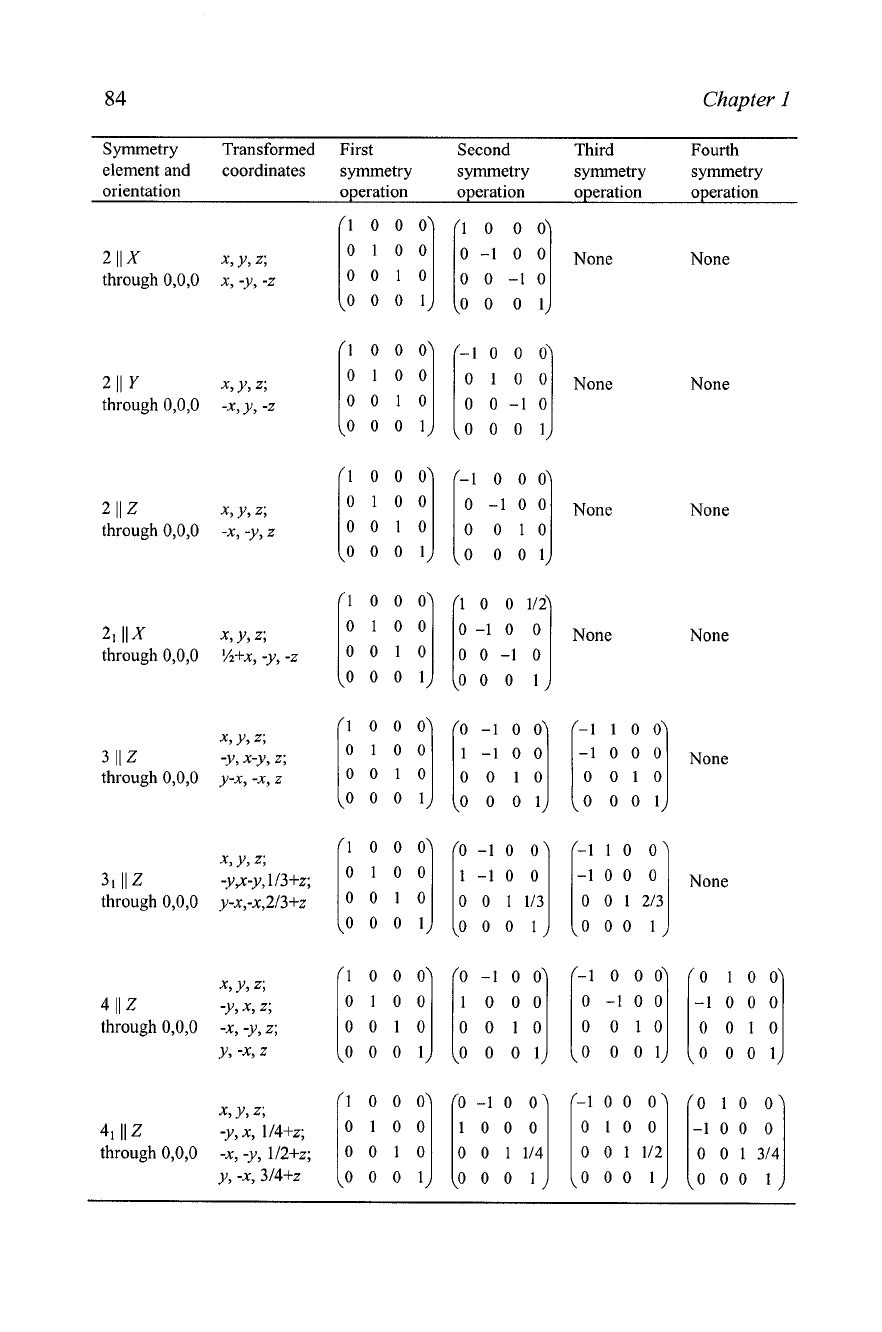
Symmetry Transformed First Second Third Fourth
elementand coordinates symmetry symmetry symmetry symmetry
orientation operation operation operation operation
2
llX
x, Y, z;
through
0,0,0
x, -y, -z
2
ll
y
x, Y, z;
through
0,0,0
-x, y, -z
2
ll
z
x, Y, z;
through
0,0,0
-x, -y, z
21
llX
x, Y, z;
through
0,0,0
%+x, -y,
-Z
x, Y, z;
3
llZ
-Y,
x-Y, z;
through
0,0,0
y-x, -x,
z
x, Y, z;
31
11
z
-y,x-y,
1
/3+z;
through
0,0,0
y-x,-x,2/3+z
x, Y, z;
4
ll
z
-Y, x, z;
through
0,0,0
-x, -y,
Z;
Y,
-4
z
x, Y, z;
41
ll
z
-y, x, 1/4+z;
through
0,0,0
-x, -y, 1/2+z;
y, -x, 314+z
None
Fundamentals
of
crystalline state
85
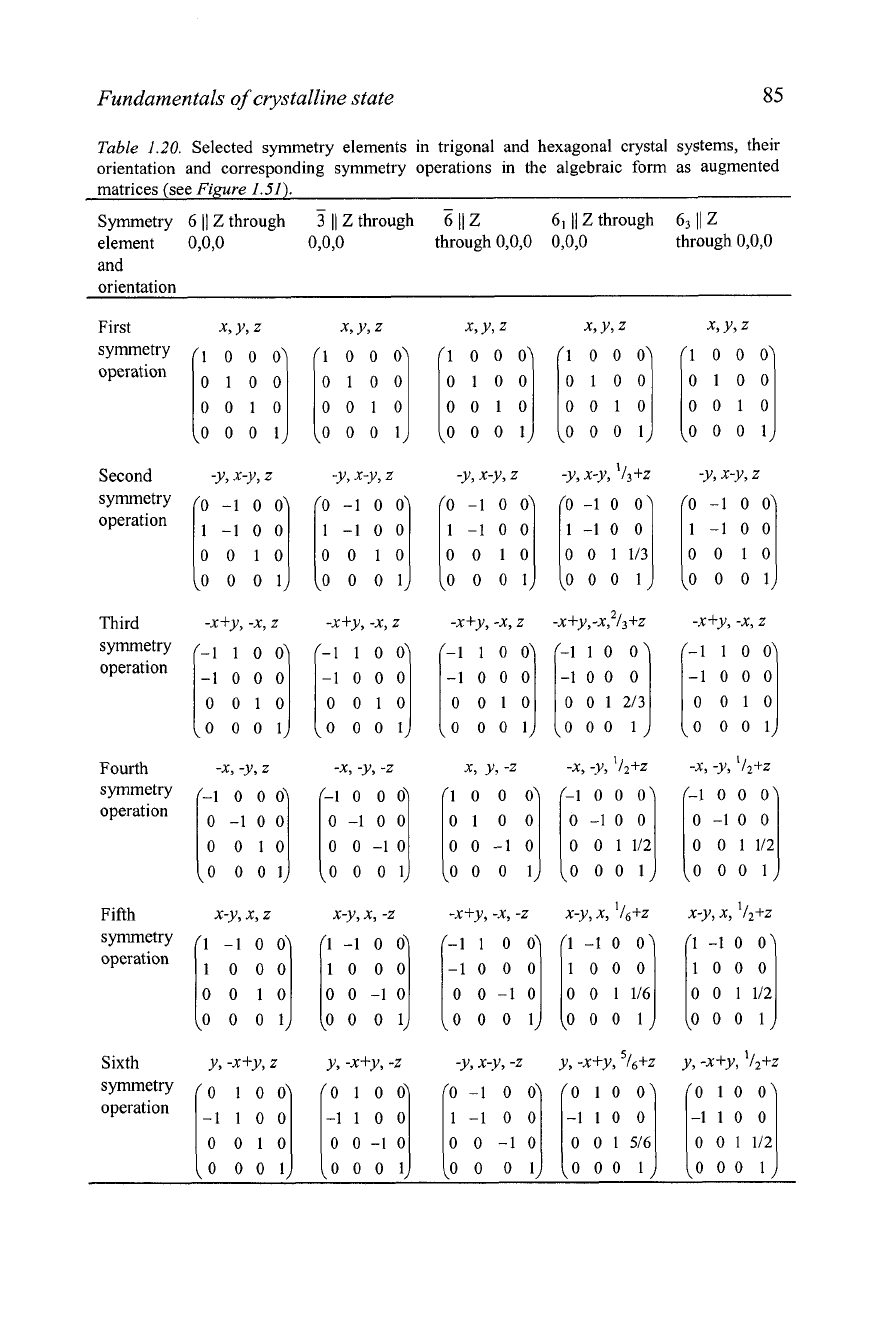
Table
1.20.
Selected symmetry elements in trigonal and hexagonal crystal systems, their
orientation and corresponding symmetry operations in the algebraic form as augmented
matrices (see
Figure
1.51).
-
-
Symmetry
6
11
Z
through
3
1)
Z
through
6
1)
Z
6'
11
Z
through
63
11
Z
element
0,0,0 0,0,0
through
0,0,0 0,0,0
through
0,0,0
and
orientation
First
x,Y, z
symmetry
1 0 0 0
operation
I
0100
0010
0001
Second
-Y, X-Y, z
-y, x-y, z -y, x-y, '13+z -y, X-y, z
0-1
ool
[-lo
O]
00
1-100
1-100 1-100
00 10
001113 00 10
0001
0001 0001
-x+y, -x, z -X+~,-X,~/~+Z -x+y, -x, z
symmetry
0
-1
0 0
operation
I
1-100
0 0 10
0 0 01
Third
-x+y, -x, z
operation
Fourth
-31,
-Y,
z
operation
Fifth
x-Y, x,
z
symmetry
1 -1 0 0
operation
I
10
00
0 0 10
0 0
01
x-y, x, -z
1-100
I;:;!
Sixth
Y, -x+Y, z y, -x+y,
-z
symmetry
0 1 0 0
operation
I
-1100
0010
0 001

86 Chapter
I
Even though the column (Table 1.19) and the row (Table 1.20) labeled
"First symmetry operation" seem redundant, their presence highlights the
fact that each symmetry element contains one-fold rotation or unity
operation. Furthermore, as easy to see from these tables, four-fold rotation
axes also contain the two-fold symmetry operation
(e.g. the third symmetry
operation for 4
11
Z
is the same as the second symmetry operation for
2
11
Z)
and six fold rotation axes contain both three- and two-fold rotations (e.g.
second and third symmetry operations for
6
11
Z
are identical to second and
third for
3
11
2,
while the fourth symmetry operation for 6
11
Z
is identical to
the second for
2
11
Z).
1.20.7
Interactions between symmetry operations
We now consider how the two interacting symmetry operations produce a
third symmetry operation, similar to how it was described in section 1.6 but
now in terms of their algebraic representation. Assume that two symmetry
operations, which are given by the two augmented matrices
A'
and A', are
applied in sequence to a point, coordinates of which are represented by the
augmented vector V. Taking into account Eq. 1.48, but written in a short
form, the first symmetry operation will result in the vector
V'
given as
The second symmetry operation applied to the vector
V'
will result in the
third vector,
v2
as follows
Recalling, that the associative law holds for symmetry operations and for
symmetry groups (see sections 1.6 and
1.7),
equation 1.50 can be rewritten
as
It follows from Eq. 1.51 and from our earlier consideration of
interactions between symmetry elements, finding which symmetry operation
appears as the result of consecutive application of any two symmetry
operations is reduced to calculating the product of the corresponding
augmented matrices. To illustrate how it is done in practice, consider Figure
1.16 and assume that the two-fold axis is parallel to
Y.
The corresponding
symmetry operations,
A'
and At, are (Table 1.19):
Fundamentals
of
crystalline state
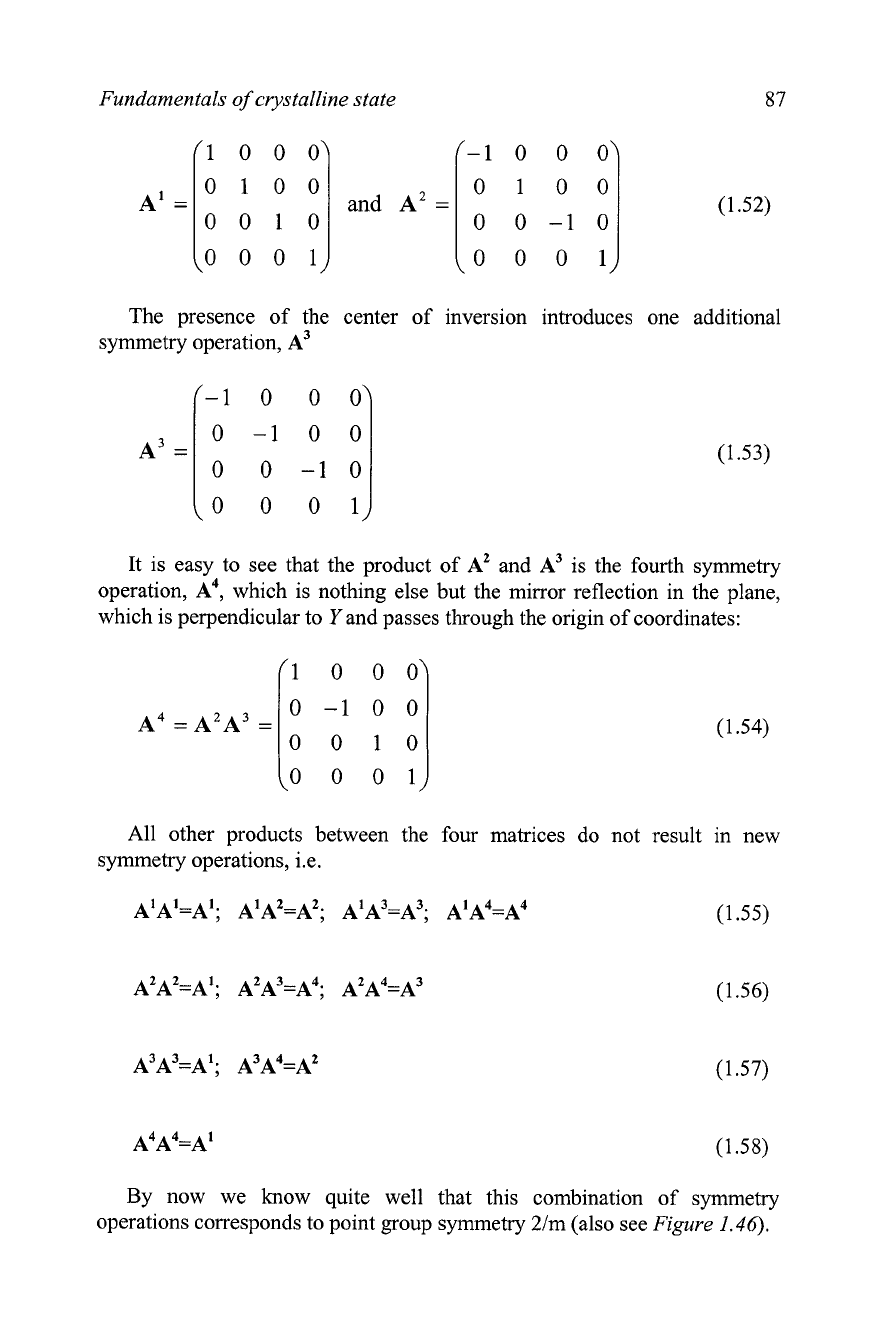
The presence of the center of inversion introduces one additional
symmetry operation,
It is easy to see that the product of
and
is the fourth symmetry
operation,
A~, which is nothing else but the mirror reflection in the plane,
which is perpendicular to
Y
and passes through the origin of coordinates:
All other products between the four matrices do not result in new
symmetry operations, i.e.
By
now we know quite well that this combination of symmetry
operations corresponds to point group symmetry 2/m (also see
Figure
1.46).

Chapter
1
1.21
Non-conventional symmetry
Conventional crystallography was developed using the explicit
assumption that crystalline objects maintain ideal periodicity in three
dimensions. As a result, any ideal three-dimensional crystal structure can be
described using a periodic lattice and one of the 230 crystallographic space
groups (see section 1.16). The overwhelming majority of both naturally
occurring and synthetic crystalline solids are indeed nearly ideal. Their
diffraction
patterns are perfectly periodic since Bragg peaks are only
observed at the corresponding points of the reciprocal lattice, which reflects
both the symmetry and three-dimensional periodicity of the crystal lattice.
Long ago the first aperiodic crystal was reported,' and the apparent absence
of the three-dimensional periodicity of diffraction patterns was later found in
a number of materials. One of the most prominent examples is the 1984
discovery of the five-fold symmetry in the diffraction pattern of rapidly
cooled A10.86Mn0.14 alloy.2 Supported by many experimental observations,
several approaches to describe the symmetry of aperiodic structures have
been developed and successfully used to establish the crystal structure of
these unusual materials.
Probably the most fruitful method has been suggested by
P.M.
de Wolff
in which more than three physical dimensions are used to represent the
crystal lattice and thus to restore its periodicity in the so-called superspace.
Then the resulting aperiodic diffraction pattern is simply a projection of the
crystal lattice, which is periodic in the superspace, upon the physical space,
which is three-dimensional. The diffraction pattern of an aperiodic crystal
usually contains a subset of strong
(i.e. highly intense) diffraction peaks,
which are called main peaks, and their indices are described using three
integers corresponding to a standard three-dimensionally periodic crystal
lattice. The subsets of the so-called satellite peaks are weaker and their
indices include more than three integers to reflect the increased
dimensionality of the superspace (see the footnote on page 401).
1.21.1
Commensurate modulation
Consider the simplest case, when the periodicity of the crystal lattice is
perturbed in one dimension by periodic deviations of atoms from their ideal
'
U.
Dehlinger, iiber die Verbreiterung der Debyelinien bei kaltbearbeiteten Metallen,
Z.
Kristallogr.
65,
6
15
(1
927).
D.
Shechtman,
I.
Blech, D. Gratias and J.W. Cahn, Metallic phase with long-range
orientational order and no translational symmetry, Phys. Rev. Lett.
53,
195
1
(1984).
P.M. de Wolff, The pseudo-symmetry of modulated crystal structures, Acta Cryst.
A30,
777
(1 974).
Fundamentals
of
crystalline state 89
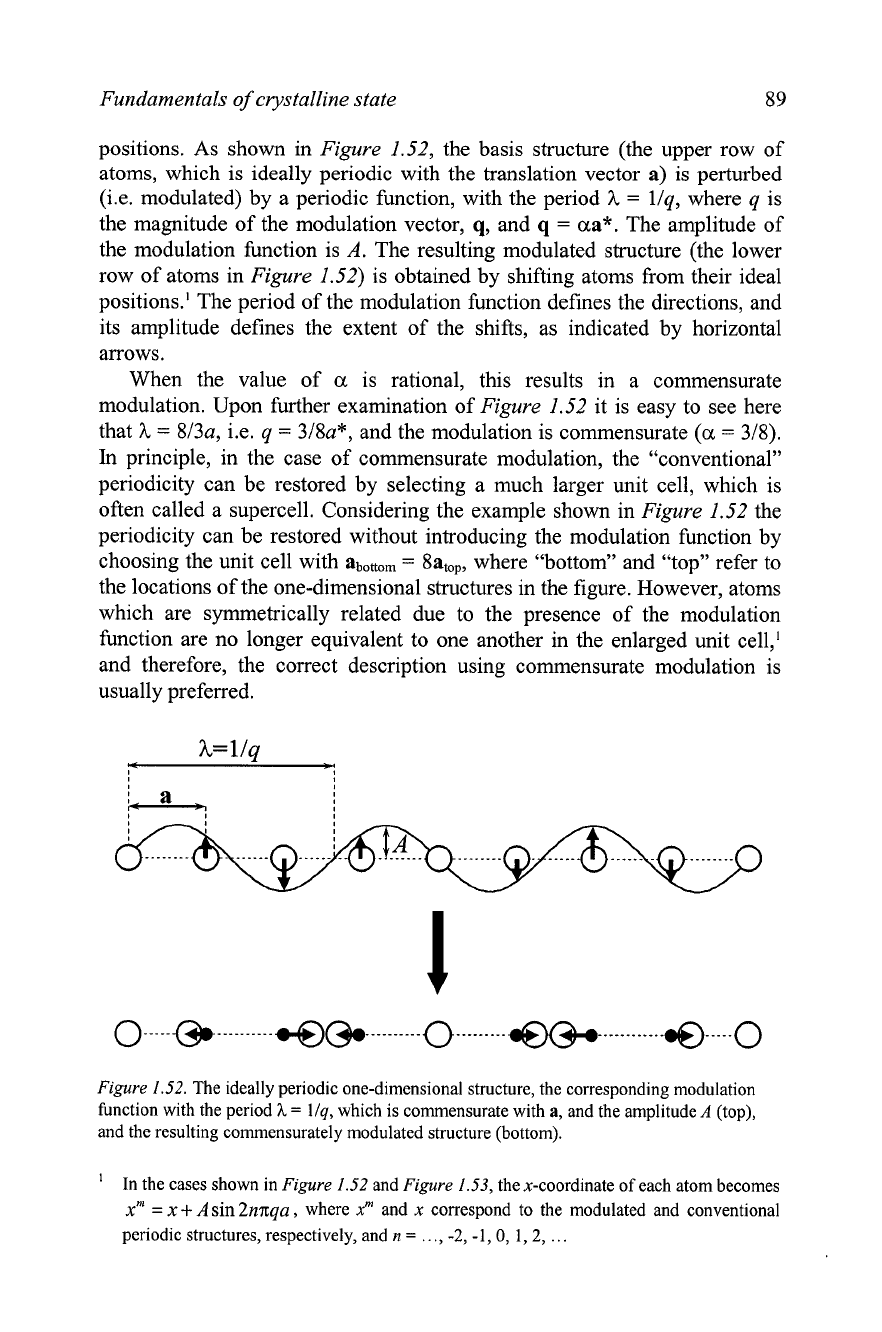
positions. As shown in Figure 1.52, the basis structure (the upper row of
atoms, which is ideally periodic with the translation vector a) is perturbed
(i.e. modulated) by a periodic function, with the period
h
=
llq, where q is
the magnitude of the modulation vector, q, and q
=
aa*. The amplitude of
the modulation function is
A.
The resulting modulated structure (the lower
row of atoms in Figure 1.52) is obtained by shifting atoms from their ideal
position^.^
The period of the modulation function defines the directions, and
its amplitude defines the extent of the shifts, as indicated by horizontal
arrows.
When the value of
a
is rational, this results in a commensurate
modulation. Upon further examination of Figure 1.52 it is easy to see here
that
h
=
8/3a, i.e. q
=
318a*, and the modulation is commensurate (a
=
318).
In
principle, in the case of commensurate modulation, the "conventional"
periodicity can be restored by selecting a much larger unit cell, which is
often called a supercell. Considering the example shown in Figure 1.52 the
periodicity can be restored without introducing the modulation function by
choosing the unit cell with
abottom
=
8atop, where "bottom" and "top" refer to
the locations of the one-dimensional structures in the figure. However, atoms
which are symmetrically related due to the presence of the modulation
function are no longer equivalent to one another in the enlarged unit
cel1,l
and therefore, the correct description using commensurate modulation is
usually preferred.
Figure
1.52.
The ideally periodic one-dimensional structure, the corresponding modulation
function with the period
h
=
llq,
which is commensurate with
a,
and the amplitude
A
(top),
and the resulting commensurately modulated structure (bottom).
'
In the cases shown in
Figure
1.52
and
Figure
1.53,
thex-coordinate of each atom becomes
xn'
=
x
+
A
sin
2nnqa,
where xm and x correspond to the modulated and conventional
periodic structures, respectively, and
n
=
..
.,
-2,
-1,0,
1,2,
.
..
