Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

428

9.4.

9.5.

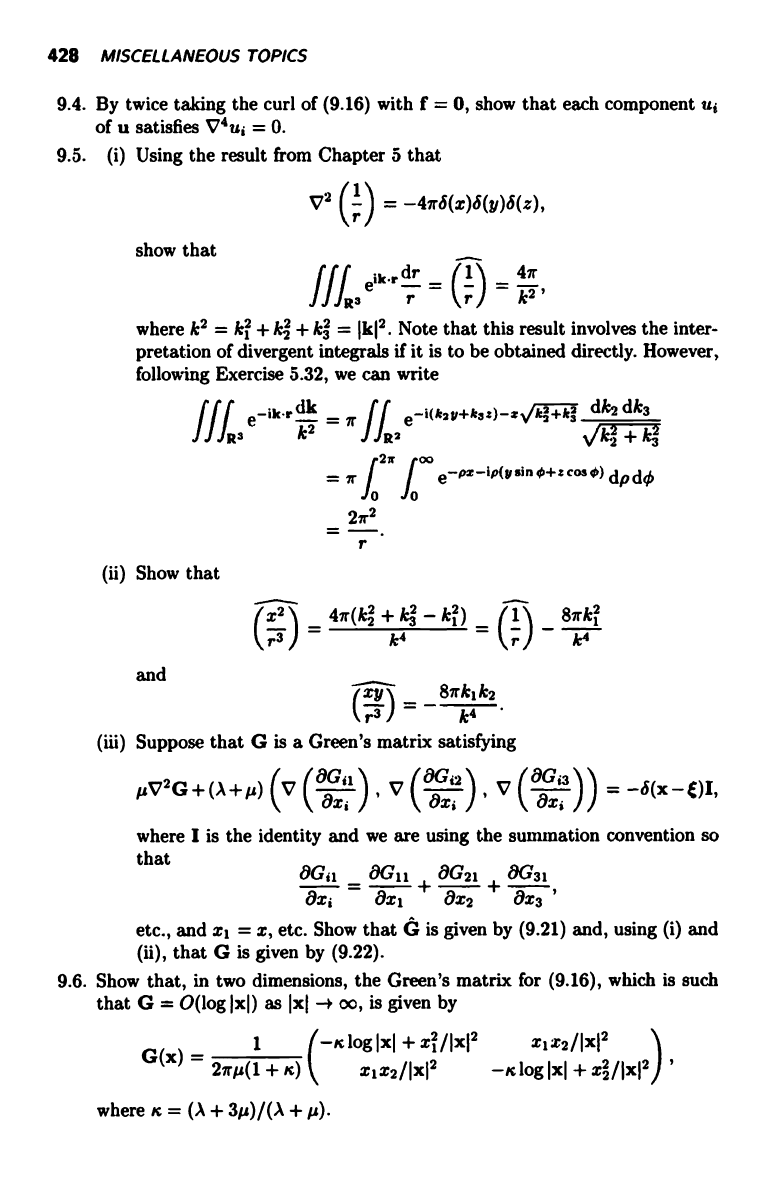
(ii) Show that

2x2

x2\1

47r (k22 + kk - kl)

87rk1

Cr3 k4

MISCELLANEOUS TOPICS

By twice taking the curl of (9.16) with f = 0, show that each component u;

of u satisfies V 4 u; = 0.

(i) Using the result from Chapter 5 that

D2 I r I

= -4ab(x)b(y)a(z),

show that

111

'e,krdr-rrl=k2,

where k2 = kl + Ice + k3 = Jk12. Note that this result involves the inter-

pretation of divergent integrals if it is to be obtained directly. However,

following Exercise 5.32, we can write

e-ik-rdk

= x

f r e-i(kSv+k,t)-:

k;+t-;

dk2 dk3

fffs

&2 k3

= e-pz-i0(ysin0+tc030)dpdq,

0 0

and

8xklk2.

(iii) Suppose that G is a Green's matrix satisfying

pV2G+(.1+µ)

(v (I)

, v (8 2)

' V 8

-&(x-F)I,

Ox;

where I is the identity and we are using the summation convention so

that

OGil OGII OG21

8G31

8xi - Oxl + Ox2 + Ox3

etc., and xl = x, etc. Show that G is given by (9.21) and, using (i) and

(ii), that G is given by (9.22).

9.6. Show that, in two dimensions, the Green's matrix for (9.16), which is such

that G = O(log Ixi) as jxi - oo, is given by

G(x) -

rc log lxi + xi /1x12

xlx2

/1x12

2xp(1 + r.)

(

xlx2/Ix12 -r. log IxI + x2/Ix12

where is = (A + 3p)/(A + p).

EXERCISES 429

9.7. Suppose that

y) satisfies

O2+2-0

for y > 0, with Cauchy data

190

2+62' 8y

on y = 0 (compare this with the example on p.46). Show that, when

`complexified' as on p. 405, the resulting problem for 0 in terms of y and Y)

for y > 0, with

024

020

= 0

aye

E 00

= o

=_rj2'

on y = 0. Draw the characteristic diagram, describe the propagation of the

singularities at t) = ±5, y = 0, and deduce that the original problem is

ill-posed.

9.8. Consider the steady-state thermistor equations of §9.4.1 in the rectangle

0 < z < a, 0 < y < b. Suppose that the boundary conditions are

8; 8T

= 0

on the sides

y-0'b'

so that these sides are both thermally and electrically insulated, and

0=0, T=To atx=0, ¢=V, T=To atx=a,

so that these ends are held at constant temperature To while a potential

difference V is applied across the device. Show that there is a one-dimensional

symmetric solution in which 0 = V/2 on the centre line x = a/2, which is

also the hottest part of the device, provided that

/'O0 dt

V < 8

J 0

Q(t) .

Remark. This solution can be shown to be unique. Now suppose the time-

independent thermistor equations are solved in a two-dimensional region D

whose boundary is divided into four parts on which the boundary conditions

above apply, alternately constant Dirichlet and homogeneous Neumann. Be-

cause the equations with these special boundary conditions are invariant

under conformal maps, D can be mapped onto a rectangle in which the

solution is as above. Thus the level curves of T and 0 coincide, so that

T = T(¢), and the above restriction on V is necessary for existence of a

solution, independently of the geometry.

430 MISCELLANEOUS TOPICS

9.9. (i) Show that the time-independent space charge equations of §9.4.2 have

a solution in which 0 = logr + 92 and p = -2/r2, and that the stream

function is t' = 28/r2.

(ii) Show that p can be eliminated from the time-independent space charge

equations to give the third-order equation

V.(V20V

(V20

Suppose that, in two dimensions, the equipotentials 0 = constant coin-

cide with the level curves of a harmonic function 1, so that

= F(+).

Deduce that

V1 VJV4'I

log(F'(4;)F"(4;)) G(4;), say.

Writing = 2 (w(z) + w(x) ), so that Q4 = 2 (w' + w', i(w' - m'))T,

show that

w"(z)

01(z)

(w' (w'(z))z

Differentiate with respect to z and then 2 to show that the only possible

form for G is G(4) = a4+ + b for real constants a and b. Hence show that

(w'(z))2

taw

+ k,

where k is a complex constant, and show that b = k + k. Finally, show

that w is determined by

z = cl r e-k.- }as2

do,

where cl is a constant, and that 0 and 4 are related by

f 4

1/2

0 = c2 J

e'61_ 0"

dt) dl;,

where c2 is purely real if the inner integral is positive.

Remark. Part (ii) gives a three-parameter family of solutions, parametrised

by a, b and k (the constants cl and c2 can be scaled out). Further properties

of these solutions are described in the paper 'Congruent harmonic functions

and space charge electrostatic fields', IMA J. Appl. Math., 39,189-214,1987,

by S. A. Smith. Unfortunately, they do not satisfy any physically convenient

boundary conditions.

9.10.

(i) Show that, when Ou/8t = 0, (9.42) can be written as

1ViuI2-uA(VAu)=-Vp

and hence that p +

is constant on a characteristic dx/dt = u.

EXERCISES 431

(ii) Show that, if u = V4' in (9.42), then the gradient of (9.51) is zero.

9.11. In Fig. 9.3, denote P by x(t) and P' by x(t) + Ew(x(t), t). In a small time

bt, P moves to Q and P to Q', where Q is x(t) + u(x, t)bt and Q' is x(t) +

ew(x(t), t) + u(x(t) + ew(x(t), t))bt. Deduce that

QQ'-PP'=ew-Vubt,

to lowest order in bt. Now show that (9.48) implies that

Ew (x(t + bt), t + bt) - ew (x(t), t) = E(w - V )U bt;

deduce that P'Q' = Ew(x(t + bt), t + bt) and hence that, if PQ lies along w

at t, then P'Q' lies along w at t + bt.

9.12. Show that, if a and a' evolve according to (9.52) and

Aa = a',

then

- -a= ((Aa) V)u-A(a-V)u

+(u-V).

Deduce that, in two dimensions at least, dA/dt satisfies (9.53).

9.13. Suppose that u and w satisfy the degenerate quasilinear system

w=VAu, V u=0,

and that data is to be prescribed on z = 0 for a solution in z > 0. Show that

a knowledge of the tangential components of u there determines the normal

component of w, and hence that prescribing u and w on z = 0 would lead

to an overdetermined problem.

Remark. It can be shown that it is sufficient for just three pieces of scalar

information to be prescribed on z = 0. For example, we could prescribe u

or, more likely, the normal component of u and the tangential components

of w.

9.14. Suppose that a sprung piston at x = X (t) oscillates with small amplitude

about x = 0 in a tube under the action of a pressure -p. The model is

d2X

02X

= 1,p,

dt2

where p is given by the acoustic model

2 2

P= 8

1Z=O'

020

a0-.

for x > 0.

Show that solutions are possible in which 0 = R Ae'ikz',ei, which corre-

sponds to outgoing waves as x -+ +oo, as long as ao = w/k and w =

(11 VI + iv/k. The fact that w is complex means that the energy radiating

to x = +oo damps the piston motion.

432 MISCELLANEOUS TOPICS

9.15. Suppose d,, p and u satisfy (9.56)-(9.58), with k > 0. Show that solutions

are possible in which

O=R

(Ae-y K -k +!Kz)

for some constant K. It can be` shown that these solutions are physically

acceptable as long as

t K ---k7 > 0 for IKI > k, and K - k < 0 for

IK) < k, the latter being a manifestation of the radiation condition. Show

that

(cK2 - k2) K2 - k2 - vk2 = 0,

and hence that, for small v (i.e. when the membrane is only slightly affected

by the fluid), either

c < 1 and K is clos e to

(- )2k

\\\\

1 c '

or c > 1 and either

K i t

, + ivc

oses c o

(

K is cl

se to

k I 1 +

)

2k2 (C - 1)2

Remark. The physical interpretation of this result is that either waves can

propagate `subsonically' along the membrane and decay as y increases in the

fluid, or they can propagate supersonically and either (i) radiate into the

fluid and decay as x increases or (ii) not radiate and decay as y increases.

(The authors are grateful to Dr R. H. Tew for this example.)

9.16.

(i) When water flows in a shallow, nearly horizontal, layer of depth ,j(x, t)

with velocity u(x, t), (7.28) and (7.29) become, after expanding 0 as a

power series in y,

017 ft

8x+a+EUO =0,

8t+8

Wuq)+38 3)

respectively, where a is a small parameter. Show that, when we set x-t =

and r = et, we obtain

+ e

(au

+ u

8u)

er8t' 0'

8t;(u-?I)+e(8t +8£t

+38

) -0,

and deduce the KdV equation in the form

222 +833

as the lowest-order approximation for small e.

EXERCISES 433

(ii) When the shallow layer in (i) is overlain by a half-space of light fluid,

the equations for u and it can be resealed to become

ax+ 8

+fua

-0,

57,

(

ax.

where p is the pressure at the base of the lighter fluid. If the lighter fluid

flows irrotationally with velocity potential , then, after linearisation in

the usual way, it follows that, to lowest order,

p+ LO+17 =0,

ony=0,

where

0 in y > 0. Show that

am a)

ax ay

ony =

where 7 l denotes the Hilbert transform, and follow the argument of (i)

to derive the Benjamin-Ono equation in the form

/ 2

2IT+3 81

-8 -?{1

at2I)

-0.

9.17. For the KdV equation in the form

+ 6u

ax3

= 0,

show that a travelling wave solution in which u = u(x - ct) exists for all

c > 0, and that

u=2sech2(v'(x-2

a)).

Show also that sech (f (x - ct)/2) is the principal eigenfunction for (9.78)

in this case.

Remark. It can be shown that, when u(x, 0) = (c/2)sech2 (fx/2), the dis-

crete spectrum of (9.78) is the single point c/4.

9.18. For the Sine-Gordon equation

a2u a2u

axe

ate

=sin u,

show that there are travelling wave solutions symmetric about x = ct and

that

4 tan- I tanh ((x - ct) /2 c2-1), c>1,

p ((

)/ vc2 tan-1 ex p

2 -1 c<1.

Conclusion

We will conclude by recapitulating what we think are the principal lessons to be

learned from an overview of what we have tried to describe in this book.

The first is the concept of well-posedness, so dramatically illustrated by the

contrast between the Cauchy problems for elliptic and hyperbolic equations. Even

the simplest examples in §3.1 give a reliable guide of how a change in sign in one

term in the equation can change all the rules of the game. It is our biggest source

of regret that it is only at all easy to give a general answer to the question of

well-posedness for hyperbolic equations and that the reality or otherwise of the

characteristics can lead to such a dichotomy; maybe in years to come, the theory

of complex characteristics will have developed to an extent that this distinction is

at least more blurred.

The second, closely related topic is that of the qualitative nature of the solu-

tion. It is always important to ask questions like `can the equation admit "wave

solutions" that propagate in the interior of the domain, as in Chapter 4, or does the

equation immediately smooth away any irregularities in the data, as in Chapters 5

and 6?'

Concerning the representation of solutions, the only all-embracing concept to

emerge is that of the formal solution of an arbitrary linear equation in terms of the

inverse of the differential operator. This is an integral of the relevant data weighted

with a suitable Green's or Riemann function, unless the Fredholm Alternative

dictates otherwise.

No such general principles apply when it comes to writing down explicit solu-

tions of partial differential equations. It is always a red-letter day when an explicit

solution emerges in an uncontrived situation and it reflects some kind of symmetry

or invariance property. However, the latter may be even harder to discern than

the solution itself. In this respect, we can only hope that the reader has not been

daunted by the plethora of tricks that have been invented to treat special types of

equation on a case-by-case basis, even though the list of those we have described

is far from exhaustive; we have only tried to describe those devices that seem to

offer most insight and generality.

There are two important aspects of the mathematics of partial differential

equations that have been woefully underemphasised in this book. The first is any

discussion of `perturbation methods'. As mentioned in the Introduction, this would

have been possible at the cost of a change in character and size. The great potential

of the ideas that we have excluded can be glimpsed in Chapter 7, where stability

theory was seen to be the basic tool for building up a theoretical framework for free

boundary problems, and in Chapter 8, where the Geometric Theory of Diffraction

is a vital aid to the understanding of high-frequency wave propagation.

434

CONCLUSION 435

The second lacuna is the lack of any treatment of the relationship between what

we have expounded and the understanding that can be obtained from numerical

computations. Again, it would have been possible, with help, to have doubled the

length of the book by including in each chapter comments about algorithms, con-

vergence, error estimates and stability analysis. Or we could have simply included

figures obtained by the easy route of attacking the differential equation with the

best available software. Apart from our desire for brevity, our only justification for

these omissions is that the delicacy of so many of the situations we have encoun-

tered demands the need for a quality control that only a mathematical approach

can provide.

We also thought about including more discussion of the implications of the

mathematics that motivated the individual equations. In many cases these can

be quite startling, ranging from the prediction of unexpected instabilities to sug-

gestions for easy and reliable algorithms that enable processes to be optimised.

But this, along with greater emphasis on the theoretical aspects, would have made

greater demands on the reader than we wanted to impose.

At the end of the day, the feature of writing this book that has given the au-

thors most pleasure has been the exposition, in fairly simple mathematical terms,

of the quantitative understanding of so many phenomena of practical importance

in everyday life. We know of no other branch of theoretical science where so many

situations could be modelled and analysed in anything like four hundred pages,

starting more or less from scratch. However, the seemingly comprehensive success

of partial differential equations in this respect must not let students be lulled into

a false sense of security. For one thing, they are rather special models that are

much better behaved in general terms than, say, discrete models. Also, it must al-

ways be remembered how dangerous it is to step away from those situations where

analytic equations are used in conjunction with analytic solutions. Although we

have only encountered one example (on p.67) where the whole theory crashes in

the face of non-analyticity, the literature contains many cases where artificially

introduced singularities can destroy all intuition about classification and qualita-

tive behaviour. Our discussion in Chapter 7 has shown that even the most prosaic

examples of models with discontinuous coefficients can lead to situations where,

with our current knowledge, there is no systematic way to make mathematical

sense of the partial differential equations.

References

The number(s) in parentheses indicate the page(s) on which the reference is cited.

[1] Arnol'd, V. I. (1984). Catastrophe theory. Springer-Verlag, New York.

[369]

[2] Arscott, F. M. (1964). Periodic differential equations. An introduction to

Mathieu, Lame, and allied functions. Pergamon Press, Oxford.

[119]

[3] Bender, C. M. and Orszag, S. A. (1978). Advanced mathematical methods for

scientists and engineers. McGraw-Hill, Tokyo. [88]

[4] Birkhoff, G. and Zarantonello, E. H. (1957). Jets, wakes and cavities. Aca-

demic Press, New York.

[342]

[5] Bleistein, N. (1984). Mathematical methods for wave phenomena. Academic

Press, London.

[364, 373]

[6) Bonnet, M. (1997). Boundary integral equations methods applied to solid and

fluid mechanics. Wiley, New York.

[174]

[7] Buckmaster, J. D. and Ludford, G. S. S. (1982). Theory of laminar flames.

Cambridge University Press.

[316]

[8) Carrier, G. F., Krook, M. and Pearson, C. E. (1966). Functions of a complex

variable. Theory and technique. McGraw-Hill, New York. [109,181,182]

[9] Carslaw, H. S. and Jaeger, J. C. (1959). Conduction of heat in solids (2nd

edn). Clarendon Press, Oxford. [254]

[10] Chester, C. R. (1971). Techniques in partial differential equations. McGraw-

Hill, New York. [394]

[11] Coddington, E. A. and Levinson, N. (1955). Theory of ordinary differential

equations. McGraw-Hill, New York. [396]

[12] Courant, R. and Hilbert, D. (1962). Methods of mathematical physics, Vols I

& U. Interscience, New York.

[47,169, 184, 334, 383]

[13] Crank, J. (1984). Free and moving boundary problems. Clarendon Press, Ox-

ford. [335]

[14) Doering, C. R. and Gibbon, J. D. (1995). Applied analysis of the Navier-

Stokes equations. Cambridge University Press, New York.

[413]

[15] Drazin, P. G. and Johnson, R. S. (1989). Solitons: an introduction. Cambridge

University Press.

[141, 425]

[16] Driscoll, T. A. and Trefethen, L. N. (2002). Schwarz-Christofel mapping.

Cambridge University Press.

[342]

[17] Elliott, C. M. and Ockendon, J. R. (1982). Weak and variational methods for

moving boundary problems. Pitman, London.

[213, 332,335, 336]

436

REFERENCES 437

Engl, H. W., Hanke, M. and Neubauer, A. (1996). Regularization of inverse

problems. Kluwer, Dordrecht.

[393]

Erdelyi, A., Magnus, W., Oberhettinger, F. and Tricomi, F. G. (1954). Tables

of integral transforms, Vol. I. McGraw-Hill, New York.

[124]

Gakhov, F. D. (1966). Boundary value problems. Pergamon Press, Oxford.

[211]

Garabedian, P. R. (1964). Partial differential equations. Wiley, New York.

[184, 405]

Hinch, E. J. (1991). Perturbation methods. Cambridge University Press.

[88, 318,406]

John, F. (1971). Partial differential equations. Springer-Verlag, New York.

[32]

[33]

[34]

[35]

[36]

[37]

[38]

[68]

Jones, D. S. (1986). Acoustic and electromagnetic waves. Clarendon Press,

Oxford.

[209]

Kevorkian, J. and Cole, J. D. (1981). Perturbation methods in applied math-

ematics. Springer-Verlag, New York.

[881

Milne-Thomson, L. M. (1948). Theoretical aerodynamics. MacMillan, London.

[403]

Milne-Thomson, L. M. (1949). Theoretical hydrodynamics (2nd edn). MacMil-

lan, London.

[155,198, 402]

Moffatt, H. K. and Tsinober, A. (1990). Topological fluid mechanics. Proceed-

ings of the IUTAM Symposium. Cambridge University Press.

[417]

Ockendon, H. and Ockendon, J. R. (1995). Viscous flow. Cambridge Univer-

sity Press, New York.

[161, 409]

Ockendon, H. and Tayler, A. B. (1983). Inviscid fluid flows. Springer-Verlag,

New York. [23,53]

Oksendal, B. (1992). Stochastic differential equations (3rd edn). Springer-

Verlag, New York.

[244, 395]

Piaggio, H. T. H. (1946). An elementary treatise on differential equations and

their applications. G. Bell & Sons Ltd, London.

[360]

PGlya, G. and Szego, G. (1951). Isoperimetric inequalities in mathematical

physics. Princeton University Press. [212]

Polyanin, A. D. (2002). Handbook of linear partial differential equations for

engineers and scientists, Chapman & Hall/CRC, Boca Raton.

[174]

Protter, M. H. and Weinberger, H. F. (1967). Maximum principles in differ-

ential equations. Prentice Hall, Englewood Cliffs, NJ. [249]

Renardy, M. and Rogers, R. C. (1993). An introduction to partial differential

equations. Springer-Verlag, New York. [68]

Richards, I. and Youn, H. (1990). Theory of distributions: a non-technical

introduction. Cambridge University Press. [97]

Rubinstein, I. and Rubinstein, L. (1993). Partial differential equations in clas-

sical mathematical physics. Cambridge University Press.

[329]