Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

388

NON-QUASILINEAR EQUATIONS

(ii) Suppose that such a ray extends to infinity. Show that

AST

(x2 +

y2)1/2

so that the 'directivity' of the field radiated to infinity is A02 T. (We used

this result in §5.6.2.)

(iii) Suppose that u = 0 and A = 1 on the initial curve r. Show that on

each ray

A = p(s)

p(s) + t'

where p(s) is the radius of curvature of I' at the point at which the ray

leaves it. (It will help to take s to be arc length along I', so that the

curvature is 1/p = yox'o - xoyo.) Show that this implies that, in the

absence of caustics, as t --> oo,

A -+

Ao (gox'o - Polio)

1/2

r1/2 \ qoA - pogo'

where Ao(s) is the initial amplitude. Deduce that, when uo(s) = 1,

Arl/2 is eventually inversely proportional to the curvature at the launch

point. (In three dimensions it can be shown that it is the inverse of the

Gaussian curvature that controls the far-field directivity; hence it is

necessary to solve a Monge-Ampere equation in order to deduce the

shape of the body from its scattered field.)

8.12. Suppose that ao is constant and

ao ((8

)2 +

)2) _

()2,

with Cauchy data u = uo(x, y) at r = 0. Show that po, qo and vo (where

v = 8u/8r) are given by

8uo 8uo

f VrIT+--qoy

Po - 8x , qo - 8y ,

vo =

Deduce that the solution is

u(z, y, r) = uo(X, Y),

where

2vot

x=2pot+X, y=2got+Y, r=-

a .

If uo is localised near x = y = 0, show that the solution is localised near the

circle x2 + y2 = aot2.

EXERCISES 389

Remark. When used as an approximation to the solution of

02'1 _ 2 (52,0

a2w\

ate

a°

ax2 aye J

this result appears to violate Huygens' principle (see §4.6.2). However, the

WKB approximation only describes very rapidly varying solutions and does

not pick up more gradual variations inside the characteristic cone x2 + y2 =

aot2.

* 8.13. For the 'inhomogeneous wave equation'

020

_ 2 (a20 a20\

ate -

ao(y)

ax2 + eye J

which is a model for sound propagation in an ocean or atmosphere whose

density varies vertically, show that the ray cones are ¢(x, y, t) = constant,

where

r \ 1

= (L,)2,

and that the bicharacteristics satisfy

dt r

= A aT = q,

aT = - QD ,

dp_

0dq_r2 d

1 dr-0,

dr ' dr 2 dy \a0}'

where r = 00/0r. Show that in t > 0 the projections of the rays through

the origin are normal to the projections of the cross-sections t = constant of

the ray cones through the origin.

When ao = 1/(l + y), for y > -1, show that the ray cone through the origin

where

2r3T2 ,x=pr, y=yr+4r2T2, t=-rT-rvr2- 1

\ 2

p2 + (a-i- 1r 2T)

= (1 +

y)r2.

* 8.14. Suppose 0 satisfies the modified Helmholtz equation

V21p -k27y=0

in the rectangle -1 < x < 1, -a < y < a, with 7jr = 1 on the boundary.

Show that the solution is

_ 2 (-1)n

7r n_on+1/2

(cos((n + 1/2)7rx) cosha,,y + cos ((n + 1/2)7ry/a) cosh $nxl

cosh [tna

cosh $n J

390

NON-QUASILINEAR EQUATIONS

where an = (n + 1/2)2ir2 + k2 and 62 = (n + 1/2)2ir2/a2 + P. Show also

that

0 = 2e -k cosh kx + 2e-k' cosh ky

satisfies the equation, that it is the limit of the exact solution as k -> oo,

and that it approximately satisfies the boundary conditions except near the

corners. If this solution is written as Ae`k" (=,y), show that, to lowest order,

u--(x+l), x-1, -(y+a), y-a

near

x=-1, x=1, y=-a, y=a,

respectively, and compare this with the answer to Exercise 8.1.

Remark. Near the ridge line x = y, 0 is approximately a-kz + e-ky, which

suggests that across a ridge line u is continuous, tending to x on one side

and y on the other, but Vu has a jump discontinuity; specifically,

19u l

- _

On .

_ 8n +

* 8.15. Small-amplitude waves created by a ship moving along the x axis with speed

-V on an ocean z < 0 are modelled by

V20=0 forz<0,

with

8t '

+ g1I = 0

on z = 0,

= 077 00

where 4(x, y, z, t) is the velocity potential and i(x, y, t) is the surface elevar

tion. Show that, if i; = x + Vt,

00 .017+V

e0+V0Gt+gq=0

onz=0.

8z - 8t 84 8t 8c

Now let

Show that

and that

n = R

(? oei(-wt+k1 f+kzy))

O _

Rei(-wt+k,(+k2y)+s k. +ks

92(ki + ks) _ (-w + Vkl)4.

EXERCISES 391

s 8.16. In an optimal control problem for x(t), it is desired to choose u(t) to minimise

a cost fo L(x,u)dt when x evolves according to i = f(x,u). Assuming

sufficient differentiability with respect to x and u, show that

OL(x,x)

_ OL

0f -

8x u

e?te = p,

say,

and

aa(x,.x) Of

Ou(x,x)

_ Of Of

ax au '

--57X/

Deduce from the Euler-Lagrange equation that

d OL(x,i) 0L(x,x)

8L OLOf

ax Ox - d Ox + Ou ax Du

=P-

8x +pax

=0.

Show further that, if H(x, p) is defined to be -L + pf

where L and f are

now thought of as functions of x and p, then

OH(x,p)

_ _aL

(_ OL

Ofl Ou(x,p) -

8a 8x + P 8x +

au +

-P ,

(-8uL +LOf NBx,P)

= 'j;

thus the system is optimally controlled by a solution of Charpit's equations.

Show that if x(0) = xo is prescribed then the second boundary condition is

p(1) = 0.

* 8.17. (i) Fermat's principle states that light travelling from the origin to x in a

medium in which the speed of light is a(x) does so along a path which

minimises the optical path length

u(x) _

r' d8

u u

between the origin and x. Writing the path as x = X(t), so that

u(x) = T

X) dt,

where

X(T) = x, X(0) = 0,

1show

that the Euler-Lagrange equations for X are

d ( :k )

+ 'I['Va = 0,

.= d

Show further that, when r is defined by dr/dt = aIXI, these equations

can be written as

1 V

(a(X)),

= P.

a3 dr

392

NON-QUASILINEAR EQUATIONS

(ii) Show that IVu(X)( = 1/a(X) and that the equations above are Char-

pit's equations for this partial differential equation.

(iii) Show that Charpit's equations are those for a Hamiltonian system in

which

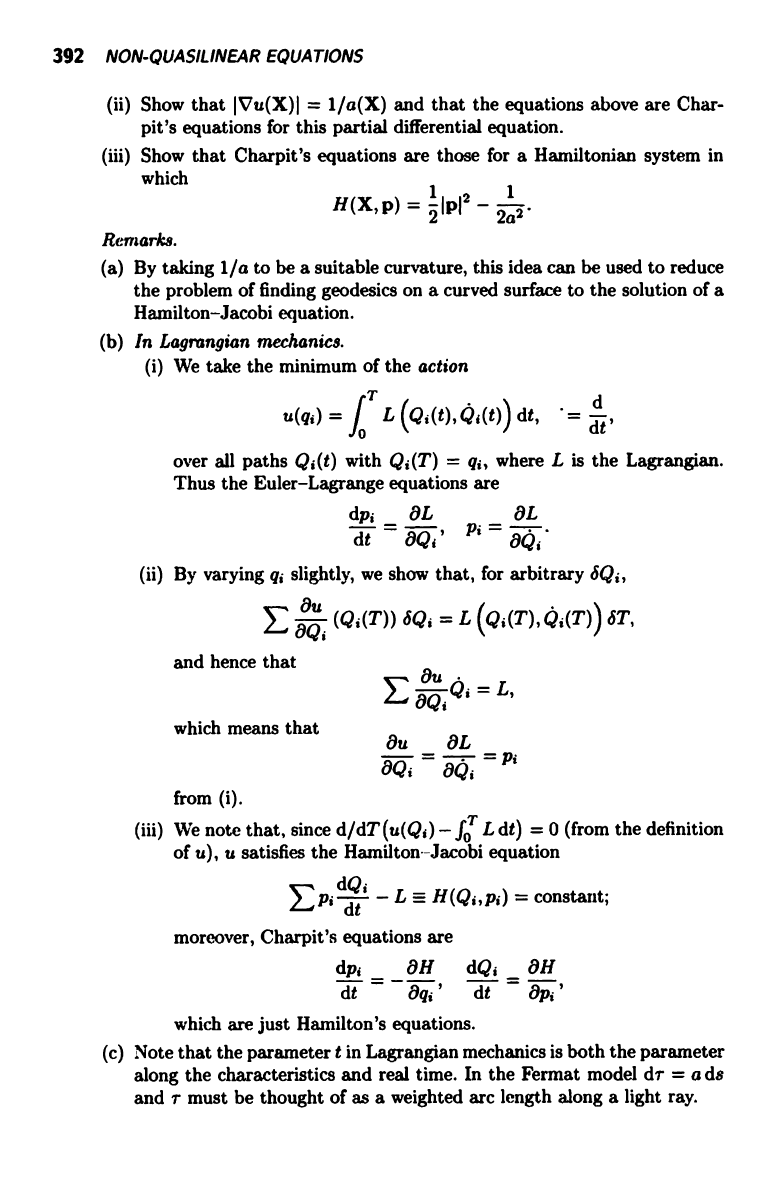
H(X,p)

1pl,

Remarks.

(a) By taking 1/a to be a suitable curvature, this idea can be used to reduce

the problem of finding geodesics on a curved surface to the solution of a

Hamilton-Jacobi equation.

(b) In Lagrangian mechanics-

(i) We take the minimum of the action

u(qi) =

(Q=(t),Qi(t)) dt,

dt'

over all paths Qi(t) with Qi(T) = qi, where L is the Lagrangian.

Thus the Euler-Lagrange equations are

dpi

-aQi, pi=aQi

(ii) By varying qi slightly, we show that, for arbitrary 6Qi,

OQi (Qi(T)) bQi = L (Q1(T),Qi(T)) ST,

and hence that

[[

L Ni Q' =

which means that

Ou OL

aQi

=-. = pi

from (i).

(iii) We note that, since d/dT(u(Q1) - fT L dt) = 0 (from the definition

of u), u satisfies the Hamilton-Jacobi equation

dQ! - L = H(Q,,pi) = constant;

moreover, Charpit's equations are

dpi

dQi OH

dt - - Oqi' dt = api'

which are just Hamilton's equations.

along the characteristics and real time. In the Fermat model dr = ads

and r must be thought of as a weighted arc length along a light ray.

Miscellaneous topics

9.1 Introduction

Our discussion over the past eight chapters has covered much ground but only

scratched the surface of what is known about many methods for special classes of

pdes 188 In this miscellany we will attempt to take the reader further into some

of the `tricks of the trade'. However, before going into detail, we will make some

general remarks about some areas of mathematics that are not only germane to

the classical theory of pdes, but also so large and important as to warrant their

own text books; yet we have scarcely mentioned them thus far.

The first concerns the study of inverse problems, some of which go under the

name of parameter identification. This is a subject whose philosophy is almost

orthogonal to ours in that it starts with observations about a phenomenon that

may be modelled by some pde, and then asks `what are the coefficients and bound-

ary data for the relevant pde?' This is a very difficult procedure for the following

reasons.

1. It is essentially nonlinear; even if a pde is linear, its solutions rarely depend

linearly on its coefficients.

2. It can so easily be ill-posed. For example, suppose that the gravitational poten-

tial 0 produced by a finite two-dimensional body of unknown constant density

p satisfies the Poisson equation

02,0

= -p,

and we can make measurements about this potential on a circle r = R, enclosing

but not touching the body. What observations would be enough to determine

its shape? Clearly, even a knowledge that 84/8r = g is constant on r = R does

not determine q,, because any circular body with radius 2Rg/p is possible.isa

Even more difficult challenges are provided by the determination of unknown

spatially-varying coefficients such as thermal conductivity. When, as is often the

case, the measurements lead to an ill-posed underdetermined problem for the un-

knowns, there is clearly great scope for finding what is likely to be the `best guess'

by using various kinds of regularisation; see [18].

1881n this chapter only, we will abbreviate 'partial differential equations' to 'pdes'.

1691n fact, non-circular bodies are also possible in this case; examples

can be constructed using

the Hele-Shaw model of Chapter 7.

393

394

MISCELLANEOUS TOPICS

In view of these difficulties we will not take this discussion much further, ex-

cept to note those aspects of the theory that we have developed in the previous

chapters that might be of especial value in inverse problems. One commonly used

technique for elliptic equations is to prescribe Dirichlet data and observe the re-

sulting Neumann data, or vice versa. The relationship between these data is a

generalisation of the Dirichlet-to-Neumann map (5.65) and (5.68) and contains

information about the parameters in the pde away from the boundary; hence it

can be of some help in turning parameter identification problems into integral

equations. In another vein, the spectrum of a pde problem involving an eigenvalue

as a parameter may easily be measured in practice by observing or hearing its

`normal modes' of vibration; this then poses the problem of reconstructing the ap-

propriate Helmholtz equation (or whatever it might be) from the spectrum. This

is a notoriously difficult problem, pioneered by a famous paper entitled `Can you

hear the shape of a drum?',170 and it transpires that knowledge of the spectrum

of, say, the Dirichlet problem alone is not sufficient to find the shape. To explain

this and its importance to modern developments in radar and tomography requires

far more knowledge of spectral theory than we were able to present in Chapter 5.

This also applies to the more practically important problem of finding the shape

or properties of a scatterer that is being insonified or irradiated by some incident

wave field, as in §8.2.3. Indeed, the one theory we have encountered that is invalu-

able in such `inverse scattering' problems is that of geometric optics. As long as

the scattering body is much larger than the incident wavelength, the fact that the

fields scattered by arbitrary bodies can be more or less drawn by hand means that

it is pretty easy to build up intuition concerning the causes of any given scattered

field. But the precise mathematical theory at arbitrary wavelength is much more

difficult; some progress can be made in one space dimension, as we shall see at the

end of this chapter.

While inverse problems can easily lead to underdetermined systems, in which

the model is lacking information, the opposite phenomenon of overdetermined sys-

tems can also occur. In particular, such systems can arise where the ideas of

group symmetry are being used, as in §6.5. There we saw that the invariance of a

pde under a certain Lie group (or generalisation thereof) can only be assured if the

functions defining the group are such that all the coefficients in the transformed

pde coincide with those of the original. This inevitably leads to an enormous sys-

tem of pdes for the defining functions, usually much larger in dimension than the

number of defining functions.

An account of overdetermined systems can be found in [10]; here we simply

note that the basic question of whether such a system has any solution at all171

can be approached systematically by repeated cross-differentiation. The idea is

to hit the system with more and more partial derivatives with respect to all

the independent variables, and use the equality of mixed derivatives until either

there is a contradiction or any further differentiation leads to equations which are

170Kac, M. (1966). 'Can you hear the shape of a drum?', Amer. Math. Monthly, 73(4), 1-23.

171Compare, for example, the systems (i) 8u/8x

= 8u/8y = 0 and (ii) 8u/8x = 0, 8u/8y = x

for the single function u(z,y).

LINEAR SYSTEMS REVISITED 395

automatically satisfied. Of course, this is a tedious procedure and much time can

be saved by using symbolic manipulators, which are now often incorporated into

packages that search systematically for group symmetries.

Another area that has gone almost unnoticed in this book concerns the study

of stochastic piles, which is an increasingly important area as far as applications

are concerned. One reason for this is the demand for risk quantification in areas

of human activity ranging from insurance and finance to management and social

policy. Unfortunately, the mastery of the subject makes two demands on the stu-

dent, namely a good knowledge of stochastic processes in general as well as the

development of the calculus needed for continuous-time random processes. In §§1.1

and 6.1 we have given a very crude description of some problems in this area, but

we overlooked both of the issues just mentioned; if we were to take the subject

any further we would immediately have to plunge more deeply into these topics.

Hence we refer the reader to [31].

Our final apologia concerns the lack of discussion of pdes with non-smooth

coefficients. Although we have placed much emphasis on non-smooth boundaries

and boundary data, our only discontinuous-coefficient examples were the oxygen

consumption model of §7.1.2 and the enthalpy formulation of the Stefan problem

of §7.4.2. It is only too easy to assume that, if a pde has discontinuous coefficients,

then the solution can be synthesised by piecing together smooth functions on either

side of some interface. Such an intuitive approach not only relies on the existence

of an interface, but also on its smoothness, and we recall the unexpected mushy

regions that can occur in Stefan problems.172 The whole question of how general

pdes should be interpreted at places where the coefficients are discontinuous is even

more delicate than those arising in the theory of shocks for conservation laws.

This chapter cannot attempt to compensate for the lack of coverage of these

aspects of pdes. Instead, we will end the book by making some observations about

some models and methods that do not fall directly under the headings of Chap-

ters 1-8, but which illustrate the power and limitations of some of the ideas in

those chapters. Inevitably. all the following sections are open-ended.

9.2

Linear systems revisited

We recall that our principal entree into the theory of applied pdes was via quasilin-

ear systems of the type A; 8u/8x; = c which, as suggested in the Introduc-

tion, cover all pdes with sufficiently smooth coefficients and with the coefficient of

the relevant highest derivative being non-zero. We now reconsider some aspects of

such systems in the light of what we have learned in Chapters 4-6. Little new gen-

eral methodology emerges, but several special methods are available for particular

problems.

Not surprisingly, for linear systems, the ideas of using Green's and Riemann

functions that were so successful in Chapters 4-6 can be generalised, but this can

only be done at some technical cost, as shown below.

172Further difficulties could arise in other practical problems, for example, if frictional effects

were modelled by terms whose sign depends on the direction of motion.

396

MISCELLANEOUS TOPICS

9.2.1

Linear systems: Green's functions

First let us recall the situation for systems of ordinary differential equations (odes).

The 'Cauchy problem' for x(t) such that

- A(t)x = b(t) (9.1)

is to find x in t > 0, with

x(0) = x0

prescribed. This can be solved in principle by finding the Green's matrix G(t, r)

such that

-dG-GA

=0 (9.2)

with

G=I att=r,

(9.3)

where I is the identity.113 This formulation for G can easily be seen to be equivalent

dG-

- GA = d(t - r)I

with

G(T, r) = 0

for some T > r,

(9.5)

which is analogous to the Riemann function formulation; equation (9.4) simply

means that

G(r - 0, r) - G(r + 0, r) = I,

and hence

G(r-0,r)=I.

With either definition of G, we can proceed by premultiplying (9.1) by G and post-

multiplying (9.2) by x, as was done repeatedly in Chapters 4-6, and subtracting,

to obtain

dt (Gx) = Gb,

(9.6)

and hence

G(t, r)b(t) dt

x(r) = G(0, r)x(0) +

(9.7)

since G(r, r) = I. Note that, if A = constant, so that'74 G = e-A(t-r) for t < r,

and b tends to a constant vector as r -> oo, then it is easy to see that, as r -* oo,

(9.7) gives

just as long as the eigenvalues of A have negative real parts.

173G is simply related to the so-called fundamental matrix that is often used in texts on odes X111.

t74 We define the matrix exponential by

°O tnA"

ewe

=2_

n=0

-'1

LINEAR SYSTEMS REVISITED 397

Two-point boundary value problems for (9.1) can also be solved in this way.

Suppose, for example, that we have the `Dirichlet' problem when x = (x 1, ... ,

X2.

)T, with (xl,...,xn)T = 0 at t = 0 and t = 1; then

1x(r) = J G(t, r)b(t) dt, (9.8)

where, to satisfy the boundary conditions, G is such that its last n columns vanish

at t = 0 and t = 1. We can define G either by

or by (9.2) and

-dG

_ GA = d(t - r)I

(9.9)

r+0

[G]

r-0 =

(9.10)

It is quite easy to relate (9.7) and (9.8) to the Green's function solutions of

the scalar equations that we described in §§4.2.1 and 5.5.1, respectively. Indeed, if

we were to write any of the scalar linear equations considered in Chapters 4-6 as

first-order systems, as we always could, we would find that the Green's functions

for these problems were simply appropriate entries in the Green's matrix defined

by generalisations of (9.2) and (9.3) or (9.9) and (9.10). However, unless A is a

constant matrix, it is unlikely that (9.1) could be recast as a scalar equation and

thus, in general, the method described above is helpful. The reason for the added

complexity of a matrix rather than a scalar Green's function is that, in order to

solve a system, we need to know how each component of x responds to being driven

by each and every component of b.

Bearing these points in mind, let us now turn to the Cauchy problem for the

linear hyperbolic system

+ Bu = f

for x> > 0,

(9.11)

=i ax'

with

u = 0

on, say, xl = 0; (9.12)

we assume that xi = 0 is not a characteristic as defined in (2.53). To solve this

problem formally, we define a Green's (or R.iemann) matrix as the solution of

- (GA;) + GB = 6(x - C)I, (9.13)

i=1

OXj

the delta function with vector argument being defined as in §4.2, and with G

vanishing on a surface analogous to I' in Fig. 4.1. Then, proceeding as usual,

L>0

8(GAru)

dx = u(f) - J

...I

Gf dx

8x1

0o z,>0

= 0. (9.14)