Ockendon J., Howison S., Lacey A., Movchan A. Applied Partial Differential Equations

Подождите немного. Документ загружается.

378

NON-QUASILINEAR EQUATIONS

* 8.3 Hamilton-Jacobi equations and quantum mechanics

The purpose of this section is to give a brief account of how the theory of partial

differential equations holds the key to the link between classical and quantum

mechanics. It is of course an intricate story and we will only give the very simplest

outline of what is involved.

We begin by recalling that many classical mechanics problems with a finite

number of degrees of freedom can be recast as Hamilton's equations

-8H(g1,P1)

i9H(qj,pj)

for i n,

P' -

8qi 8Pi

for the generalised coordinates qi(t) and generalised momenta pi(t). In §8.2.2 we

have already remarked that these equations are Charpit's equations for the follow-

ing differential equation for u:

H(q,p)=O,

q={qj), P={Pi}_{8

};

(8.41)

we simply have to replace x in (8.21) by q to conform with popular convention

and to suppress the dependence of p and q on time t. Hence we have a route from

Newton's laws via Charpit's equation to (8.41), which is called the Hamilton-

Jacobi equation. However, both Newton's laws and the Hamilton-Jacobi equation

are equivalent to the principle of least action, namely that the action

u = JL(q, il) dt (8.42)

is minimised over all possible q when the integration is taken along a solution of

Newton's equations (i.e. along a ray of Charpit's equations), assuming q(O) and

q(T) are prescribed. Here L is called the Lagrangian and the equivalence of (8.42)

and Newton's laws is given in [46]. It is interesting to remark that the equivalence

of (8.42) to the Hamilton-Jacobi equation (8.41) is analogous to the equivalence

between Fermat's principlelse and the eikonal equation (8.1) (see Exercise 8.17).

We now ask ourselves `what partial differential equations may give rise to the

Hamilton-Jacobi equation in the WKB limit, in the way that Helmholtz' equation

gave rise to (8.1)?' This question clearly has no unique answer, since the WKB

approximation does not usually `balance' the highest derivatives and all the lower-

derivative terms in any given linear differential equation, but one possibility is the

Schrodinger equation

(qi, -ih

8 )r4(gi)

= E9y(q ).

(8.43)

8qi

Here t' is called the wave function and E is a constant, the energy level of the

system; h is also a constant which is very small when we work on scales much

166This states that the travel time (which is equivalent to arc length in a homogeneous medium)

adopted by a light ray is a minimum compared to other geometrically possible paths.

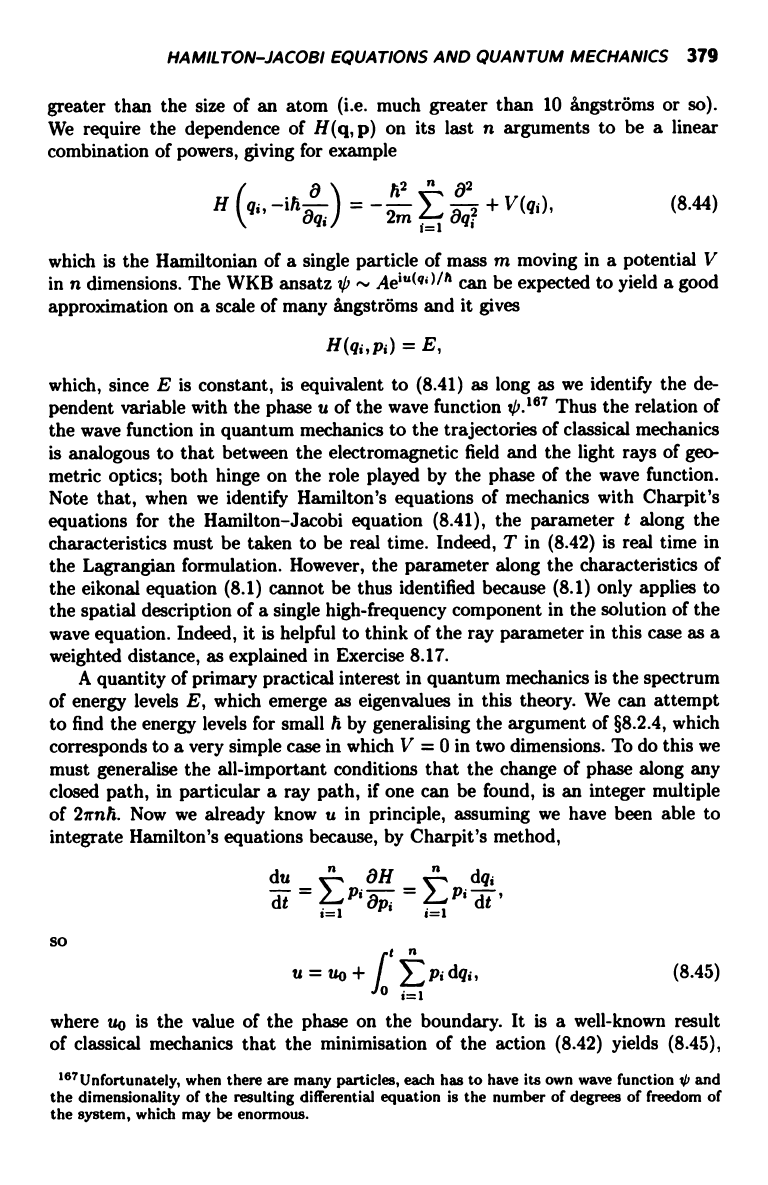
HAMILTON-JACOBI EQUATIONS AND QUANTUM MECHANICS 379

greater than the size of an atom (i.e. much greater than 10 angstroms or so).

We require the dependence of H(q, p) on its last n arguments to be a linear

combination of powers, giving for example

e) =

ntt az

H (qi, -'h

aq;

- 2m

aq2 +

V (qi),

(8.44)

which is the Hamiltonian of a single particle of mass m moving in a potential V

in n dimensions. The WKB ansatz

' - Ae1°(q,)/h can be expected to yield a good

approximation on a scale of many angstroms and it gives

H(g1,p1) = E,

which, since E is constant, is equivalent to (8.41) as long as we identify the de-

pendent variable with the phase u of the wave function t'.187 Thus the relation of

the wave function in quantum mechanics to the trajectories of classical mechanics

is analogous to that between the electromagnetic field and the light rays of geo-

metric optics; both hinge on the role played by the phase of the wave function.

Note that, when we identify Hamilton's equations of mechanics with Charpit's

equations for the Hamilton-Jacobi equation (8.41), the parameter t along the

characteristics must be taken to be real time. Indeed, T in (8.42) is real time in

the Lagrangian formulation. However, the parameter along the characteristics of

the eikonal equation (8.1) cannot be thus identified because (8.1) only applies to

the spatial description of a single high-frequency component in the solution of the

wave equation. Indeed, it is helpful to think of the ray parameter in this case as a

weighted distance, as explained in Exercise 8.17.

A quantity of primary practical interest in quantum mechanics is the spectrum

of energy levels E, which emerge as eigenvalues in this theory. We can attempt

to find the energy levels for small h by generalising the argument of §8.2.4, which

corresponds to a very simple case in which V = 0 in two dimensions. To do this we

must generalise the all-important conditions that the change of phase along any

closed path, in particular a ray path, if one can be found, is an integer multiple

of 21rnh. Now we already know u in principle, assuming we have been able to

integrate Hamilton's equations because, by Charpit's method,

n dqi

dt = p'8

p, _

p` at

u=u+

p;dg,

(8.45)

i=1

where uo is the value of the phase on the boundary. It is a well-known result

of classical mechanics that the minimisation of the action (8.42) yields (8.45),

167 Unfortunately, when there are many particles, each has to have its own wave function t and

the dimensionality of the resulting differential equation is the number of degrees of freedom of

the system, which may be enormous.

360

NON-QUASILINEAR EQUATIONS

and hence we can also identify the vitally important phase function u with the

minimising action. Moreover, in principle we only have to ensure that

pi dqi,

i-1

which is the change of phase along any closed path, is 2nirh. As in §8.2.4, even

when we are fortunate enough to be able to take this integral along a closed ray

path, care must be taken when evaluating the contributions from regions where

the path touches an envelope. Unfortunately, this may be a laborious task, except

in very simple geometries, because there will be many more `families' ui than the

two we encountered in geometric optics.

* 8.4

Higher-order equations

A remarkable number of phenomena have been illuminated by using non-quasi-

linear equations directly, and many of them would have been obscured had we

prosaically differentiated the equations into quasilinear systems. Indeed, many

aspects of the topics that we have mentioned richly deserve to be taken further, but

most of them would require a considerable background knowledge of asymptotics.

Another reason for not proceeding further with non-quasilinear equations is

that none of the results mentioned so far can be generalised to equations other

than scalar first-order ones. Such generalised equations are relatively rare, but one

interesting example is the Monge-Ampere equation of differential geometry, defined

below. In its simplest form it concerns developable surfaces, i.e. ones that are

envelopes of a one-parameter family of planes. Such surfaces are of great practical

importance, say, in making curved sheets of glass or metal by bending plane sheets,

to which they are isometric. They are special cases of ruled surfaces, as mentioned

after (8.25). A simple paper bending experiment shows that a developable surface

is necessarily the envelope of a one-parameter family of planes, say

p(A)x + q(A)y = u + A

(8.46)

as A varies. Hence it is the eliminant of A between (8.46) and

p'(A)x + q'(A)y = 1.

Now (8.46) is a Legendre or `contact' transformation, as in §4.8, and

OA _

= P +

(zp,

(A) + yq(A) - 1)

8x =

and similarly Ou/8y = q. Thus, since p and q are both functions of one variable A,

(

)

(8.47)

for some function f, and we have an equation that can be solved by (8.25). It can

also be observed that (8.47) is the general first integral of the simplest form of the

Monge-Ampere equation

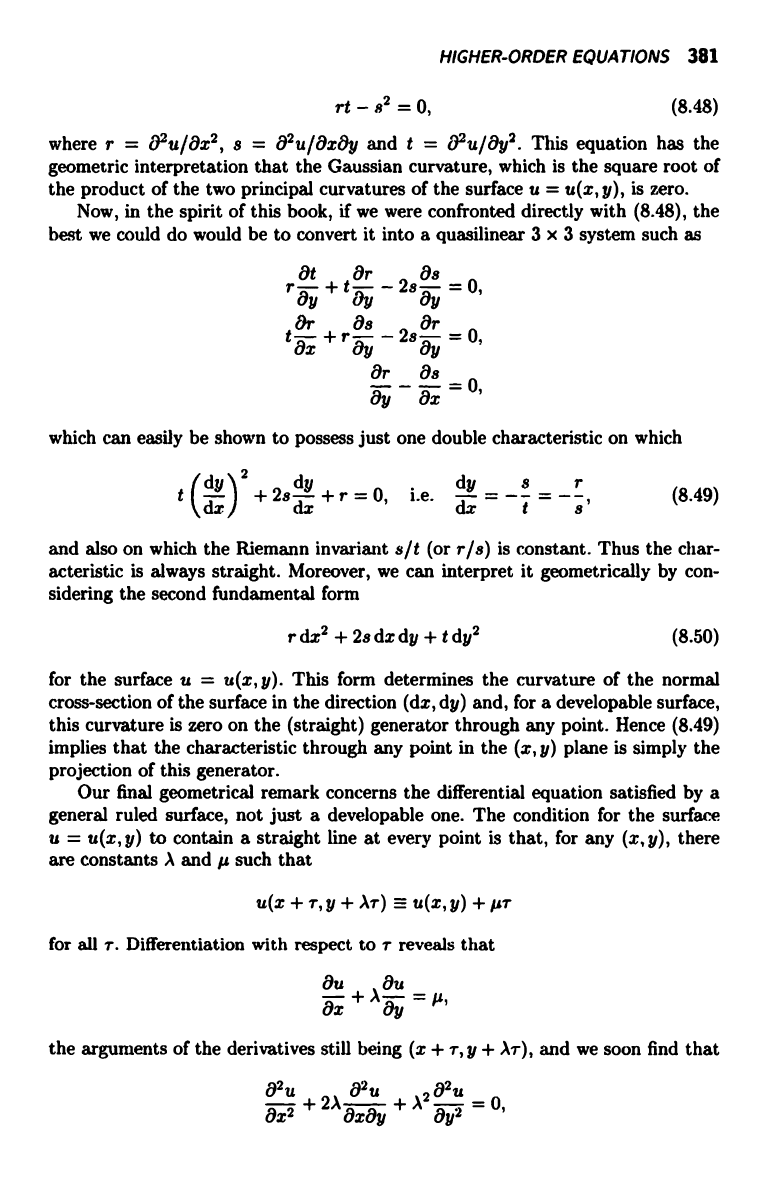
HIGHER-ORDER EQUATIONS 381

rt -

= 0,

(8.48)

where r = 82u/8x2, s = 02u/OxOy and t = 82u/8y2. This equation has the

geometric interpretation that the Gaussian curvature, which is the square root of

the product of the two principal curvatures of the surface u = u(x, y), is zero.

Now, in the spirit of this book, if we were confronted directly with (8.48), the

best we could do would be to convert it into a quasilinear 3 x 3 system such as

ray

tOy -

2sOy

= 0,

tOx

rO -

2sOy

= 0,

which can easily be shown to possess just one double characteristic on which

/ \z

t I I

+ 2s

+ r = 0,

i.e.

-t = -3, (8.49)

and also on which the Riemann invariant s/t (or r/s) is constant. Thus the char-

acteristic is always straight. Moreover, we can interpret it geometrically by con-

sidering the second fundamental form

r dx2 + 2s dx dy + t dy2 (8.50)

for the surface u = u(x, y). This form determines the curvature of the normal

cross-section of the surface in the direction (dx, dy) and, for a developable surface,

this curvature is zero on the (straight) generator through any point. Hence (8.49)

implies that the characteristic through any point in the (x, y) plane is simply the

projection of this generator.

Our final geometrical remark concerns the differential equation satisfied by a

general ruled surface, not just a developable one. The condition for the surface

u = u(x,y) to contain a straight line at every point is that, for any (x, y), there

are constants A and µ such that

u(x+r,y+Ar) a u(x,y)+ pr

for all r. Differentiation with respect to r reveals that

On On

8x +

A8y =

the arguments of the derivatives still being (x + r, y + Ar), and we soon find that

092

+2A8 a +a28 u =o

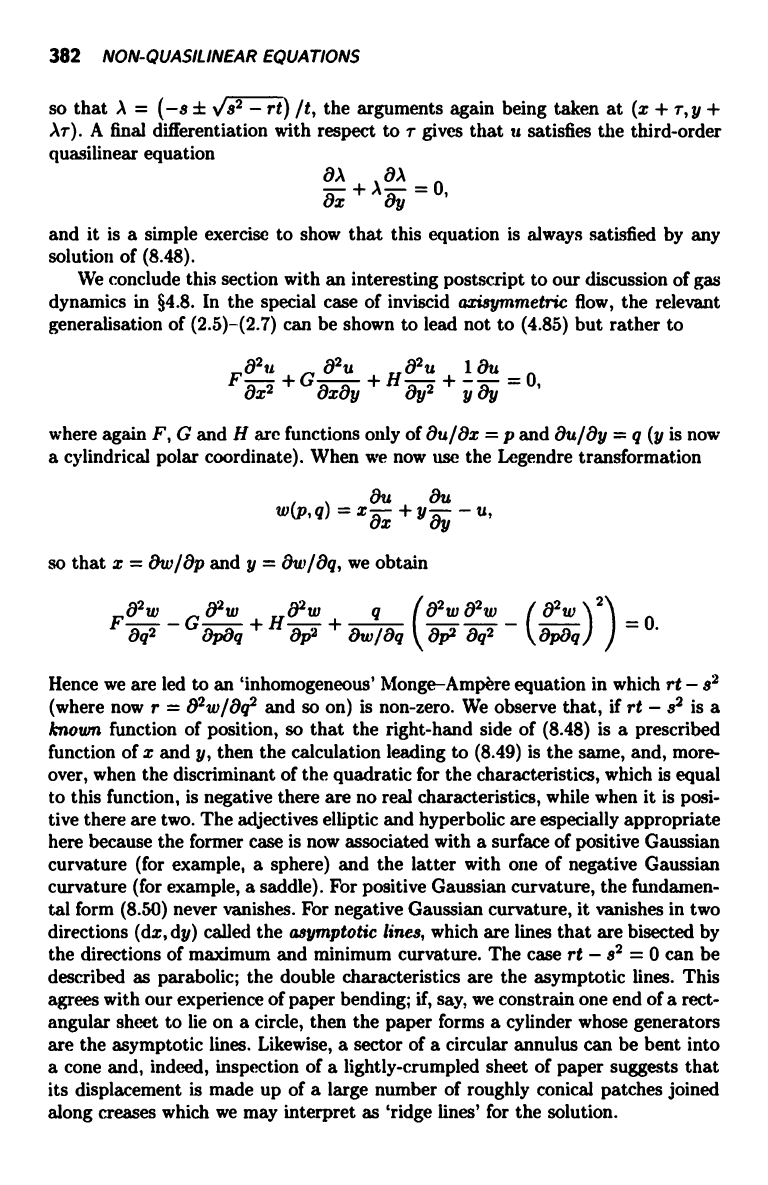
382

NON-QUASILINEAR EQUATIONS

so that A = (-s ±

s2 - rt It, the arguments again being taken at (x + r, y +

Ar). A final differentiation with respect to r gives that u satisfies the third-order

quasilinear equation

+ A ay = 0,

and it is a simple exercise to show that this equation is always satisfied by any

solution of (8.48).

We conclude this section with an interesting postscript to our discussion of gas

dynamics in §4.8. In the special case of inviscid axisymmetric flow, the relevant

generalisation of (2.5)-(2.7) can be shown to lead not to (4.85) but rather to

02U

2 2

F8x

2 +G8x8 + H-

18u

- 0,

0y2

y ay

where again F, G and H are functions only of 8u/8x = p and 8u/8y = q (y is now

a cylindrical polar coordinate). When we now use the Legendre transformation

w(p,q)=xF +yby-u,

so that x = 8w/8p and y = 8w/8q, we obtain

F82w-G02w

+H82w+

92W-92-W

(82w)2 0.

8q2 8p8q 8p2 8w/Oq

8P2 8q2 - \ 8p8q /

Hence we are led to an `inhomogeneous' Monge-Ampere equation in which rt - 82

(where now r = 82w/8g2 and so on) is non-zero. We observe that, if rt - s2 is a

known function of position, so that the right-hand side of (8.48) is a prescribed

function of x and y, then the calculation leading to (8.49) is the same, and, more-

over, when the discriminant of the quadratic for the characteristics, which is equal

to this function, is negative there are no real characteristics, while when it is posi-

tive there are two. The adjectives elliptic and hyperbolic are especially appropriate

here because the former case is now associated with a surface of positive Gaussian

curvature (for example, a sphere) and the latter with one of negative Gaussian

curvature (for example, a saddle). For positive Gaussian curvature, the fundamen-

tal form (8.50) never vanishes. For negative Gaussian curvature, it vanishes in two

directions (dx, dy) called the asymptotic lines, which are lines that are bisected by

the directions of maximum and minimum curvature. The case rt - s2 = 0 can be

described as parabolic; the double characteristics are the asymptotic lines. This

agrees with our experience of paper bending; if, say, we constrain one end of a rect-

angular sheet to lie on a circle, then the paper forms a cylinder whose generators

are the asymptotic lines. Likewise, a sector of a circular annulus can be bent into

a cone and, indeed, inspection of a lightly-crumpled sheet of paper suggests that

its displacement is made up of a large number of roughly conical patches joined

along creases which we may interpret as `ridge lines' for the solution.

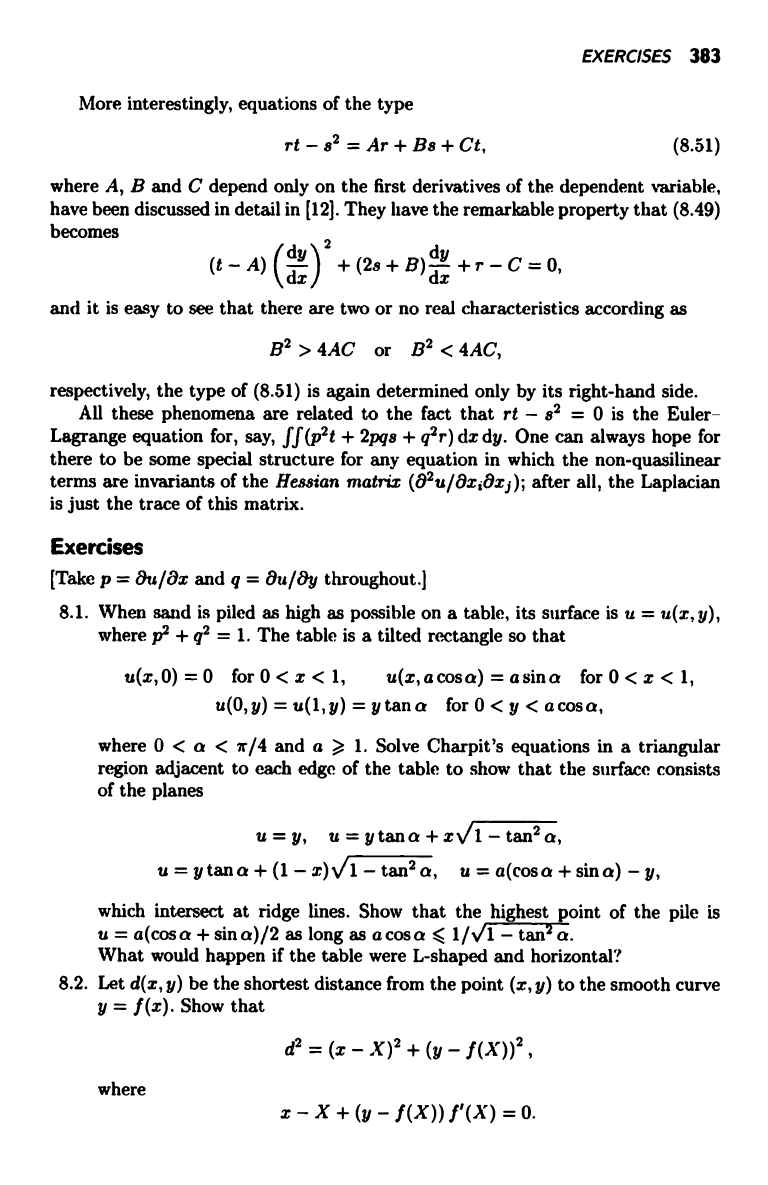
EXERCISES 383

More interestingly, equations of the type

rt - s2 = Ar + Bs + Ct, (8.51)

where A, B and C depend only on the first derivatives of the dependent variable,

have been discussed in detail in (12]. They have the remarkable property that (8.49)

becomes

(t - A) l

z I

+(2s+B)d +r-C=O,

and it is easy to see that there are two or no real characteristics according as

B2 > 4AC or B2 < 4AC,

respectively, the type of (8.51) is again determined only by its right-hand side.

All these phenomena are related to the fact that rt - s2 = 0 is the Euler-

Lagrange equation for, say, f f (pet + 2pqs + qtr) dx dy. One can always hope for

there to be some special structure for any equation in which the non-quasilinear

terms are invariants of the Hessian matrix (82u/(9x;8xj ); after all, the Laplacian

is just the trace of this matrix.

Exercises

(Take p = 8u/8x and q = 8u/8y throughout.]

8.1. When sand is piled as high as possible on a table, its surface is u = u(x, y),

where p2 + q2 = 1. The table is a tilted rectangle so that

u(x, 0) = 0

for 0 < x < 1,

u(x, a cos a) = a sin a

for 0 < x < 1,

u(0,y)=u(1,y)=ytana for0<y<acosa,

where 0 < a < a/4 and a > 1. Solve Charpit's equations in a triangular

region adjacent to each edge of the table to show that the surface consists

of the planes

u=y, u=ytana+x 1-tan2a,

u=ytana+(1-x) 1-tan2a,

u=a(cosa+sina)-y,

which intersect at ridge lines. Show that the highest point of the pile is

u = a(cos a + sin a)/2 as long as a cos a <, 11,V1 - tan a.

What would happen if the table were L-shaped and horizontal?

8.2. Let d(x, y) be the shortest distance from the point (x, y) to the smooth curve

y = f (x). Show that

d2 = (x-X)2+(y-f(X))2,

where

x - X +(y - f(X)) f'(X) =0.

384

NON-QUASILINEAR EQUATIONS

Deduce that these formulae provide the general solution of

( 8d)2+ \8U/2

You can see this without differentiation by taking the origin at (x, y) with

the x axis along the normal from (x, y) to the curve, showing that 8d/8y = 0,

and noting that IOdI2 is invariant under rotation of the axes.

8.3. Suppose that u satisfies Clairaut's equation

u = xp + yq - f(p,q)

Show that, if u = u0(a) on x = xo(s), y = yo(s), then

x = xoet +

(po, qo)

(et -1),

y = yoet +

88f

(po,go)(et - 1),

u = uoet -

(po Lf (po, qo)

+ qo 8q

(po, qo)

- f(po,

qo)) (et

- 1),

where po(s) and qo(s) satisfy

t4 = pox' + goyo,

uo = xopo + yogo - f(po,go)

Noting that, when p and q are constant, Clairaut's equation is that of a

plane in (u, x, y) space, deduce that u = ax + fly - f (a, 0) is a solution for

any constants a and 9. Hence show that the general solution is the result of

eliminating a between

u = ax + F(a)y - f (a, F(a)),

x + d

(u_ 8q

(a,

F(a)))

(a, F(a)) = 0,

where F is arbitrary.

Remark. This is the only first-order equation whose general solution can be

written down without any integrations, by virtue of the Legendre transfor-

mation (4.86).

8.4. Suppose that pq = 1 with u = 0 on x + y = 1. Use Charpit's method to

show that u = x + y - l or u = -(x + y - 1). Show also that, if u = uo(s)

on x = xo(s), y = yo(s), then a solution only exists if u' > 4xoy .

By finding the solution for which all the characteristics pass through x =

y = 0, show that the integral conoid through the origin is u2 = 4xy.

EXERCISES 385

8.5. Suppose that u = f (x, y, p, q). Show that p and q satisfy the 2 x 2 system

Of 8P

Of 89

8p 8x

8q 8x

8x'

Of 8p

Of 8g

8pOy8gayq-

Deduce that the characteristics are given by

Of/Of

twice,

dx 8q

and use the Fredholm Alternative to show that, along a characteristic.

dx _ dy

_ dp

_ dq

8f l8P

Of /Oq

p - 9f /8x

q - Of /8y

Deduce from the differential equation that these ratios are also equal to

/(8f

pap +g8q

* 8.6. Suppose that

with

82u

for 0 < x < 1,

8x2

u(x,0) = 0,

u(0,r) = 1,

u(1,r) = 100.

Use a WKB ansatz it - a(x, r)e_t (z)/r, or the similarity solution (6.45), to

indicate that, despite the different wall temperatures, the location at r = 0+

of the minimum value of it is at x = 1/2.

8.7. Check that u = -x satisfies the equation of geometric optics and that it

represents a plane wave of light incident from x = +oo. When this light is

incident on a parabolic reflector y2 = 4x, the reflected field has to satisfy

u = -x on the parabola. Denote the values of p and q on the parabola by

po = coss and qo = sins, and show that u = uo = -xo(s), x = xo(s),

y = yo(s) satisfy

-xo = Poxo + goyo,

yoyo = 2xo1

Deduce that yo = -2tan(s/2) and xo = tan2(s/2), and hence that the

reflected rays are

y=(x-1) tans,

so that they all pass through the focus (0, 1).

386

NON-QUASILINEAR EQUATIONS

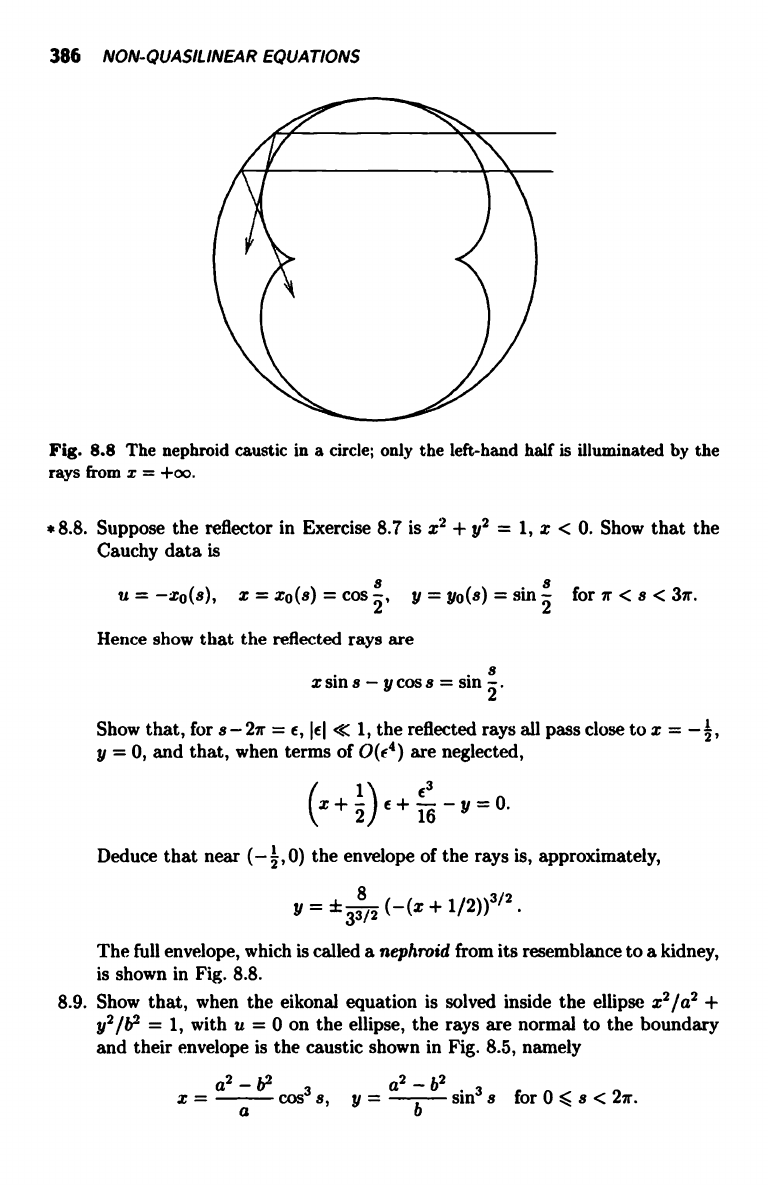
Fig. 8.8 The nephroid caustic in a circle; only the left-hand half is illuminated by the

rays from x = +oo.

8.8. Suppose the reflector in Exercise 8.7 is x2 + y2 = 1, x < 0. Show that the

Cauchy data is

u= -xo(s),

x = xo(s) = cos

y = yo(s) =sin

Hence show that the reflected rays are

x sin s- y cos s= sin

for it < s < 31r.

Show that, for s - 2a = E, AEI K 1, the reflected rays all pass close to x = - 121

y = 0, and that, when terms of 0(E4) are neglected,

1).E

x++ -y=0.

Deduce that near (- s , 0) the envelope of the rays is, approximately,

Y =

382 (-(x + 1/2))3/2 .

The full envelope, which is called a nephroid from its resemblance to a kidney,

is shown in Fig. 8.8.

8.9. Show that, when the eikonal equation is solved inside the ellipse x2/a2 +

y2/b2 = 1, with u = 0 on the ellipse, the rays are normal to the boundary

and their envelope is the caustic shown in Fig. 8.5, namely

a2-b2

a c o s 3 s, y=

sin3 s for 0 5 s< 27r.

EXERCISES 387

8.10. Suppose that the temperature T(x) satisfies the convection-diffusion equa-

tion

(v °)T = e°2 T,

where v(x) is a prescribed velocity field. Show that, if we seek a WKB solu-

tion in which T - Ae"(x)/,, then

(V. °)u

= IVuI2.

Show also that Charpit's equations are

dx_v-2

dt dt

where p = Vu. and deduce that to lowest order the isotherms T = constant

bisect the angle between the characteristics and the streamlines, which are

parallel to v.

8.11. (i) Let t be a variable along a ray for the eikonal equation p2 + q2 = 1,

scaled so that dx/dt = p, etc., and so

x = pot + xO,

y = qot + yo.

Define

Show that

where, as on p.371,

Show from (8.30) that

T(s) =

9ox0 - lto?lo

gopo

- pogo

2 OA

= -°2U.

_q _N

Invert J and use the relation

8p 8q

v2uax+a

Ss ,

=po8x+go8y

to show that V2u = J-18J/8t. Deduce that

e(x,U)

xo+tpo Ilo+tq'o

8(s, I)

IJI = (gopo - pogo) (t + T(s)),

(A2J) = 0,

°2u

T + t'

zit-

where T is defined after (8.29). Finally deduce (8.31).