Nakahara M. Geometry, Topology and Physics
Подождите немного. Документ загружается.

8.7.1 Definitions
There are some differentiable manifolds which carry a similar structure to
complex manifolds. To study these manifolds, we somewhat relax the condition
(8.16) and require a weaker condition here.
Definition 8.5. Let M be a differentiable manifold. The pair (M, J ),orsimply
M, is called an almost complex manifold if there exists a tensor field J of type
(1, 1) such that at each point p of M, J
2
p
=−id
T
p
M
. The tensor field J is also
called the almost complex structure.
Since J
2
p
=−id
T
p
M
, J
p
has eigenvalues ±i. If there are m + i, then there
must be an equal number of −i, hence J
p
is a 2m × 2m matrix and J
2
p
=−I
2m
.
Thus, M is an even-dimensional manifold. Note that not all even-dimensional
manifolds are almost complex manifolds. For example, S
4
is not an almost
complex manifold (Steenrod 1951). Note also that we now require a weaker
condition J
2
p
=−I
2m
. Of course, the tensor J
p
defined by (8.16) satisfies
J
2
p
=−I
2m
, hence a complex manifold is an almost complex manifold. There
are almost complex manifolds which are not complex manifolds. For example, it
is known that S
6
admits an almost complex structure, although it is not a complex
manifold (Fr¨ohlicher 1955).
Let us complexify a tangent space of an almost complex manifold (M, J ).
Given a linear transformation J
p
at T
p
M such that J
2
p
=−I
2m
,weextendJ
p
to a
-linear map defined on T
p
M . J
p
defined on T
p
M also satisfies J
2
p
=−I
2m
,
J
2
p
(X + iY ) = J
2
p
X + i J
2
p
Y =−X + i(−Y ) =−(X + iY )
where X, Y ∈ T
p
M. Let us divide T
p
M into two disjoint vector subspaces,
according to the eigenvalue of J
p
,
T
p
M = T
p
M
+
⊕ T
p
M
−
(8.121)
where
T
p
M
±
={Z ∈ T
p
M |J
p
Z =±iZ}. (8.122)
Any vector V ∈ T
p
M is written as V = W
1
+ W
2
,whereW
1
, W
2
∈ T
p
M
+
.
Note that J
p
V = iW
1
−iW
2
. At this stage the reader might have noticed that we
can follow the classification scheme of vectors and vector fields developed for the
complex manifolds in section 8.2. In fact, the only difference is that on a complex
manifold the almost complex structure is explicitly given by (8.18), while on
an almost complex manifold, it is required to satisfy the less strict condition
J
2
p
=−I
2m
. To classify the complexified tangent spaces and complexified vector
spaces, we only need the latter condition. Accordingly, we separate T
p
M into
T
p
M
±
and (M) into (M)
±
, although there does not necessarily exist a basis
of T
p
M
+
of the form {∂/∂z
µ
}. For example, we may still define the projection
operators
±
≡
1
2
(id
T
p
M
∓ i J
p
) : T
p
M → T
p
M
±
. (8.123)
We call a vector in T
p
M
+
(T
p
M
−
) a holomorphic (anti-holomorphic) vector and
a vector field in
(M)
+
( (M)
−
) a holomorphic (anti-holomorphic) vector field.
Definition 8.6. Let (M, J ) be an almost complex manifold. lf the Lie bracket of
any holomorphic vector fields X, Y ∈
+
(M) is again a holomorphic vector field,
[X, Y ]∈
+
(M), the almost complex structure J is said to be integrable.
Let (M, J ) be an almost complex manifold. Define the Nijenhuis tensor
field N :
(M) × (M) → (M) by
N(X, Y ) ≡[X, Y ]+J[JX, Y ]+J[X, JY]−[JX, JY]. (8.124)
Given a basis {e
µ
= ∂/∂x
µ
} and the dual basis {dx
µ
}, the almost complex
structure is expressed as J = J
µ
ν
dx
µ
⊗ ∂/∂x
ν
. The component expression
of N is
N(X, Y ) = (X
ν
∂
ν
Y
µ
− Y
ν
∂
ν
X
µ
)e
µ
+ J
λ
µ
{J
κ
ν
X
κ
∂
ν
Y
λ
− Y
ν
∂
ν
(J
κ
λ
X
κ
)}e
µ
+ J
λ
µ
{X
ν
∂
ν
(J
κ
λ
Y
κ
) − J
κ
ν
Y
κ
∂
ν
X
λ
}e
µ
−{J
κ
ν
X
κ
∂
ν
(J
λ
µ
Y
λ
) − J
κ
ν
Y
κ
∂
ν
(J
λ
µ
X
λ
)}e
µ
= X
κ
Y
ν
[−J
λ
µ
(∂
ν
J
κ
λ
) + J
λ
µ
(∂
κ
J
ν
λ
)
− J
κ
λ
(∂
λ
J
ν
µ
) + J
ν
λ
(∂
λ
J
κ
µ
)]e
µ
. (8.125)
Thus, N is indeed linear in X and Y and hence a tensor. If J is a complex
structure, J is given by (8.18) and the Nijenhuis tensor field trivially vanishes.
Theorem 8.12. An almost complex structure J on a manifold M is integrable if
and only if N(A, B) = 0foranyA, B ∈
(M).
Proof.LetZ = X +iY , W = U +iV ∈
(M) . We extend the Nijenhuis tensor
field so that its action on vector fields in
(M) is given by
N(Z , W ) =[Z, W ]+J [JZ, W ]+J [Z , JW]−[JZ, JW]
={N (X, U ) − N(Y, V )}+i{N(X, V ) + N(Y, U )}. (8.126)
Suppose that N(A, B) = 0foranyA, B ∈
(M). From (8.126), it turns
out that N(Z , W ) = 0forZ , W ∈
(M).LetZ , W ∈
+
(M) ⊂ (M) .
Since JZ = iZ and JW = iW ,wehaveN(Z , W ) = 2{[Z , W ]+iJ [Z , W ]}.By
assumption, N(Z, W ) = 0andwefind[Z , W ]=−iJ [Z, W ] or J [Z , W ]=
i[Z , W ],thatis,[Z, W ]∈
+
(M). Thus, the almost complex structure is
integrable.
Conversely, suppose that J is integrable. Since
(M) is a direct sum of
+
(M) and
−
(M), we can separate Z, W ∈ (M) as Z = Z
+
+ Z
−
and
W = W
+
+ W
−
.Then
N(Z , W ) = N(Z
+
, W
+
) + N(Z
+
, W
−
) + N(Z
−
, W
+
) + N(Z
−
, W
−
).
Since JZ
±
=±iZ
±
and JW
±
=±iW
±
, it is easy to see that N(Z
+
, W
−
) =
N(Z
−
, W
+
) = 0. We also have
N(Z
+
, W
+
) =[Z
+
, W
+
]+J [iZ
+
, W
+
]+J [Z
+
, iW
+
]−[iZ
+
, iW
+
]
= 2[Z
+
, W
+
]−2[Z
+
, W
+
]=0
since J [Z
+
, W
+
]=i[Z
+
, W
+
]. Similarly, N(Z
−
, W
−
) vanishes and we have
shown that N(Z, W ) = 0foranyZ , W ∈
(M). In particular, it should vanish
for Z , W ∈
(M).
If M is a complex manifold, the complex structure J is a constant tensor
field and the Nijenhuis tensor field vanishes. What about the converse? We now
state an important (and difficult to prove) theorem.
Theorem 8.13. (Newlander and Nirenberg 1957) Let (M, J ) be a 2m-dimensional
almost complex manifold. If J is integrable, the manifold M is a complex
manifold with the almost complex structure J .
In summary we have:
Integrable almost
complex structure
=
Vanishing Nijenhuis
tensor field
= Complex manifold.
8.8 Orbifolds
Let M be a manifold and let G be a discrete group which acts on M. Then the
quotient space ≡ M/G is called an orbifold. As we will see later there are
fixed points in M, which do not transform under the action of G. These points
are singular and the orbifold is not a manifold in general. Thus, even though we
start with a simple manifold M, the orbifold M/G may have quite a complicated
topology.
8.8.1 One-dimensional examples
To obtain a concrete idea, let us consider a simple example. Take M =
2
which
is to be identified with the complex plane
.LetustakeG =
3
and identify
the points z,e
2πi/3
z and e
4πi/3
z. The orbifold M/G consists of a third of the
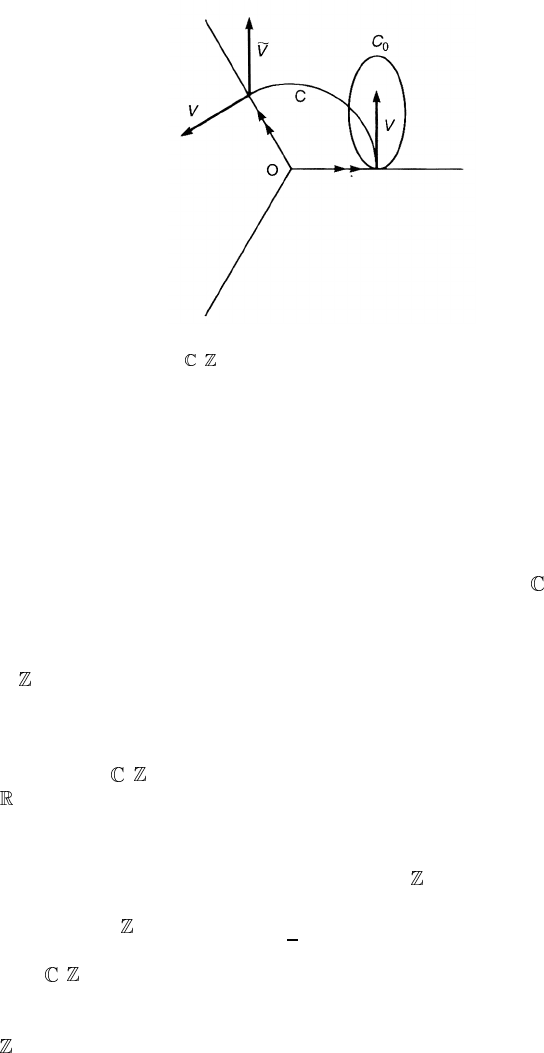
Figure 8.6. The orbifold /
3
is a third of the complex plane. The edges of the orbifold
are identified as shown in the figure. V becomes a vector
˜
V after parallel transportation
along C. The angle between V and
˜
V is 2π/3.
complex plane and after the identification of the edges we end up with a cone,
see figure 8.6. It is interesting to see what the holonomy group of this orbifold
is. We use the flat connection induced by the Euclidean metric of
. Then, after
the parallel transport of a vector V along the loop C (this is indeed a loop!), we
obtain a vector
˜
V which is different from V after the identification. Observe that
the angle between V and
˜
V is 2π/3. It is easy to verify that the holomony group
is
3
. Since the holonomy is trivial for the loop C
0
which does not encircle the
origin, we find that the curvature is singular at the origin (recall that the curvature
measures the non-triviality of the holonomy, see section 7.3). In general the fixed
points (the origin in the present case) are singular points of the curvature. Note,
however, that
/
3
is a manifold since it has an open covering homeomorphic to
2
.
A less trivial example is obtained by taking the torus as the manifold. We
identify the points z and z + m + ne
iπ/3
(m, n ∈ ) in the complex plane; see
figure 8.7(a). If we identify the edges of the parallelogram OPQR, we have the
torus T
2
.Let
3
act on T
2
as α : z → e
2πi/3
z. We find that there are three
inequivalent fixed points z = (n/
√
3)e
πi/6
where n = 0, 1 and 2. This orbifold
=
/
3
consists of two triangles surrounding a hollow; see figure 8.7(b).If
the flat connection induced by the flat metric of the torus is employed to define the
parallel transport of vectors, we find that the holonomy around each fixed point is
3
.
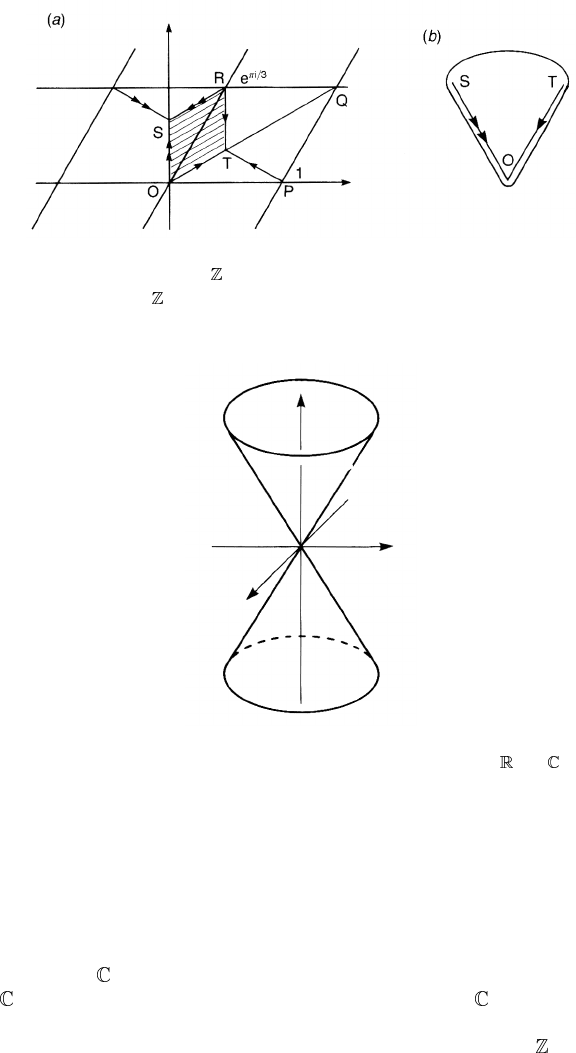
Figure 8.7. Under the action of
3
, points of the torus T
2
are identified. The shaded area
is the orbifold = T
2
/
3
. If the edges of the orbifold are identified, we end up with the
object in figure 8.7(b), which is homeomorphic to the sphere S
2
.
Figure 8.8. The conical singularity. The origin does not look like
n
or
n
.
8.8.2 Three-dimensional examples
Orbifolds with three complex dimensions have been proposed as candidates for
superstring compactification. The detailed treatment of this subject is outside the
scope of this book and the reader should consult Dixson et al (1985, 1986) and
Green et al (1987).
Let T =
3
/L be a three-dimensional complex torus, where L is a lattice
in
3
. For definiteness, let (z
1
, z
2
, z
3
) be the coordinates of
3
and identify z
i
and z
i
+ m + ne
πi/3
. Under this identification, T is identified with a product of
three tori, T = T
1
× T
2
× T
3
. T admits, as before, the action of
3
defined
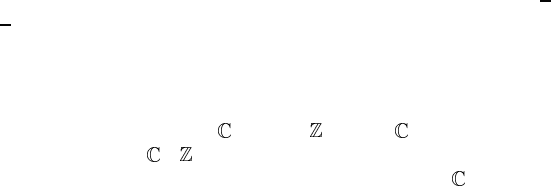
by α : z
i
→ e
2πi/3
z
i
. If each z
i
takes one of the values 0,(1/
√
3)e
iπ/6
,
(2/
√
3)e
πi/6
, the action of α leaves the point (z
i
) invariant. Thus, there are
3
3
= 27 fixed points in the orbifold. In the present case, the fixed point is a
conical singularity (figure 8.8) and the orbifold cannot be a manifold. [Remarks:
The appearance of the conical singularity can be understood more easily from a
simpler example. Let (x , y) ∈
2
and let
2
act on
2
as (x , y) →±(x, y).
Then the orbifold =
2
/
2
has a conical singularity at the origin. In fact, let
[(x, y)]→(x
2
, xy, y
2
) ≡ (X, Y, Z ) be an embedding of in
3
. Note that X, Y
and Z satisfy a relation Y
2
= XZ.IfX, Y and Z are thought of as real variables,
this is simply the equation of a cone.]

9
FIBRE BUNDLES
A manifold is a topological space which looks locally like
m
, but not necessarily
so globally. By introducing a chart, we give a local Euclidean structure to a
manifold, which enables us to use the conventional calculus of several variables.
A fibre bundle is, so to speak, a topological space which looks locally like a direct
product of two topological spaces. Many theories in physics, such as general
relativity and gauge theories, are described naturally in terms of fibre bundles.
Relevant references are Choquet-Bruhat et al (1982), Eguchi et al (1980) and
Nash and Sen (1983). A complete analysis is found in Kobayashi and Nomizu
(1963, 1969) and Steenrod (1951).
9.1 Tangent bundles
For clarification, we begin our exposition with a motivating example. A tangent
bundle TM over an m-dimensional manifold M is a collection of all the tangent
spaces of M:
TM ≡
+
p∈M
T
p
M. (9.1)
The manifold M over which TM is defined is called the base space.Let{U
i
} be
an open covering of M.Ifx
µ
= ϕ
i
( p) is the coordinate on U
i
, an element of
TU
i
≡
+
p∈U
i
T
p
M
is specified by a point p ∈ M and a vector V = V
µ
( p)(∂/∂x
µ
)|
p
∈ T
p
M.
Noting that U
i
is homeomorphic to an open subset ϕ(U
i
) of
m
and each
T
p
M is homeomorphic to
m
,wefindthatTU
i
is identified with a direct
product
m
×
m
(figure 9.1). If ( p, V ) ∈ TU
i
, the identification is given by
( p, V ) → (x
µ
( p), V
µ
( p)). TU
i
is a 2m-dimensional differentiable manifold.
What is more, TU
i
is decomposed into a direct product U
i
×
m
.Ifwepickup
a point u of TU
i
, we can systematically decompose the information u contains
into a point p ∈ M and a vector V ∈ T
p
M. Thus, we are naturally led to the
concept of projection π : TU
i
→ U
i
(figure 9.1). For any point u ∈ TU
i
, π(u)
is a point p ∈ U
i
at which the vector is defined. The information about the vector
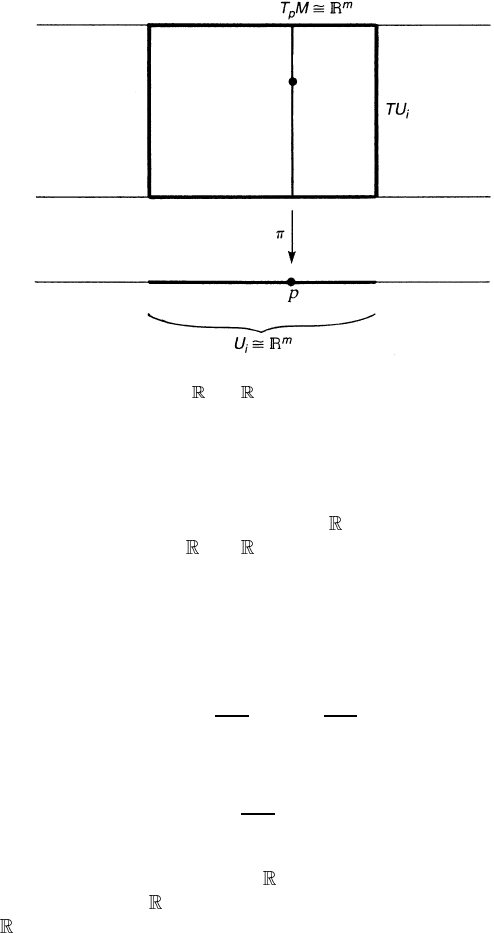
Figure 9.1. A local piece TU
i
m
×
m
of a tangent bundle TM. The projection π
projects a vector V ∈ T
p
M to p.
is completely lost under the projection. Observe that π
−1
( p) = T
p
M.Inthe
context of the theory of fibre bundles, T
p
M is called the fibre at p.
It is obvious by construction that if M =
m
, the tangent bundle itself is
expressed as a direct product
m
×
m
. However, this is not always the case
and the non-trivial structure of the tangent bundle measures the topological non-
triviality of M. To see this, we have to look not only at a single chart U
i
but also
at other charts. Let U
j
be a chart such that U
i
∩ U
j
=∅and let y
µ
= ψ(p) be
the coordinates on U
j
. Take a vector V ∈ T
p
M where p ∈ U
i
∩ U
j
. V has two
coordinate presentations,
V = V
µ
∂
∂x
µ
p
=
˜
V
µ
∂
∂y
µ
p
. (9.2)
It is easy to see that they are related as
˜
V
ν
=
∂y
ν
∂x
µ
( p)V
µ
. (9.3)
For {x
µ
} and {y
ν
} to be good coordinate systems, the matrix (G
ν
µ
) ≡ (∂y
ν
/∂x
µ
)
must be non-singular: (G
ν
µ
) ∈ GL(m, ). Thus, fibre coordinates are rotated
by an element of GL(m,
) whenever we change the coordinates. The group
GL(m,
) is called the structure group of TM. In this way fibres are
interwoven together to form a tangent bundle, which consequently may have quite
a complicated topological structure.
We note en passant that the projection π can be defined globally on M.It
is obvious that π(u) = p does not depend on a special coordinate chosen. Thus,
π : TM → M is defined globally with no reference to local charts.
Let X ∈ (M) be a vector field on M. X assigns a vector X|
p
∈ T
p
M
at each point p ∈ M. From our viewpoint, X is looked upon as a smooth map
M → TM. This map is not utterly arbitrary since a point p must be mapped to
a point u ∈ TM such that π(u) = p.Wedefineasection (or a cross section)
of TM as a smooth map s : M → TM such that π ◦ s = id
M
. If a section
s
i
: U
i
→ TU
i
is defined only on a chart U
i
, it is called a local section.
9.2 Fibre bundles
The tangent bundle in the previous section is an example of a more general
framework called a fibre bundle. Definitions are now in order.
9.2.1 Definitions
Definition 9.1. A (differentiable) fibre bundle (E,π,M, F, G) consists of the
following elements:
(i) A differentiable manifold E called the total space.
(ii) A differentiable manifold M called the base space.
(iii) A differentiable manifold F called the fibre (or typical fibre).
(iv) A surjection π : E → M called the projection. The inverse image
π
−1
( p) = F
p
∼
=
F is called the fibre at p.
(v) A Lie group G called the structure group, which acts on F on the left.
(vi) A set of open covering {U
i
} of M with a diffeomorphism φ
i
: U
i
× F →
π
−1
(U
i
) such that π ◦ φ
i
( p, f ) = p.Themapφ
i
is called the local
trivialization since φ
−1
i
maps π
−1
(U
i
) onto the direct product U
i
× F .
(vii) If we write φ
i
( p, f ) = φ
i, p
( f ),themapφ
i, p
: F → F
p
is a
diffeomorphism. On U
i
∩ U
j
=∅, we require that t
ij
( p) ≡ φ
−1
i, p
◦ φ
j, p
:
F → F be an element of G.Thenφ
i
and φ
j
are related by a smooth map
t
ij
: U
i
∩U
j
→ G as (figure 9.2)
φ
j
( p, f ) = φ
i
( p, t
ij
( p) f ). (9.4)
The maps t
ij
are called the transition functions.
[Remarks: We often use a shorthand notation E
π
−→ M or simply E to denote a
fibre bundle (E,π,M, F, G).
Strictly speaking, the definition of a fibre bundle should be independent of
the special covering {U
i
} of M. In the mathematical literature, this definition
is employed to define a coordinate bundle (E,π,M, F, G, {U
i
}, {φ
i
}).Two
coordinate bundles (E,π,M, F, G, {U
i
}, {φ
i
}) and (E,π,M, F, G, {V
i
}, {ψ
i
})
are said to be equivalent if (E,π,M, F, G, {U
i
}∪{V
j
}, {φ
i
}∪{ψ
j
}) is again a
coordinate bundle. A fibre bundle is defined as an equivalence class of coordinate
bundles. In practical applications in physics, however, we always employ a certain
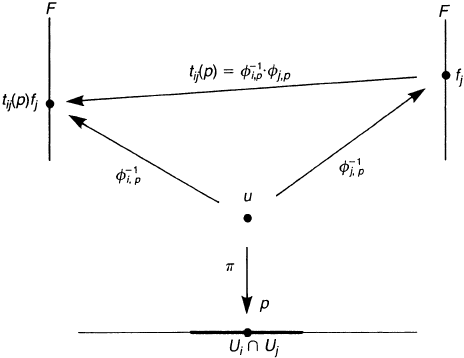
Figure 9.2. On the overlap U
i
∩U
j
, two elements f
i
, f
j
∈ F are assigned to u ∈ π
−1
( p),
p ∈ U
i
∩U
j
. They are related by t
ij
( p) as f
i
= t
ij
( p) f
j
.
definite covering and make no distinction between a coordinate bundle and a fibre
bundle.]
We need to clarify several points. Let us take a chart U
i
of the base space M.
π
−1
(U
i
) is a direct product diffeomorphic to U
i
× F, φ
−1
i
: π
−1
(U
i
) → U
i
× F
being the diffeomorphism. If U
i
∩ U
j
=∅,wehavetwomapsφ
i
and φ
j
on
U
i
∩ U
j
. Let us take a point u such that π(u) = p ∈ U
i
∩ U
j
. We then assign
two elements of F, one by φ
−1
i
and the other by φ
−1
j
,
φ
−1
i
(u) = ( p, f
i
), φ
−1
j
(u) = ( p, f
j
) (9.5)
seefigure9.2.Thereexistsamapt
ij
: U
i
∩ U
j
→ G which relates f
i
and f
j
as
f
i
= t
ij
( p) f
j
. This is also written as (9.4).
We require that the transition functions satisfy the following consistency
conditions:
t
ii
( p) = identity map ( p ∈ U
i
) (9.6a)
t
ij
( p) = t
ji
( p)
−1
( p ∈ U
i
∩U
j
) (9.6b)
t
ij
( p) ·t
jk
( p) = t
ik
( p)(p ∈ U
i
∩U
j
∩U
k
). (9.6c)
Unless these conditions are satisfied, local pieces of a fibre bundle cannot be glued
together consistently. If all the transition functions can be taken to be identity
maps, the fibre bundle is called a trivial bundle. A trivial bundle is a direct
product M × F.
