Nakahara M. Geometry, Topology and Physics

Подождите немного. Документ загружается.

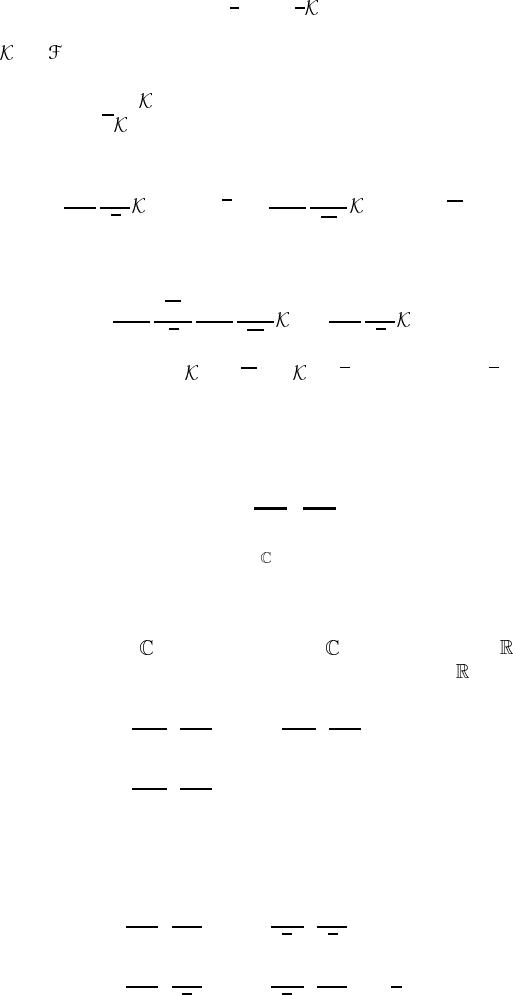
Suppose that a Hermitian metric g is given on a chart U

= ∂

∂

(8.83)

where

∈ (U

). Clearly this metric satisﬁes the condition (8.82), hence it is

K¨ahler. Conversely, it can be shown that any K¨ahler metric is locally expressed

as (8.83). The function

is called the K

ahler potential of a K¨ahler metric. It

follows that  = i∂

∂

on U

Let (U

,ϕ

) and (U

,ϕ

) be overlapping charts. On U

∩U

,wehave

∂

∂z

∂

∂z

⊗ dz

∂

∂w

∂

∂w

⊗ dw

where z = ϕ

( p) and w = ϕ

( p). It then follows that

∂w

∂z

∂w

∂z

∂

∂w

∂

∂w

∂

∂z

∂

∂z

. (8.84)

This is satisﬁed if and only if

(w, w) =

(z, z) + φ

(z) + ψ

(z) where φ

(ψ

) is holomorphic (anti-holomorphic) in z.

Exercise 8.8. Let M be a compact K¨ahler manifold without a boundary. Show

that



≡  ∧...∧ 

-. /

is closed but not exact where m = dim M [Hint: Use Stokes’ theorem.] Thus,

the 2mth Betti number cannot vanish, b

≥ 1. We will see later that b

2 p

≥ 1for

1 ≤ p ≤ m.

Example 8.6. Let M =

={(z

,...,z

)}.

is identiﬁed with

by the

identiﬁcation z

→ x

+ iy

.Letδ be the Euclidean metric of



∂

∂x

∂

∂x



= δ



∂

∂y

∂

∂y



= δ

µν



∂

∂x

∂

∂y



= 0.

(8.85a)

Noting that J ∂/∂x

= ∂/∂y

and J ∂/∂y

=−∂/∂x

,weﬁndthatδ is a

Hermitian metric. In complex coordinates, we have



∂

∂z

∂

∂z



= δ



∂

∂z

∂

∂z



= 0



∂

∂z

∂

∂z



= δ



∂

∂z

∂

∂z



µν

(8.85b)

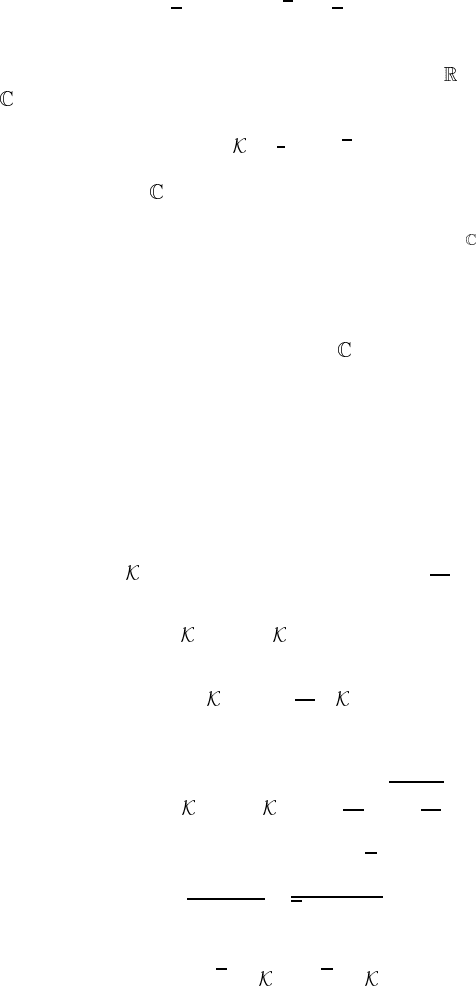
The K¨ahler form is given by

 =



µ=1

∧ dz



µ=1

∧ dy

. (8.86)

Clearly, d = 0 and we ﬁnd that the Euclidean metric δ of

is a K¨ahler metric

.TheK¨ahler potential is



. (8.87)

The K¨ahler manifold

is called the complex Euclid space.

Example 8.7. Any orientable complex manifold M with dim

M = 1isK¨ahler.

Take a Hermitian metric g whose K¨ahler form is .Since is a real two-form, a

three-form d has to vanish on M. One-dimensional compact orientable complex

manifolds are known as Riemann surfaces.

Example 8.8. A complex projective space

is a K¨ahler manifold. Let

,ϕ

) be a chart whose inhomogeneous coordinates are ϕ

( p) = ξ

(α)

, ν = α

(see example 8.3). It is convenient to introduce a tidier notation {ζ

(α)

|1 ≤ ν ≤

m} by

(α)

= ζ

(α)

(ν ≤ α −1)ξ

ν+1

(α)

= ζ

(α)

(ν ≥ α). (8.88)

{ζ

(α)

} is just a renaming of {ξ

(α)

}. Deﬁne a positive-deﬁnite function

( p) ≡



ν=1

|ζ

(α)

( p)|

+ 1 =

m+1



ν=1



. (8.89)

At a point p ∈ U

∩U

( p) and

( p) are related as

( p) =



( p). (8.90)

Then it follows that

log

= log

+ log

. (8.91)

Since z

is a holomorphic function, we have ∂ log z

= 0. Also

∂

log z

= ∂ log z

= 0.

Then it follows that

∂

∂ log

= ∂∂ log

. (8.92)

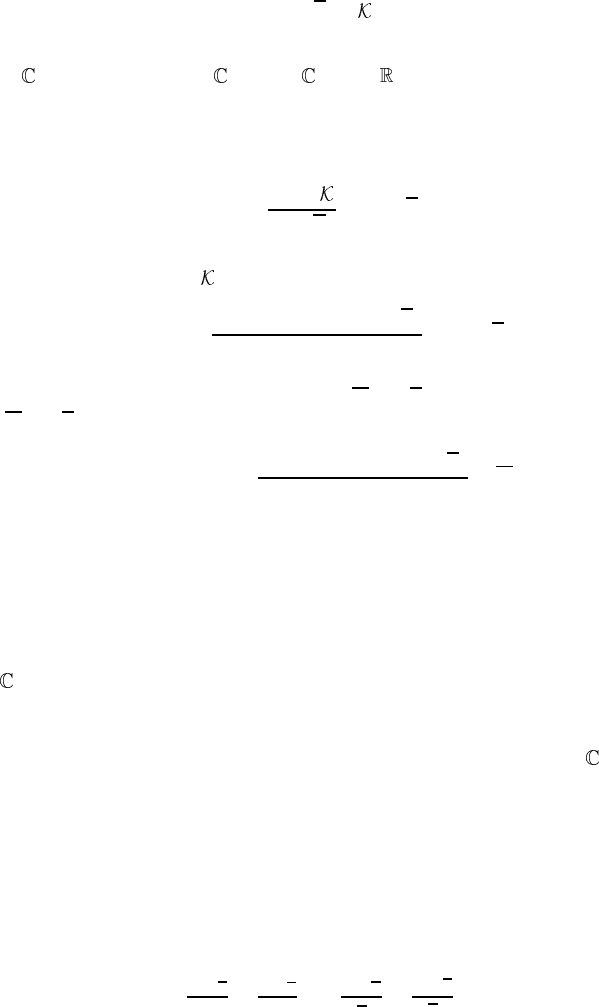
A closed two-form  is locally deﬁned by

 ≡ i∂

∂ log

. (8.93)

There exists a Hermitian metric whose K¨ahler form is .TakeX, Y ∈

and deﬁne g : T

⊗ T

→ by g(X, Y ) = (X, JY).To

prove that g is a Hermitian metric, we have to show that g satisﬁes (8.50) and is

positive deﬁnite. The Hermiticity is obvious since g(JX, JY) =−(JX, Y ) =

(Y, JX) = g(X, Y ). Next, we show that g is positive deﬁnite. On a chart

,ϕ

), we obtain

 = i

∂

log

∂ζ

dζ

∧ dζ

(8.94)

where we have dropped the subscript (α) to simplify the notation. If we substitute

the expression (8.89) for

on U

,wehave

 = i



µ,ν

µν

(



|ζ

+ 1) − ζ

(



|ζ

+ 1)

dζ

∧ dζ

. (8.95)

Let X be a real vector, X = X

∂/∂ζ

+ X

∂/∂ζ

and JX = iX

∂/∂ζ

−

∂/∂ζ

.Then

g(X, X) = (X, JX) = 2



µ,ν

µν

(



|ζ

+ 1) − ζ

(



|ζ

+ 1)

= 2









|ζ

+ 1



−











|ζ

+ 1



−2

From the Schwarz inequality



|ζ

≥



,weﬁndthe

metric g is positive deﬁnite. This metric is called the Fubini–Study metric of

A few useful facts are:

(a) S

is the only sphere which admits a complex structure. Since S

 P

,it

is a K¨ahler manifold.

(b) A product of two odd-dimensional spheres S

2m+1

× S

2n+1

always admits a

complex structure. This complex structure does not admit a K¨ahler metric.

8.5.2 K

ahler geometry

AK¨ahler metric g is characterized by (8.82):

∂g

∂z

∂g

λν

∂z

∂g

∂z

∂g

µλ

∂z

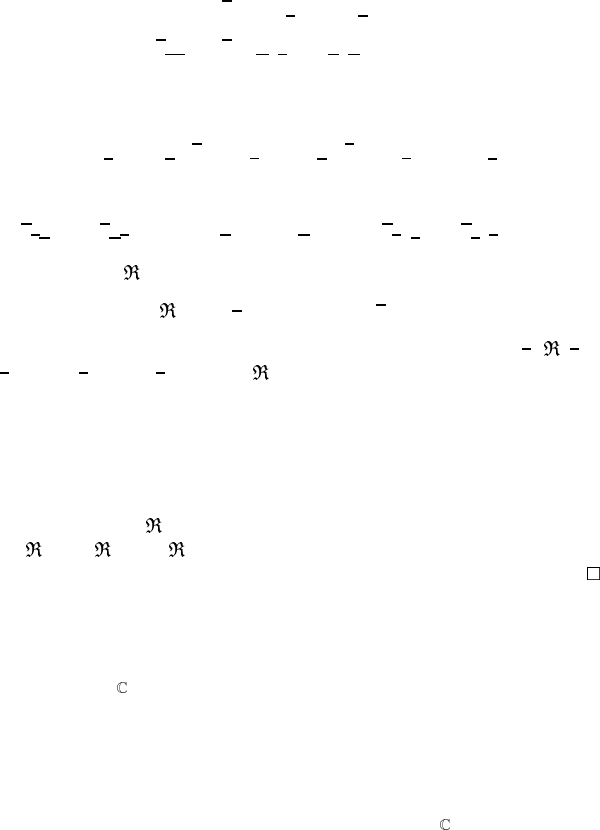
This ensures that the K¨ahler metric is torsion free:

µν

= g

ξλ

(∂

νξ

− ∂

µξ

) = 0 (8.96a)

µν

= g

λξ

(∂

νξ

− ∂

µξ

) = 0. (8.96b)

In this sense, the K¨ahler metric deﬁnes a connection which is very similar to the

Levi-Civita connection. Now the Riemann tensor has an extra symmetry

λµ

=−∂

ξκ

∂

λξ

) =−∂

ξκ

∂

µξ

) = R

µλ

(8.97)

as well as those obtained from (8.97) by known symmetry operations,

λµν

= R

µλν

, R

= R

, R

λµν

= R

νµλ

. (8.98)

The Ricci form

is deﬁned as before,

=−i∂

∂

log G dz

∧ dz

Because of (8.97), the components of the Ricci form agree with Ric

µν

;

µν

≡

κµ

= R

µκ

= Ric

.IfRic = = 0, the K¨ahler metric is said to be Ricci

ﬂat.

Theorem 8.6. Let (M, g) beaK¨ahler manifold. If M admits a Ricci ﬂat metric h,

then its ﬁrst Chern class must vanish.

Proof. By assumption,

= 0forthemetrich. As was shown in the previous

section,

(g) − (h) = (g) = dω. Hence, c

(M) computed from g agrees

with that computed from h and hence vanishes.

A compact K¨ahler manifold with vanishing ﬁrst Chern class is called a

Calabi–Yau manifold. Calabi (1957) conjectured that if c

(M) = 0, the K¨ahler

manifold M admits a Ricci-ﬂat metric. This is proved by Yau (1977). Calabi–Yau

manifolds with dim

M = 3 have been proposed as candidates for superstring

compactiﬁcation (see Horowitz (1986) and Candelas (1988)).

8.5.3 The holonomy group of K

ahler manifolds

Before we close this section, we brieﬂy look at the holonomy groups of K¨ahler

manifolds. Let (M, g) be a Hermitian manifold with dim

M = m.Takea

vector X ∈ T

and parallel transport it along a loop c at p. Then we end up

with a vector X



∈ T

where X



= X

. Note that ∇ does not mix the

holomorphic indices with anti-holomorphic indices, hence X



has no components

in T

−

. Moreover, ∇ preserves the length of a vector. These facts tell us that

(c)) is contained in U(m) ⊂ O(2m).

Theorem 8.7. If g is the Ricci-ﬂat metric of an m-dimensional Calabi–Yau

maifold M, the holonomy group is contained in SU(m).

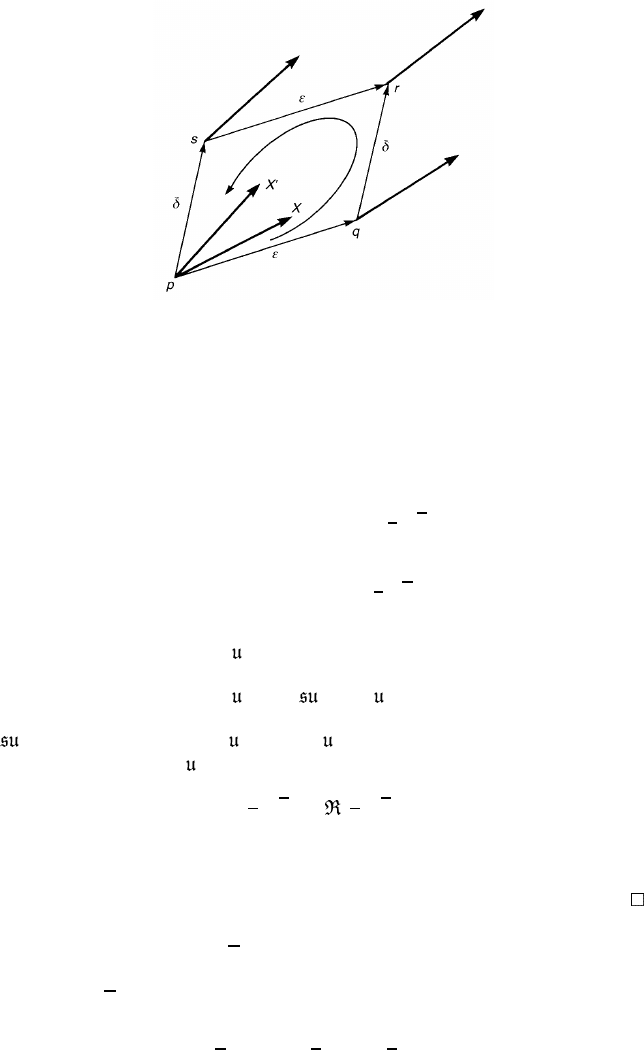
Figure 8.5. X ∈ T

is parallel transported along pqrs and comes back as a vector



∈ T

Proof. Our proof is sketchy. If X = X

∂/∂z

∈ T

is parallel transported

along the small parallelogram in ﬁgure 8.5 back to p,wehaveX



∈ T

whose

components are (cf (7.44))



= X

+ X

νκ

(8.99)

from which we ﬁnd

= δ

+ R

µκ

. (8.100)

U(m) is decomposed as U(m) = SU(m)×U(1) in the vicinity of the unit element.

In particular, the Lie algebra

(m) = T

(U(m)) is separated into

(m) = (m) ⊕ (1). (8.101)

(m) is the traceless part of (m) while (1) contains the trace. Since the present

metric is Ricci ﬂat, the

(1) part vanishes,

κµ

= 0.

This shows that the holonomy group is contained in SU(m).[Remark: Strictly

speaking, we have only shown that the restricted holonomy group is contained in

SU(m). This statement remains true even when M is multiply connected.]

8.6 Harmonic forms and ∂-cohomology groups

The (r, s)th

∂-cohomology group is deﬁned by

r,s

∂

(M) ≡ Z

r,s

∂

(M)/B

r,s

∂

(M). (8.102)

8.6 HARMONIC FORMS AND ∂-COHOMOLOGY GROUPS 337

An element [ω]∈H

r,s

∂

(M) is an equivalence class of ∂-closed forms of bidegree

(r, s) which differ from ω by a

∂-exact form,

[ω]={η ∈ 

r,s

(M)|

∂η = 0,ω− η = ∂ψ,ψ ∈ 

r,s−1

(M)}. (8.103)

Clearly H

r,s

∂

(M) is a complex vector space. Similarly to the de Rham

cohomology groups, the

∂-cohomology groups of

are trivial, that is, all the

closed (r, s)-forms are exact. The

∂-cohomology groups measure the topological

non-triviality of a complex manifold M.

8.6.1 The adjoint operators ∂

†

and ∂

†

Let M be a Hermitian manifold with dim M = m. Deﬁne the inner product

between α, β ∈ 

r,s

(M)(0 ≤ r, s ≤ m) by

(α, β) ≡



α ∧ ∗β (8.104)

where

∗:

r,s

(M) → 

m−r,m−s

(M) is the Hodge ∗ deﬁned by

∗β ≡ ∗β =∗β (8.105)

where ∗β is computed according to (7.173) extended to 

r+s

(M) .[Remark: ∗

maps an (r, s)-form to an (m −s, m −r)-form since it acts on a basis of 

r,s

(M),

up to an irrelevant factor, as

∗ dz

∧ ...∧ dz

∧ dz

∧ ...∧ dz

∼ ε

...µ

r+1

...

...ν

s+1

...ν

× dz

r+1

∧ ...∧ dz

∧ dz

s+1

∧ ...∧ dz

Note that the above ε-symbols are the only non-vanishing components in a

Hermitian manifold. Now it follows that

∗:

r,s

(M) → 

m−r,m−s

(M).]

We deﬁne the adjoint operators ∂

†

and ∂

†

of ∂ and ∂ by

(α, ∂β) = (∂

†

α, β) (α, ∂β) = (∂

†

α, β). (8.106)

The operators ∂

†

and ∂

†

change the bidegrees as ∂

†

: 

r,s

(M) → 

r−1,s

(M)

and

∂

†

: 

r,s

(M) → 

r,s−1

(M). Clearly d

†

= ∂

†

+ ∂

†

. Noting that a

complex manifold M is even dimensional as a differentiable manifold, we have

(see (7.184a))

†

=−∗d ∗ . (8.107)

Proposition 8.3.

∂

†

=−∗∂∗, ∂

†

=−∗∂ ∗ . (8.108)

Proof.Letω ∈ 

r−1,s

(M) and ψ ∈ 

r,s

(M). If we note that ω ∧ ∗ψ ∈



m−1,m

(M) and hence ∂(ω ∧ ∗ψ) = 0, we ﬁnd that

d (ω ∧

∗ψ) = ∂(ω ∧ ∗ψ) = ∂ω ∧ ∗ψ + (−1)

r+s−1

ω ∧ ∂(∗ψ)

= ∂ω ∧

∗ψ + (−1)

r+s−1

ω ∧ (−1)

r+s+1

∗∗∂(∗ψ)

= ∂ω ∧

∗ψ + ω ∧ ∗∗∂∗ψ (8.109)

where use has been made of the facts ∂

∗ψ ∈ 

2m−r−s−1

(M), ∗∗β =∗∗β and

(7.176a). If (8.109) is integrated over a compact complex manifold M with no

boundary, we have

0 = (∂ω, ψ) + (ω,

∗∂∗ψ).

The second term is

(ω,

∗∂∗ψ) = (ω, ∗∂ ∗ ψ) = (ω, ∗∂ ∗ ψ).

We ﬁnally ﬁnd 0 = (∂ω, ψ) + (ω, ∗

∂ ∗ ψ), namely ∂

†

=−∗∂∗. The other

formula

∂

†

=−∗∂∗ follows similarly.

As a corollary of proposition 8.3, we have

(∂

†

)

= (∂

†

)

= 0. (8.110)

8.6.2 Laplacians and the Hodge theorem

Besides the usual Laplacian  = (dd

†

d), we deﬁne other Laplacians 

∂

and



∂

on a Hermitian manifold,



∂

≡ (∂ + ∂

†

)

= ∂∂

†

+ ∂

†

∂ (8.111a)



∂

≡ (∂ + ∂

†

)

= ∂∂

†

+ ∂

†

∂. (8.111b)

An (r, s)-form ω which satisﬁes 

∂

ω = 0 (

∂

ω = 0) is said to be ∂-harmonic

(

∂-harmonic). If 

∂

ω = 0 (

∂

ω = 0), ω satisﬁes ∂ω = ∂

†

ω = 0 (∂ω = ∂

†

ω =

0).

We have the complex version of the Hodge decomposition. Let Harm

r,s

∂

(M)

be the set of

∂-harmonic (r, s)-forms,

Harm

r,s

∂

(M) ≡{ω ∈ 

r,s

(M)|

∂

ω = 0}. (8.112)

Theorem 8.8. (Hodge’s theorem) 

r,s

(M) has a unique orthogonal decomposi-

tion:



r,s

(M) = ∂

r,s−1

(M) ⊕ ∂

†



r,s+1

(M) ⊕ Harm

r,s

∂

(M) (8.113a)

8.6 HARMONIC FORMS AND ∂-COHOMOLOGY GROUPS 339

namely an (r, s)-form ω is uniquely expressed as

ω =

∂α + ∂

†

β + γ(8.113b)

where α ∈ 

r,s−1

(M), β ∈ 

r,s+1

(M) and γ ∈ Harm

r,s

∂

(M).

The proof is found in lecture 22, Schwartz (1986), for example. If ω is

∂-

closed, we have

∂ω = ∂ ∂

†

β = 0. Then 0 =β, ∂ ∂

†

β=∂

†

β, ∂

†

β≥0

implies

∂

†

β = 0. Thus, any closed (r, s)-form ω is written as ω = γ + ∂α,

α ∈ 

r,s−1

(M). This shows that H

r,s

∂

(M) ⊂ Harm

r,s

∂

(M). Note also that

Harm

r,s

∂

(M) ⊂ Z

r,s

∂

(M) since ∂γ = 0forγ ∈ Harm

r,s

∂

(M). Moreover,

Harm

r,s

∂

(M) ∩ B

r,s

∂

(M) =∅since B

r,s

∂

(M) = ∂

r,s−1

(M) is orthogonal to

Harm

r,s

∂

(M). Then it follows that Harm

r,s

∂

(M)

∼

r,s

∂

(M).IfP : 

r,s

(M) →

Harm

r,s

∂

(M) denotes the projection operator to a harmonic (r, s)-form, [ω]∈

r,s

∂

(M) has a unique harmonic representative Pω ∈ Harm

r,s

∂

(M).

8.6.3 Laplacians on a K

ahler manifold

In a general Hermitian manifold, there exist no particular relationships among

the Laplacians , 

∂

and 

∂

.However,ifM is a K¨ahler manifold, they are

essentially the same. [Note that the Levi-Civita connection is compatible with the

Hermitian connection in a K¨ahler manifold.]

Theorem 8.9. Let M beaK¨ahler manifold. Then

 = 2

∂

= 2

∂

. (8.114)

The proof requires some technicalities and we simply refer to Schwartz

(1986) and Goldberg (1962). This theorem puts constraints on the cohomology

groups of a K¨ahler manifold M. A form ω which satisﬁes

∂ω = ∂

†

ω = 0

also satisﬁes ∂ω = ∂

†

ω = 0. Let ω be a holomorphic p-form; ∂ω = 0.

Since ω contains no d

in its expansion, we have ∂

†

ω = 0, hence 

∂

ω =

(

∂ ∂

†

+ ∂

†

∂)ω = 0. According to theorem 8.9, we then have ω = 0, that is any

holomorphic form is automatically harmonic with respect to the K¨ahler metric.

Conversely ω = 0 implies

∂ω = 0, hence every harmonic form of bidegree

( p, 0) is holomorphic.

8.6.4 The Hodge numbers of K

ahler manifolds

The complex dimension of H

r,s

∂

(M) is called the Hodge number b

r,s

.The

cohomology groups of a complex manifold are summarized by the Hodge

diamond,







m,m

m,m−1

m−1,m

...

m,0

m−1,1

... b

1,m−1

0,m

...

1,0

0,1

0,0







. (8.115)

These (m + 1)

Hodge numbers are far from independent as we shall see later.

Theorem 8.10. Let M be a K¨ahler manifold with dim

M = m. Then the Hodge

numbers satisfy

(a) b

r,s

= b

s,r

(8.116)

(b) b

r,s

= b

m−r,m−s

. (8.117)

Proof.(a)Ifω ∈ 

r,s

(M) is harmonic, it satisﬁes 

∂

ω = 

∂

ω = 0. Then the

(s, r)-form

ω is also harmonic, 

∂

ω = 0since

∂

ω = 

∂

ω = 

∂

ω = 0 (note

that 

∂

= 

∂

). Thus, for any harmonic form of bidegree (r, s), there exists a

harmonic form of bidegree (s, r ) and vice versa. Thus, it follows that b

r,s

= b

s,r

(b) Let ω ∈ 

r,s

(M) and ψ ∈ H

m−r,m−s

∂

(M).Thenω ∧ ψ is a volume element

and it can be shown (Schwartz 1986) that



ω ∧ ψ deﬁnes a non-singular

map H

r,s

∂

(M) × H

m−r,m−s

∂

(M) → , hence the duality between H

r,s

∂

(M) and

m−r,m−s

∂

(M). This shows that H

r,s

∂

(M) is isomorphic to H

m−r,m−s

∂

(M) as a

vector space and it follows that dim

r,s

∂

(M) = dim H

m−r,m−s

∂

(M) hence

r,s

= b

m−r,m−s

Accordingly, the Hodge diamond of a K¨ahler manifold is symmetric about

the vertical and horizontal lines. These symmetries reduce the number of

independent Hodge numbers to (

m + 1)

if m is even and

(m + 1)(m + 3)

if m is odd.

In a general Hermitian manifold, there are no direct relations between the

Betti numbers and the Hodge numbers. If M is a K¨ahler manifold, however,

theorem 8.11 establishes close relationships between them.

Theorem 8.11. Let M be a K¨ahler manifold with dim

M = m and ∂ M =∅.

Then the Betti numbers b

(1 ≤ p ≤ 2m) satisfy the following conditions;

(a) b



r+s=p

r,s

(8.118)

(b) b

2 p−1

is even (1 ≤ p ≤ m) (8.119)

2 p

≥ 1 (1 ≤ p ≤ m) (8.120)

Proof.(a)H

r,s

∂

(M) is a complex vector space spanned by 

∂

-harmonic (r, s)-

forms, H

r,s

∂

(M) ={[ω]|ω ∈ 

r,s

(M), 

∂

ω = 0}. Note also that, H

(M)

is a real vector space spanned by -harmonic p-forms, H

(M) ={[ω]|ω ∈



(M), ω = 0}. Then the complexiﬁcation of H

(M) is H

(M) ={[ω]|ω ∈



(M) ,ω = 0}.SinceM is K¨ahler, any form ω which satisﬁes 

∂

ω = 0also

satsiﬁes ω = 0andvice versa.Since



(M) =⊕

r+s=p



r,s

(M)

we ﬁnd that

(M) =⊕

r+s=p

r,s

(M).

Noting that dim

(M) = dim H

(M) , we obtain b



r+s=p

r,s

(b) From (a) and (8.116), it follows that

2 p−1



r+s=2p−1

r,s

= 2



r+s=2p−1

r>s

r,s

Thus, b

2 p−1

must be even.

form, d = 0, and the real 2p-form



=  ∧...∧

-. /

is also closed, d

= 0. We show that 

is not exact. Suppose 

= dη for

some η ∈ 

2 p−1

(M).Then

= 

m−p

∧

= d (

m−p

∧η). It follows from

Stokes’ theorem that







d(

m−p

∧ η) =



∂ M



m−p

∧ η = 0.

Since the LHS is the volume of M, this is in contradiction. Thus, there is at least

one non-trivial element of H

2 p

(M) and we have proved that b

2 p

≥ 1.

If a K¨ahler manifold is Ricci ﬂat, there exists an extra relationship among

the Hodge numbers, which further reduces the independent Hodge numbers, see

Horowitz (1986) and Candelas (1988).

8.7 Almost complex manifolds

This and the next sections deal with spaces which are closely related to complex

manifolds. These are somewhat specialized topics and may be omitted on a ﬁrst

reading.