Myint Tyn U., Debnath L. Linear Partial Differential Equations for Scientists and Engineers
Подождите немного. Документ загружается.

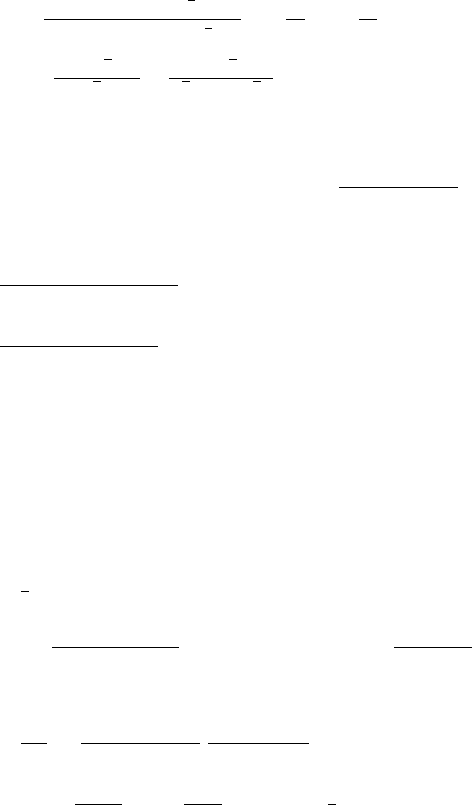
10.13 Exercises 731
10.13 Exercises
1. u (x, y, z)=
sinh
[
(π/b)
2
+(π/c)
2
]
1
2
(a−x)
sinh
[
(π/b)
2
+(π/c)
2
]
1
2
a
sin
πy
b
sin
πz
c
.
2. u (x, y, z)=
sinh
(
√
2 πz
)
√
2 π
−
cosh
(
√
2 πz
)
√
2 π tanh
√
2 π
cos πx cos πy.
4. (a) u (r, θ, z)=
∞
m=0
∞
n=1
(a
mn
cos mθ + b
mn
sin mθ) J
mn
(a
mn
r/a)
×
sinh α
mn
(l−z)/a
sinh α
mn
l/a
,
where
a
mn
=
2
a
2
πε
n
[J
m+1
(α
mn
)]
2
2π
0
a
0
f (r, θ) J
m
(α
mn
r/a)cosmθrdrdθ
b
mn
=
2
a
2
π [J
m+1
(α
mn
)]
2
2π
0
a
0
f (r, θ) J
m
(α
mn
r/a)sinmθrdrdθ
with
ε
n
=
⎧
⎪
⎨
⎪
⎩
1, f or m =0
2, f or m =0
and α
mn
is the nth root of the equation J
m
(α
mn
)=0.
5. u (r, θ)=
1
3
+
2/3a
2
r
2
P
2
(cos θ).
7. u (r, z)=
∞
n=1
a
n
sinh α
n
(l−z)/a
cosh α
n
l/a
J
0
(α
n
r/a), where a
n
=
2qu
kα
2
n
J
0
(α
n
)
and
α
n
is the root of J
0
(α
n
)=0andk is the coefficient of heat conduction.
8. u (r, z)=
4u
0
π
∞
n=1
[I
0
(2n+1)(πr/l)]
[I
0
(2n+1)(πa/l)]
sin(2n+1)πz/l
(2n+1)
.
9. u (r, θ)=u
2
+
u
1
−u
2
2
∞
n=1
2n+1
n+1
P
n−1
(0)
r
a
n
P
n
(cos θ).
11. u (r, θ, φ)=C +
∞
n=1
∞
m=0
r
n
P
m
n
(cos θ)[a
nm
cos mφ + b
nm
sin nφ] ,
where
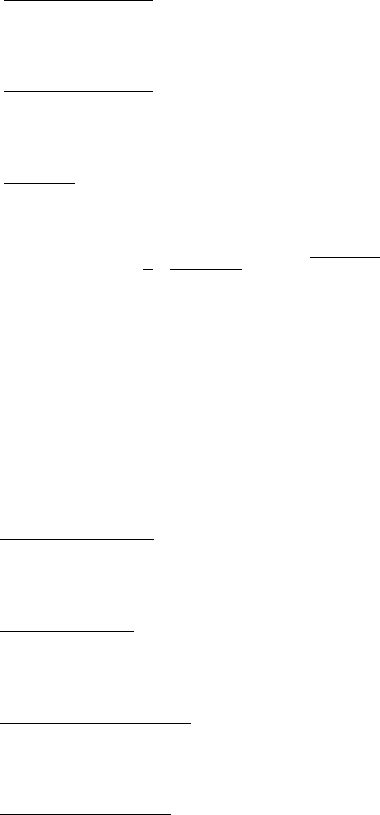
732 Answers and H ints to Selected Exercises
a
nm
=
(2n +1)(n − m)!
2nπ (n + m)!
2π
0
π
0
f (θ, ϕ) P
m
n
(cos θ)cosmϕ sin θdθdϕ
b
nm
=
(2n +1)(n − m)!
2nπ (n + m)!
2π
0
π
0
f (θ, ϕ) P
m
n
(cos θ)sinmϕ sin θdθdϕ
a
n0
=
(2n +1)
4nπ
2π
0
π
0
f (θ, ϕ) P
n
(cos θ)sinθdθdϕ.
12. u (x, y, t)=
∞
n=1,3,4,...
−
4
π
[1−(−1)
n
]
n(n
2
−4)
cos
(n
2
+1)πct
(sin nπx sin nπy).
13. u (r, θ, t)=
∞
n=0
∞
m=1
J
n
(α
mn
r/a) cos (α
mn
ct/a)[a
mn
cos nθ + b
mn
sin nθ]
+
∞
n=0
∞
m=1
J
n
(α
mn
r/a)sin(α
mn
ct/a)[c
mn
cos nθ + d
mn
sin nθ],
where
a
mn
=
2
πa
2
ε
n
[J
′
n
(α
mn
)]
2
2π
0
a
0
f (r, θ) J
n
(α
mn
r/a)cosnθrdrdθ
b
mn
=
2
πa
2
[J
′
n
(α
mn
)]
2
2π
0
a
0
f (r, θ) J
n
(α
mn
r/a)sinnθrdrdθ
c
mn
=
2
πacα
mn
ε
n
[J
′
n
(α
mn
)]
2
2π
0
a
0
g (r, θ) J
n
(α
mn
r/a)cosnθrdrdθ
d
mn
=
2
πacα
mn
[J
′
n
(α
mn
)]
2
2π
0
a
0
g (r, θ) J
n
(α
mn
r/a)sinnθrdrdθ
in which α
mn
is the root of the equation J
n
(α
mn
)=0and
ε
n
=
⎧
⎪
⎨
⎪
⎩
2 n =0
1 n =0
.

10.13 Exercises 733
15. u (r, θ, t)=
∞
n=0
∞
m=1
J
n
(α
mn
r)exp(−α
mn
kt)[a
mn
cos nθ + b
mn
sin nθ],
where
a
nm
=
2
πε
n
[J
′
n
(α
mn
)]
2
2π
0
1
0
f (r, θ) J
n
(α
mn
r)cosnθrdrdθ,
b
nm
=
2
π [J
′
n
(α
mn
)]
2
2π
0
1
0
f (r, θ) J
n
(α
mn
r)sinnθrdrdθ,
where α
mn
is the root of the equation J
n
(α
mn
)=0and
ε
n
=
⎧
⎪
⎨
⎪
⎩
1forn =0
2forn =0.
16. u (x, y, z, t)=sinπx sin πy sin πz cos
√
3 πct
.
18. u (r, θ, z, t)=
∞
n=0
∞
m=1
∞
l=1
J
n
(α
mn
r/a)sin(mπz/l) cos (ωct)
×[a
nml
cos nθ + b
nml
sin nθ]
+
∞
n=0
∞
m=1
∞
l=1
J
n
(α
mn
r/a)sin(mπz/l)sin(ωct)
×[c
nml
cos nθ + d
nml
sin nθ],
where
a
nml
=
4
πa
2
lε
n
[J
′
n
(α
mn
)]
2
a
0
2π
0
l
0
f (r, θ, z) J
n
(α
mn
r/a)
×sin (mπz/l)cosnθrdrdθdz,
b
nml
=
4
πa
2
l [J
′
n
(α
mn
)]
2
a
0
2π
0
l
0
f (r, θ, z) J
n
(α
mn
r/a)
×sin (mπz/l)sinnθrdrdθdz,
c
nml
=
4 ω
−1
πa
2
lε
n
[J
′
n
(α
mn
)]
2
a
0
2π
0
l
0
g (r, θ, z) J
n
(α
mn
r/a)
×sin (mπz/l)cosnθrdrdθdz,

734 Answers and H ints to Selected Exercises
d
nml
=
4 ω
−1
πa
2
l [J
′
n
(α
mn
)]
2
a
0
2π
0
l
0
g (r, θ, z) J
n
(α
mn
r/a)
×sin (mπz/l)sinnθrdrdθdz,
where α
mn
is the root of the equation J
n
(α
mn
)=0and
ω =
"
(mπ/l)
2
+(α
mn
/a)
2
#
1
2
,ε
n
=
⎧
⎪
⎨
⎪
⎩
1; for n =0
2; for n =0.
20. u (r, θ, z, t)=
∞
n=0
∞
m=1
∞
p=1
(a
nmp
cos nθ + b
nmp
sin nθ)
×J
n
(α
mn
r/a)sin(pπz/l) e
−ωt
,
where
a
nmp
=
4
πa
2
lε
n
[J
′
n
(α
mn
)]
2
a
0
2π
0
l
0
f (r, θ, z) J
n
(α
mn
r/a)
×sin (pπz/l)cosnθrdrdθdz
b
nmp
=
4
πa
2
l [J
′
n
(α
mn
)]
2
a
0
2π
0
l
0
f (r, θ, z) J
n
(α
mn
r/a)
×sin (pπz/l)sinnθrdrdθdz,
in which
ε
n
=
⎧
⎪
⎨
⎪
⎩
1forn =0
2forn =0
and ω =
"
(pπ/l)
2
+(α
mn
/a)
2
#
.
23. u (x, y, t)=
∞
m=1
∞
n=1
u
mn
(t)sinmx sin ny,
where
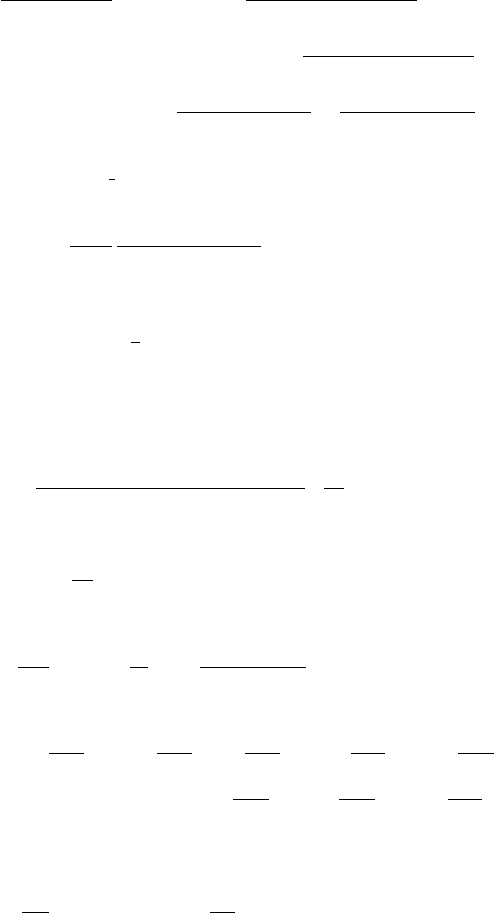
10.13 Exercises 735
u
mn
(t)=
4(−1)
m+n+1
mn α
mn
c
sin (α
mn
ct)
cos (1 − α
mn
c) t − 1
2(1− α
mn
c)
+
cos (1 + α
mn
c) t − 1
2(1+α
mn
c)
+ cos (α
mn
ct)
sin (1 − α
mn
c) t
2(1− α
mn
c)
+
sin (1 + α
mn
c) t
2(1+α
mn
c)
,
and α
mn
=
m
2
+ n
2
1
2
.
25. u (x, y, t)=
∞
n=1
∞
m=1
4A
mnπ
2
[(−1)
n
−1][(−1)
n
−1]
k(n
2
+m
2
)
"
1 − e
−k
(
n
2
+m
2
)
t
#
×sin nx (sin my − m cos my).
27. u (x, y, t)=x (x − π)
1 −
y
π
sin t +
∞
n=1
∞
m=1
v
mn
(t)sinnx sin my,where
α
2
mn
=
m
2
+ n
2
and
v
mn
(t)=
8exp
−c
2
α
2
tα
2
mn
[1 − (−1)
n
]
π
2
mn (1 + c
4
α
4
mn
)
c
2
n
2
α
2
mn
− n
2
×
&
cos t exp
−c
2
α
2
tα
2
mn
− 1
'
+
1
n
2
+ c
4
α
2
mn
sin t exp
−c
2
α
2
tα
2
mn
.
30. u (x, y, t)=
4qb
4
π
5
D
∞
n=1,3,...
1
n
5
"
1 −
v
n
(x)
1+cosh(nπa/b)
#
sin (nπy/b), where
v
n
(x) = 2 cosh
nπa
2b
cosh
nπx
b
+
nπa
2b
sinh
nπa
2b
cosh
nπx
b
−
nπx
b
sinh
nπx
b
cosh
nπa
2b
.
32. Hint: In region 1, x ≤−a, the solution of the Schr¨odinger equation
d
2
ψ
dx
2
= κ
2
ψ, κ
2
=
2M
2
(V
0
− E) ,
is
ψ
1
(x)=Ae
κx
+ Be
−κx
,
where A and B are constants. For boundedness of the solution as

736 Answers and H ints to Selected Exercises
x →−∞, B ≡ 0, and hence, ψ
1
(x)=Ae
κx
.
In region 2, x ≥ a, the solution of the Schr¨odinger equation is
ψ
2
(x)=Ce
κx
+ De
−κx
.
For boundedness as x →∞, C ≡ 0. The solution is ψ
2
(x)=De
−κx
.
In region 3, −a ≤ x ≤ a, the potential is zero and hence, the equation
takes the simple form ψ
xx
+ k
2
ψ =0,wherek
2
=
2M
2
E.Thesolu-
tion is ψ
3
(x)=E sin kx + F cos kx. For matching conditions at x = a,
ψ
2
(a)=ψ
3
(a),
or, De
−aκ
= E sin ka+ F cos ak. (1)
Similarly, matching conditions at x = −a gives ψ
1
(−a)=ψ
3
(−a),
or Ae
−aκ
= −E sin ak + F cos ak. (2)
Further, matching the d erivatives ψ
′
(a), ψ
′
(−a)givesψ
′
2
(a)=ψ
′
3
(a)
and ψ
′
1
(−a)=ψ
′
3
(−a),
or −κDe
−aκ
= k (E cos ak − F sin ak), (3)
κAe
−κa
= k (E cos ak − F sin ak). (4)
Adding and subtracting (1) and (2) gives
2F cos ak =(A + D) e
−aκ
, 2E sin ak = −(A − D) e
−aκ
.
Adding and subtracting (3) and (4) gives
2kEcos ak = −κ (A − D) e
−aκ
, 2kF sin ak = κ (A + D) e
−aκ
.
Setting A − D = −A
1
and A + D = A
2
, the last two sets of equations
can be combined and rewritten as
2E sin ak − A
1
e
−aκ
=0
2kEcos ak + κA
1
e
−aκ
=0
⎫
⎪
⎬
⎪
⎭
(5)
and
2F cos ak − A
2
e
−aκ
=0
2kFsin ak − κA
2
e
−aκ
=0
⎫
⎪
⎬
⎪
⎭
. (6)
The set (5) has nontrivial solutions for E and A
1
only if
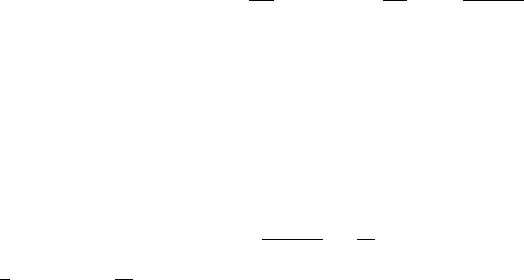
10.13 Exercises 737
2sinak −e
−aκ
2k cos ak κe
−aκ
= 0 which gives k cot ak = −κ.
Similarly, the set (6) has nontrivial solutions for F and A
2
only if
k tan ak = κ.
Note that it is impossible to satisfy both k cot ak = −κ and k tan ak = κ
simultaneously. Hence, there are two classes of solutions, and solution
is possible in quantum mechanics only if the energy satisfies certain
conditions.
1. Odd solutions: k cot ak = −κ. In this case, F = A
2
=0.Interms
of dimensionless variables, ξ = ak and η = aκ with definitions of k and
κ, it follows that
ξ
2
+ η
2
= a
2
k
2
+ κ
2
= a
2
2M
2
(V
0
− E)+
2M
2
E
!
=
2MV
0
a
2
2
. (7)
This represents a circle. In terms of ξ and η,wewritek cot ak = −κ as
ak cot ak = −aκ,orξ cot ξ = −η. (8)
The simultaneous solut ions of equations (7) and (8) can be determined
from graphs of these functions at their point of intersection. It turns out
that both ξ and η assume the positive values in the first quadrant only.
Clearly, in the range 0 ≤ α =
2MV
0
a
2
2
<
π
2
4
, there is no solution. For
π
2
2
≤ α ≤
3π
2
2
, there is one solution. Thus, the existence of solu-
tions depends on the parameters, M , V
0
and the range of the p otential.
A simultaneous solution determines the allowed energy for which the
quantum mechanical motion is described by an odd solution.
2. Even solutions: k tan ak = κ. In this case, E = A
1
= 0, and (5)
still holds. We can write the above condition in terms of nondimensional
variables as

738 Answers and H ints to Selected Exercises
ξ tan ξ = η. (9)
The simultaneous solutions of (7) and (9) can be found graphically as
before. It follows from the graphical representation that (7) and (9) in-
tersect once if 0 ≤ α<π
2
in the first quadrant. There are two points of
intersection if π
2
≤ α<(2π)
2
. The number of intersections (solutions)
increases with the value of the parameter α. For each such allowed
value of the energy determined from the points of intersection, there is
an even solution in the present case.
Note also that, for both even and odd solutions, ψ (x) is nonzero out-
side the finite square well so that there exists a nonzero probability for
finding the particle there. This result is different from what is expected
in classical mechanics. Finally, if V
0
→∞, it is easy to see that the
intersections occur at ξ = nπ,
n +
1
2
π which are in agreement with
the analysis of th e infinite square well potential discussed in Example
10.10.1.
33. Hint: The boundary conditions at x = −a yields the matching condi-
tions
Ae
−ika
+ Be
ika
= Ce
aκ
+ De
−aκ
,
Ae
−ika
− Be
ika
=
iκ
k
Ce
aκ
− De
−aκ
.
These results give the desired solution.
Similarly, matching conditions at x = a gives the desired answer.
Combining the matching relations leads to the final matrix equation.
11.11 Exercises 739
11.11 Exercises
3. u (ρ, θ)=
1
2π
2π
0
(
ρ
2
−1
)
f(β)dβ
[1−ρ
2
−2ρ cos(β−θ)]
.
7. u (x, y)=−
2
b
∞
n=1
sin(nπ y/b)
sinh(nπ a/b )
sinh
&
nπ
b
(a − x)
'
x
0
f (ξ)sinh
nπξ
b
dξ
+sinh
nπx
b
a
0
f (ξ)sinh
&
nπ
b
(a − ξ)
'
dξ
.
8. u (r, θ)=−
∞
n=0
∞
k=1
(R/α
nk
)
2
J
n
(α
nk
r/R)(A
nk
cos nθ + B
nk
sin nθ),
where
A
0k
=
1
πR
2
J
2
1
(α
0k
)
R
0
2π
0
rf (r, θ) J
0
(α
0k
r/R) dr dθ
A
nk
=
2
πR
2
J
2
n+1
(α
nk
)
R
0
2π
0
rf (r, θ) J
n
(α
nk
r/R)cosnθ dr dθ
B
nk
=
2
πR
2
J
2
n+1
(α
nk
)
R
0
2π
0
rf (r, θ) J
n
(α
nk
r/R)sinnθ dr dθ
n =1, 2, 3,...; k =1, 2, 3,... and α
nk
are the roots of J (α
nk
)=0.
9. G (r, r
′
)=
e
ik
|
r−r
′
|
|r−r
′
|
−
e
ik
|
ρ−r
′
|
|ρ−r
′
|
,
where r =(ξ, η,ζ), r
′
=(x, y, z), and ρ =(ξ,η,−ζ).
10. G (r, r
′
)=
e
ik
|
r−r
′
|
|r−r
′
|
+
e
ik
|
ρ−r
′
|
|ρ−r
′
|
.
14. G = −
4a
π
∞
n=1
∞
0
1
(α
2
a
2
+n
2
π
2
)
sin
nπx
a
sin
nπξ
a
sin αy sin αη dα.
16. u (r, z)=
2C
π
∞
0
∞
0
1
(κ
2
−λ
2
−β
2
)
J
0
(βr) J
1
(βa)cosλz dβ dλ.
17. u (r, θ)=Ar
1
2
sin (θ/2).
18. G = −
2
a
∞
n=1
sinh σy
′
sinh σ(y−b)
σ sinh σb
sin
nπx
a
sin
mπ x
′
a
,
σ =
(κ
2
+(n
2
π
2
) /α
2
), 0 <x
′
<x<a, 0 <y
′
<y<b.
740 Answers and H ints to Selected Exercises
12.18 Exercises
1. (a) F (k)=
(1/2a) e
−k
2
/4a
,(b)F (k)=
%
2
π
a
(a
2
+k
2
)
.
2. F
a
(k)=
(2/π)(sinka) /k. This function F
a
(k) is called the band
limited function.
3. (a) F
a
(k)=1/ |k|.(b)F (k)=
1
√
2π
a
−a
e
−iω x
dx =
%
2
π
sin aω
ω
.
(c) F (k)=
1
√
2π
a
−a
e
−ikx
1 −
|x|
a
dx =
2
√
2π
a
0
1 −
x
a
cos kx dx
=
2a
√
2π
1
0
(1 − x) cos ( a kx) dx =
2a
√
2π
1
0
(1 − x)
d
dx
sin akx
ak
dx
=
2a
√
2π
1
0
sin(akx)
ak
dx =
a
√
2π
1
0
d
dx
sin
2
(
1
2
akx
)
(
1
2
ak
)
2
dx
=
a
√
2π
sin
2
(
1
2
ak
)
(
1
2
ak
)
2
.
(d) F (k)=
π
2
exp(−a|k|)
a
.
4. (a) F (k)=
(1/2) sin
k
2
4
+
π
4
,(b)F (k)=
(1/2) cos
k
2
4
−
π
4
.
6. Hint: I (a, b)=
∞
0
e
−a
2
x
2
cos bx dx.
∂I
∂b
= −
b
2a
2
I ⇒ I = C exp
−
b
2
4a
2
.
Since I (a, 0) = C =
∞
0
e
−a
2
x
2
dx =
√
π
2a
, I (a, b)=
√
π
2a
exp
−b
2
/4a
2
.
7. (a) f (x)=
∞
−∞
f (t) δ (x − t) dt =
∞
−∞
f (t) dt
1
2π
∞
−∞
e
ik(x−t)
dk
=
1
√
2π
∞
−∞
e
ikx
dk
1
√
2π
∞
−∞
e
−ikt
f (t) dt =
1
√
2π
∞
−∞
e
ikx
F (k) dk.
8. (b) Omit the factor
1
√
2π
in the definition of convolution.
[f ∗(g ∗ h)] (x)=
∞
−∞
f (x − ξ)(g ∗ h)(ξ) dξ
=
∞
−∞
f (x − ξ) dξ
∞
−∞
g (ξ − t) h (t) dt
=
∞
−∞
∞
−∞
f (x − ξ) g (ξ − t)
h (t) dt (ξ − t = η)
