Myint Tyn U., Debnath L. Linear Partial Differential Equations for Scientists and Engineers
Подождите немного. Документ загружается.

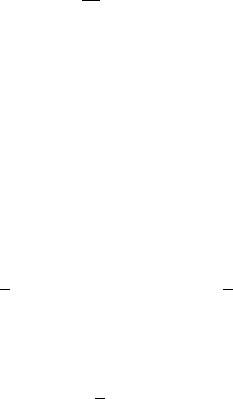
13.12 The Nonlinear Schr¨odinger Equation and Solitary Waves 581
The canonical form of the KdV equation
u
t
− 6uu
x
+ u
xxx
=0, (13.11.35)
canbewrittenas
(u)
t
+
−3u
2
+ u
xx
x
=0
so that
T = u and X = −3u
2
+ u
xx
. (13.11.36)
If we assume that u is periodic or that u and its derivatives decay very
rapidly as |x|→∞,then
d
dt
∞
−∞
udx =0.
This leads to the conservation of mass, that is,
∞
−∞
udx = constant. (13.11.37)
This is often called th e time invariant function of the solutions of the KdV
equation.
The second conservation law for (13.11.34) can be obtained by multi-
plying it by u so that
1
2
u
2
t
+
−2u
3
+ uu
x
−
1
2
u
2
x
x
=0. (13.11.38)
This gives
∞
−∞
1
2
u
2
dx = constant. (13.11.39)
This is the principle of conservation of energy.
It is well known that the KdV equation has an infinite number of poly-
nomial conservation laws. It is generally believed that the existence of a
soliton solution to t he KdV equation is closely related to the existence of
an infinite number of conservation laws.
13.12 The Nonlinear Schr¨odinger Equation and
Solitary Waves
We first d erive the one-dimensional linear Schr¨odinger equation from the
Fourier integral representation of the plane wave solution

582 13 Nonlinear Partial Differential Equations with Applications
φ (x, t)=
∞
−∞
F (k)exp[i (kx − ωt)] dk, (13.12.1)
where the spectrum function F (k) is determined from the given initial or
boundary conditions.
We assume that the wave is slowly modulated as it propagates in a dis-
persive medium. For such a modulated wave, most of the energy is confined
in the neighborhood of k = k
0
so that th e dispersion relation ω = ω (k)can
be expan ded about the point k = k
0
as
ω = ω (k)=ω
0
+(k − k
0
) ω
′
0
+
1
2
(k − k
0
)
2
ω
′′
0
+ ..., (13.12.2)
where ω
0
≡ ω (k
0
), ω
′
0
≡ ω
′
(k
0
), ω
′′
0
≡ ω
′′
(k
0
).
In view of (13.12.2), we can rewrite (13.12.1) as
φ (x, t)=ψ (x, t)exp[i (k
0
x − ω
0
t)] , (13.12.3)
where the amplitude ψ (x, t)isgivenby
ψ (x, t)=
∞
−∞
F (k)exp
i (k − k
0
) x − i
(k − k
0
) ω
′
0
+
1
2
(k − k
0
)
2
ω
′′
0
t
dk. (13.12.4)
Evidently, this represents the slowly vary ing (or modulated) part of the
basic wave. A simple computation of ψ
t
, ψ
x
,andψ
xx
gives
ψ
t
= −i
(k − k
0
) ω
′
0
+
1
2
(k − k
0
)
2
ω
′′
0
ψ
ψ
x
= i (k − k
0
) ψ
ψ
xx
= −(k − k
0
)
2
ψ
so that
i (ψ
t
+ ω
′
0
ψ
x
)+
1
2
ω
′′
0
ψ
xx
=0. (13.12.5)
The dispersion relation associated with this linear equation is given by
ω = kω
′
0
+
1
2
k
2
ω
′′
0
. (13.12.6)
If we choose a frame of reference moving with the linear group velocity,
that is, x
∗
= x −ω
′
0
t, t
∗
= t, the term involving ψ
x
is dropped and then, ψ
satisfies the linear Schr¨odinger equation, dropping the asterisks,

13.12 The Nonlinear Schr¨odinger Equation and Solitary Waves 583
iψ
t
+
1
2
ω
′′
0
ψ
xx
=0. (13.12.7)
We next derive the nonlinear Schr¨odinger equation from the nonlinear
dispersion relation involving both frequency and amplitude in the most
general form
ω = ω
k, a
2
. (13.12.8)
We first expand ω in a Taylor series about k = k
0
and |a|
2
= 0 in the
form
ω = ω
0
+(k − k
0
)
∂ω
∂k
k=k
0
+
1
2
(k − k
0
)
2
∂
2
ω
∂k
2
k=k
0
+
∂ω
∂ |a|
2
|a|
2
=0
|a|
2
, (13.12.9)
where ω
0
≡ ω (k
0
).
If we now replace , ω − ω
0
by i (∂/∂t)andk − k
0
by −i (∂/∂x), and
assume that the resulting operators act on a, we obtain
i (a
t
+ ω
′
0
a
x
)+
1
2
ω
′′
0
a
xx
+ γ |a|
2
a =0, (13.12.10)
where
ω
′
0
≡ ω
′
(k
0
) ,ω
′′
0
≡ ω
′′
(k
0
) , and γ ≡−
∂ω
∂ |a|
2
|a|
2
=0
is a constant.
Equation (13.12.10) is known as the nonlinear Schr¨odinger (NLS) equa-
tion. If we choose a frame of reference moving with the linear group velocity
ω
′
0
,thatis,x
∗
= x − ω
′
0
t and t
∗
= t, the term involving a
x
will drop out
from (13.12.10), and the amplitude a (x, t) satisfies the normalized NLS
equation, dropping the asterisks,
ia
t
+
1
2
ω
′′
0
a
xx
+ γ |a|
2
a =0. (13.12.11)
The corresponding dispersion relation is given by
ω =
1
2
ω
′′
0
k
2
− γa
2
. (13.12.12)
According to the stability criterion established in Section 13.5, the wave
modulation is stable if γω
′′
0
< 0 and unstable if γω
′′
0
> 0.
To study the solitary wave solution, it is convenient to use the NLS
equation in the standard form
iψ
t
+ ψ
xx
+ γ |ψ|
2
ψ =0, −∞ <x<∞,t≥ 0. (13.12.13)
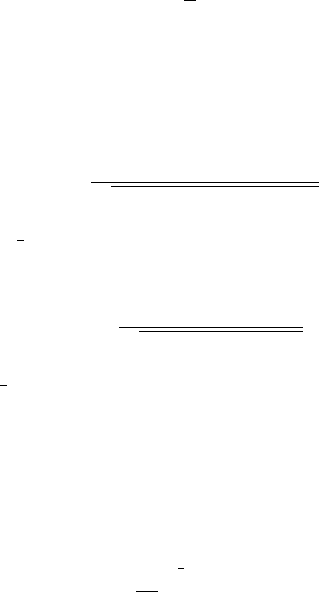
584 13 Nonlinear Partial Differential Equations with Applications
We seek waves of permanent form by assuming the solution
ψ = f (X) e
i(mX−nt)
,X= x − Ut (13.12.14)
for some functions f an d constant wave speed U to be determined, and m,
n are constants.
Substitution of (13.12.14) into (13.12.13) gives
f
′′
+ i (2m − U ) f
′
+
n − m
2
f + γ |f |
2
f =0. (13.12.15)
We eliminate f
′
by setting 2m − U =0,andthen,writen = m
2
−α so
that f can be assumed to be real. Thus, equation (13.12.15) becomes
f
′′
− αf + γf
3
=0. (13.12.16)
Multiplying this equation by 2f
′
and integrating, we find that
f
′2
= A + αf
2
−
γ
2
f
4
≡ F (f ) , (13.12.17)
where F (f) ≡
α
1
− α
2
f
2
β
1
− β
2
f
2
,sothat α = −(α
1
β
2
+ α
2
β
1
),
A = α
1
β
1
, γ = −2(α
2
β
2
), and α
′
s and β
′
s are assumed to be real and
distinct.
Evidently,
X =
f
0
df
(α
1
− α
2
f
2
)(β
1
− β
2
f
2
)
. (13.12.18)
Putting (α
2
/α
1
)
1
2
f = u in this integral, we deduce the following elliptic
integral of the first kind (see Dutta and Debnath (1965)):
σX =
u
0
du
(1 − u
2
)(1− κ
2
u
2
)
, (13.12.19)
where σ =(α
2
β
1
)
1
2
and κ =(α
1
β
2
) / (β
1
α
2
).
Thus, the final solution can be expressed in terms of the Jacobian sn
function
u = sn (σX, κ) ,
or,
f (X)=
α
1
α
2
1
2
sn (σX, κ) . (13.12.20)
In particular, when A =0,α>0, and γ>0, we obtain a solitary wave
solution. In this case, equation (13.12.17) can be rewritten as
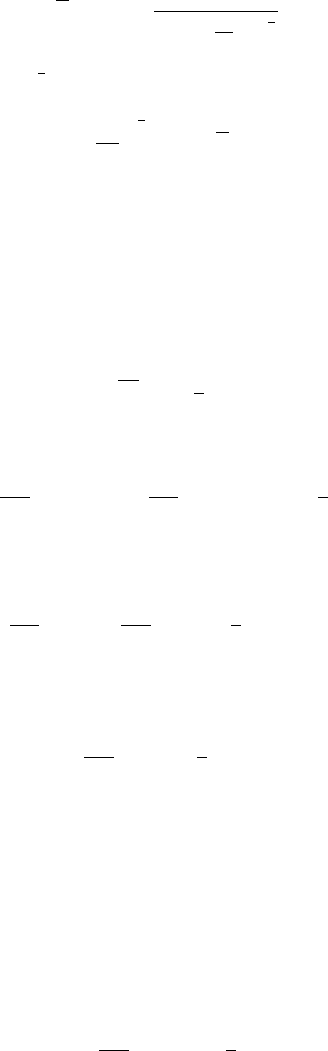
13.12 The Nonlinear Schr¨odinger Equation and Solitary Waves 585
√
αX =
f
0
df
f
1 −
γ
2α
f
2
1
2
. (13.12.21)
Substitution of (γ/2α)
1
2
f = sech θ in this integral gives the exact solution
f (X)=
2α
γ
1
2
sech
√
α (x − Ut)
!
. (13.12.22)
This represents a solitary wave which propagates without change of shape
with constant velocity U. Unlike the solution of the KdV equation, the
amplitude and the velocity of the wave are indep endent parameters. It is
noted that the solitary wave exists only for the unstable case (γ>0). This
means that small modulations of the unstable wavetrain lead to a series of
solitary waves.
The nonlinear dispersion relation for deep water waves is
ω =
gk
1+
1
2
a
2
k
2
. (13.12.23)
Therefore,
ω
′
0
=
ω
0
2k
0
,ω
′′
0
= −
ω
0
4k
2
0
, and γ = −
1
2
ω
0
k
2
0
, (13.12.24)
and the NLS equation for deep water waves is obtained from (13.12.10) in
the form
i
a
t
+
ω
0
2k
0
a
x
−
ω
0
8k
2
0
a
xx
−
1
2
ω
0
k
2
0
|a|
2
a =0. (13.12.25)
The normalized form of this equation in a frame of reference moving with
the linear group velocity ω
′
0
is
ia
t
−
ω
0
8k
2
0
a
xx
=
1
2
ω
0
k
2
0
|a|
2
a. (13.12.26)
Since γω
′′
0
=
ω
2
0
/8
> 0, this equation confirms the instability of deep
water waves. This i s one of the most remarkable recent results in the theory
of water waves.
We next discuss the uniform solution and the solitary wave solution of
(13.12.26). We look for solutions in the form
a (x, t)=A (X)exp
iγ
2
t
,X= x − ω
′
0
t (13.12.27)
and substitute this into equation (13.12.26) to obtain
A
XX
= −
8k
2
0
ω
0
γ
2
A +
1
2
ω
0
k
2
0
A
3
. (13.12.28)
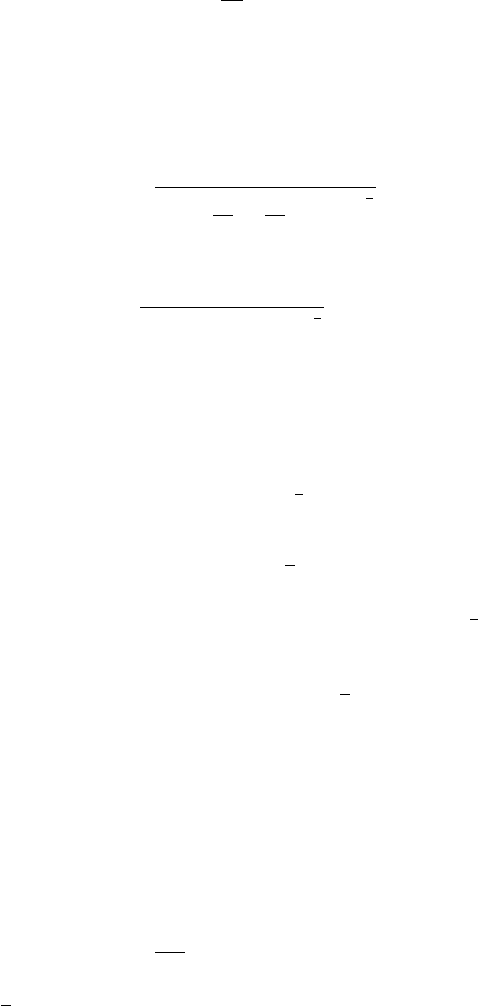
586 13 Nonlinear Partial Differential Equations with Applications
We multiply this equation by 2A
X
and then integrate to find
A
2
X
= −
A
4
0
m
′2
+
8
ω
0
γ
2
k
2
0
A
2
+2k
4
0
A
4
=
A
2
0
− A
2
A
2
− m
′2
A
2
0
, (13.12.29)
where
A
4
0
m
′2
is an integrating constant and 2k
4
0
=1,m
′2
=1− m
2
,and
A
2
0
=4γ
2
/ω
0
k
2
0
m
2
− 2
which relates A
0
, γ,andm.
Finally, we rewrite equation (13.12.29) in the form
A
2
0
dX =
dA
"
1 −
A
2
A
2
0
A
2
A
2
0
− m
′2
#
1
2
, (13.12.30)
or,
A
0
(X − X
0
)=
′
ds
[(1 − s
2
)(s
2
− m
′2
)]
1
2
,s=(A/A
0
) .
This can readily be expressed in terms of the Jacobian dn function (see
Dutta and Debnath (1965))
A = A
0
dn [A
0
(X − X
0
) ,m] , (13.12.31)
where m is the modulus of the dn function.
In the limit m → 0, dn z → 1andγ
2
→−
1
2
ω
0
k
2
0
A
2
0
. Hence, the solution
is
a (x, t)=A (t)=A
0
exp
−
1
2
iω
0
k
2
0
A
2
0
t
. (13.12.32)
On the other hand, when m → 1, dn z → sech z and γ
2
→−
1
4
ω
0
k
2
0
A
2
0
.
Therefore, the solitary wave solution is
a (x, t)=A
0
sech [A
0
(x − ω
′
0
t − X
0
)] exp
−
1
4
ω
0
k
2
0
A
2
0
t
. (13.12.33)
We next use the NLS equation (13.12.26) to discuss the instability of
deep water waves, which is known as the Benjamin and Feir instability.We
consider a perturbation of (13.12.32) and write
a (X, t)=A (t)[1+B (X, t)] , (13.12.34)
where A (t) is the uniform solution given by (13.12.32).
Substituting equation (13.12.34) into (13.12.26) gives
iA
t
(1 + B)+iA (t) B
t
−
ω
0
8k
2
0
A (t) B
XX
=
1
2
ω
0
k
2
0
A
2
0
[(1 + B)+BB
∗
(1 + B)+(B + B
∗
) B +(B + B
∗
)] A,
13.12 The Nonlinear Schr¨odinger Equation and Solitary Waves 587
where B
∗
is the complex conjugate of B.
Neglecting squares of B, it follows that
iB
t
−
ω
0
8k
2
0
B
XX
=
1
2
ω
0
k
2
0
A
2
0
(B + B
∗
) . (13.12.35)
We now seek a solution of the form
B (X, t)=B
1
e
Ωt+iκX
+ B
2
e
Ωt−iκX
, (13.12.36)
where B
1
, B
2
are complex constants, κ is a real wavenumber, and Ω is a
growth rate (possibly complex) to be determined.
Substitution of B into (13.12.35) leads to the pair of coupled equations
iΩ +
ω
0
κ
2
8k
2
0
B
1
−
1
2
ω
0
k
2
0
A
2
0
(B
1
+ B
∗
2
)=0, (13.12.37)
iΩ +
ω
0
κ
2
8k
2
0
B
2
−
1
2
ω
0
k
2
0
A
2
0
(B
∗
1
+ B
2
)=0. (13.12.38)
It is convenient to take the complex conjugate of (13.12.38) so that it as-
sumes the for m
−iΩ +
ω
0
κ
2
8k
2
0
B
∗
2
−
1
2
ω
0
k
2
0
A
2
0
(B
1
+ B
∗
2
)=0. (13.12.39)
The pair of linear homogeneous equations (13.12.37) and (13.12.39) for
B
1
and B
∗
2
admits a nontri vial solution provided
iΩ +
ω
0
κ
2
8k
2
0
−
1
2
ω
0
k
2
0
A
2
0
−
1
2
ω
0
k
2
0
A
2
0
−
1
2
ω
0
k
2
0
A
2
0
iΩ +
ω
0
κ
2
8k
2
0
−
1
2
ω
0
k
2
0
A
2
0
=0,
or
Ω
2
=
ω
2
0
κ
2
8k
2
0
k
2
0
A
2
0
−
κ
2
8k
2
0
. (13.12.40)
The growth rate Ω is purely imaginary or real and positive depending on
whether
κ
2
/k
2
0
> 8k
2
0
A
2
0
or
κ
2
/k
2
0
< 8k
2
0
A
2
0
. The former case corre-
sponds to a wave (an oscillatory solution) for B, and the latter case rep-
resents the Benjamin and Feir instability criterion with 5κ =(κ/k
0
)asthe
non-dimensional wavenumber so that
5κ
2
< 8k
2
0
A
2
0
. (13.12.41)
The range of instability is given by
0 < 5κ<5κ
c
=2
√
2(k
0
A
0
) . (13.12.42)

588 13 Nonlinear Partial Differential Equations with Applications
Since Ω is a function of 5κ, maximum instability occurs at 5κ = 5κ
max
=
2k
0
A
0
with a maximum growth rate given by
(Re Ω)
max
=
1
2
ω
0
k
2
0
A
2
0
. (13.12.43)
To establish the connection with the Benjamin–Feir instability, we have
to find the velocity potential for the fundamental wave mode multiplied by
exp (kz). It turns out that the term proportional to B
1
is the upper side-
band, whereas that proportional to B
2
is the lower sideband. The main con-
clusion of the preceding analysis is that Stokes water waves are definitely
unstable. In 1967, Benjamin and Feir (see Whitham (1976) or Debnath
(2005)) confirmed these remarkable results both theoretically an d experi-
mentally.
Conservation Laws for the NLS Equation
Zakharov and Shabat (1972) proved that equation (13.12.13) has an infinite
number of polynomial conservation laws. Each has the form of an integral,
with respect to x, of a p ol ynomial expression in terms of the function ψ (x, t)
and its derivatives with respect to x. These laws are somewhat similar to
those already proved for the KdV equation. Therefore, the proofs of the
conservation laws are based on similar assumptions used in the context of
the KdV equation.
We prove here three conservation laws for the nonlinear Schr¨odinger
equation (13.12.13):
∞
−∞
|ψ|
2
dx = constant = C
1
, (13.12.44)
∞
−∞
i
ψ
ψ
x
− ψψ
x
dx = constant = C
2
, (13.12.45)
∞
−∞
|ψ
x
|
2
−
1
2
γ |ψ|
4
dx = constant = C
3
, (13.12.46)
where the bar denotes the complex conjugate.
We multiply (13.12.13) by
ψ and its complex conjugate by ψ and sub-
tract the latter from the former to obtain
i
d
dt
ψ
ψ
+
d
dx
ψ
x
ψ − ψ
x
ψ
=0. (13.12.47)
Integration with respect to x in −∞ <x<∞ gives
i
d
dt
∞
−∞
|ψ|
2
dx =0.
This proves result (13.12.44).
13.12 The Nonlinear Schr¨odinger Equation and Solitary Waves 589
We multiply (13.12.13) by
ψ
x
and its complex conjugate by ψ
x
and then,
add them to obtain
i
ψ
t
ψ
x
− ψ
t
ψ
x
+
ψ
xx
ψ
x
+ ψ
xx
ψ
x
+ γ |ψ|
2
ψ ψ
x
+ ψ
x
ψ
x
=0.
(13.12.48)
We differentiate (13.12.13) and its complex conjugate with respect to
x, and multiply the former by
ψ and the latter by ψ andthenaddthem
together. This leads to the result
i
ψ
x
ψ
xt
− ψ ψ
xt
+
ψ
xxx
ψ + ψ
xxx
ψ
+γ
"
ψ
|ψ|
2
ψ
x
+ ψ
|ψ|
2
ψ
x
#
=0. (13.12.49)
If we subtract (13.12.49) from (13.12.48) and then simplify, we have
i
d
dt
ψψ
x
− ψ ψ
x
=
d
dx
ψ
x
ψ
x
+
d
dx
ψψ
xx
+ ψ ψ
xx
−
d
dx
ψ
x
ψ
x
+ γ
d
dx
ψ
ψ
2
=
d
dx
ψψ
xx
+ ψ ψ
xx
+ γ
d
dx
|ψ|
4
.
Integrating this result with respect to x, we obtain
d
dt
∞
−∞
i
ψψ
x
− ψ ψ
x
dx =0.
This proves the second result.
We multiply (13.12.13) by
ψ
t
and its complex conjugate by ψ
t
and add
the resulting equations to derive
ψ
t
ψ
xx
+ ψ
xx
ψ
t
+ γ
ψ
2
ψ ψ
t
+ ψ
2
ψψ
t
=0,
or,
d
dx
ψ
t
ψ
x
+ ψ
t
ψ
x
−
d
dt
ψ
x
ψ
x
+
γ
2
d
dt
ψ
2
ψ
2
=0.
Integrating this with respect to x,wehave
d
dt
∞
−∞
|ψ
x
|
2
−
γ
2
|ψ|
4
dx =
∞
−∞
d
dx
ψ
t
ψ
x
+ ψ
t
ψ
x
dx =0. (13.12.50)
This gives (13.12.46).
The above three conservation integrals have a simple physical meaning.
In fact, the constants of motion C
1
, C
2
and C
3
are related to th e number
of par ticles, the momentum, and th e energy of a system governed by the
nonlinear Schr¨odinger equation.
590 13 Nonlinear Partial Differential Equations with Applications
An analysis of this section reveals several remarkable features of the
nonlinear Schr¨odinger equation. This equation can also be used to investi-
gate instability phenomena in many other physical systems. Like the various
forms of the KdV equation, the NLS equation arises in many physical prob-
lems, including nonlinear water waves an d ocean waves, waves in plasma,
propagation of heat pulses in a solid, self-trapping phenomena in nonlinear
optics, nonlinear waves in a fluid filled viscoelastic tube, and various non-
linear instability phenomena in fluids and plasmas (see Debnat h (2005)).
13.13 The Lax Pair and the Zakharov and Shabat
Scheme
In his 1968 seminal paper, Lax developed an elegant formalism for finding
isospectral potentials as solutions of a nonlinear evolution equation with
all of its integrals. This work deals with some new and fundamental ideas,
deeper results, and their application to the KdV model. This work sub-
sequently paved the way to generalizations of the technique as a method
for solving other nonlinear partial differential equations. Introducing the
Heisenberg picture, Lax develop ed the method of inverse scattering based
upon an abstract formulation of evolution equations and certain properties
of operators on a Hilbert space, some of which are familiar in the context of
quantum mechanics. His formulation has the feature of associating certain
nonlinear evolution equations with linear equations that are analogs of the
Schr¨odinger equation for the KdV equation.
To formulate Lax’s method (1968), we consider two linear operators L
and M. The eigenvalue equation related to the operator L corresponds to
the Schr¨odinger equation for the KdV equation. The general form of this
eigenvalue equation is
Lψ = λψ, (13.13.1)
where ψ is the eigenfunction and λ is the corresponding eigenvalue. The
operator M describ es the change of the eigenvalues with the parameter t,
which usually represents time in a nonlinear evolution equation. The general
form of this evolution equation is
ψ
t
= Mψ. (13.13.2)
Differentiating (13.13.1) with respect to t gives
L
t
ψ + Lψ
t
= λ
t
ψ + λψ
t
. (13.13.3)
We next eliminate ψ
t
from (13.13.3) by using (13.13.2) and obtain
L
t
ψ + LMψ = λ
t
ψ + λMψ = λ
t
ψ + Mλψ = λ
t
ψ + MLψ, (13.13.4)
