Myint Tyn U., Debnath L. Linear Partial Differential Equations for Scientists and Engineers
Подождите немного. Документ загружается.

12.15 Finite Fourier Transforms and Applications 501
Example 12.15.1. Consider the motion of a string of length π due to a force
acting on it. Let the string be fixed at both ends. The motion is thus
governed by
u
tt
= c
2
u
xx
+ f (x, t) , 0 <x<π, t>0,
u (x , 0) = 0,u
t
(x, 0) = 0, 0 <x<π, (12.15.7)
u (0,t)=0,u(π, t)=0,t>0.
Applying the finite Fourier sine transform to the equation of motion with
respect to x gives
F
s
u
tt
− c
2
u
xx
− f (x, t)
!
=0.
Due to its linearity (see Problem 52, 12.18 Exercises), this can be written
in the form
F
s
[u
tt
] − c
2
F
s
[u
xx
]=F
s
[f (x, t)] . (12.15.8)
Let U (n, t) be the finite Fourier sine transform of u (x, t). Then we have
F
s
[u
tt
]=
2
π
π
0
u
tt
sin nx dx
=
d
2
dt
2
2
π
π
0
u (x , t)sinnx dx
=
d
2
U
s
(n, t)
dt
2
.
We also have, from Theorem 12.15.1,
F
s
[u
xx
]=
2n
π
[u (0,t) − (−1)
n
u (π, t)] − n
2
U
s
(n, t) .
Because of the boundary conditions
u (0,t)=u (π, t)=0,
F
s
[u
xx
] becomes
F
s
[u
xx
]=−n
2
U
s
(n, t) .
If we denote the finite Fourier sine transform of f (x, t)by
F
s
(n, t)=
2
π
π
0
f (x, t)sinnx dx,
then, equation (12.15.8) takes the form
d
2
U
s
dt
2
+ n
2
c
2
U
s
= F
s
(n, t) .
This is a second-order ordinary differential equation, the solution of which
is given by

502 12 Integral Transform Methods with Applications
U
s
(n, t)=A cos nct + B sin nct +
1
nc
t
0
F
s
(n, τ )sinnc (t − τ ) dτ.
Applying the initial conditions
F
s
[u (x, 0)] =
2
π
π
0
u (x , 0) sin nx dx = U
s
(n, 0) = 0,
and
F
s
[u
t
(x, 0)] =
d
dt
U
s
(n, 0) = 0,
we have
U
s
(n, t)=
1
nc
t
0
F
s
(n, τ )sinnc (t − τ ) dτ.
Thus, the inverse transform of U
s
(n, t)is
u (x , t)=
∞
n=1
U
s
(n, t)sinnx
=
∞
n=1
1
nc
t
0
F
s
(n, τ )sinnc (t − τ ) dτ
sin nx.
In the case when f (x, t)=h which is a constant, then
F
s
[h]=
2
π
π
0
h sin nx dx =
2h
nπ
[1 − (−1)
n
] .
Now, we evaluate
U
s
(n, t)=
1
nc
t
0
2h
nπ
[1 − (−1)
n
]sinnc (t − τ ) dτ
=
2h
n
3
πc
2
[1 − (−1)
n
](1− cos nct) .
Hence, the solution is given by
u (x , t)=
2h
πc
2
∞
n=1
[1 − (−1)
n
]
n
3
(1 − cos nct)sinnx.
Example 12.15.2. Findthetemperaturedistributioninarodoflengthπ.
The heat is generated in the rod at the rate g (x, t) per unit time. The ends
are insulated. The initial temperature distribution is given by f (x).
The problem is to find the temperature function u (x, t) of the system
u
t
= u
xx
+ g (x, t) , 0 <x<π, t>0,
u (x , 0) = f (x) , 0 ≤ x ≤ π,
u
x
(0,t)=0,u
x
(π, t)=0,t≥ 0.

12.15 Finite Fourier Transforms and Applications 503
Let U
s
(n, t) be the finite Fourier cosine transform of u (x, t). As before,
transformation of the heat equation with respect to x, using the boundary
conditions, yields
dU
s
dt
= −n
2
U
s
+ G
s
(n, t) ,
where
G
s
(n, t)=
2
π
π
0
g (x, t)cosnx dx.
Rewriting this equation, we obtain
d
dt
e
n
2
t
U
s
= G
s
e
n
2
t
.
Thus, the solution is
U
s
(n, t)=
t
0
e
−n
2
(t−τ)
G
s
(n, τ ) dτ + Ae
−n
2
t
.
Transformation of the initial condition gives
U
s
(n, 0) =
2
π
π
0
u (x , 0) cos nx dx =
2
π
π
0
f (x)cosnx dx.
Hence, U
s
(n, t) takes the form
U
s
(n, t)=
t
0
e
−n
2
(t−τ)
G
s
(n, τ ) dτ + U
s
(n, 0) e
−n
2
t
.
The solution u (x, t), therefore, is given by
u (x , t)=
U
s
(0, 0)
2
+
∞
n=1
U
s
(n, t)cosnx.
Example 12.15.3. A rod with diffusion constant κ contains a fuel which
produces neutrons by fission. The ends of the rod are perfectly reflecting. If
the initial neutron distribution is f (x), find the neutron distribution u (x, t)
at any subsequent time t.
The problem is governed by
u
t
= κu
xx
+ bu,
u (x , 0) = f (x) , 0 <x<l, t>0,
u
x
(0,t)=u
x
(l, t)=0.
If U (n, t) is the finit e Fourier cosine transform of u (x, t), then by trans-
forming the equation and using the boundary conditions, we obtain
504 12 Integral Transform Methods with Applications
U
t
+
κn
2
− b
U =0.
The solution of this equation is
U (n, t)=Ce
−
(
κn
2
−b
)
t
where C is a constant. Then applying the initial condition, we obtain
U (n, t)=U (n, 0) e
−
(
κn
2
−b
)
t
,
where
U (n, 0) =
2
l
l
0
f (x)cosnx dx.
Thus, the solution takes the form
u (x , t)=
U (0, 0)
2
+
∞
n=1
U (n, t)cosnx.
If for instance f (x)=x in 0 <x<π, then, U (0, 0) = π,and
U (n, 0) =
2
n
2
π
[(−1)
n
− 1] ,n=1, 2, 3,...
the solution is given by
u (x , t)=
π
2
+
∞
n=1
2
n
2
π
[(−1)
n
− 1] exp
&
−
κn
2
− b
t
'
cos nx.
12.16 Finite Hankel Transforms and Applications
The Fourier–Bessel series representation of a function f (r)definedin0≤
r ≤ a can be stated in the following theorem:
Theorem 12.16.1. If f (r) is defined in 0 ≤ r ≤ a and
F
n
(k
i
)=H
n
{f (r)} =
a
0
rf (r) J
n
(rk
i
) dr, (12.16.1)
then
f (r)=H
−1
n
{F
n
(k
i
)} =
2
a
2
∞
i=1
F
n
(k
i
)
J
n
(rk
i
)
J
2
n+1
(ak
i
)
, (12.16.2)
where k
i
(0 <k
1
<k
2
<...) are the roots of the equation
J
n
(ak
i
)=0.
12.16 Finite Hankel Transforms and Applications 505
The function F
n
(k
i
) defined by (12.16.1) is called the nth-order finite
Hankel transform of f (r), and the inverse Hankel transform is defined by
(12.16.2). In particular, when n = 0, the finite Hankel transform of order
zero and its inverse are defined by t he integral and series respectively
F (k
i
)=H
0
{f (r)} =
a
0
rf (r) J
0
(rk
i
) dr, (12.16.3)
f (r)=H
−1
0
{F (k
i
)} =
2
a
2
∞
i=1
F (k
i
)
J
0
(rk
i
)
J
2
1
(ak
i
)
. (12.16.4)
Example 12.16.1. Find the nth-order finite Hankel transform of f (r)=r
n
.
We have the following result for the Bessel function
a
0
r
n+1
J
n
(k
i
r) dr =
a
n+1
k
i
J
n+1
(ak
i
) ,
so that
H
n
{r
n
} =
a
n+1
k
i
J
n+1
(ak
i
) .
In particular, when n =0
H
0
{1} =
a
k
i
J
1
(ak
i
)
or, equivalently,
1=H
−1
0
a
k
i
J
1
(ak
i
)
=
2
a
∞
i=1
J
0
(rk
i
)
k
i
J
1
(ak
i
)
.
Example 12.16.2. Find H
0
&
a
2
− r
2
'
.
We have by definition
H
0
&
a
2
− r
2
'
=
a
0
a
2
− r
2
rJ
0
(k
i
r) dr
=
4a
k
3
i
J
1
(ak
i
) −
2a
2
k
2
i
J
0
(ak
i
) ,
where k
i
is a root of J
0
(ax) = 0. Hence,
H
0
&
a
2
− r
2
'
=
4a
k
3
i
J
1
(ak
i
) .
We state the following operational properties of the finite Hankel trans-
form:

506 12 Integral Transform Methods with Applications
(i) H
n
df
dr
=
k
i
2n
[(n − 1) H
n+1
{f (r)}−(n +1)H
n−1
{f (r)}] ,
(ii) H
1
df
dr
= −k
i
H
0
{f (r)},
(iii) H
n
1
r
d
dr
{rf
′
(r)}−
n
2
r
2
f (r)
= −ak
i
f (a) J
′
n
(ak
i
) − k
2
i
H
n
{f (r)},
(12.16.5)
(iv) H
0
f
′′
(r)+
1
r
f
′
(r)
= ak
i
f (a) J
1
(ak
i
) − k
2
i
H
0
{f (r)}. (12.16.6)
Example 12.16.3. Find the solution of the axisymmetric heat conduction
equation
u
t
= κ
u
rr
+
1
r
u
r
, 0 <r<a, t>0,
with the boundary and initial conditions
u (r, t)=f (t)onr = a, t ≥ 0,
u (r, 0) = 0, 0 ≤ r ≤ a,
where u (r, t) represents the temperature distribution.
We apply the finite Hankel transform defined by
U (k, t)=H
0
{u (r, t)} =
a
0
rJ
0
(k
i
r) u (r, t) dr
so that the given equation with the boundary condition becomes
dU
dt
+ κk
2
i
U = κak
i
J
1
(ak
i
) f (t) .
The solution of this equation with the tran sformed initial condition is
U (k, t)=aκk
i
J
1
(ak
i
)
t
0
f (τ) e
−κk
2
i
(t−τ)
dτ.
The inverse transformation gives the solution as
u (r, t)=
2κ
a
∞
i=1
k
i
J
0
(rk
i
)
J
1
(ak
i
)
t
0
f (τ) e
−κk
2
i
(t−τ)
dτ. (12.16.7)
In particular, if f (t)=T
0
= constant, th en this solution becomes
u (r, t)=
2T
0
a
∞
i=1
J
0
(rk
i
)
k
i
J
1
(ak
i
)
1 − e
−κk
2
i
t
. (12.16.8)

12.16 Finite Hankel Transforms and Applications 507
In view of Example 12.16.1, result (12.16.8) becomes
u (r, t)=T
0
1 −
2
a
∞
i=1
J
0
(rk
i
)
k
i
J
1
(ak
i
)
e
−κk
2
i
t
. (12.16.9)
This solution consists of the steady-state term, and the transient term which
tends to zero as t →∞. Consequently, the steady-state is attained in the
limit t →∞.
Example 12.16.4. (Unsteady Viscous Flow in a Rotating Cylinder ). The ax-
isymmetric unsteady motion of a viscous fluid in an infinitely long circular
cylinder of radius a is governed by
v
t
= ν
v
rr
+
1
r
v
r
−
v
r
2
, 0 ≤ r ≤ a, t > 0,
where v = v (r, t) is the tangential fluid velocity and ν is the kinematic
viscosity of the fl uid .
The cylinder is at rest until at t = 0+ it is caused to rotate, so that the
boundary and initial conditions are
v (r, t)=aΩf (t) H (t)onr = a,
v (r, t)=0, at t =0 for r<a,
where f (t) is a physically realistic function of t.
We solve the problem by using the joint Laplace an d finite Hankel trans-
forms of order one defined by
V (k
m
,s)=
a
0
rJ
1
(rk
m
) dr
∞
0
e
−st
v (r, t) dt,
where
V (k
m
,s) is the Laplace transform of V (k
m
,t), and k
m
are the roots
of equation J
1
(ak
m
)=0.
Application of the transform yields
s
ν
V (k
m
,s)=−ak
m
V (a, s) J
′
1
(ak
m
) − k
2
m
V (k
m
,s) ,
V (a, s)=aΩf (s) ,
where f (s) is the Laplace transform of f (t).
The solution of this system is
V (k
m
,s)=−
a
2
νk
m
Ω
f (s) J
′
1
(ak
m
)
(s + νk
2
m
)
.
The joint inverse transformation gives
508 12 Integral Transform Methods with Applications
v (r, t)=
2
a
2
∞
m=1
J
1
(rk
m
)
[J
′
1
(ak
m
)]
2
1
2πi
c+i∞
c−i∞
e
st
V (k
m
,s) ds
= −2νΩ
∞
m=1
k
m
J
1
(rk
m
)
J
′
1
(ak
m
)
1
2πi
c+i∞
c−i∞
e
st
f (s)
(s + νk
2
m
)
ds
= −2νΩ
∞
m=1
k
m
J
1
(rk
m
)
J
′
1
(ak
m
)
t
0
f (τ)exp
−νk
2
m
(t − τ)
!
dτ,
by the Convolution Theorem of the Laplace transform.
In particular, when f (t)=cosωt, the velocity field becomes
v (r, t)=−2νΩ
∞
m=1
k
m
J
1
(rk
m
)
J
′
1
(ak
m
)
t
0
cos ωτ exp
−νk
2
m
(t − τ)
!
dτ
=2νΩ
∞
m=1
k
m
J
1
(rk
m
)
J
′
1
(ak
m
)
×
νk
2
m
exp
−νtk
2
m
−
ω sin ωt + νk
2
m
cos ωt
(ω
2
+ ν
2
k
4
m
)
= v
st
(s, t)+v
tr
(r, t) , (12.16.10)
where the steady-state flow field v
st
and the transient flow field v
tr
are
given by
v
st
(r, t)=−2νΩ
∞
m=1
k
m
J
1
(rk
m
)
ω sin ωt + νk
2
m
cos ωt
J
′
1
(ak
m
)(ω
2
+ ν
2
k
4
m
)
, (12.16.11)
v
tr
(r, t)=2ν
2
Ω
∞
m=1
J
1
(rk
m
) k
3
m
e
−νtk
2
m
J
′
1
(ak
m
)(ω
2
+ ν
2
k
4
m
)
. (12.16.12)
Thus, th e solution consists of the steady-state and transient components.
In the limit t →∞, the latter decays to zero, and the ultimate steady-state
is attained and is given by (12.16.11), which has the form
v
st
(r, t)=−2νΩ
∞
m=1
k
m
J
1
(rk
m
) cos (ωt − α)
J
′
1
(ak
m
)(ω
2
+ ν
2
k
4
m
)
1
2
, (12.16.13)
where tan α =
ω/ν k
2
m
. Thus, we see that the steady solution suffers from
a phase change of α + π. The amplitude of the motion remains bounded for
all values of ω.
The frictional couple exerted on the fluid by unit length of the cylinder
of radius r = a is given by
C =
2π
0
[P
rθ
]
r=a
a
2
dθ =2πa
2
[P
rθ
]
r=a
,
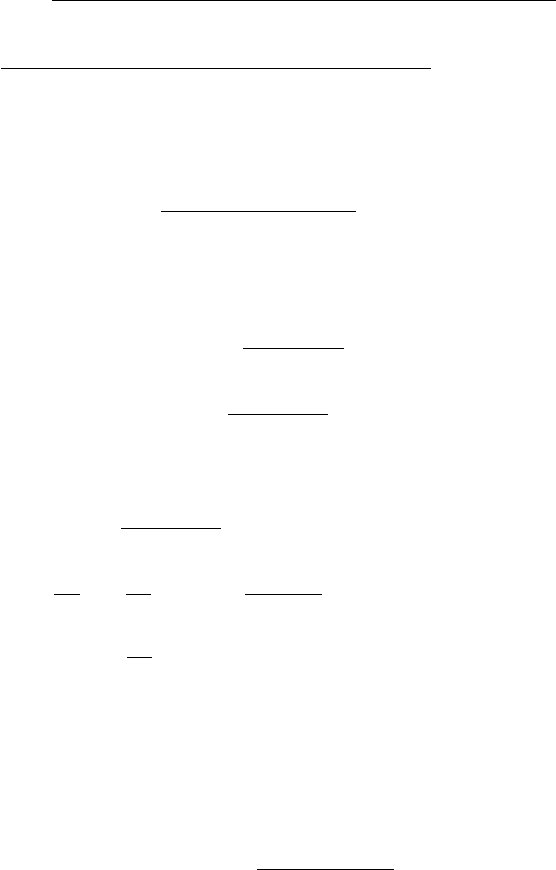
12.16 Finite Hankel Transforms and Applications 509
where P
rθ
= µr(d/dr)(v/r)withµ = νρ calculated from (12.16.10).
Thus,
C =
4πµΩ
−a
∞
m=1
νk
m
J
1
(ak
m
)
νk
2
m
exp
−νtk
2
m
−
νk
2
m
cos ωt + ω sin ωt
!
(ω
2
+ ν
2
k
4
m
) J
′
1
(ak
m
)
+ a
2
∞
m=1
νk
2
m
νk
2
m
exp
−νtk
2
m
−
νk
2
m
cos ωt + ω sin ωt
!
(ω
2
+ ν
2
k
4
m
) J
′
1
(ak
m
)
. (12.16.14)
A particular case corresponding to ω = 0 is of special interest. The
solution assumes the form
v (r, t)=−2Ω
∞
m=1
J
1
(rk
m
)
1 − e
−νtk
2
m
k
m
J
′
1
(ak
m
)
= v
st
+ v
tr
, (12.16.15)
where v
st
and v
tr
represent the steady-state and the transient flow fields
respectively given by
v
st
(r, t)=−2Ω
∞
m=1
J
1
(rk
m
)
k
m
J
′
1
(ak
m
)
, (12.16.16)
v
tr
(r, t)=2Ω
∞
m=1
J
1
(rk
m
)
k
m
J
′
1
(ak
m
)
e
−νtk
2
m
. (12.16.17)
It follows from (12.16.16) that
v
st
(r, t)=2Ω
∞
m=1
J
1
(rk
m
)
k
m
J
2
(ak
m
)
=
2Ω
a
2
∞
m=1
a
2
k
m
J
2
(ak
m
) ·
J
1
(rk
m
)
J
2
2
(ak
m
)
= ΩH
−1
1
a
2
k
m
J
2
(ak
m
)
= Ωr, by Example 12.16.1.
Thus, the steady-state solution has the closed form
v
st
(r, t)=rΩ . (12.16.18)
This represents the rigid body rotation of the fluid inside the cylinder. Thus,
the final form of (12.16.15) is given by
v (r, t)=rΩ − 2Ω
∞
m=1
J
1
(rk
m
) e
−νtk
2
m
k
m
J
2
(ak
m
)
. (12.16.19)
In the limit t →∞, the transients die out and the ultimate steady-state is
attained as the rigid body rotation about the axis of the cylinder.
510 12 Integral Transform Methods with Applications
12.17 Solution of Fractional Partial Differential
Equations
(a) Fractional Diffusion Equation
The fractional diffusion equation is given by
∂
α
u
∂t
α
= κ
∂
2
u
∂x
2
,x∈ R, t > 0, (12.17.1)
with the boundary and initial conditions
u (x , t) → 0as|x|→∞, (12.17.2)
0
D
α−1
t
u (x , t)
!
t=0
= f (x)forx ∈ R, (12.17.3)
where κ is a diffusivity constant and 0 <α≤ 1.
Application of the Fourier transform to (12.17.1) with respect to x and
using the boundary conditions (12.17.2) and (12.17.3) yields
0
D
α
t
˜u (k, t)=−κk
2
˜u, (12.17.4)
0
D
α−1
t
˜u (k, t)
!
t=0
=
˜
f (k) , (12.17.5)
where ˜u (k, t) is the Fourier transform of u (x, t) defined by (12.2.1).
The Laplace transform solution of (12.17.4) and (12.17.5) yields
˜
¯u (k, s)=
˜
f (k)
(s
α
+ κk
2
)
. (12.17.6)
The inverse Laplace transform of (12.17.6) gives
˜u (k, t)=
˜
f (k) t
α−1
E
α,α
−κk
2
t
α
, (12.17.7)
where E
α,β
is the Mittag-Leffler function defined by
E
α,β
(z)=
∞
m=0
z
m
Γ (αm + β)
,α>0,β>0. (12.17.8)
Finally, the inverse Fourier transform leads to th e solution
u (x , t)=
∞
−∞
G (x − ξ, t) f (ξ) dξ, (12.17.9)
where
G (x, t)=
1
π
∞
−∞
t
α−1
E
α,α
−κk
2
t
α
cos kx dk. (12.17.10)
