Myint Tyn U., Debnath L. Linear Partial Differential Equations for Scientists and Engineers

Подождите немного. Документ загружается.

11.7 Method of Images 421

Since the two triangles OPQ and OPQ

′

are similar by virtue of the

hypothesis (OQ)(OQ

′

)=σ

and by possessing a common angle at O,we

have

′

, (11.7.1)

where r

′

= PQ

′

and ρ = OQ.

If σ = 1, equation (11.7.1) becomes



′



=1.

Then, we clearly see that the quantity

2π

log



′



2π

log r −

2π

log r

′

2π

log

(11.7.2)

which vanishes on the boundary σ =1,isharmonicinD except at Q,and

satisﬁes equation (11.3.3). (Note the log r

′

is harmonic everywhere except

at Q

′

, which is outside the domain D.) This suggests that we should choose

the Green’s function

G =

2π

log r −

2π

log r

′

2π

log

. (11.7.3)

Noting that Q

′

is at (1/ρ, θ), the function G in polar coordinates takes the

form

G (ρ, θ, σ, β)=

4π

log

+ ρ

− 2σρ cos (β − θ)

−

4π

log



+ ρ

− 2

cos (β − θ)



2π

log

(11.7.4)

which is the same as G given by (11.5.6).

It is quite interesting to observe the physical interpretation of the

Green’s function (11.7.3) and (11.7.4). The ﬁrst term represents the po-

tential due to a unit line charge at the source point, whereas the second

term represents the potential due to a negative unit charge at the image

point. The third term represents a uniform potential. The sum of these

potentials makes up the total potential ﬁeld.

Example 11.7.1. To illustrate an obvious and simple case, consider the semi-

inﬁnite plane η>0. The problem is to solve

∇

u = h in η>0,

u = f on η =0.

422 11 Green’s Functions and Boundary-Value Problems

The image point should be obvious by inspection. Thus, if we construct

G =

4π

log

(ξ − x)

+(η − y)

−

4π

(ξ − x)

+(η + y)

, (11.7.5)

the condition that G =0onη = 0 is clearly satisﬁed. It is also evident

that G is harmonic in η>0 except at the source p oi nt, and that G satisﬁes

equation (11.3.3).

With G

=[−G

]

η=0

, the solution (11.5.3) is given by

u (x , y)=



∞

−∞

f (ξ) dξ

(ξ − x)

+ y

4π



∞



∞

−∞

log



(ξ − x)

+(η − y)

(ξ − x)

+(η + y)



h (ξ, η) dξ dη. (11.7.6)

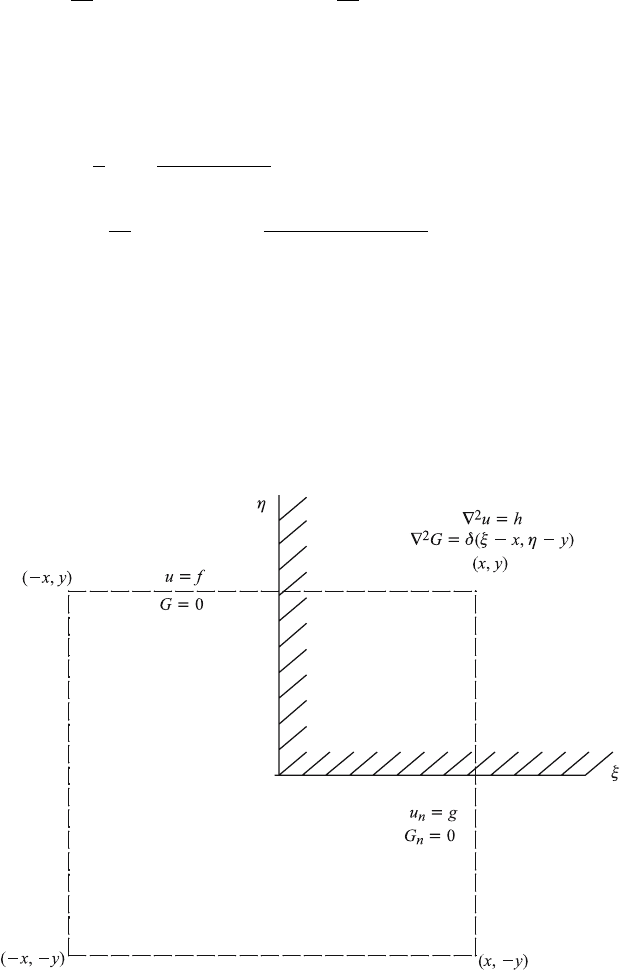
Example 11.7.2. Another example that illustrates the method of i mages

well is the Robin’s problem on the quarter inﬁnite plane, that is,

∇

u = h (ξ, η)inξ>0,η>0,

u = f (η)onξ =0, (11.7.7)

= g (ξ)onη =0.

This illustrated in Figure 11.7.2.

Figure 11.7.2 Images in the Robin problem.

11.8 Method of Eigenfunctions 423

Let (−x, y), (−x, −y), and (x, −y) be the three image points of the

source point (x, y). Then, by inspection, we can immediately construct

Green’s function

G =

4π

log

(ξ − x)

+(η − y)

(ξ − x)

+(η + y)

(ξ + x)

+(η − y)

(ξ + x)

+(η + y)

. (11.7.8)

This function satisﬁes ∇

G = 0 except at the source point, and G =0on

ξ = 0 and G

=0onη =0.

The solution from equation (11.3.3) is thus given by

u (x , y)=



G h dξ dη +



(Gu

− uG

) ds,



∞



∞

G h dξ dη +



∞

g (ξ) G (ξ, 0; x, y) dξ,



∞

f (η) G

(0,η; x, y) dξ.

11.8 Method of Eigenfunctions

In this section, we will apply the method of eigenfunctions, described in

Chapter 10, to obtain the Green’s function.

We consider the boundary-value problem

∇

u = h in D,

(11.8.1)

u = f on B.

For this problem, G must satisfy

∇

G = δ (ξ − x, η − y)inD,

(11.8.2)

G =0 onB,

and hence, the associated eigenvalue problem is

∇

φ + λφ =0 inD,

(11.8.3)

φ =0 onB.

Let φ

be the eigenfunctions and λ

be the corresponding eigenvalues.

We then expand G and δ in terms of the eigenfun ctions φ

. Consequently,

we write

424 11 Green’s Functions and Boundary-Value Problems

G (ξ, η; x, y)=



(x, y) φ

(ξ,η) , (11.8.4)

δ (ξ − x, η − y)=



(x, y) φ

(ξ,η) , (11.8.5)

where

φ





δ (ξ − x, η − y) φ

(ξ,η) dξ dη

(x, y)

φ



(11.8.6)

in which

φ





dξ dη.

Now substitu tin g equations (11.8.4) and (11.8.5) into equation (11.8.2) and

using the relation from equation (11.8.3) that

∇

+ λ

=0,

we obtain

−



(x, y) φ

(ξ,η)=



(x, y) φ

(ξ,η)

φ



Hence,

(x, y)=−

(x, y)

φ



, (11.8.7)

and the Green’s function is therefore given by

G (ξ, η; x, y)=−



(x, y) φ

(ξ,η)

φ



. (11.8.8)

Example 11.8.1. As a part icular example, consider the Dirichlet problem in

a rectangular domain

∇

u = h in D {0 <x<a, 0 <y<b},

u =0 onB.

The eigenfunctions can be obtained explicitly by the method of separa-

tion of variables. We assume a nontrivial solution in the form

u (ξ ,η)=X (ξ) Y (η) .

Substituting this in the following system

11.9 Higher-Dimensional Problems 425

∇

u + λu =0 inD,

u =0 onB,

yields, with α

as separation constant,

′′

+ α

X =0,

′′



λ − α



Y =0.

With the homogeneous boundary conditions X (0) = X (a)=0andY (0) =

Y (b) = 0, functions X and Y are found to be

(ξ)=A

sin



mπξ



(η)=B

sin



nπη



We then have

= π





Thus, we obtain the eigenfunctions

(ξ,η)=sin



mπξ



sin



nπη



Knowing φ

, we compute φ

 and obtain

φ





sin



mπξ



sin



nπη



dξ dη =





We then obtain from equation (11.8.8) the Green’s function

G (ξ, η; x, y)=−

4ab

∞



m=1

∞



n=1

sin



mπ x



sin



nπy



sin



mπ ξ



sin



nπη



+ n

)

11.9 Higher-Dimensional Problems

The Green’s function method can be easily extended for applications in

three and more dimensions. Since most of the problems encountered i n the

physical sciences are in three dimensions, we will illustrate the method with

some examples suitable for practical application.

We ﬁrst extend our deﬁnition of the Green’s function in three dimen-

sions.

The Green’s function for the Dirichlet problem involving the Laplace

operator is the function that satisﬁes

426 11 Green’s Functions and Boundary-Value Problems

(a) ∇

G = δ (x − ξ,y − η, z − ζ)inR, (11.9.1)

G =0 onS. (11.9.2)

(b) G (x, y, z; ξ, η,ζ )=G (ξ, η,ζ ; x, y, z) . (11.9.3)

ε→0



∂G

∂n

ds =1, (11.9.4)

where n is the outward unit normal to the surface

:(x − ξ)

+(y − η)

+(z − ζ)

= ε

Proceeding as in the two-dimensional case, the solution of the Dirich let

problem

∇

u = h in R,

(11.9.5)

u = f on S,

u (x , y, z)=



hGdR+



dS. (11.9.6)

Again we let

G (ξ, η,ζ; x, y, z)=F (ξ, η, ζ ; x, y, z)+g (ξ,η,ζ; x, y, z) ,

where

∇

F = δ (x − ξ,y − η, z − ζ)inR,

and

∇

g =0 inR,

u = −F on S.

Example 11.9.1. We consider a spherical domain with radius a.Wemust

have

∇

F =0

except at the source point. For

r =

(ξ − x)

+(η − y)

+(ζ − z)

> 0

with (x, y, z) as the origin, we have

∇

F =





=0.

11.9 Higher-Dimensional Problems 427

Integration then yields

F = A +

for r>0.

Applying condition (11.9.4) we obtain

lim

ε→0



dS = lim

ε→0



dS =1.

Consequently, B = −(1/4π)andA is arbitrary. If we set t he boundedness

condition at inﬁnity for exterior problems so that A =0,wehave

F = −

4πr

. (11.9.7)

We apply the method of images to obtain the Green’s function. If we

draw a three-dimensional diagram analogous to Figure 11.7.1, we will have

a relation similar to (11.7.1), namely,

′





r, (11.9.8)

where r

′

and ρ are measured in three-dimensional space. Thus, we seek

Green’s function

G =

−1

4πr

a/ρ

4πr

′

(11.9.9)

whichisharmoniceverywhereinr except at the source point, and is zero

on the surface S.

In terms of spherical coordinates

ξ = τ cos ψ sin α, x = ρ cos φ sin θ,

η = τ sin ψ sin α, y = ρ sin φ sin θ,

ζ = τ cos α, z = ρ cos θ,

the Green’s function G canbewrittenintheform

G =

−1

4π (τ

+ ρ

− 2τρcos γ)

4π

+ a

− 2τρcos γ

, (11. 9.10)

where γ is the angle between r and r

′

. Now diﬀerentiating G,wehave



∂G

∂τ



τ=a

− ρ

4πa (a

+ ρ

− 2aρ cos γ)

Thus, the solution of the Dirichlet problem for h =0is

428 11 Green’s Functions and Boundary-Value Problems

u (ρ, θ, φ)=



− ρ



4π



2π



f (α, ψ)sinαdαdψ

+ ρ

− 2aρ cos γ)

, (11.9.11)

where cos γ =cosα cos θ +sinα sin θ cos (ψ − φ). This integral is called the

three-dimensional Poisson integral formula.

For the exterior problem where the ou tward normal is radially inward to-

wards the origin, the solution can be simply obtained by replacing



− ρ





− a



in equation (11.9.11).

Example 11.9.2. An example involving the Helmholtz operator is the three-

dimensional radiation problem

∇

u + κ

u =0, (11.9.12)

lim

r→∞

r (u

+ iκu)=0,

where i =

√

−1; the limit condition is called the radiation condition,andr

is the ﬁeld point distance.

In this case, the Green’s function must satisfy

∇

G + κ

G = δ (ξ − x, η − y, ζ − z) . (11.9.13)

Since the point source solution is dependent only on r, we write the

Helmholtz equation

+ κ

G =0 for r>0.

Note that the source point is taken as the origin. If we write the above

equation in the form

(Gr)

+ κ

(Gr)=0 for r>0 (11.9.14)

then the solution can easily be seen to be

Gr = Ae

iκr

+ Be

−iκr

or, equivalently,

G = A

iκr

+ B

−iκr

. (11.9.15)

In order for G to satisfy the radiation condition

lim

r→∞

r (G

+ iκG)=0,

the constant A =0,andG then takes the form

G = B

−iκr

11.9 Higher-Dimensional Problems 429

To determine B,wehave

lim

ε→0



∂G

∂n

dS = − lim

ε→0



−iκr



+ iκ



dS =1

from which we obtain B = −1/4π, and consequently,

G = −

−iκr

4πr

. (11.9.16)

Note that this reduces to (1/4πr)whenκ =0.

Example 11.9.3. Show that the solution of the Poisson equation

−∇

u = f (x, y, z) , (11.9.17)

u (x , y, z)=



G (r) f (ξ, η, ζ ) dξ dη dζ, (11.9.18)

where the Green’s function G (r)is

G (r)=

4πr

4π

(

(x − ξ)

+(y − η)

+(z − ζ)

)

−

. (11.9.19)

The Green’s function G satisﬁes the equation

−∇

G = δ (x − ξ) δ (y − η) δ (z − ζ) . (11.9.20)

Itisnotedthateverywhereexceptat(x, y, z)=(ξ,η,ζ ), equation (11.9.20)

is a homogeneous equation that can be solved by the method of separation of

variables. However, at the point (ξ, η,ζ ) this equation is no longer homoge-

neous. Usually, this point (ξ,η,ζ ) represents a source point or a source point

singularity in electrostatics or ﬂuid mechanics. In order to solve (11.9.17), it

is necessary to take into account the source point at (ξ, η, ζ ). Without loss

of generality, it is convenient to transform the frame of reference so that

the source point is at the origin. This can be done by the transformation

= x − ξ, y

= y − η,andz

= z − ζ. Consequently, equation (11.9.20)

becomes

∇

G = −δ (x

) δ (y

) δ (z

) , (11.9.21)

where ∇

is the Laplacian in terms of x

, y

,andz

Introducing the spherical polar coordinates

= r sin θ cos φ, y

= r sin θ sin φ, z

= r cos θ,

equation (11.9.21) reduces to the form

430 11 Green’s Functions and Boundary-Value Problems

∇

G = −

δ (r)

4πr

, (11.9.22)

where

∇

G ≡

∂

∂r



∂G

∂r



sin θ

∂

∂θ



sin θ

∂G

∂θ



sin

∂

∂φ

. (11.9.23)

Since the ri ght hand side of (11.9.22) is a function of r alone, and hence,

G must be a function of r alone, we write (11.9.22) with (11.9.23) as

∂

∂r



∂G

∂r



= −

δ (r)

4πr

. (11.9.24)

We assume that G tends to zero as r →∞.

The solution of the corresponding homogeneous equation of (11.9.24) is

G (r)=

+ b, (11.9.25)

where a and b are constants of integration. Since G → 0asr →∞, b =0

and we set a =

4π

. Consequently, th e solution for G is

G (r)=

4πr

. (11.9.26)

This solution can be interpreted as the potential produced by a point charge

at the point (ξ, η, ζ ).

Finally, the solution of (11.9.17) is then given by

u (x , y, z)=−



∞

−∞

G (r) f (ξ, η, ζ ) dξ dη dζ

4π



∞

−∞

(x − ξ)

+(y − η)

+(z − ζ)

−

×f (ξ, η, ζ ) dξ dη dζ. (11.9.27)

Physically, this solution of the Poisson equation represents the potential

u (x , y, z) produced by a charge distribution of volume density f (x, y, z).

11.10 Neumann Problem

We have noted in the chapter on boun dar y-value problems that the Neu-

mann problem requires more attention than Dirichlet’s problem because

an additional condition is necessary for the existence of a solution of the

Neumann problem.

We now consider the Neumann problem