Moran M.J., Shapiro H.N. Fundamentals of Engineering Thermodynamics

Подождите немного. Документ загружается.

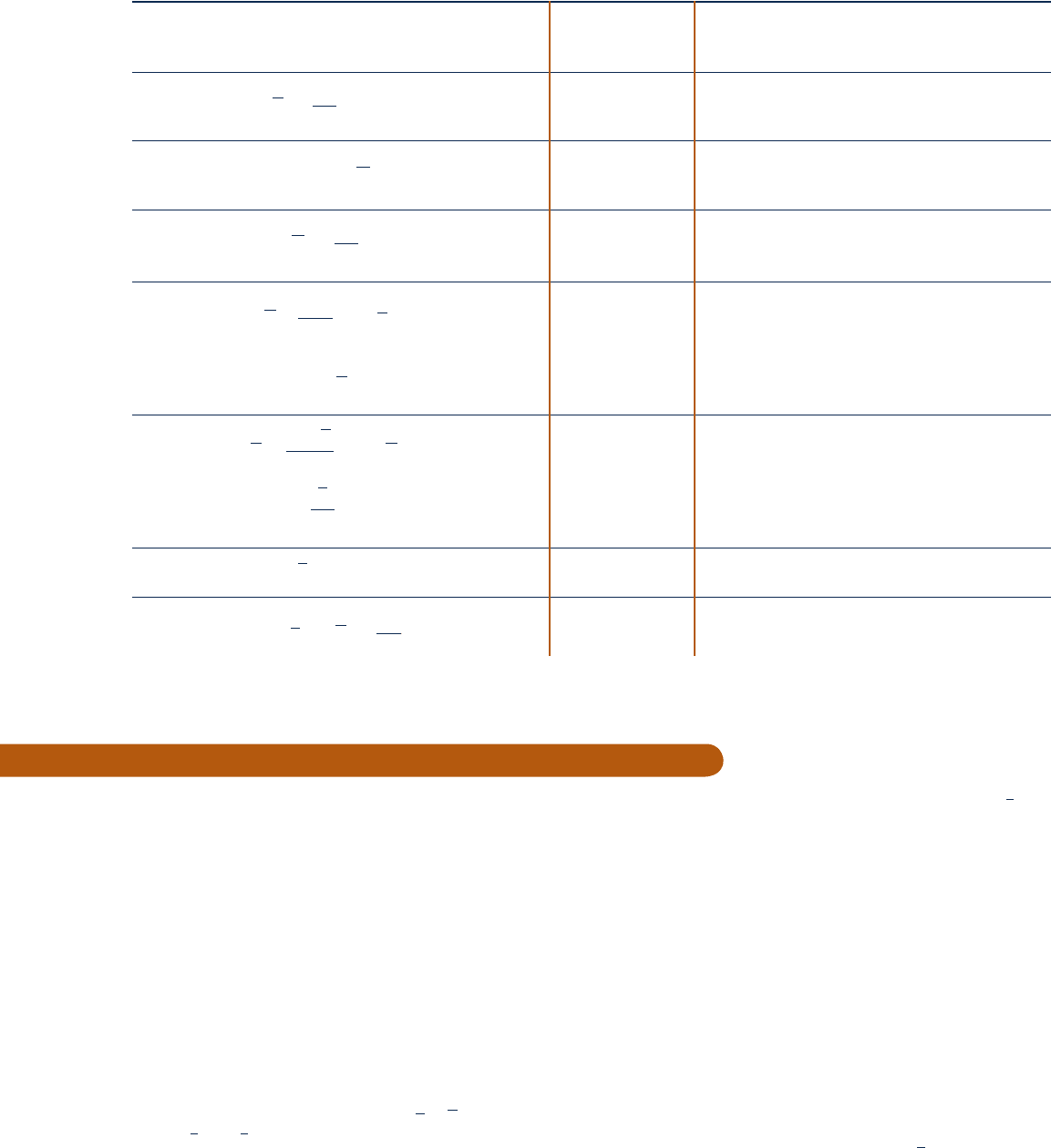
Properties of Multicomponent Mixtures

c, i



c, i

(11.97) p. 676

Kay’s rule for critical temperature and

pressure of mixtures

, n

(11.102) p. 680

Partial molal property

—

and its relation to

extensive property X

X 5

(11.103) p. 680

X as a weighted sum of partial molal

properties

5 G

, n

(11.107) p. 682 Chemical potential of species i in a mixture

RT a

0ln f

5 y

(11.122) p. 685

Expressions for evaluating fugacity of a

single-component system

lim

5 1

(11.123) p. 685

T a

0 l

n f

T, n

5 V

(11.126a) p. 687

Expressions for evaluating fugacity of

mixture component i

lim

5 1

(11.126b) p. 687

(11.134) p. 688 Lewis–Randall rule for ideal solutions

5 g

81RT ln

ref

(11.144) p. 690

Chemical potential of component i in an

ideal gas mixture

c EXERCISES: THINGS ENGINEERS THINK ABOUT

1. What is an advantage of using the Redlich–Kwong equation

of state in the generalized form given by Eq. 11.9 instead of

Eq. 11.7? A disadvantage?

2. To determine the specific volume of superheated water vapor

at a known pressure and temperature, when would you use each

of the following: the steam tables, the generalized compressibility

chart, an equation of state, the ideal gas model?

3. If the function p 5 p(T, y) is an equation of state, is

a property? What are the independent variables of

4. In the expression

, what does the subscript y signify?

5. Explain how a Mollier diagram provides a graphical

representation of the fundamental function h(s, p).

6. How is the Clapeyron equation used?

7. For a gas whose equation of state is

py 5 RT, are the specific

heats

and c

necessarily functions of temperature alone?

8. Referring to the phase diagram for water (Fig. 3.5), explain

why ice melts under the blade of an ice skate.

9. Can you devise a way to determine the specific heat

of a

gas by direct measurement? Indirectly, using other measured

data?

10. For an ideal gas, what is the value of the Joule–Thomson

coefficient?

11. At what states is the entropy departure negligible? The

fugacity coefficient, f/p, closely equal to unity?

12. In Eq. 11.107, what do the subscripts T, p, and n

signify?

What does i denote?

13. How does Eq. 11.108 reduce for a system consisting of a

pure substance? Repeat for an ideal gas mixture.

14. If two different liquids of known volumes are mixed, is the

final volume necessarily equal to the sum of the original

volumes?

15. For a binary solution at temperature T and pressure p, how

would you determine the specific heat

? Repeat for an

ideal solution and for an ideal gas mixture.

Exercises: Things Engineers Think About 693

c11ThermodynamicRelations.indd693 Page 693 6/21/10 9:37:24 PM user-s146 c11ThermodynamicRelations.indd693 Page 693 6/21/10 9:37:24 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

694 Chapter 11

Thermodynamic Relations

c PROBLEMS: DEVELOPING ENGINEERING SKILLS

Using Equations of State

11.1 Owing to safety requirements, the pressure within a

19.3 ft

cylinder should not exceed 52 atm. Check the pressure

within the cylinder if filled with 100 lb of CO

maintained

at 2128F using the

(a) van der Waals equation.

(b) compressibility chart.

11.2 Ten pounds mass of propane have a volume of 2 ft

and

a pressure of 600 lbf/in.

Determine the temperature, in 8R,

using the

(a) van der Waals equation.

(b) compressibility chart.

(d) propane tables.

11.3 The pressure within a 23.3-m

tank should not exceed

105 bar. Check the pressure within the tank if filled with

1000 kg of water vapor maintained at 3608C using the

(a) ideal gas equation of state.

(b) van der Waals equation.

(d) compressibility chart.

(e) steam tables.

11.4 Estimate the pressure of water vapor at a temperature of

5008C and a density of 24 kg/m

using the

(a) steam tables.

(b) compressibility chart.

(d) van der Waals equation.

(e) ideal gas equation of state.

11.5 Methane gas flows through a pipeline with a volumetric

flow rate of 11 ft

/s at a pressure of 183 atm and a

temperature of 568F. Determine the mass flow rate, in lb/s,

using the

(a) ideal gas equation of state.

(b) van der Waals equation.

11.6 Determine the specific volume of water vapor at 20 MPa

and 4008C, in m

/kg, using the

(a) steam tables.

(b) compressibility chart.

(d) van der Waals equation.

(e) ideal gas equation of state.

11.7 A vessel whose volume is 1 m

contains 4 kmol of methane

at 1008C. Owing to safety requirements, the pressure of the

methane should not exceed 12 MPa. Check the pressure

using the

(a) ideal gas equation of state.

(b) Redlich–Kwong equation.

11.8 Methane gas at 100 atm and 2188C is stored in a 10-m

tank. Determine the mass of methane contained in the tank,

in kg, using the

(a) ideal gas equation of state.

(b) van der Waals equation.

11.9 Using the Benedict–Webb–Rubin equation of state,

determine the volume, in m

, occupied by 165 kg of methane

at a pressure of 200 atm and temperature of 400 K. Compare

with the results obtained using the ideal gas equation of state

and the generalized compressibility chart.

11.10 A rigid tank contains 1 kg of oxygen (O

) at p

5 40 bar,

5 180 K. The gas is cooled until the temperature drops

to 150 K. Determine the volume of the tank, in m

, and the

final pressure, in bar, using the

(a) ideal gas equation of state.

(b) Redlich–Kwong equation.

11.11 One pound mass of air initially occupying a volume of

0.4 ft

at a pressure of 1000 lbf/in.

expands in a piston–cylinder

assembly isothermally and without irreversibilities until the

volume is 2 ft

. Using the Redlich–Kwong equation of state,

determine the

(a) temperature, in 8R.

(b) final pressure, in lbf/in.

11.12 Water vapor initially at 2408C, 1 MPa expands in a

piston–cylinder assembly isothermally and without internal

irreversibilities to a final pressure of 0.1 MPa. Evaluate the

work done, in kJ/kg. Use a truncated virial equation of state

with the form

Z 5 1

where B and C are evaluated from steam table data at 2408C

and pressures ranging from 0 to 1 MPa.

11.13 Referring to the virial series, Eqs. 3.30 and 3.31, show

that B

RT, C

C 2 B

11.14 Express Eq. 11.5, the van der Waals equation, in terms

of the compressibility factor Z

(a) as a virial series in y

. [Hint: Expand the

y¿

2 1

term of Eq. 11.5 in a series.]

(b) as a virial series in p

)

and higher in the virial

series of part (b), obtain the following approximate form:

Z 5 1 1 a

(d) Compare the compressibility factors determined from

the equation of part (c) with tabulated compressibility

factors from the literature for 0 , p

, 0.6 and each of

5 1.0, 1.2, 1.4, 1.6, 1.8, 2.0. Comment on the realm of

validity of the approximate form.

c11ThermodynamicRelations.indd694 Page 694 6/22/10 7:20:57 PM user-s146 c11ThermodynamicRelations.indd694 Page 694 6/22/10 7:20:57 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

11.15 The Berthelot equation of state has the form

p 5

y 2 b

(a) Using Eqs. 11.3, show that

a 5



b 5

(b) Express the equation in terms of the compressibility factor

Z, the reduced temperature T

, and the pseudoreduced specific

volume, y

11.16 The Beattie–Bridgeman equation of state can be

expressed as

p 5

RT 11 2 e21y 1 B2

where

5 A

a1 2



B 5 B

a1 2

e 5

and A

, B

, a, b, and c are constants. Express this equation

of state in terms of the reduced pressure, p

, reduced

temperature, T

, pseudoreduced specific volume, y

, and

appropriate dimensionless constants.

11.17 The Dieterici equation of state is

p 5 a

y 2 b

b exp a

RTy

(a) Using Eqs. 11.3, show that

a 5



b 5

(b) Show that the equation of state can be expressed in

terms of compressibility chart variables as

Z 5 a

y¿

2 1

b exp a

y¿

(Hint: Expand the

y¿

2 1

term in a series. Also expand

the exponential term in a series.)

11.18 The Peng–Robinson equation of state has the form

p 5

y 2 b

Using Eqs. 11.3, evaluate the constants a, b, c in terms of the

critical pressure p

, critical temperature T

, and critical

compressibility factor Z

11.19 The p–y–T relation for chlorofluorinated hydrocarbons

can be described by the Carnahan–Starling–DeSantis equation

of state

1 1 b 1

2 b

1 1 b

RT1y 1 b2

where

5 b

4y, a 5 a

exp (a

T 1 a

), and b 5 b

1 b

. For Refrigerants 12 and 13, the required coefficients

for T in K, a in J ? L/(mol)

, and b in L/mol are given in

Table P11.19. Specify which of the two refrigerants would

allow the smaller amount of mass to be stored in a 10-m

vessel at 0.2 MPa, 808C.

Using Relations from Exact Differentials

11.20 The differential of pressure obtained from a certain

equation of state is given by one of the following expressions.

Determine the equation of state.

dp 5

21y 2 b

y 1

1y 2 b2

dp 52

y 2 b

dy 1

y 2 b

11.21 Introducing

int

5 T dS into Eq. 6.8 gives

int

5 dU 1 p dV

Using this expression together with the test for exactness,

demonstrate that

int

is not a property.

11.22 Show that Eq. 11.16 is satisfied by an equation of state

with the form p 5 [RT/(y 2 b)] 1 a.

11.23 For the functions x 5 x(y, w), y 5 y(z, w), z 5 z(x, w),

demonstrate that

5 1

11.24 Using Eq. 11.35, check the consistency of

(a) the steam tables at 2 MPa, 4008C.

(b) the Refrigerant 134a tables at 2 bar, 508C.

11.25 Using Eq. 11.35, check the consistency of

(a) the steam tables at 100 lbf/in.

, 6008F.

(b) the Refrigerant 134a tables at 40 lbf/in.

, 1008F.

11.26 At a pressure of 1 atm, liquid water has a state of maxi-

mum density at about 48C. What can be concluded about

(a) 38C?

(b) 48C?

11.27 A gas enters a compressor operating at steady state and is

compressed isentropically. Does the specific enthalpy increase

or decrease as the gas passes from the inlet to the exit?

11.28 Show that T, p, h, c, and g can each be determined from

a fundamental thermodynamic function of the form u 5

u(s, y).

3 10

R-12 3.52412 22.77230 20.67318 0.15376 21.84195 25.03644

R-13 2.29813 23.41828 21.52430 0.12814 21.84474 210.7951

Table P11.19

Problems: Developing Engineering Skills 695

c11ThermodynamicRelations.indd695 Page 695 6/22/10 7:20:59 PM user-s146 c11ThermodynamicRelations.indd695 Page 695 6/22/10 7:20:59 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

696 Chapter 11

Thermodynamic Relations

11.29 Evaluate p, s, u, h, c

, and c

for a substance for which

the Helmholtz function has the form

c 52RT

y¿

2 cT¿ c1 2

T¿

where y9 and T9 denote specific volume and temperature,

respectively, at a reference state, and c is a constant.

11.30 The Mollier diagram provides a graphical representation

of the fundamental thermodynamic function h 5 h(s, p).

Show that at any state fixed by s and p the properties T, y,

u, c, and g can be evaluated using data obtained from the

diagram.

11.31 Derive the relation c

52T10

Evaluating Ds, Du, and Dh

11.32 Using p–y–T data for saturated ammonia from Table

A-13E, calculate at 208F

(a) h

2 h

(b) u

2 u

2 s

Compare with values obtained from Table A-13E.

11.33 Using p–y–T data for saturated water from the steam

tables, calculate at 508C

(a) h

2 h

(b) u

2 u

2 s

Compare with values obtained from the steam tables.

11.34 Using h

, y

, and p

sat

at 108F from the Refrigerant 134a

tables, estimate the saturation pressure at 208F. Comment on

the accuracy of your estimate.

11.35 Using h

, y

, and p

sat

at 268C from the ammonia tables,

estimate the saturation pressure at 308C. Comment on the

accuracy of your estimate.

11.36 Using triple-point data for water from Table A-6E,

estimate the saturation pressure at 2408F. Compare with the

value listed in Table A-6E.

11.37 At 08C, the specific volumes of saturated solid water (ice)

and saturated liquid water are, respectively, y

5 1.0911 3

/kg and y

5 1.0002 3 10

/kg, and the change in

specific enthalpy on melting is h

5 333.4 kJ/kg. Calculate the

melting temperature of ice at (a) 250 bar, (b) 500 bar. Locate

your answers on a sketch of the p–T diagram for water.

11.38 The line representing the two-phase solid–liquid region

on the phase diagram slopes to the left for substances that

expand on freezing and to the right for substances that

contract on freezing (Sec. 3.2.2). Verify this for the cases of

lead that contracts on freezing and bismuth that expands on

freezing.

11.39 Consider a four-legged chair at rest on an ice rink. The

total mass of the chair and a person sitting on it is 80 kg. If

the ice temperature is 228C, determine the minimum total

area, in cm

, the tips of the chair legs can have before the

ice in contact with the legs would melt. Use data from

Problem 11.37 and let the local acceleration of gravity be

9.8 m/s

11.40 Over a certain temperature interval, the saturation

pressure–temperature curve of a substance is represented by

an equation of the form ln p

sat

5 A 2 B/T, where A and B

are empirically determined constants.

(a) Obtain expressions for h

2 h

and s

2 s

in terms of

p–y–T data and the constant B.

(b) Using the results of part (a), calculate h

2 h

and s

2 s

for water vapor at 258C and compare with steam table data.

11.41 Using data for water from Table A-2, determine the

constants A and B to give the best fit in a least-squares sense

to the saturation pressure in the interval from 20 to 308C by

the equation ln p

sat

5 A 2 B/T. Using this equation, determine

sat

/dT at 258C. Calculate h

at 258C and compare with

the steam table value.

11.42 Over limited intervals of temperature, the saturation

pressure–temperature curve for two-phase liquid–vapor states

can be represented by an equation of the form ln p

sat

A 2 B/T, where A and B are constants. Derive the following

expression relating any three states on such a portion of the

curve:

sat, 3

sat, 1

5 a

sat, 2

sat,1

where t = T

2 T

)/T

2 T

11.43 Use the result of Problem 11.42 to determine

(a) the saturation pressure at 308C using saturation pressure–

temperature data at 20 and 408C from Table A-2. Compare

with the table value for saturation pressure at 308C.

(b) the saturation temperature at 0.006 MPa using saturation

pressure–temperature data at 20 to 408C from Table A-2.

Compare with the saturation temperature at 0.006 MPa

given in Table A-3.

11.44 Complete the following exercises dealing with slopes:

(a) At the triple point of water, evaluate the ratio of the

slope of the vaporization line to the slope of the sublimation

line. Use steam table data to obtain a numerical value for

the ratio.

(b) Consider the superheated vapor region of a temperature–

entropy diagram. Show that the slope of a constant specific

volume line is greater than the slope of a constant pressure

line through the same state.

used in analyzing steam turbines. Obtain an expression for

the slope of a constant-pressure line on such a diagram in

terms of p–y–T data only.

(d) A pressure–enthalpy diagram is often used in the

refrigeration industry. Obtain an expression for the slope of

an isentropic line on such a diagram in terms of p–y–T data

only.

11.45 Using only p–y–T data from the ammonia tables, evaluate

the changes in specific enthalpy and entropy for a process

from 70 lbf/in.

, 408F to 14 lbf/in.

, 408F. Compare with the

table values.

11.46 One kmol of argon at 300 K is initially confined to one

side of a rigid, insulated container divided into equal volumes

of 0.2 m

by a partition. The other side is initially evacuated.

The partition is removed and the argon expands to fill the

c11ThermodynamicRelations.indd696 Page 696 6/22/10 7:21:02 PM user-s146 c11ThermodynamicRelations.indd696 Page 696 6/22/10 7:21:02 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

entire container. Using the van der Waals equation of state,

determine the final temperature of the argon, in K. Repeat

using the ideal gas equation of state.

11.47 Obtain the relationship between c

and c

for a gas that

obeys the equation of state p(y 2 b) 5 RT.

11.48 The p–y–T relation for a certain gas is represented closely

by y = RT/p 1 B 2 A/RT, where R is the gas constant and

A and B are constants. Determine expressions for the changes

in specific enthalpy, internal energy, and entropy, [h(p

, T) 2

h(p

, T)], [u(p

, T) 2 u(p

, T)], and [s(p

, T) 2 s(p

, T)],

respectively.

11.49 Develop expressions for the specific enthalpy, internal

energy, and entropy changes [h(y

, T) 2 h(y

, T)], [u(y

, T) 2

u(y

, T)], [s(y

, T) 2 s(y

, T)], using the

(a) van der Waals equation of state.

(b) Redlich–Kwong equation of state.

11.50 At certain states, the p–y–T data of a gas can be

expressed as Z 5 1 2 Ap/T

, where Z is the compressibility

factor and A is a constant.

(a) Obtain an expression for (0p

0T)

in terms of p, T, A, and

the gas constant R.

(b) Obtain an expression for the change in specific entropy,

[s(p

, T) 2 s (p

, T)].

[h(p

, T) 2 h (p

, T)].

11.51 For a gas whose p–y–T behavior is described by Z 5

1 1 Bp/RT, where B is a function of temperature, derive

expressions for the specific enthalpy, internal energy, and

entropy changes, [h(p

, T) 2 h(p

, T)], [u(p

, T) 2 u(p

, T)],

and [s(p

, T) 2 s(p

, T)].

11.52 For a gas whose p–y–T behavior is described by Z 5

1 1 B/y 1 C/y

, where B and C are functions of temperature,

derive an expression for the specific entropy change, [s(y

, T) 2

s(y

, T)].

Using Other Thermodynamic Relations

11.53 The volume of a 1-kg copper sphere is not allowed to

vary by more than 0.1%. If the pressure exerted on the

sphere is increased from 10 bar while the temperature

remains constant at 300 K, determine the maximum allowed

pressure, in bar. Average values of r, b, and k are 8888 kg/m

49.2 3 10

(K)

, and 0.776 3 10

211

/N respectively.

11.54 The volume of a 1-lb copper sphere is not allowed to

vary by more than 0.1%. If the pressure exerted on the

sphere is increased from 1 atm while the temperature remains

constant at 808F, determine the maximum allowed pressure,

in atm. Average values of r, b, and k are 555 lb/ft

, 2.75 3

(8R)

, and 3.72 3 10

210

/lbf, respectively.

11.55 Develop expressions for the volume expansivity b and

the isothermal compressibility k for

(a) an ideal gas.

(b) a gas whose equation of state is p(y 2 b) 5 RT.

11.56 Derive expressions for the volume expansivity b and the

isothermal compressibility k in terms of T, p, Z, and the first

partial derivatives of Z. For gas states with p

, 3, T

, 2,

determine the sign of k. Discuss.

11.57 Show that the isothermal compressibility k is always

greater than or equal to the isentropic compressibility a.

11.58 Prove that 10b

0p2

5210k

0T2

11.59 For aluminum at 08C, r 5 2700 kg/m

, b 5 71.4 3 10

(K)

, k 5 1.34 3 10

213

/N, and c

5 0.9211 kJ/kg ? K.

Determine the percent error in c

that would result if it were

assumed that c

5 c

11.60 Estimate the temperature rise, in 8C, of mercury, initially

at 08C and 1 bar if its pressure were raised to 1000 bar

isentropically. For mercury at 08C, c

5 28.0 kJ/kmol ? K,

y 5 0.0147 m

kmol, and b 5 17.8 3 10

(K)

11.61 At certain states, the p–y–T data for a particular gas

can be represented as Z 5 1 2 Ap/T

, where Z is the

compressibility factor and A is a constant. Obtain an

expression for the specific heat c

in terms of the gas constant

R, specific heat ratio k, and Z. Verify that your expression

reduces to Eq. 3.47a when Z 5 1.

11.62 For a gas obeying the van der Waals equation of state,

(a) show that

5 0.

(b) develop an expression for c

2 c

, y

) 2 u(T

, y

)] and [s(T

, y

)

2 s(T

, y

)].

(d) complete the Du and Ds evaluations if c

5 a 1 bT,

where a and b are constants.

11.63 If the value of the specific heat c

of air is 0.1965 Btu/

lb ? 8R at T

5 10008F, y

5 36.8ft

/lb, determine the value

of c

at T

5 10008F, y

5 0.0555 ft

/lb. Assume that air obeys

the Berthelot equation of state

p 5

y 2 b

where

a 5



b 5

11.64 Show that the specific heat ratio k can be expressed as

k 5 c

k/(c

k 2 Tyb

). Using this expression together with

data from the steam tables, evaluate k for water vapor at

200 lbf/in.

, 5008F.

11.65 For liquid water at 408C, 1 atm estimate

(a) c

, in kJ/kg ? K.

(b) the velocity of sound, in m/s.

Use Data from Table 11.2, as required.

11.66 Using steam table data, estimate the velocity of sound

in liquid water at (a) 208C, 50 bar, (b) 508F, 1500 lbf/in.

11.67 At a certain location in a wind tunnel, a stream of air is

at 5008F, 1 atm and has a velocity of 2115 ft/s. Determine the

Mach number at this location.

11.68 For a gas obeying the equation of state p(y 2 b) 5 RT,

where b is a positive constant, can the temperature be

reduced in a Joule–Thomson expansion?

Problems: Developing Engineering Skills 697

c11ThermodynamicRelations.indd697 Page 697 6/21/10 9:37:30 PM user-s146 c11ThermodynamicRelations.indd697 Page 697 6/21/10 9:37:30 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

698 Chapter 11

Thermodynamic Relations

11.69 A gas is described by y 5 RT/p 2 A/T 1 B, where A

and B are constants. For the gas

(a) obtain an expression for the temperatures at the Joule–

Thomson inversion states.

(b) obtain an expression for c

2 c

11.70 Determine the maximum Joule–Thomson inversion

temperature in terms of the critical temperature T

predicted

by the

(a) van der Waals equation.

(b) Redlich–Kwong equation.

11.71 Derive an equation for the Joule–Thomson coefficient

as a function of T and y for a gas that obeys the van der

Waals equation of state and whose specific heat c

is given

by c

5 A 1 BT 1 CT

, where A, B, C are constants.

Evaluate the temperatures at the inversion states in terms of

R, y, and the van der Waals constants a and b.

11.72 Show that Eq. 11.77 can be written as

01y

(a) Using this result, obtain an expression for the Joule–

Thomson coefficient for a gas obeying the equation of state

y 5

where A is a constant.

(b) Using the result of part (a), determine c

, in kJ/kg ? K,

for CO

at 400 K, 1 atm, where m

5 0.57 K/atm. For CO

A 5 2.78 3 10

? K

/kg ? N.

Developing Property Data

11.73 If the specific heat c

of a gas obeying the van der Waals

equation is given at a particular pressure, p9, by c

5 A 1

BT, where A and B are constants, develop an expression for

the change in specific entropy between any two states 1 and

2: [s(T

, p

) 2 s(T

, p

)].

11.74 For air, write a computer program that evaluates the

change in specific enthalpy from a state where the temperature

is 258C and the pressure is 1 atm to a state where the

temperature is T and the pressure is p. Use the van der Waals

equation of state and account for the variation of the ideal

gas specific heat as in Table A-21.

11.75 Using the Redlich–Kwong equation of state, determine

the changes in specific enthalpy, in kJ/kmol, and entropy, in

kJ/kmol ? K, for ethylene between 400 K, 1 bar and 400 K,

100 bar.

11.76 Using the Benedict–Webb–Rubin equation of state

together with a specific heat relation from Table A-21,

determine the change in specific enthalpy, in kJ/kmol, for

methane between 300 K, 1 atm and 400 K, 200 atm.

11.77 A certain pure, simple compressible substance has the

following property relations. The p–y–T relationship in the

vapor phase is

y 5

where y is in ft

/lb, T is in 8R, p is in lbf/ft

, R 5 50 ft ? lbf/

lb ? 8R, and B 5 100 ft

? (8R)

/lb ? lbf. The saturation

pressure, in lbf/ft

, is described by

ln p

sat

5 12 2

2400

The Joule–Thomson coefficient at 10 lbf/in.

, 2008F is

0.0048R ? ft

/lbf. The ideal gas specific heat c

is constant

over the temperature range 0 to 3008F.

(a) Complete the accompanying table of property values

T p v

08F 0.03 0 0.000

1008F 0.03

for p in lbf/in.

, y in ft

/lb, h in Btu/lb, and s in Btu/lb ? 8R.

(b) Evaluate y, h, s at the state fixed by 15 lbf/in.

, 3008F.

11.78 In Table A-2, at temperatures up to 508C, the values of

and h

differ in most cases by 0.01 kJ/kg. Yet at each of

these temperatures the product p

sat

is small enough to be

neglected and the table values of u

and h

should be the

same. In a memorandum, provide a plausible explanation.

Using Enthalpy and Entropy Departures

11.79 Beginning with Eq. 11.90, derive Eq. 11.91.

11.80 Derive an expression giving

(a) the internal energy of a substance relative to that of its

ideal gas model at the same temperature: [u(T, y) 2 u*(T)].

(b) the entropy of a substance relative to that of its ideal gas

model at the same temperature and specific volume: [s(T, y) 2

s*(T, y)].

11.81 Derive expressions for the enthalpy and entropy

departures using an equation of state with the form Z 5 1 1

, where B is a function of the reduced temperature, T

11.82 The following expression for the enthalpy departure is

convenient for use with equations of state that are explicit

in pressure:

1T22 h1T, y2

5 T

c1 2 Z 2

cT a

2 p ddy d

(a) Derive this expression.

(b) Using the given expression, evaluate the enthalpy

departure for a gas obeying the Redlich–Kwong equation of

state.

specific enthalpy, in kJ/kmol, for CO

undergoing an isothermal

process at 300 K from 50 to 20 bar.

11.83 Using the equation of state of Problem 11.14 (c), evaluate

y and c

for water vapor at 5508C, 20 MPa and compare with

data from Table A-4 and Fig. 3.9, respectively. Discuss.

11.84 Ethylene at 678C, 10 bar enters a compressor operating

at steady state and is compressed isothermally without

internal irreversibilities to 100 bar. Kinetic and potential

energy changes are negligible. Evaluate in kJ per kg of

ethylene flowing through the compressor

(a) the work required.

(b) the heat transfer.

c11ThermodynamicRelations.indd698 Page 698 6/21/10 9:37:32 PM user-s146 c11ThermodynamicRelations.indd698 Page 698 6/21/10 9:37:32 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

11.85 Methane at 278C, 10 MPa enters a turbine operating at

steady state, expands adiabatically through a 5 : 1 pressure

ratio, and exits at 2488C. Kinetic and potential energy effects

are negligible. If

5 35 kJ

kmol ? K, determine the work

developed per kg of methane flowing through the turbine.

Compare with the value obtained using the ideal gas

model.

11.86 Nitrogen (N

) enters a compressor operating at steady

state at 1.5 MPa, 300 K and exits at 8 MPa, 500 K. If the

work input is 240 kJ per kg of nitrogen flowing, determine

the heat transfer, in kJ per kg of nitrogen flowing. Ignore

kinetic and potential energy effects.

11.87 Oxygen (O

) enters a control volume operating at steady

state with a mass flow rate of 9 kg/min at 100 bar, 287 K and

is compressed adiabatically to 150 bar, 400 K. Determine the

power required, in kW, and the rate of entropy production, in

kW/K. Ignore kinetic and potential energy effects.

11.88 Argon gas enters a turbine operating at steady state at 100

bar, 325 K and expands adiabatically to 40 bar, 235 K with no

significant changes in kinetic or potential energy. Determine

(a) the work developed, in kJ per kg of argon flowing

through the turbine.

(b) the amount of entropy produced, in kJ/K per kg of argon

flowing.

11.89 Oxygen (O

) undergoes a throttling process from 100

bar, 300 K to 20 bar. Determine the temperature after

throttling, in K, and compare with the value obtained using

the ideal gas model.

11.90 Water vapor enters a turbine operating at steady state at

30 MPa, 6008C and expands adiabatically to 6 MPa with no

significant change in kinetic or potential energy. If the isentropic

turbine efficiency is 80%, determine the work developed, in

kJ per kg of steam flowing, using the generalized property

charts. Compare with the result obtained using steam table

data. Discuss.

11.91 Oxygen (O

) enters a nozzle operating at steady state at

60 bar, 300 K, 1 m/s and expands isentropically to 30 bar.

Determine the velocity at the nozzle exit, in m/s.

11.92 A quantity of nitrogen gas in a piston–cylinder assembly

undergoes a process at a constant pressure of 80 bar from

220 to 300 K. Determine the work and heat transfer for the

process, each in kJ per kmol of nitrogen.

11.93 A closed, rigid, insulated vessel having a volume of

0.142 m

contains oxygen (O

) initially at 100 bar, 78C. The

oxygen is stirred by a paddle wheel until the pressure

becomes 150 bar. Determine the

(a) final temperature, in 8C.

(b) work, in kJ.

Let T

5 78C.

Evaluating p–y–T for Gas Mixtures

11.94 A preliminary design calls for a 1 kmol mixture of CO

and C

(ethane) to occupy a volume of 0.15 m

at a

temperature of 400 K. The mole fraction of CO

is 0.3.

Owing to safety requirements, the pressure should not

exceed 180 bar. Check the pressure using

(a) the ideal gas equation of state.

(b) Kay’s rule together with the generalized compressibility

chart.

compressibility chart.

Compare and discuss these results.

11.95 A gaseous mixture with a molar composition of 60% CO

and 40% H

enters a turbine operating at steady state at

3008F, 2000 lbf/in.

and exits at 2128F, 1 atm with a volumetric

flow rate of 20,000 ft

/min. Estimate the volumetric flow rate

at the turbine inlet, in ft

/min, using Kay’s rule. What value

would result from using the ideal gas model? Discuss.

11.96 A 0.1-m

cylinder contains a gaseous mixture with a molar

composition of 97% CO and 3% CO

initially at 138 bar. Due

to a leak, the pressure of the mixture drops to 129 bar while the

temperature remains constant at 308C. Using Kay’s rule, estimate

the amount of mixture, in kmol, that leaks from the cylinder.

11.97 A gaseous mixture consisting of 0.75 kmol of hydrogen

) and 0.25 kmol of nitrogen (N

) occupies 0.085 m

258C. Estimate the pressure, in bar, using

(a) the ideal gas equation of state.

(b) Kay’s rule together with the generalized compressibility

chart.

for the constants a and b.

(d) the rule of additive pressure together with the generalized

compressibility chart.

11.98 A gaseous mixture of 0.5 lbmol of methane and 0.5 lbmol

of propane occupies a volume of 7.65 ft

at a temperature of

1948F. Estimate the pressure using the following procedures

and compare each estimate with the measured value of

pressure, 50 atm:

(a) the ideal gas equation of state.

(b) Kay’s rule together with the generalized compressibility

chart.

for the constants a and b.

(d) the rule of additive pressures together with the van der

Waals equation.

(e) the rule of additive pressures together with the generalized

compressibility chart.

(f) the rule of additive volumes together with the van der

Waals equation.

11.99 One lbmol of a gaseous mixture occupies a volume of

1.78 ft

at 2128F. The mixture consists of 69.5% carbon

dioxide and 30.5% ethylene (C

) on a molar basis. Estimate

the mixture pressure, in atm, using

(a) the ideal gas equation of state.

(b) Kay’s rule together with the generalized compressibility

chart.

compressibility chart.

(d) the van der Waals equation together with mixture values

for the constants a and b.

Problems: Developing Engineering Skills 699

c11ThermodynamicRelations.indd699 Page 699 6/22/10 7:21:05 PM user-s146 c11ThermodynamicRelations.indd699 Page 699 6/22/10 7:21:05 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

700 Chapter 11

Thermodynamic Relations

11.100 Air having an approximate molar composition of 79%

and 21% O

fills a 0.36-m

vessel. The mass of mixture is

100 kg. The measured pressure and temperature are 101 bar

and 180 K, respectively. Compare the measured pressure

with the pressure predicted using

(a) the ideal gas equation of state.

(b) Kay’s rule.

equation.

(d) the additive volume rule with the Redlich–Kwong

equation.

11.101 A gaseous mixture consisting of 50% argon and 50%

nitrogen (molar basis) is contained in a closed tank at 20 atm,

21408F. Estimate the specific volume, in ft

/lb, using

(a) the ideal gas equation of state.

(b) Kay’s rule together with the generalized compressibility

chart.

and b.

(d) the additive volume rule together with the generalized

compressibility chart.

11.102 Using the Carnahan–Starling–DeSantis equation of

state introduced in Problem 11.19, together with the following

expressions for the mixture values of a and b:

a 5 y

1 2y

1 2 f

1 y

b 5 y

1 y

where f

is an empirical interaction parameter, determine

the pressure, in kPa, at y = 0.005 m

/kg, T 5 1808C for a

mixture of Refrigerants 12 and 13, in which Refrigerant 12

is 40% by mass. For a mixture of Refrigerants 12 and 13,

5 0.035.

11.103 A rigid vessel initially contains carbon dioxide gas at

328C and pressure p. Ethylene gas is allowed to flow into the

tank until a mixture consisting of 20% carbon dioxide and

80% ethylene (molar basis) exists within the tank at a

temperature of 438C and a pressure of 110 bar. Determine

the pressure p, in bar, using Kay’s rule together with the

generalized compressibility chart.

11.104 Two tanks having equal volumes are connected by a

valve. One tank contains carbon dioxide gas at 1008F and

pressure p. The other tank contains ethylene gas at 1008F

and 1480 lbf/in.

The valve is opened and the gases mix,

eventually attaining equilibrium at 1008F and pressure p9

with a composition of 20% carbon dioxide and 80% ethylene

(molar basis). Using Kay’s rule and the generalized

compressibility chart, determine in lbf/in.

(a) the initial pressure of the carbon dioxide, p.

(b) the final pressure of the mixture, p9.

Analyzing Multicomponent Systems

11.105 A binary solution at 258C consists of 59 kg of ethyl

alcohol (C

OH) and 41 kg of water. The respective partial

molal volumes are 0.0573 and 0.0172 m

/kmol. Determine the

total volume, in m

. Compare with the volume calculated using

the molar specific volumes of the pure components, each a

liquid at 258C, in the place of the partial molal volumes.

11.106 The following data are for a binary solution of ethane

) and pentane (C

) at a certain temperature and

pressure:

mole fraction of 0.2 0.3 0.4 0.5 0.6 0.7 0.8

ethane

volume (in m

) per 0.119 0.116 0.112 0.109 0.107 0.107 0.11

kmol of solution

Estimate

(a) the specific volumes of pure ethane and pure pentane,

each in m

/kmol.

(b) the partial molal volumes of ethane and pentane for an

equimolar solution, each in m

/kmol.

11.107 The following data are for a binary mixture of carbon

dioxide and methane at a certain temperature and pressure:

mole fraction of 0.000 0.204 0.406 0.606 0.847 1.000

methane

volume (in ft

) per 1.506 3.011 3.540 3.892 4.149 4.277

lbmol of mixture

Estimate

(a) the specific volumes of pure carbon dioxide and pure

methane, each in ft

/lbmol.

(b) the partial molal volumes of carbon dioxide and methane

for an equimolar mixture, each in ft

/lbmol.

11.108 Using p–y–T data from the steam tables, determine the

fugacity of water as a saturated vapor at (a) 2808C, (b) 5008F.

Compare with the values obtained from the generalized

fugacity chart.

11.109 Determine the fugacity, in atm, for

(a) butane at 555 K, 150 bar.

(b) methane at 1208F, 800 lbf/in.

11.110 Using the equation of state of Problem 11.14 (c),

evaluate the fugacity of ammonia at 750 K, 100 atm and

compare with the value obtained from Fig. A-6.

11.111 Using tabulated compressibility factor data from the

literature, evaluate f/p at T

5 1.40 and p

5 2.0. Compare

with the value obtained from Fig. A-6.

11.112 Consider the truncated virial expansion

Z 5 1 1 B

1 C

1 D

(a) Using tabulated compressibility factor data from the

literature, evaluate the coefficients B

, and D

for 0 , p

, 1.0

and each of T

5 1.0, 1.2, 1.4, 1.6, 1.8, 2.0.

(b) Obtain an expression for ln (f/p) in terms of T

and p

Using the coefficients of part (a), evaluate f/p at selected

states and compare with tabulated values from the literature.

11.113 Derive the following approximation for the fugacity of

a liquid at temperature T and pressure p:

f1T, p2< f

sat

1T2exp e

1T2

3p 2 p

sat

1T24f

where

sat

is the fugacity of the saturated liquid at temperature

T. For what range of pressures might the approximation

T, p

< f

sat

apply?

c11ThermodynamicRelations.indd700 Page 700 6/21/10 9:37:34 PM user-s146 c11ThermodynamicRelations.indd700 Page 700 6/21/10 9:37:34 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

11.114 Beginning with Eq. 11.122,

(a) evaluate ln f for a gas obeying the Redlich–Kwong

equation of state.

(b) Using the result of part (a), evaluate the fugacity, in bar,

for Refrigerant 134a at 908C, 10 bar. Compare with the

fugacity value obtained from the generalized fugacity chart.

11.115 Consider a one-inlet, one-exit control volume at steady

state through which the flow is internally reversible and

isothermal. Show that the work per unit of mass flowing can

be expressed in terms of the fugacity f as

int

rev

52RT lna

2 V

1 g1z

2 z

11.116 Methane expands isothermally and without

irreversibilities through a turbine operating at steady state,

entering at 60 atm, 778F and exiting at 1 atm. Using data

from the generalized fugacity chart, determine the work

developed, in Btu per lb of methane flowing. Ignore kinetic

and potential energy effects.

11.117 Propane (C

) enters a turbine operating at steady

state at 100 bar, 400 K and expands isothermally without

irreversibilities to 10 bar. There are no significant changes in

kinetic or potential energy. Using data from the generalized

fugacity chart, determine the power developed, in kW, for a

mass flow rate of 50 kg/min.

11.118 Ethane (C

) is compressed isothermally without

irreversibilities at a temperature of 320 K from 5 to 40 bar.

Using data from the generalized fugacity and enthalpy departure

charts, determine the work of compression and the heat transfer,

each in kJ per kg of ethane flowing. Assume steady-state

operation and neglect kinetic and potential energy effects.

11.119 Methane enters a turbine operating at steady state at

100 bar, 275 K and expands isothermally without irreversibilities

to 15 bar. There are no significant changes in kinetic or

potential energy. Using data from the generalized fugacity

and enthalpy departure charts, determine the power

developed and heat transfer, each in kW, for a mass flow

rate of 0.5 kg/s.

11.120 Methane flows isothermally and without irreversibilities

through a horizontal pipe operating at steady state, entering

at 50 bar, 300 K, 10 m/s and exiting at 40 bar. Using data

from the generalized fugacity chart, determine the velocity

at the exit, in m/s.

11.121 Determine the fugacity, in atm, for pure ethane at 310 K,

20.4 atm and as a component with a mole fraction of 0.35 in

an ideal solution at the same temperature and pressure.

11.122 Denoting the solvent and solute in a dilute binary liquid

solution at temperature T and pressure p by the subscripts

1 and 2, respectively, show that if the fugacity of the solute

is proportional to its mole fraction in the solution:

5 ky

where k is a constant (Henry’s rule), then the fugacity of the

solvent is

5 y

, where y

is the solvent mole fraction and

is the fugacity of pure 1 at T, p.

11.123 A tank contains 310 kg of a gaseous mixture of 70%

ethane and 30% nitrogen (molar basis) at 311 K and 170

atm. Determine the volume of the tank, in m

, using data

from the generalized compressibility chart together with (a)

Kay’s rule, (b) the ideal solution model. Compare with the

measured tank volume of 1 m

11.124 A tank contains a mixture of 75% argon and 25% ethylene

on a molar basis at 778F, 81.42 atm. For 157 lb of mixture,

estimate the tank volume, in ft

, using

(a) the ideal gas equation of state.

(b) Kay’s rule together with data from the generalized

compressibility chart.

generalized compressibility chart.

11.125 A tank contains a mixture of 70% ethane and 30%

nitrogen (N

) on a molar basis at 400 K, 200 atm. For 2130

kg of mixture, estimate the tank volume, in m

, using

(a) the ideal gas equation of state.

(b) Kay’s rule together with data from the generalized

compressibility chart.

generalized compressibility chart.

11.126 An equimolar mixture of O

and N

enters a compressor

operating at steady state at 10 bar, 220 K with a mass flow rate

of 1 kg/s. The mixture exits at 60 bar, 400 K with no significant

change in kinetic or potential energy. Stray heat transfer from

the compressor can be ignored. Determine for the compressor

(a) the power required, in kW.

(b) the rate of entropy production, in kW/K.

Assume the mixture is modeled as an ideal solution. For the

pure components:

10 bar, 220 K 60 bar, 400 K

h (kJ/kg) s (kJ/kg ? K) h (kJ/kg) s (kJ/kg ? K)

Oxygen 195.6 5.521 358.2 5.601

Nitrogen 224.1 5.826 409.8 5.911

11.127 A gaseous mixture with a molar analysis of 70% CH

and 30% N

, enters a compressor operating at steady state at

10 bar, 250 K and a molar flow rate of 6 kmol/h. The mixture

exits the compressor at 100 bar. During compression, the

temperature of the mixture departs from 250 K by no more

than 0.1 K. The power required by the compressor is reported

to be 6 kW. Can this value be correct? Explain. Ignore kinetic

and potential energy effects. Assume the mixture is modeled

as an ideal solution. For the pure components at 250 K:

h (kJ/kg) s (kJ/kg ? K)

10 bar 100 bar 10 bar 100 bar

Methane 506.0 358.6 10.003 8.3716

Nitrogen 256.18 229.68 5.962 5.188

11.128 The departure of a binary solution from ideal solution

behavior is gauged by the activity coefficient, g

5 a

, where a

is the activity of component i and y

is its mole fraction in the

solution (i 5 1, 2). Introducing Eq. 11.140, the activity coefficient

can be expressed alternatively as g

5 f

f 8

. Using this

expression together with the Gibbs–Duhem equation, derive

the following relation among the activity coefficients and the

mole fractions for a solution at temperature T and pressure p:

0 ln g

p, T

5 ay

0 ln

p, T

How might this expression be used?

Problems: Developing Engineering Skills 701

c11ThermodynamicRelations.indd701 Page 701 6/21/10 9:37:35 PM user-s146 c11ThermodynamicRelations.indd701 Page 701 6/21/10 9:37:35 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New

702 Chapter 11

Thermodynamic Relations

c DESIGN & OPEN-ENDED PROBLEMS: EXPLORING ENGINEERING PRACTICE

11.1D Design a laboratory flask for containing up to 10 kmol

of mercury vapor at pressures up to 3 MPa, and temperatures

from 900 to 1000 K. Consider the health and safety of the

technicians who would be working with such a mercury

vapor-filled container. Use a p-y-T relation for mercury vapor

obtained from the literature, including appropriate property

software. Write a report including at least three references.

11.2D The p–h diagram (Sec. 10.2.4) used in the refrigeration

engineering field has specific enthalpy and the natural

logarithm of pressure as coordinates. Inspection of such a

diagram suggests that in portions of the vapor region constant-

entropy lines are nearly linear, and thus the relation between h,

ln p and s might be expressible there as

h1s, p25 1A s 1 B2 ln p 1 1C s 1 D2

Investigate the viability of this expression for pressure

ranging up to 10 bar, using data for Refrigerant 134a.

Summarize your conclusions in a memorandum.

11.3D Compressed natural gas (CNG) is being used as a fuel to

replace gasoline for automobile engines. Aluminum cylinders

wrapped in a fibrous composite can provide lightweight,

economical, and safe on-board storage. The storage vessels

should hold enough CNG for 100 to 125 miles of urban travel,

at storage pressures up to 3000 lbf/in.

, and with a maximum

total mass of 150 lb. Adhering to applicable U.S. Department

of Transportation standards, specify both the size and number

of cylinders that would meet the above design constraints.

11.4D A portable refrigeration machine requiring no external

power supply and using carbon dioxide at its triple point is

described in U.S. Patent No. 4,096,707. Estimate the cost of

the initial carbon dioxide charge required by such a machine

to maintain a 6 ft by 8 ft by 15 ft cargo container at 358F for

up to 24 hours, if the container is fabricated from sheet metal

covered with a 1-in. layer of polystyrene. Would you

recommend the use of such a refrigeration machine? Report

your findings in a PowerPoint presentation.

11.5D A power plant located at a river’s mouth where

freshwater river currents meet saltwater ocean tides can

generate electricity by exploiting the difference in composition

of the freshwater and saltwater. The technology for generating

power is called reverse electrodialysis. While only small-scale

demonstration power plants using reverse electrodialysis

have been developed thus far, some observers have high

expectations for the approach. Investigate the technical

readiness and economic feasibility of this renewable power

source for providing 3%, or more, of annual U.S. electricity

by 2030. Present your conclusions in a report, including a

discussion of potential adverse environmental effects of such

power plants and at least three references.

11.6D During a phase change from liquid to vapor at fixed

pressure, the temperature of a binary nonazeotropic solution

such as an ammonia–water solution increases rather than

remains constant as for a pure substance. This attribute is

exploited in both the Kalina power cycle and in the Lorenz

refrigeration cycle. Write a report assessing the status of

technologies based on these cycles. Discuss the principal

advantages of using binary nonazeotropic solutions. What

are some of the main design issues related to their use in

power and refrigeration systems?

11.7D The following data are known for a 100-ton ammonia–

water absorption system like the one shown in Fig. 10.12.

The pump is to handle 570 lb of strong solution per minute.

The generator conditions are 175 lbf/in.

, 2208F. The absorber

is at 29 lbf/in.

with strong solution exiting at 808F. For the

evaporator, the pressure is 30 lbf/in.

and the exit temperature

is 108F. Specify the type and size, in horsepower, of the pump

required. Present your findings in a memorandum.

11.8D The Servel refrigerator works on an absorption principle

and requires no moving parts. An energy input by heat

transfer is used to drive the cycle, and the refrigerant circulates

due to its natural buoyancy. This type of refrigerator is

commonly employed in mobile applications, such as

recreational vehicles. Liquid propane is burned to provide

the required energy input during mobile operation, and

electric power is used when the vehicle is parked and can be

connected to an electrical outlet. Investigate the principles of

operation of commercially available Servel-type systems, and

study their feasibility for solar-activated operation. Consider

applications in remote locations where electricity or gas is

not available. Write a report summarizing your findings.

11.9D In the experiment for the regelation of ice, a small-diameter

wire weighted at each end is draped over a block of ice. The

loaded wire is observed to cut slowly through the ice without

leaving a trace. In one such set of experiments, a weighted

1.00-mm-diameter wire is reported to have passed through 08C

ice at a rate of 54 mm/h. Perform the regelation experiment

and propose a plausible explanation for this phenomenon.

11.10D Figure P11.10D shows the schematic of a hydraulic

accumulator in the form of a cylindrical pressure vessel with

a piston separating a hydraulic fluid from a charge of nitrogen

gas. The device has been proposed as a means for storing

some of the exergy of a decelerating vehicle as it comes to

rest. The exergy is stored by compressing the nitrogen. When

the vehicle accelerates again, the gas expands and returns

some exergy to the hydraulic fluid which is in communication

with the vehicle’s drive train, thereby assisting the vehicle to

accelerate. In a proposal for one such device, the nitrogen

operates in the range 50–150 bar and 200–350 K. Develop a

thermodynamic model of the accumulator and use the model

to assess its suitability for vehicle deceleration/acceleration.

Hydraulic

fluid

Nitrogen

gas

Piston

Fig. P11.10D

c11ThermodynamicRelations.indd702 Page 702 6/22/10 7:21:08 PM user-s146 c11ThermodynamicRelations.indd702 Page 702 6/22/10 7:21:08 PM user-s146 /Users/user-s146/Desktop/Merry_X-Mas/New/Users/user-s146/Desktop/Merry_X-Mas/New