Moran M.J., Shapiro H.N. Fundamentals of Engineering Thermodynamics
Подождите немного. Документ загружается.

used as the source. Specify the typical operating pressures for
such applications and estimate the variation in coefficient of
performance for the range of hot water temperature. Prepare a
memorandum summarizing your results.
10.9D Common cryogenic refrigeration applications include air
separation to obtain oxygen and nitrogen, large-scale produc-
tion of liquid hydrogen, and the liquefaction of natural gas.
Describe the equipment used to achieve the low temperatures
required in these applications. How do cryogenic systems dif-
fer from systems used for common refrigeration and air-
conditioning applications?
10.10D Determine the current status of magnetic refrigeration
technology for use in the 80 to 300 K range. Does this tech-
nology hold promise as an economical alternative to vapor-
compression systems? Discuss.
10.11D New Materials may Revive Thermoelectric Cooling
(see box Sec. 10.3). Investigate the current state-of-the-art of
nanotechnology in an area of current interest. Write a report
including at least three references.
486 Chapter 10 Refrigeration and Heat Pump Systems
487
11
C
H
A
P
T
E
R
Thermodynamic
Relations
ENGINEERING CONTEXT As seen in previous chapters, applica-
tion of thermodynamic principles to engineering systems requires data for specific internal
energy, enthalpy, entropy, and other properties. The objective of the present chapter is to
introduce thermodynamic relations that allow u, h, s, and other thermodynamic properties
of simple compressible systems to be evaluated from data that are more readily measured.
Primary emphasis is on systems involving a single chemical species such as water or a
mixture such as air. An introduction to general property relations for mixtures and
solutions is also included.
Means are available for determining pressure, temperature, volume, and mass experi-
mentally. In addition, the relationships between the specific heats c
v
and c
p
and tempera-
ture at relatively low pressure are accessible experimentally. Values for certain other
thermodynamic properties also can be measured without great difficulty. However, specific
internal energy, enthalpy, and entropy are among those properties that are not easily
obtained experimentally, so we resort to computational procedures to determine values
for these.
11.1 Using Equations of State
An essential ingredient for the calculation of properties such as the specific internal energy,
enthalpy, and entropy of a substance is an accurate representation of the relationship among
pressure, specific volume, and temperature. The p–v–T relationship can be expressed alter-
natively: There are tabular representations, as exemplified by the steam tables. The rela-
tionship also can be expressed graphically, as in the p–v–T surface and compressibility factor
charts. Analytical formulations, called equations of state, constitute a third general way of
expressing the p–v–T relationship. Computer software such as Interactive Thermodynamics:
IT also can be used to retrieve p–v–T data.
The virial equation and the ideal gas equation are examples of analytical equations of state
introduced in previous sections of the book. Analytical formulations of the p–v–T relation-
ship are particularly convenient for performing the mathematical operations required to
calculate u, h, s, and other thermodynamic properties. The object of the present section is to
expand on the discussion of p–v–T relations for simple compressible substances presented
in Chap. 3 by introducing some commonly used equations of state.
chapter objective
equations of state
488 Chapter 11 Thermodynamic Relations
virial equation
11.1.1 Getting Started
Recall from Sec. 3.4 that the virial equation of state can be derived from the principles of
statistical mechanics to relate the p–v–T behavior of a gas to the forces between mole-
cules. In one form, the compressibility factor Z is expanded in inverse powers of specific
volume as
(11.1)
The coefficients B, C, D, etc. are called, respectively, the second, third, fourth, etc. virial co-
efficients. Each virial coefficient is a function of temperature alone. In principle, the virial
coefficients are calculable if a suitable model for describing the forces of interaction between
the molecules of the gas under consideration is known. Future advances in refining the theory
of molecular interactions may allow the virial coefficients to be predicted with considerable
accuracy from the fundamental properties of the molecules involved. However, at present,
just the first two or three coefficients can be calculated and only for gases consisting of rel-
atively simple molecules. Equation 11.1 also can be used in an empirical fashion in which
the coefficients become parameters whose magnitudes are determined by fitting p–v–T data
in particular realms of interest. Only the first few coefficients can be found this way, and the
result is a truncated equation valid only for certain states.
In the limiting case where the gas molecules are assumed not to interact in any way, the
second, third, and higher terms of Eq. 11.1 vanish and the equation reduces to Z 1. Since
this gives the ideal gas equation of state The ideal gas equation of
state provides an acceptable approximation at many states, including but not limited to states
where the pressure is low relative to the critical pressure and/or the temperature is high rel-
ative to the critical temperature of the substance under consideration. At many other states,
however, the ideal gas equation of state provides a poor approximation.
Over 100 equations of state have been developed in an attempt to improve on the ideal
gas equation of state and yet avoid the complexities inherent in a full virial series. In gen-
eral, these equations exhibit little in the way of fundamental physical significance and are
mainly empirical in character. Most are developed for gases, but some describe the p–v–T
behavior of the liquid phase, at least qualitatively. Every equation of state is restricted to par-
ticular states. This realm of applicability is often indicated by giving an interval of pressure,
or density, where the equation can be expected to represent the p–v–T behavior faithfully.
When it is not stated, the realm of applicability of a given equation can be approximated by
expressing the equation in terms of the compressibility factor Z and the reduced properties
p
R
, T
R
, v
R
and superimposing the result on a generalized compressibility chart or comparing
with tabulated compressibility data obtained from the literature.
11.1.2 Two-Constant Equations of State
Equations of state can be classified by the number of adjustable constants they include. Let
us consider some of the more commonly used equations of state in order of increasing
complexity, beginning with two-constant equations of state.
VAN DER WAALS EQUATION
An improvement over the ideal gas equation of state based on elementary molecular arguments
was suggested in 1873 by van der Waals, who noted that gas molecules actually occupy more
than the negligibly small volume presumed by the ideal gas model and also exert long-range
attractive forces on one another. Thus, not all of the volume of a container would be avail-
able to the gas molecules, and the force they exert on the container wall would be reduced
pv R
T.Z pv
RT,
Z 1
B1T2
v
C 1T2
v
2
D1T2
v
3
###
11.1 Using Equations of State 489
because of the attractive forces that exist between molecules. Based on these elementary
molecular arguments, the van der Waals equation of state is
(11.2)
The constant b is intended to account for the finite volume occupied by the molecules, the
term accounts for the forces of attraction between molecules, and is the universal gas
constant. Note than when a and b are set to zero, the ideal gas equation of state results.
The van der Waals equation gives pressure as a function of temperature and specific vol-
ume and thus is explicit in pressure. Since the equation can be solved for temperature as a
function of pressure and specific volume, it is also explicit in temperature. However, the
equation is cubic in specific volume, so it cannot generally be solved for specific volume
in terms of temperature and pressure. The van der Waals equation is not explicit in specific
volume.
EVALUATING a AND b. The van der Waals equation is a two-constant equation of state.
For a specified substance, values for the constants a and b can be found by fitting the equa-
tion to p–v–T data. With this approach several sets of constants might be required to cover
all states of interest. Alternatively, a single set of constants for the van der Waals equation
can be determined by noting that the critical isotherm passes through a point of inflection at
the critical point, and the slope is zero there. Expressed mathematically, these conditions are,
respectively
(11.3)
Although less overall accuracy normally results when the constants a and b are determined
using critical point behavior than when they are determined by fitting p–v–T data in a par-
ticular region of interest, the advantage of this approach is that the van der Waals constants
can be expressed in terms of the critical pressure p
c
and critical temperature T
c
, as demon-
strated next.
For the van der Waals equation at the critical point
Applying Eqs. 11.3 with the van der Waals equation gives
Solving the foregoing three equations for a, b, and in terms of the critical pressure and
critical temperature
(11.4a)
(11.4b)
(11.4c)
v
c
3
8
R T
c
p
c
b
RT
c
8p
c
a
27
64
R
2
T
2
c
p
c
v
c
a
0p
0v
b
T
RT
c
1v
c
b2
2
2a
v
3
c
0
a
0
2
p
0v
2
b
T
2RT
c
1v
c
b2
3
6a
v
4
c
0
p
c
RT
c
v
c
b
a
v
2
c
a
0
2
p
0v
2
b
T
0,
a
0p
0v
b
T
0
1critical point2
Ra
v
2
p
RT
v b
a
v
2
van der Waals equation
p
v
∂p
–––
∂v
(
(
T
= 0
T < T
c
T = T
c
T > T
c
Critical
point
_

490 Chapter 11 Thermodynamic Relations
Values of the van der Waals constants a and b determined from Eqs. 11.4a and 11.4b for
several common substances are given in Table A-24 for pressure in bar, specific volume in
m
3
/kmol, and temperature in K.
GENERALIZED FORM. Introducing the compressibility factor the reduced tem-
perature T
R
TT
c
, the pseudoreduced specific volume and the foregoing
expressions for a and b, the van der Waals equation can be written in terms of Z, v
R
, and
T
R
as
(11.5)
or alternatively in terms of Z, T
R
, and p
R
as
(11.6)
The details of these developments are left as exercises. Equation 11.5 can be evaluated for
specified values of v
R
and T
R
and the resultant Z values located on a generalized compress-
ibility chart to show approximately where the equation performs satisfactorily. A similar ap-
proach can be taken with Eq. 11.6.
The compressibility factor at the critical point yielded by the van der Waals equation is
determined from Eq. 11.4c as
Actually, Z
c
varies from about 0.23 to 0.33 for most substances (see Tables A-1). Accord-
ingly, with the set of constants given by Eqs. 11.4, the van der Waals equation is inaccurate
in the vicinity of the critical point. Further study would show inaccuracy in other regions as
well, so this equation is not suitable for many thermodynamic evaluations. The van der Waals
equation is of interest to us primarily because it is the simplest model that accounts for the
departure of actual gas behavior from the ideal gas equation of state.
REDLICH–KWONG EQUATION
Three other two-constant equations of state that have been widely used are the Berthelot, Di-
eterici, and Redlich–Kwong equations. The Redlich–Kwong equation, considered by many
to be the best of the two-constant equations of state, is
(11.7)
This equation, proposed in 1949, is mainly empirical in nature, with no rigorous justification
in terms of molecular arguments. The Redlich–Kwong equation is explicit in pressure but not
in specific volume or temperature. Like the van der Waals equation, the Redlich–Kwong equa-
tion is cubic in specific volume.
Although the Redlich–Kwong equation is somewhat more difficult to manipulate math-
ematically than the van der Waals equation, it is more accurate, particularly at higher pres-
sures. In recent years, several modified forms of this equation have been proposed to
achieve improved accuracy. The two-constant Redlich–Kwong equation performs better
p
R T
v b
a
v 1v b2T
1
2
Z
c
p
c
v
c
R T
c
0.375
Z
3
a
p
R
8T
R
1b Z
2
a
27p
R
64T
2
R
b Z
27p
2
R
512T
3
R
0
Z
v¿
R
v¿
R
1
8
27
64
T
R
v¿
R
v¿
R
p
c
v
RT
c
,
Z pv
RT,
Redlich–Kwong equation
11.1 Using Equations of State 491
than some equations of state having several adjustable constants; still, two-constant equa-
tions of state tend to be limited in accuracy as pressure (or density) increases. Increased
accuracy at such states normally requires equations with a greater number of adjustable
constants.
EVALUATING a AND b. As for the van der Waals equation, the constants a and b in Eq. 11.7
can be determined for a specified substance by fitting the equation to p–v–T data, with sev-
eral sets of constants required to represent accurately all states of interest. Alternatively, a
single set of constants in terms of the critical pressure and critical temperature can be eval-
uated using Eqs. 11.3, as for the van der Waals equation. The result is
(11.8)
where a0.42748 and b 0.08664. Evaluation of these constants is left as an exercise.
Values of the Redlich–Kwong constants a and b determined from Eqs. 11.8 for several com-
mon substances are given in Table A-24 for pressure in bar, specific volume in m
3
/kmol, and
temperature in K.
GENERALIZED FORM. Introducing the compressibility factor Z, the reduced temperature
T
R
, the pseudoreduced specific volume v
R
, and the foregoing expressions for a and b, the
Redlich–Kwong equation can be written as
(11.9)
Equation 11.9 can be evaluated at specified values of v
R
and T
R
and the resultant Z values
located on a generalized compressibility chart to show the regions where the equation per-
forms satisfactorily. With the constants given by Eqs. 11.8, the compressibility factor at the
critical point yielded by the Redlich–Kwong equation is Z
c
0.333, which is at the high end
of the range of values for most substances, indicating that inaccuracy in the vicinity of the
critical point should be expected.
In Example 11.1, the pressure of a gas is determined using three equations of state and
the generalized compressibility chart. The results are compared.
Z
v¿
R
v¿
R
b¿
a¿
1v¿
R
b¿ 2 T
3
2
R
a a¿
R
2
T
5
2
c
p
c
and
b b¿
R T
c
p
c
EXAMPLE 11.1 Comparing Equations of State
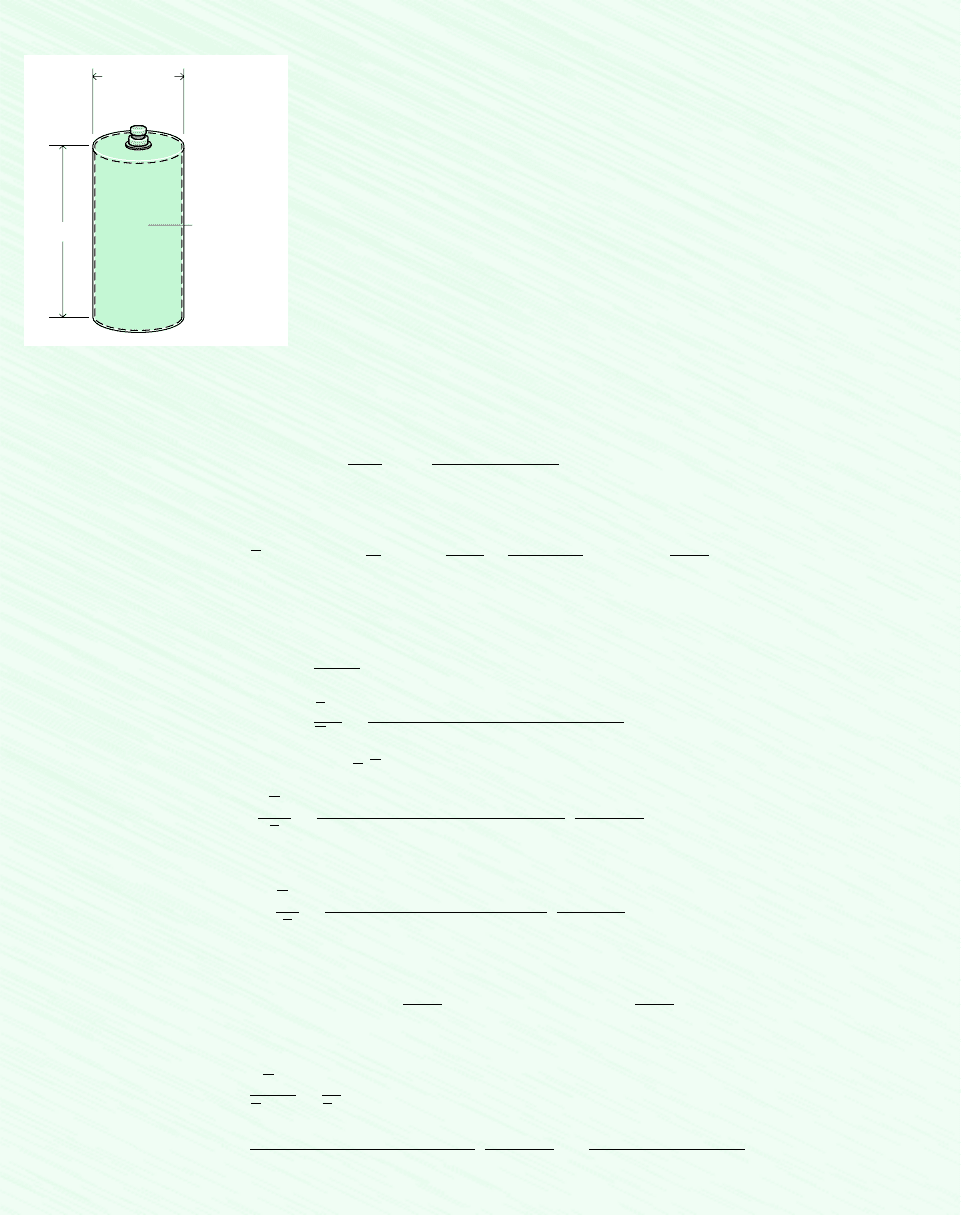
A cylindrical tank containing 4.0 kg of carbon monoxide gas at 50C has an inner diameter of 0.2 m and a length of
1 m. Determine the pressure, in bar, exerted by the gas using (a) the generalized compressibility chart, (b) the ideal gas
equation of state, (c) the van der Waals equation of state, (d) the Redlich–Kwong equation of state. Compare the results
obtained.
SOLUTION
Known: A cylindrical take of known dimensions contains 4.0 kg of CO gas at 50C.
Find: Determine the pressure exerted by the gas using four alternative methods.
492 Chapter 11 Thermodynamic Relations
Schematic and Given Data:
D = 0.2 m
L = 1 m
4 kg CO gas
at –50°C
Figure E11.1
Assumptions:
1. As shown in the accompanying figure, the closed system is taken as the gas.
2. The system is at equilibrium.
Analysis: The molar specific volume of the gas is required in each part of the solution. Let us begin by evaluating it. The
volume occupied by the gas is
The molar specific volume is then
(a) From Table A-1 for CO, T
c
133 K, p
c
35 bar. Thus, the reduced temperature T
R
and pseudoreduced specific volume
are, respectively
Turning to Fig. A-2, Z 0.9. Solving for pressure and inserting known values
(b) The ideal gas equation of state gives
(c) For carbon monoxide, the van der Waals constants a and b given by Eqs. 11.4 can be read directly from Table A-24. Thus
Substituting into Eq. 11.2
72.3 bar
18314 N
#
m/kmol
#
K2
1223 K2
10.2198 0.03952 1m
3
/kmol2
`
1 bar
10
5
N/m
2
`
1.474 bar 1m
3
/kmol2
2
10.2198 m
3
/kmol2
2
p
R
T
v b
a
v
2
a 1.474 bar a
m
3
kmol
b
2
and
b 0.0395
m
3
kmol
p
R
T
v
18314 N
#
m/ kmol
#
K21223 K2
10.2198 m
3
/kmol2
`
1 bar
10
5
N/m
2
` 84.4 bar
p
ZR
T
v
0.918314 N
#
m/ kmol
#
K2
1223 K2
10.2198 m
3
/kmol2
`
1 bar
10
5
N/m
2
` 75.9 bar
Z pv
RT
v¿
R
v
p
c
RT
c
10.2198 m
3
/kmol2 135 10
5
N/m
2
2
18314 N
#
m/kmol
#
K2 1133 K2
0.696
T
R
223 K
133 K
1.68
v¿
R
v Mv M a
V
m
b a28
kg
kmol
b
a
0.0314 m
3
4.0 kg
b 0.2198
m
3
kmol
V a
pD
2
4
b L
p10.2 m2
2
11.0 m2
4
0.0314 m
3

11.1 Using Equations of State 493
Alternatively, the values for and T
R
obtained in the solution of part (a) can be substituted into Eq. 11.5, giving Z 0.86.
Then, with bar. The slight difference is attributed to roundoff.
(d) For carbon monoxide, the Redlich–Kwong constants given by Eqs. 11.8 can be read directly from Table A-24. Thus
Substituting into Eq. 11.7
Alternatively, the values for and T
R
obtained in the solution of part (a) can be substituted into Eq. 11.9, giving Z 0.89.
Then, with bar.
In comparison to the value of part (a), the ideal gas equation of state predicts a pressure that is 11% higher and the van der
Waals equation gives a value that is 5% lower. The Redlich–Kwong value is about 1% less than the value obtained using the
compressibility chart.
p ZR
T
v, p 75.1
v
R
¿
75.1 bar
18314 N
#
m/kmol
#
K2 1223 K2
10.2198 0.027372 m
3
/kmol
`
1 bar
10
5
N/m
2
`
17.22 bar
10.21982 10.247172 12232
1
2
p
RT
v b
a
v 1v b2T
1
2
a
17.22 bar 1m
6
21K2
1
2
1kmol2
2
and
b 0.02737 m
3
/kmol
p ZR
T
v, p 72.5
v
R
¿
11.1.3 Multiconstant Equations of State
To fit the p–v–T data of gases over a wide range of states, Beattie and Bridgeman
proposed in 1928 a pressure-explicit equation involving five constants in addition to the
gas constant. The Beattie–Bridgeman equation can be expressed in a truncated virial
form as
(11.10)
where
(11.11)
The five constants a, b, c, A, and B appearing in these equations are determined by curve fit-
ting to experimental data.
Benedict, Webb, and Rubin extended the Beattie–Bridgeman equation of state to cover a
broader range of states. The resulting equation, involving eight constants in addition to the
gas constant, has been particularly successful in predicting the p–v–T behavior of light
hydrocarbons. The Benedict–Webb–Rubin equation is
(11.12)
c
v
3
T
2
a1
g
v
2
b exp a
g
v
2
bp
R T
v
aBR T A
C
T
2
b
1
v
2
1bR T a2
v
3
aa
v
6
d BbcR
T
2
g BbRT Aa BcR
T
2
b BRT A cR
T
2
p
R T
v
b
v
2
g
v
3
d
v
4
Beattie–Bridgeman
equation
Benedict–Webb–Rubin
equation
Values of the constants appearing in Eq. 11.12 for five common substances are given in
Table A-24 for pressure in bar, specific volume in m
3
/kmol, and temperature in K. Because
Eq. 11.12 has been so successful, its realm of applicability has been extended by introduc-
ing additional constants.
Equations 11.10 and 11.12 are merely representative of multiconstant equations of state.
Many other multiconstant equations have been proposed. With high-speed computers, equa-
tions having 50 or more constants have been developed for representing the p–v–T behavior
of different substances.
494 Chapter 11 Thermodynamic Relations
11.2 Important Mathematical Relations
Values of two independent intensive properties are sufficient to fix the state of a simple com-
pressible system of specified mass and composition (Sec. 3.1). All other intensive properties
can be determined as functions of the two independent properties: p p(T, v), u u(T, v),
h h(T, v), and so on. These are all functions of two independent variables of the form
z z(x, y), with x and y being the independent variables. It might also be recalled that the
differential of every property is exact (Sec. 2.2.1). The differentials of nonproperties such as
work and heat are inexact. Let us review briefly some concepts from calculus about functions
of two independent variables and their differentials.
The exact differential of a function z, continuous in the variables x and y,is
(11.13a)
This can be expressed alternatively as
(11.13b)
where M (0z0x)
y
and N (0z0y)
x
. The coefficient M is the partial derivative of z with
respect to x (the variable y being held constant). Similarly, N is the partial derivative of z
with respect to y (the variable x being held constant).
If the coefficients M and N have continuous first partial derivatives, the order in which a
second partial derivative of the function z is taken is immaterial. That is
(11.14a)
or
(11.14b)
which can be called the test for exactness, as discussed next.
In words, Eqs. 11.14 indicate that the mixed second partial derivatives of the function z
are equal. The relationship in Eq. 11.14 is both a necessary and sufficient condition for the
exactness of a differential expression, and it may therefore be used as a test for exactness.
When an expression such as Mdx Ndydoes not meet this test, no function z exists whose
differential is equal to this expression. In thermodynamics, Eq. 11.14 is not generally used
to test exactness but rather to develop additional property relations. This is illustrated in
Sec. 11.3 to follow.
a
0M
0y
b
x
a
0N
0x
b
y
0
0y
ca
0z
0x
b
y
d
x
0
0x
ca
0z
0y
b
x
d
y
dz M dx N dy
dz a
0z
0x
b
y
dx a
0z
0y
b
x
dy
exact differential
test for exactness
11.2 Important Mathematical Relations 495
Two other relations among partial derivatives are listed next for which applications are
found in subsequent sections of this chapter. These are
(11.15)
and
(11.16)
for example. . .
consider the three quantities x, y, and z, any two of which may
be selected as the independent variables. Thus, we can write x x(y, z) and y y(x, z). The
differentials of these functions are, respectively
Eliminating dy between these two equations results in
(11.17)
Since x and z can be varied independently, let us hold z constant and vary x. That is, let
dz 0 and dx 0. It then follows from Eq. 11.17 that the coefficient of dx must vanish, so
Eq. 11.15 must be satisfied. Similarly, when dx 0 and dz 0, the coefficient of dz in
Eq. 11.17 must vanish. Introducing Eq. 11.15 into the resulting expression and rearranging
gives Eq. 11.16. The details are left as an exercise.
APPLICATION. An equation of state p p(T, v) provides a specific example of a function
of two independent variables. The partial derivatives (0p0T )
v
and (0p0v)
T
of p(T, v) are im-
portant for subsequent discussions. The quantity (0p0T )
v
is the partial derivative of p with
respect to T (the variable v being held constant). This partial derivative represents the slope
at a point on a line of constant specific volume (isometric) projected onto the p–T plane.
Similarly, the partial derivative (0p0v)
T
is the partial derivative of p with respect to v (the
variable T being held constant). This partial derivative represents the slope at a point on
a line of constant temperature (isotherm) projected on the p–v plane. The partial derivatives
(0p0T )
v
and (0p0v)
T
are themselves intensive properties because they have unique values
at each state.
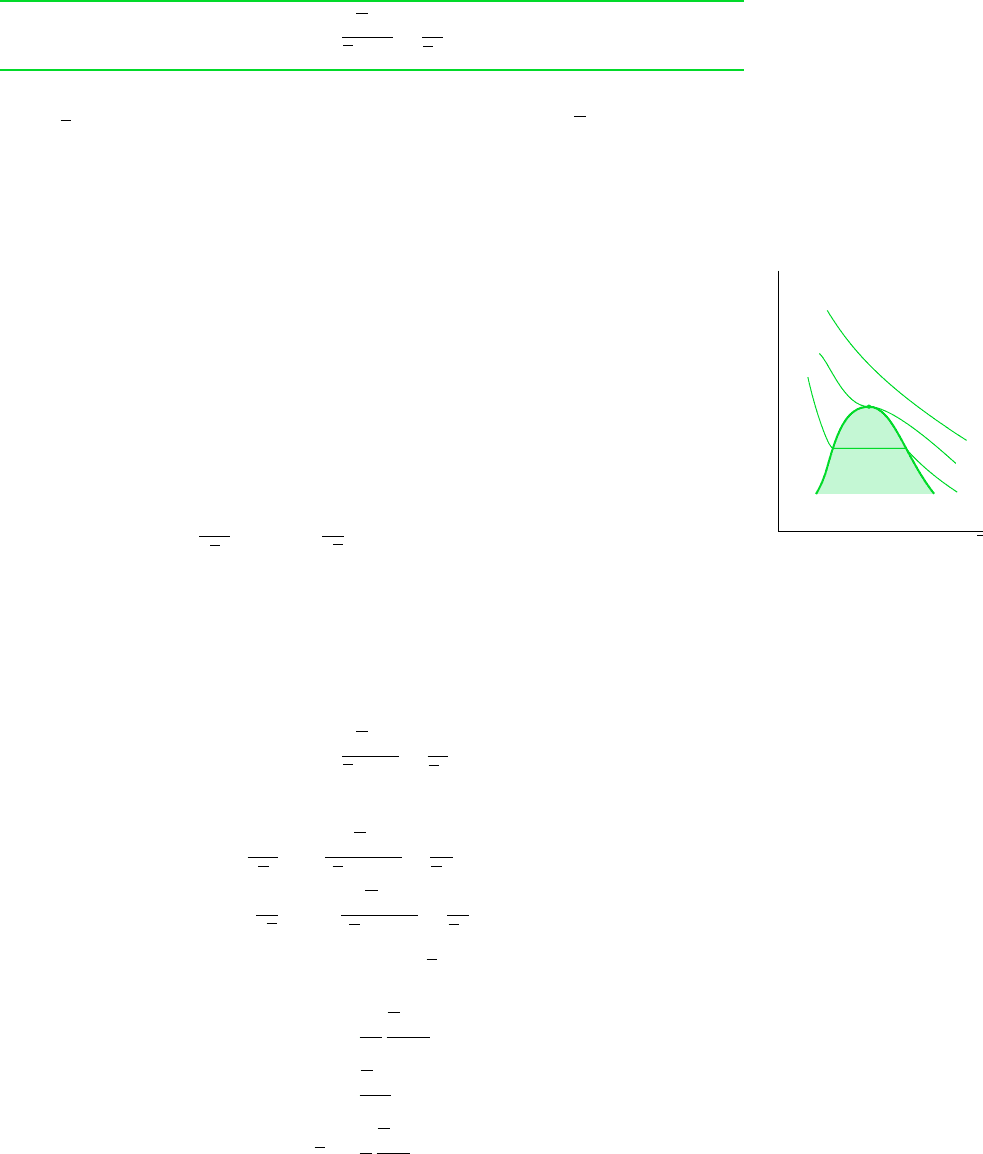
The p–v–T surfaces given in Figs. 3.1 and 3.2 are graphical representations of func-
tions of the form p p(v, T ). Figure 11.1 shows the liquid, vapor, and two-phase regions
of a p–v–T surface projected onto the p–v and p–T planes. Referring first to Fig. 11.1a,
note that several isotherms are sketched. In the single-phase regions, the partial deriva-
tive (0p0v)
T
giving the slope is negative at each state along an isotherm except at the crit-
ical point, where the partial derivative vanishes. Since the isotherms are horizontal in the
two-phase liquid–vapor region, the partial derivative (0p0v)
T
vanishes there as well. For
these states, pressure is independent of specific volume and is a function of temperature
only: p p
sat
(T ).
Figure 11.1b shows the liquid and vapor regions with several isometrics (constant specific
volume lines) superimposed. In the single-phase regions, the isometrics are nearly straight
or are slightly curved and the partial derivative (0p0T )
v
is positive at each state along the
curves. For the two-phase liquid–vapor states corresponding to a specified value of temper-
ature, the pressure is independent of specific volume and is determined by the temperature
c1 a
0x
0y
b
z
a
0y
0x
b
z
d dx ca
0x
0y
b
z
a
0y
0z
b
x
a
0x
0z
b
y
d dz
dx a
0x
0y
b
z
dy a
0x
0z
b
y
dz
and
dy a
0y
0x
b
z
dx a
0y
0z
b
x
dz
a
0y
0z
b
x
a
0z
0x
b
y
a
0x
0y
b
z
1
a
0x
0y
b
z
a
0y
0x
b
z
1
