Mindlin R.D., Yang J. An Introduction to the Mathematical Theory of Vibrations of Elastic Plates
Подождите немного. Документ загружается.

Solutions
of
the Three-Dimensional Equations
51
and (2.103), for the antisymmetric modes, becomes
A{X + 2{i}}2
sin7
72*
= Ak cos j]
2
b
D cos
7^
= 0
Thus the dilatational and equivoluminal modes are no longer coupled.
The equivoluminal modes have become identical with the simple
thickness-shear modes (Section 2.03) with
a
=
2*.
!JL
=
2*. J£
(
2.i08)
2b \p 2b ^p
where n or q is odd for the antisymmetric modes and even for the
symmetric modes. Since the frequencies are independent of k, the
ordinates, at
£b=0,
of
the
curves n= constant, in Fig. 2.092, do not change
as k is varied.
The frequencies of
the
dilatational modes have become
a =
Mfi±lE
(2.109)
b
V P
where {rjib) are the roots of
tan0y
2
6) = -
(,
fc*fr
+ 2/<)
(2.1010)
kb
for the symmetric modes and
C0X
(
Jl2b
)JjlSM2A (2.10H)
kb
for the antisymmetric modes. As
k-—
°°,
the roots approach
T]
2
b =
— (2.1012)
where m is even for the symmetric modes and odd for the antisymmetric
modes. The corresponding frequencies are given by the ordinates, at
£6=0,
of the curves /w=constant in Fig. 2.092. As &-«-0, the roots
approach

52
Mathematical Theory of Vibrations of Elastic Plates
r?
2
b =
I
j- (2.1013)
where p is odd for the symmetric modes and even for the antisymmetric
modes. The corresponding frequencies are those of the simple
thickness-stretch modes and are given by the points markedp=0, 1,2, ....
in Fig. 2.092. As k diminishes from infinity to zero, the ordinates, at £6=0,
drop.
For example, referring to Fig. 2.092(a) the curve for the symmetric
mode which starts out as w=2 at
h=oo,
(for which the figure is drawn)
descends in the spectrum until its ordinate, at £b=0, reaches the point
markedp=\ when £=0. The whole curve distorts in the process and ends
up as the heavy full line which is the second from the bottom in Fig.
2.113(a). Similarly, the curve for the antisymmetic mode, which starts
out as m=\ in Fig. 2.092, descends in the spectrum until its ordinate, at
gb=0, is zero. Its limiting shape, at k=0, is given by the lowest dashed
line in Fig. 2.113(a). Meanwhile, the curves marked «=2 and n=\, while
retaining their ordinates at
£b=0,
shift their positions to the third, heavy,
full line and the second dashed line, respectively, in Fig. 2.113(a).
During the passage from
k=<x
to k=0 the mode-shapes (i.e., the
variation of displacement with x
2
) do not change, in the case of the
equivoluminal modes, when £b=0, since the boundary conditions are
independent of k. The mode-shapes of the dilatational modes, however,
depend on k. When $>=0, the dilatational modes involve only the
component of displacement u
2
. This has nodes or antinodes at x
2
=+b
according as k is infinity or zero. At intermediate values of k there are
neither nodes or antinodes at the surfaces. The shape of the lowest
antisymmetric mode is of special interest. Here the displacement is
proportional to cosrj
2
b where r\
2
b is the lowest root of (2.1011). When
k=&>,
y\
2
b=nl2
(i.e., m=\) so that the displacement varies as a half-sine
wave through the thickness, with a node at each surface and the
maximum at the center. As k diminishes, so does the first root of (2.1011).
Hence the nodes spread outward from the surfaces of the plate, leaving a
displacement, inside the plate, which approaches a uniform distribution

Solutions of the Three-Dimensional Equations
53
across the thickness as k approaches zero. Meanwhile the frequency,
which is proportional to
rj
2
b,
has also diminished and is approaching the
lowest dashed line in Fig. 2.113(a). This mode, then, is the one which
contributes the uniform part of the transverse displacement
accompanying low-frequency flexural vibrations of
plates.
It is to be noted that (2.1011) has a root 7726=0 only when k=0. On
the other hand, (2.1010), which governs the frequencies of
the
symmetric
modes, always has a root 7726=0. Hence there is always present a mode
whose limiting frequency, at £6=0, is zero. Similarly, there is zero-root of
the second of (2.106), governing the symmetric thickness-shear modes.
This accounts for the presence of the lowest, heavy, full curve, in Fig.
2.113(a), despite the fact that the next higher symmetric dilatational
mode, which starts out, at
&=oo,
as the curve TW=2 in Fig. 2.092(a), never
falls below the point
p=\
at £6=0.
2.11 Coupled Dilatational and Equivoluminal Modes in an Infinite,
Isotropic Plate with Free Faces (Plane Strain)
We come, now, to the problem of the vibrations, in a state of plane
strain, of a plate with traction-free faces (Rayleigh, 1889). The boundary
conditions are obtained from (2.102) and (2.103) by setting
k=0,
with the
results, for the symmetric modes,
5 (^
2
-/?
2
)cos
«/3
+
2
C £/?
cos/?/3
= 0
2B$a sin ab - c(%
2
- p
1
)sin fib
=
0
and, for the antisymmetric modes,
A(£
2
-/3
2
)sinab-2D
%J3 sin J3b =
0
t
\ (2.112)
2
-/?
2
)cos/?6 = 0
These equations have the four solutions
54
Mathematical Theory of Vibrations of Elastic Plates
5 = 0, £ = 0, sin
,96
= 0
C = 0, £ = 0, cosar6 = 0
,4 = 0, £ = 0, cosfib
=
0
(2113)
£>
= 0, £ = 0, sin a* = 0
which lead to the simple thickness-modes discussed in Section 2.03.
Equations (2.111) and (2.112) also have the two solutions
5 = 0, £
2
=fi
2
, cosj3b
=
0
,
,
(2-114)
A
=
0, %
2
=fi
2
, sinfib
=
0
which lead to the equivoluminal modes discussed in Section 2.07. Finally,
(2.111),
governing the symmetric motions, have the solution
5_
2{ficosfib
C-({
2
-f)cosab
(2
-
U5)
Um0b_ A?afi
T~~l
\7
(2.116)
tanab
V;
2
-
fi
2
f
and (2.112), governing the antisymmetric motions, have the solution
(2.117)
(2.118)
A
D
tar
(f
ifib _
I
^fi sin
fib
-fi
2
(e-
)
sin ab
-Pi
tanab
4£ afi
These solutions, first obtained by Rayleigh in 1889, have been the
subject of extensive study in the ensuing years (see, for example, Lamb,
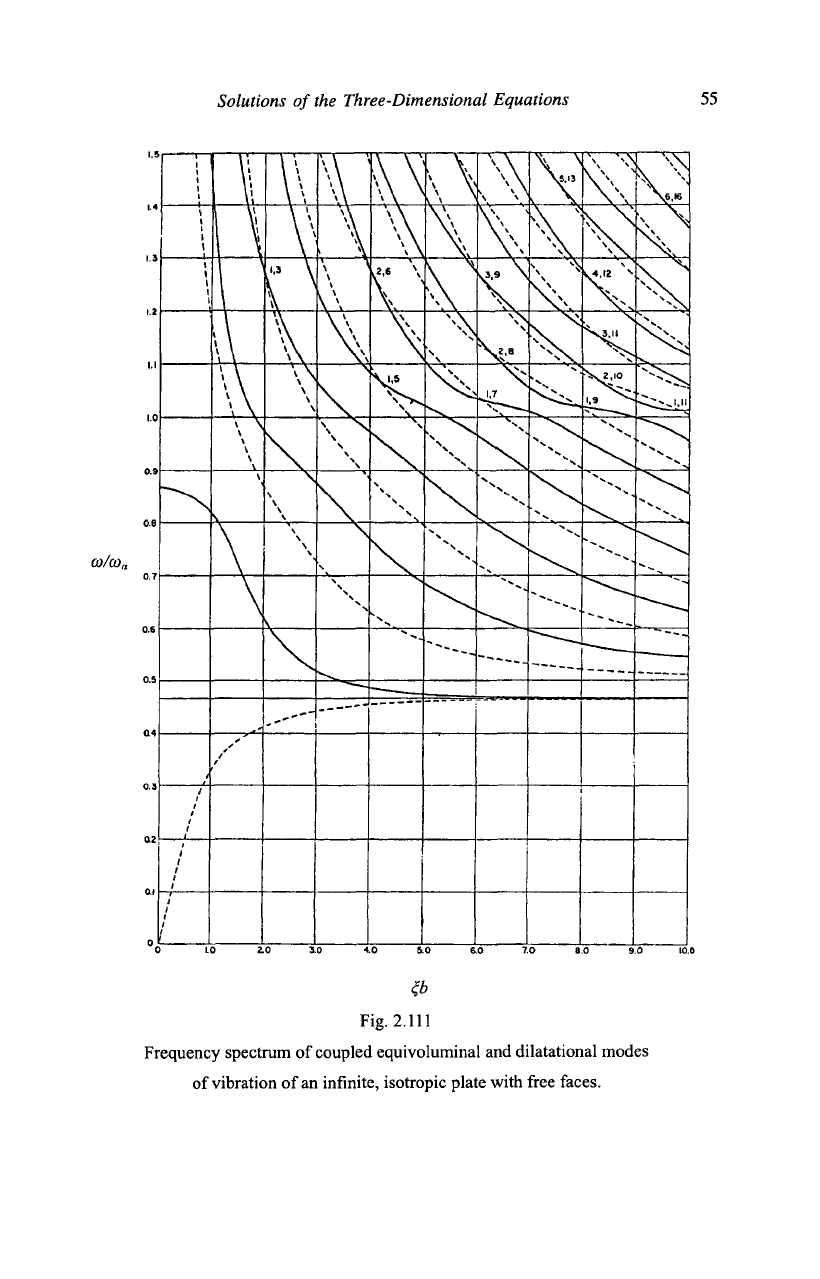
1917 and Holden, 1951). The frequency spectra determined by (2.116)
and (2.118) are illustrated in Fig. 2.111 for
X=2jx,
i.e.,
v=l/3.
The full
lines are for the symmetric modes and the dashed lines are for the
antisymmetric modes. We proceed
to a
detailed analysis
of
the
frequencies.
Solutions of the Three-Dimensional Equations
55
/
1
1
1
1
1
1
f
1
1
1
f
1
1
1
1
\
\
I \
I \
\ \
\ \
\ \
\ \
\ \
\ \
\ \
\ \
\
\
\
\
\
\
/
/
\ \
\ \
\ \
\ \
V
\
\ 1
\ \
\ \
*.3 \
\\
\\
\\
\
\
\ \
\
A
\
\
\
\
\
\
\
\
,,---
* t *
\ \
\ \
»
\
\ \
\ \
\
L \
\ \
\ \
\ \
\ \
\ \
\ \
\ ^
\
\
\
\
'
\
\
\
\
N
\V
\ \
\ \
\ \
\ \
\ \
V
>
V
\\
\ \
Vl,5
\
\
"S
\N
\
\
\\
\\
\\
s \
\
\
•
---—-.
\ \
\ \
\
\
W
Z,B
\
\
^'
\
N
\S.I3
\
\. \
\ s
X \
X
v
v
v
"*
-~-^
\ X
^VJ.
11
1,9 ^~-
-^
"^
^--,,
•^^
N
^
\ X
\ x^
:^
"*"•-
0 LO 2.0 3.0 4.0 fcO 6.0 7.0 8.0 9.0 IO.0
&
Fig. 2.111
Frequency spectrum of coupled equivoluminal and dilatational modes
of vibration of an infinite, isotropic plate with free faces.

56
Mathematical Theory of Vibrations of Elastic Plates
Now,
a
S
p
=s
hi
H
-1
-1
(2.119)
where co
a
and
cop
are defined by (2.099). In an infinite plate £ must be
real. Hence a is real if
co>co
a
and imaginary if
a)<co
a
;
/? is real if
co>a>p
and imaginary if
co<a>p.
Recalling that co
a
>copwe see that there are
three ranges of frequencies
co<cop,a and/? imaginary
a>p<co<a>
a
,
a imaginary,
/?
real
co
>
co
a
,
a and p real
in which the roots of (2.116) and (2.118) (and also the mode-shapes) will
have different characters since the transcendental functions of a and ft
will be trigonometric or hyperbolic according as a and ft are real or
imaginary. When a or/? is imaginary we shall let a=ia
u
orfi=i/3\, where
a.\
and/?i are real.
In the lowest frequency range
{a><cop),
equation (2.116), for the
symmetric modes, becomes (since tan?z=z'tanhz)
tanhflft
=
4^,A (2.1H0)
tanha^ (<f+/?,
2
j
and equation (2.118), for the antisymmetric modes, becomes
tanhfl^
+
fl
2
)
2
tanha,6 4^
2
«,
y
5
1
For very short wave-lengths along x\, &-*&, a^-'-oo,
p
x
b^co.
Hence, both (2.1110) and (2.1111) reduce to
Solutions of the Three-Dimensional Equations 57
(^
2
+A
2
)
2
-4^
2
a
1
A=0
(2.1112)
or
CO
co.
6 4
^V-8^
+
8
/
CO
7»
&>«
3-2-
oo
2\
V
fi*
a
J
1-3
V <°°J
= 0 (2.1113)
which is the equation governing the frequency of surface-waves
(Rayleigh, 1887). This equation has only one root in the range 0<a><cap.
For
v=l/3,
co/cop=0.932
and, since
co
p
I
co
a
=
1/2,
co/a>
a
=0A66.
This is
the asymptotic value of the two lowest curves in Fig.
2.111.
For very long wave-lengths along x
u
£b—-0,
ab~*0,
/?Z?-«-0.
At this
limit, equation (2.1110) has no root in the range
0<a><a>p.
As for
(2.1111), for the antisymmetric motion, we write
tanner's a,6
r 1
1--O.V
tanhfl6*fl&|l—#V
(2.1114)
and find
CO
H
1
2^
(2.1115)
on the supposition that
colcop
is small, which is seen to be verified. For
v=l/3,
(2.1115) may be written
co/co
a
=^b/2.
This gives the initial slope of
the lowest curve in Fig.
2.111.
Equation (2.1115) may be written as
® = #
2
6,
3p(l-v
2
)
(2.1116)
which is the familiar form in the classical theory of flexure of thin plates.
58
Mathematical Theory of Vibrations of Elastic Plates
In the intermediate range of frequencies (co
p
<co<co
a
)we write,
for the symmetric modes,
«?/» _
Afaj,
and, for the antisymmetric modes,
tan/76 (f-fi
2
J
, , , (2.1118)
tanha^ 4£
2
a,/7
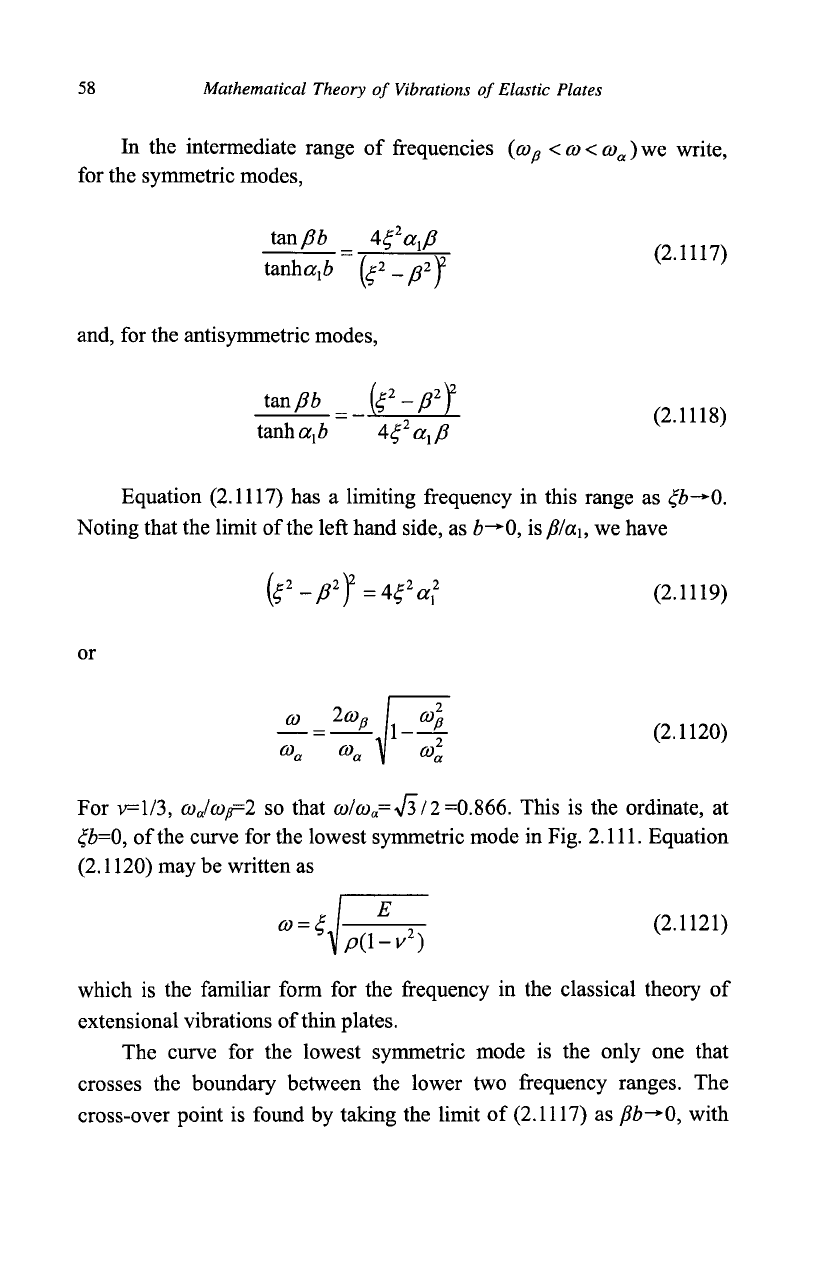
Equation (2.1117) has a limiting frequency in this range as £b-*0.
Noting that the limit of the left hand side, as b~-0, is pia
u
we have
(%
2
-j3
2
}
=4£
2
a? (2.1119)
or
(O 2(0/, | (On
—
=
—S-\\ f (2.1120)
For
v=l/3,
coj(op=2
so that
(o/a>
a
=
yfe/2
=0.866.
This is the ordinate, at
<f6=0, of the curve for the lowest symmetric mode in Fig.
2.111.
Equation
(2.1120) may be written as
a=
H-ir^
(2
-
1121)
\p(l-v )
which is the familiar form for the frequency in the classical theory of
extensional vibrations of thin plates.
The curve for the lowest symmetric mode is the only one that
crosses the boundary between the lower two frequency ranges. The
cross-over point is found by taking the limit of (2.1117) as fib-*0, with

Solutions of the Three-Dimensional Equations 59
the result
2tanh«
1
6 = (l-v)a,6 (2.1122)
Then the abscissa of the cross-over point is
Zb
=
a
x
bj2{\-v) (2.1123)
where a
x
b is the root of (2.1122). For
v=l/3,
a
{
b=2.99 and £6=3.46, as
shown in Fig.
2.111.
The curves for all the higher modes approach co^m
a
(=1/2 for v=l/3)
asymptotically as
#>—<x>.
That is, except for the first symmetric and first
antisymmetric modes,
a>-*a>p
as the wave-length approaches zero.
In the highest range of frequencies
(co>a>
a
)
we use (2.116) and
(2.118),
since both a and/? are real. Both equations have the special roots
sincc6=0, sin/?6=0; cosctZ>=0, cos/?6=0
so that the frequency curves for both symmetric and antisymmetric
modes pass through the intersections of curves m odd, n odd and also m
even, n even of
Fig.
2.091,
in the manner indicated by the curve £=0 in
Fig. 2.101 for the antisymmetric modes. With this information and with
the results of the analysis of limiting cases given above, the frequency
spectrum of
co/co
a
vs. gb may be sketched approximately, following the
pattern indicated in Fig.
2.101.
For detailed calculations of intermediate points, it is convenient to
alter the form of the transcendental equations.
Let
a =
% '
r = ab (2
-
1124)
Then
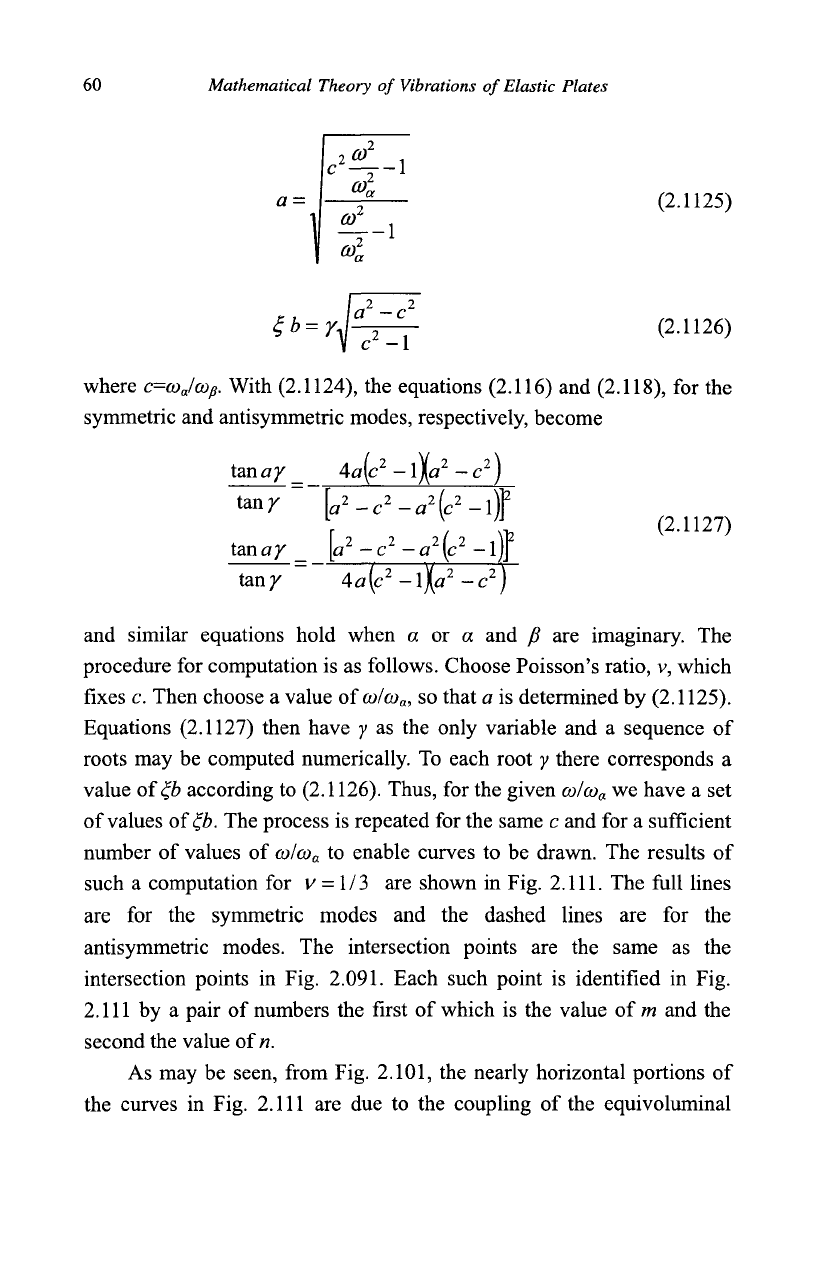
60
Mathematical Theory of Vibrations of Elastic Plates
a
—
c<-i
—^ (2.1125)
^
b
=
Y
TJ^\
(2-1126)
where
c=cojco
fs
.
With (2.1124), the equations (2.116) and (2.118), for the
symmetric and antisymmetric modes, respectively, become
tan ay _ 4a(c
2
- \\a
2
- c
2
)
t*nr~~[a
2
-c
2
-a
2
{c
2
-if
r / vb
(
2
-
1127
>
tan ay _
[a
2
-c
2
- a
2
[c
2
- 1J
tany ~ 4a{c
2
-\\a
2
-c
2
)
and similar equations hold when a or a and /? are imaginary. The
procedure for computation is as follows. Choose Poisson's ratio, v, which
fixes c. Then choose a value of
co/co
a
,
so that a is determined by (2.1125).
Equations (2.1127) then have y as the only variable and a sequence of
roots may be computed numerically. To each root y there corresponds a
value of
<fft
according to (2.1126). Thus, for the given
co/co
a
we have a set
of values of
gb.
The process is repeated for the same c and for a sufficient
number of values of
co/co
a
to enable curves to be drawn. The results of
such a computation for v = 1/3 are shown in Fig.
2.111.
The full lines
are for the symmetric modes and the dashed lines are for the
antisymmetric modes. The intersection points are the same as the
intersection points in Fig.
2.091.
Each such point is identified in Fig.
2.111 by a pair of numbers the first of which is the value of m and the
second the value of n.
As may be seen, from Fig.
2.101,
the nearly horizontal portions of
the curves in Fig. 2.111 are due to the coupling of the equivoluminal
