Mench M.M. Fuel Cell Engines

Подождите немного. Документ загружается.

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

230 Transport in Fuel Cell Systems

Table 5.11 Henry’s Law Constants (atm · cm

/mol) as a Function of Temperature for Gases into

Water

Species 290 K 300 K 310 K 320 K 330 K 340 K

66 94 119 149 176 —

2079 2462 2845 3119 3338 3557

3666 3940 4104 4159 4213 4159

CO 2791 3283 3666 4049 4378 4596

Air 3393 4049 4596 5034 5417 5691

4159 4870 5527 6019 6457 6785

Source: Data adapted from [29].

Notice that the oxygen constant increases with temperature, which means the oxygen

concentration in the liquid decreases with temperature. This is why the water temperature in

a ﬁshbowl is so critical. If the water temperature is too high, too little oxygen concentration

is available for the ﬁsh and they will suffocate. The oxygen into water can be curve-ﬁt using

thedatafromTable5.11as

−water



atm · cm

mol



= exp



−1048

+ 11.29



(5.65)

For PEFC electrolyte the oxygen and hydrogen ﬂux is very important in the membrane.

Henry’s constant (in slightly different units, so that the solid-phase concentration and not

the mole fraction is known) has been correlated [30]:

−Naﬁon



atm · cm

mol



= exp



−666.0

+ 14.1



(5.66)

For hydrogen into Naﬁon, Henry’s constant is relatively constant in temperature, as mea-

sured for Naﬁon 120 polymer at 25

◦

C[30]:

−Naﬁon

(atm · cm

/mol) = 4.5 × 10

(5.67)

Once the reactant is across the phase boundary, it diffuses in the new phase toward the

catalyst.

Example 5.11 Crossover through Solid Electrolyte Calculate the molar ﬂow rate of

hydrogen crossover into and through a 51-µm-thick Naﬁon electrolyte. The anode is op-

erating at 353 K and 1 atm pressure with a mole fraction of 0.6 hydrogen. Determine the

mass transfer limiting hydrogen crossover current density from this value.

SOLUTION First you can solve for the concentration at the anode side in the Naﬁon:

/membrane side



mol



i,gas side

gas side

H(T )

0.6 × 1

4.5 × 10



mol



= 1.33 × 10

−5

mol/cm

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

5.3 Gas-Phase Mass Transport 231

Now this concentration must diffuse toward the cathode, which, presumably, has zero

concentration of hydrogen. From Fick’s law and Eq. (5.61) at 353 K

=−D

−Naﬁon

∂C

∂x

= 4.1 × 10

−3

exp



−2602



1.33 × 10

−5

51 × 10

−4



mol

· s



= 6.74 × 10

mol/cm

· s

As discussed in Chapter 4, fuel crossover is typically measured as an effective crossover

current density. The mass transfer limiting hydrogen crossover current density is the maxi-

mum current density that could be achieved if all of the hydrogen crossover were used as

fuel. This corresponds to a limiting current density of

= 6.74 × 10

mol/s ⇒ (6.74 × 10

)nF = i

limiting

= 1.31 mA/cm

COMMENTS: Typical PEFCs have a range of about 1–10 mA/cm

hydrogen crossover.

Also, there is some additional resistance from the ionomer in the catalyst layers which

was not accounted for here. We could account for this by assuming an equivalent ionomer

thickness in the catalyst layer based on the ionomer content.

Example 5.12 Mass Transfer Limiting Current Density with Film Resistances for PEFC

In Example 5.8, we solved for the mass transport limiting current density of an electrode

based on the resistance to gas-phase transport only. In the PEFC and some other fuel cells,

a thin layer of liquid or ionomer may cover portions of the catalyst surface, resulting in an

additional ﬁlm resistance. Symbolically show this situation to form a more precise model of

mass transfer limited current density at an electrode. Ignore convective effects and Knudsen

diffusion in this problem.

SOLUTION The ﬁlm resistance acts in series with the resistance in the diffusion media

and catalyst layer. We can start by showing the resistance ﬂow through the diffusion media,

into the catalyst layer in the gas phase, and into an ionomer and liquid ﬁlms:

=−nFD

eff,DM

∞

− C

=−nFD

eff,CL

− C

−

=−nFD

R,water

− C

−

=−nFD

R,NF

− C

which represents four equations and eight unknowns: C

∞

, C

−

, C

−

, C

, and i

. One of the remaining necessary four equations comes from the ideal gas

law:

∞

Another unknown is eliminated under limiting conditions as C

goes to zero at i = i

Another two come from Henry’s law, to step across the gas–liquid phase boundary and the

gas–Naﬁon phase boundary:

i,liquid/membrane side



mol



i,gas side

gas side

H(T )

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

232 Transport in Fuel Cell Systems

−



mol



(T )

and C



mol



(T )

A ﬁnal equation can be determined by substitution of one of the diffusion equations into

another to substitute for an unknown. Any can be chosen. For example,

−nFD

R,water

− C

−

=−nFD

R,NF

− C

⇒

R,water

R,NF



− C

−



= C

The resulting calculations are very tedious by hand but yield substantially reduced limiting

current density values that are closer to those actually experienced.

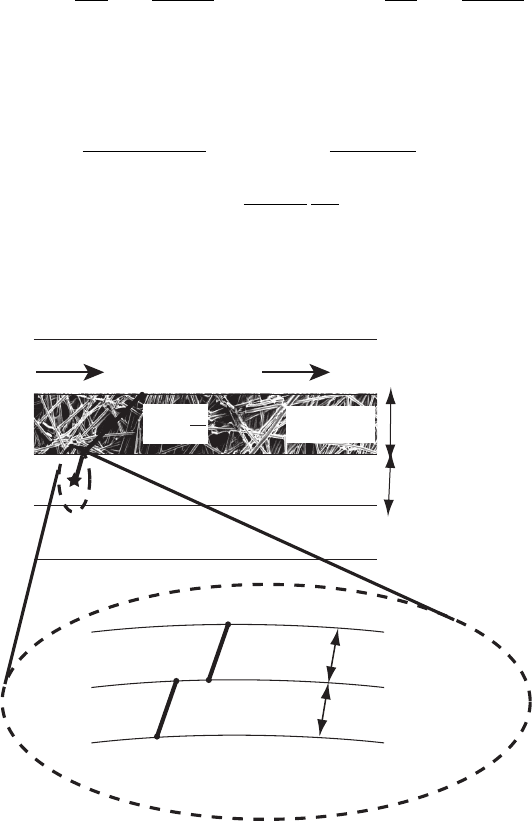
Reactant Flow

Electrolyte

Catalyst Layer

Diffusion

Media (DM)

Flow Channel

= −

∂

H2O

Water Layer

Ionomer Layer

Catalyst

= 0

δNF

δW

δDM

δCL

COMMENTS: We could also have added the catalyst layer diffusion resistance. This

model, while serving as a useful qualitative tool, is not precise, simply because we have

no idea of the thickness of any layer of liquid water of ionomer locally in the electrode

structures. Also, some local ﬂooding simply turns off the current in this location by these

effects, but this only means areas which are ﬂooding have reduced performance. Other

areas in the fuel cell may not be ﬂooded, so that the net effect of the ﬂooding is actually

to reduce the electrochemically active surface area, which is an approach taken by modely

including ﬂooding, discussed later in this chapter.

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

5.4 Single-Phase Flow in Channels 233

Table 5.12 Summary of Orders of Magnitude of Diffusion

Coefﬁcients for Various Modes of Transport

Mode of Diffusion Order (cm

/s)

Bulk gas in gas O(0.1)

Dissolved gas in liquid O(10

−5

)

Gas into liquid O(10

−4

)

Knudsen O(10

−3

–0) f (pore size)

in Naﬁon O(10

−6

) at 353 K

Surface diffusion O(10

−5

–10

−7

)

5.3.7 Surface Diffusion

At the catalyst–electrolyte surface we have gas-phase diffusion, and there can also be

additional surface diffusion. In surface diffusion, gas molecules physically or chemically

absorb onto a solid surface. If it is physical absorption, the species are highly mobile. If

it is chemisorption and the molecule is more strongly bonded to the speciﬁc site, species

are not directly mobile but can move via a hopping mechanism. Surface diffusion rates can

be measured by direct measurement of the ﬂux of a nonreacting gas across the material

surface. The difference between the measured diffusion and predicted Knudsen diffusion is

calculated to be the surface diffusion component. Values of the surface diffusion coefﬁcient

)are∼10

−5

/s in solids and liquids, but these vary widely since surface interaction

is involved. Also, D

is a strong function of temperature and surface concentration. Surface

diffusion adds to the overall diffusion but is typically less than one-half of the Knudsen

component and so has been mostly neglected in fuel cell analysis.

5.3.8 Diffusion Summary

To help summarize the concepts covered on diffusion transport, Table 5.12 is provided.

Tortuosity and porosity further modify the gas–gas diffusivities if ﬂow is through a porous

media. Pressure, temperature, and other parametric dependencies vary between the modes.

5.4 SINGLE-PHASE FLOW IN CHANNELS

It is the goal of reactant ﬂow manifold and channel design in PEFCs to provide excellent

access to the catalyst while suffering the lowest possible pressure drop. Since parasitic

blowers or fans typically must power air and possibly fuel through the fuel cell, the pressure

loss is directly related to the power output of the fuel cell. For some higher pressure systems,

the power consumed by pumps and blowers can reach 30% of the power generated by the

fuel cell.

5.4.1 Pressure Drop

One of the most important aspects of fuel cell design is the reactant ﬂow through the

manifolds and ﬂow ﬁeld, because parasitic losses from driving the ﬂow through the cell can

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

234 Transport in Fuel Cell Systems

Electrolyte

Catalyst layer

Diffusion media

Flow channel

Consumption flow

to catalyst layer

Product flow uptake

Frictional pressure drop

∆P

Slug

blockage

Droplet

Figure 5.17 Schematic of pressure drop and mass exchange in the ﬂow channel of fuel cell.

be the dominant. The pressure change in ﬂow channels is a result of several phenomena, as

illustrated in Figure 5.17:

1. Frictional Losses This is the most easily recognized source of pressure loss in the

ﬂow channel. In all channels, the viscous losses retard the ﬂow so that a pressure

drop per length of the channel is required to maintain a given ﬂow rate. In the limit

of high stoichiometry, this loss dominates pressure drop.

2. Consumption of Reactant The consumption of reactant reduces the pressure in the

fuel cell ﬂow channel. In the limit, with neat hydrogen and a high fuel utilization,

the ﬂow at the exit can be near a vacuum state.

3. Production of Species Product species are added to the ﬂow in the channel. Water

vapor generated by reaction will enter the vapor state until the saturation limit.

4. Two-Phase Flow or Blockages In low-temperature PEFCs, liquid water droplets

can accumulate in a sporadic and nonuniform fashion and block ﬂow channels,

increasing pressure drop. In all fuel cells, partial channel blockage from materials

in the ﬂow channel (e.g., in PEFCs, the gas diffusion media can sometimes sag into

the channel) will result in increased pressure drop.

Frictional Losses The basic dimensionless parameter representing the ratio of momentum

to vicious losses is the Reynolds number Re:

Re =

ρVd

(5.68)

where V is the velocity, ρ is the ﬂuid density, µ is the ﬂuid viscosity, and d

is the hydraulic

diameter, deﬁned as

(5.69)

where A

is the cross-sectional area of ﬂow and P

is the length of the perimeter wet by

ﬂow. For round channels, this reduces to simply the channel diameter, where for rectangular

In this text, it is assumed the reader has a basic background in ﬂuid mechanics. The reader is referred to

undergraduate texts in ﬂuid mechanics, such as F. M. White, Fluid Mechanics, 5th ed., McGraw Hill, New York,

2003.

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

5.4 Single-Phase Flow in Channels 235

(a)

(b)

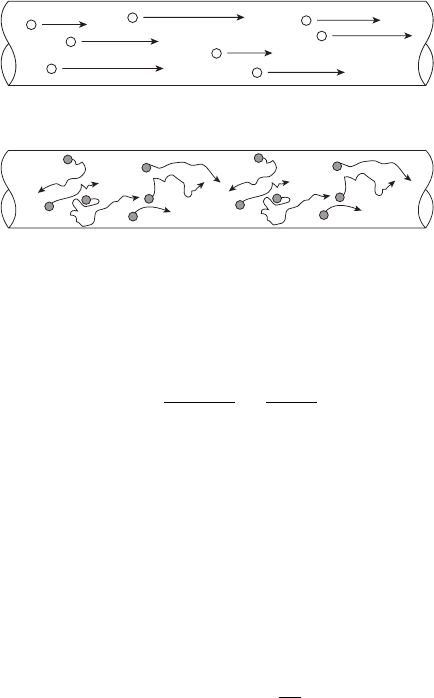
Figure 5.18 Illustration of individual particle ﬂow trajectories in (a) laminar and (b) turbulent ﬂow.

channels [2] of width w and height h

4wh

2w + 2h

2wh

w + h

(5.70)

For Re < 3000 in an internal channel, the ﬂow is typically laminar, a condition characterized

by uniform streamlines and locally steady ﬂow. For an internal channel with Re > 3000,

the ﬂow transitions to turbulent ﬂow, characterized by local velocity proﬁle ﬂuctuations

and enhanced mixing, as illustrated in Figure 5.18. The ﬂow in a vast majority of fuel cells

is highly laminar, which greatly simpliﬁes many important calculations.

Physically, the cause of the pressure drop for internal ﬂow is the viscous interaction

with the wall, where there is a no-slip condition (V

wall

= V

ﬂuid

). The one-dimensional shear

force at the wall for a Newtonian ﬂuid is proportional to the ﬂuid strain and is related to

ﬂuid viscosity:

wall

= τ A = Aµ

(5.71)

where A is the surface area of contact between the ﬂuid and the wall and du/dy is the slope

of the change in velocity with distance from the wall. To overcome the shear restraining

force imposed by the channel walls and the viscous nature of the ﬂuid, the ﬂow will

experience a pressure drop along the channel.

In open-ﬁeld ﬂow, such as in the atmosphere, there are no restraining walls or objects

that impose the no-slip condition. When ﬂow from an open channel or ﬂow manifold

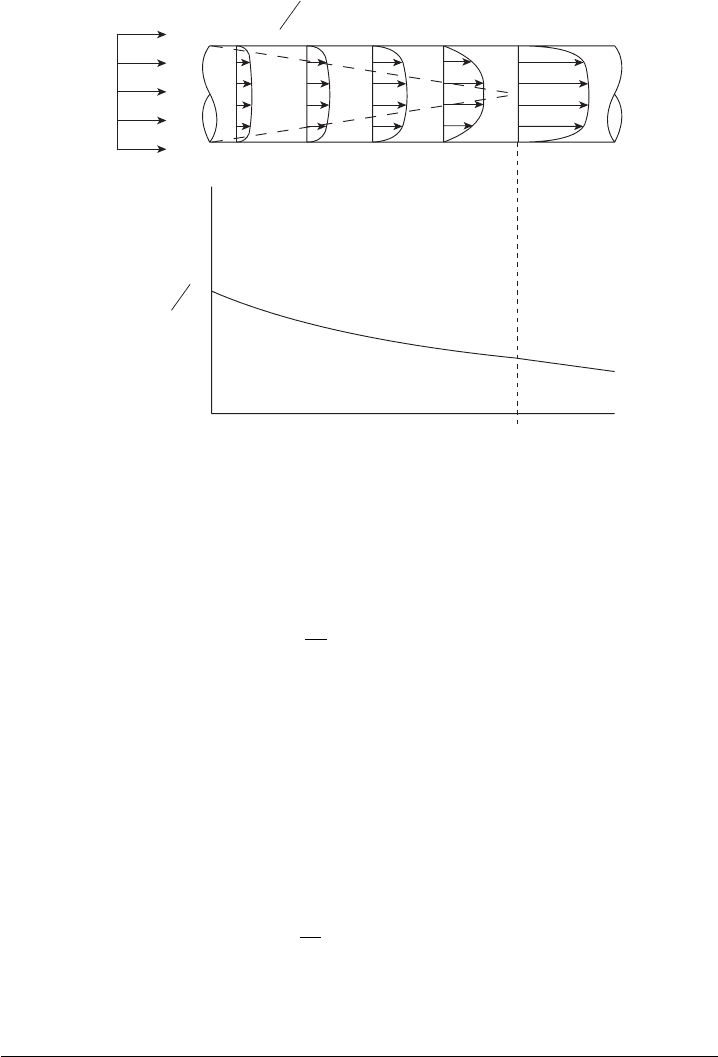
enters a channel, the ﬂow ﬁeld develops along an entry length. The internal ﬂow proﬁle for

developing ﬂow is shown in Figure 5.19. At ﬁrst, the ﬂow entering the conﬁned channel is

undeveloped, and the ﬂow proﬁle still varies along the axial direction. Because of the no-slip

condition, the particles at the wall must have the same velocity as the wall, which sets up a

very steep du/dy proﬁle within the developing boundary layer. Hence, in this developing

entrance region, the pressure drop per unit length is higher than in the developed section

of the channel. Further on down the channel, the boundary layers grow and eventually

converge until the ﬂow proﬁle no longer changes in the axial direction. The distance from

the entrance of the channel to the point where the internal ﬂow proﬁle becomes independent

of the axial location is termed the entrance length (L

) and is characterized by a higher

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

236 Transport in Fuel Cell Systems

at wall is very steep

∆

from manifold

∞

Figure 5.19 Developing ﬂow proﬁle in a channel. The shear forces that accumulate along the wall

result in a pressure drop along the channel that must be provided for by the reactant ﬂow system.

pressure drop and mixing per unit length compared to the fully developed portion of the

channel. The entrance length for laminar internal ﬂow can be estimated from

≈ 0.06 Re (5.72)

With a critical Reynolds number for transition to turbulence around 3000,

the max-

imum length of developing ﬂow is about 180 diameters. A typical fuel cell ﬂow channel

hydraulic diameter is around 1 mm, which means the ﬂow will be developing (and expe-

riencing greater pressure loss and enhanced mixing) for up to 18 cm of the ﬂow channel

length. When the ﬂow experiences a sudden turn, or switchback, recirculation zones and

additional development length after the turn occur. As a result of entrance and ﬂow ﬁeld

effects, the actual frictional pressure drop in a fuel cell ﬂow channel is typically greater

than predicted by fully developed ﬂow theory.

For turbulent ﬂow, the following relationship for entrance length is used:

≈ 4.4Re

1/6

(5.73)

The entrance length for turbulent ﬂow can be technically inﬁnite if Re is inﬁnite but is

typically less than that for laminar ﬂow because of the enhanced mixing. Additionally,

Many authors refer to the critical Reynolds number (Re

crit

) as 2300. The transition from laminar to turbulent

proﬁles can actually occur over a relatively broad range and is a function of the channel geometry and surface

roughness. In this text, Re

crit

= 3000 is used as a general guideline.

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

5.4 Single-Phase Flow in Channels 237

(a)

(b)

Figure 5.20 Laminar and turbulent proﬁle boundaries: (a) fully developed laminar proﬁle; (b) fully

developed turbulent proﬁle. Notice the turbulent du/dy proﬁle is much steeper, leading to a higher

pressure drop per unit length via Eq. (5.71).

the initial and fully developed ﬂow proﬁles are more similar (see Figure 5.20). For a very

turbulent Reynolds number of 1,000,000, the entrance length is still only 44 hydraulic

diameters. While this may suggest that reduced pressure drop could be obtained in fuel

cells if the ﬂow was turbulent, the overall pressure drop (and parasitic losses required to

obtain turbulent ﬂow velocity) is far greater for turbulent ﬂow due to the higher du/dy

proﬁle at the wall for a turbulent-ﬂow proﬁle.

From a force balance on the internal ﬂow surface and conservation of energy, we can

derive the following equation relating frictional pressure drop per unit length in a closed

channel for fully developed ﬂow:

P

= f

ρV

(5.74)

where f is the Darcy friction factor. As discussed, the velocity V is not constant in a fuel

cell due to consumption and other factors and is a function of location along the channel.

For laminar ﬂow, f can be determined from ﬂow theory [2]:

lam

(5.75)

Therefore, for laminar ﬂow, the pressure drop is proportional to the velocity. For laminar,

fully developed ﬂow, the pressure drop can be modeled as Hagen–Pouiseville ﬂow:

P

= 32

µV

(laminar ﬂow) (5.76)

where V is the velocity and P is the pressure drop through the pore. For turbulent ﬂow,

much experimental data have been correlated, and the following equation by Colebrook

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

238 Transport in Fuel Cell Systems

[31] is the most commonly applied:

turb

=−2.0log



ε/d

3.7

2.51

Re f

1/2

turb



(5.77)

This implicit equation for f can be easily solved computationally but is rarely needed in

fuel cells since the ﬂow is typically laminar in nature. Here ε is the wall roughness height,

which is a function of the surface of the channel walls.

For advanced modeling purposes, the addition of minor loss, ﬂow ﬁeld switchback,

and manifolding effects can be approached analytically. In practice, however, the actual

pressure drop in an cell or stack is very difﬁcult to predict with high precision due to

the effects of entry length, local turbulence, additional minor losses from switchback,

consumption, uptake and other effects. Additionally, in PEFCs and AFCs with a porous

diffusion media, there can be unintentional convective ﬂow under the channels, which

reduces overall pressure drop, as discussed in Chapter 6. Therefore, a good starting point is

to assume the frictional pressure drop dominates (which has been found to be true in certain

PEFCs [32]) and calculate an expected loss from Eq. (5.76). For a particular fuel cell, the

pressure drop can be correlated as a function of entrance velocity, since this is relatively

easy experimental data to obtain.

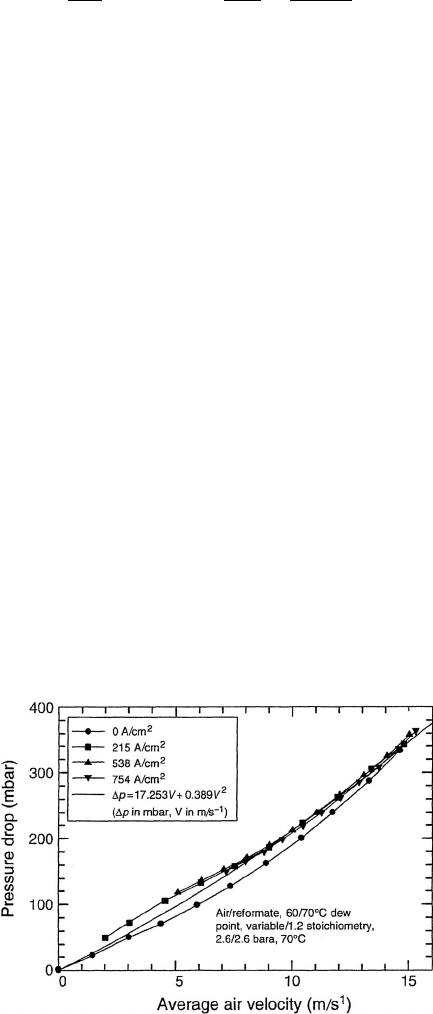
An example is shown in Figure 5.21 where the general linearity indicates pressure

drop is dominated by laminar frictional losses. For the particular stack in Figure 5.21

(and in fact many stacks), the pressure drop can be correlated as a polynomial function of

velocity, which globally accounts for the laminar (V dependence) and turbulent/minor loss

dependency (∼V

dependence):

P = aV + bV

(5.78)

where a and b are empirically derived constants for a particular fuel cell and are by no

means uniform for different cell, manifold, stack designs, or operating conditions.

Consumption of Reactant Due to consumption, the uptake of product or moisture, the

molar ﬂow rate (and velocity) of the reactant mixture in the channel is not a constant and

Figure 5.21 Measured and correlated pressure drop in a Ballard fuel cell as a function of velocity.

(From Ref. [32] with permission.)

c05 JWPR067-Mench January 23, 2008 18:58 Char Count=

5.5 Multiphase Mass Transport in Channels and Porous Media 239

varies with location along the channel length. At the inlet, we can show that

mix

(

inlet

)

= QA =

(5.79)

where Q is the volume ﬂow rate, y

is the mole fraction of the reacting species, and I is

the current. For a constant current across the electrode surface, we can show that along the

ﬂow path the initial reactant gas is reduced by consumption:

mix

(x) =



−



(5.80)

where x is the length along the channel and L is the total length of the channel. Consumption

or uptake of species into the ﬂow results in a changing ﬂow velocity, but the pressure is

always highest at the input and decreases along the ﬂow channel. Along the channel, with

a net uptake of mass considered (e.g., water vapor uptake), conservation of mass yields

mix

(x) =



−



uptake

(x) (5.81)

where

uptake

water vapor and other gas-phase species production combined and the current

density is assumed to be uniform across the fuel cell surface. At the exit of the fuel cell

under the same assumptions, we can show that

mix,out

mix,in

−

consumed

uptake

−



− 1



uptake

(5.82)

The fuel cell polarization model developed in Chapter 4 can be extended to multiple

dimensions, and the current density distribution can also be modeled based on concepts

in this book. In the limit of high stoichiometry, Eq. (5.81) becomes invariant in x, and the

reactant concentration is constant in the ﬂow channel. At low stoichiometry, however, the

reactant concentration decreases throughout the channel length and can result in greatly

decreased current density at the exit locations [33]. In general, almost nothing in a fuel cell

is actually homogeneous. The current, temperature, and species vary throughout the fuel

cell in all directions for almost every fuel cell design. In many cases, the variation in many

parameters such as temperature can be used to an advantage and is a critical component in

design optimization.

5.5 MULTIPHASE MASS TRANSPORT IN CHANNELS

AND POROUS MEDIA

5.5.1 Multiphase Flow in Gas Channels

Mixed liquid/gas-phase ﬂow can occur in direct alcohol and hydrogen polymer electrolyte

fuel cells. For the hydrogen fuel cell, liquid water can build up in either the anode or

This section may be omitted without loss of continuity providing detailed analysis of PEFC ﬂooding covered in

Chapter 6 is also omitted.