Mench M.M. Fuel Cell Engines
Подождите немного. Документ загружается.


c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
100 Thermodynamics of Fuel Cell Systems
Relating Temperature Change to Maximum Efficiency Recall the expression for maxi-
mum thermodynamic efficiency of a fuel cell, and noting that the enthalpy of reaction for
a galvanic (exothermic) process is negative, we can show that
η
t
= 1 −
T S
H
= 1 −
T S
negative
⇒ sign of η
t
∝ 1 + T S
or
η
t
(T ) ∝ S (3.105)
That is, since the absolute temperature is always positive, the dependence of the maximum
efficiency varies with temperature, according to the sign of the change in entropy. There
are three possibilities, as depicted in Figure 3.13:
1. The entropy change is quite small, and there is almost no variation of maximum
thermodynamic efficiency with temperature.
2. The entropy change is significant, and the net change is positive (this would corre-
spond to the presence of more thermodynamic microstates in the product compared
to the reactant). In this case the maximum thermodynamic efficiency would increase
with temperature.
3. The entropy change is significant, and the net change is negative (this would corre-
spond to the presence of fewer thermodynamic microstates in the product compared
to the reactant). In this case the maximum thermodynamic efficiency would decrease
with temperature, as in a hydrogen fuel cell.
The key to predicting the qualitative relationship between temperature and the maxi-
mum thermal efficiency is in the evaluation of the entropy change (number of microstates)
between the products and reactants. Comparing the potential microstates available to a solid
or liquid, a low-density gas-phase species has much greater entropy. Therefore, the num-
ber of microstates varies directly with the number of moles of gas-phase species, and the
contribution of liquid- and solid-phase species is comparatively insignificant. This makes
sense, because for an ideal gas the volume of a mole of gas at a given temperature and
pressure is constant and thus a lower number of moles of gas results in a lower volume
of gas and a correspondingly lower entropy. Consider the hydrogen fuel cell overall redox
reaction:
H
2
+
1
2
O
2
1.5molgas
−−−−−−→ H
2
O
lorg
1mol
if gas phase
or 0 mol
if liquid
The S is proportional to the moles of gas-phase species of products and reactants:
S ∝ (n
g,P
− n
g,R
) (3.106)
Here, S is negative, because the product has fewer moles of gas than the reactants. From
Eq. (3.105), we can qualitatively predict that the maximum thermodynamic efficiency will

c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
3.6 Thermodynamic Efficiency of a Fuel Cell 101
decrease with increasing operation temperature for this fuel cell, which correctly predicts
the actual calculated relationship shown in Figure 3.13.
As a second example, consider the direct methane fuel cell overall reaction:
(CH
4
)
g
+ 2O
2
3.0molgas
−−−−→CO
2
+ 2H
2
O
g
3.0molgas
In this case S is nearly zero, since the moles of gas-phase species of reactants and products
are equivalent. In this case, the entropy may be slightly positive or negative depending on
the difference between the molecular structures of the products and reactants, but we can
qualitatively predict from Eq. (3.105) that the maximum thermodynamic efficiency will be
nearly invariant with temperature, which would correspond to the straight line shown in
Figure 3.13.
Finally, consider the direct formic acid fuel cell overall reaction:
HCOOH
l
+
1
2
O
2
0.5molgas
−−−−→CO
2
+ H
2
O
g
2.0molgas
In this case, S is positive, since the moles of gas-phase species of the products are
greater than the reactants. We can qualitatively predict that the maximum thermodynamic
efficiency will actually increase with temperature, which would correspond to the rising
line in Figure 3.13. At a high enough temperature, we expect the maximum thermodynamic
efficiency to be greater than 100%! How can this possibly be true? When the theoretical
maximum thermodynamic efficiency is greater than 100%, it means that, thermal energy is
taken from the environment surrounding the fuel cell and converted into electrical potential.
Although use of ambient heat to generate power with an efficiency greater than 100% seems
like an amazing possibility, it is of course not realistic in practice. Removal of heat from
the environment at a lower temperature than the fuel cell and transforming this heat into
electrical energy would be a violation of the second law by pumping heat from a low-
temperature to a high-temperature reservoir without doing work. This process can work if
the ambient temperature is higher than the fuel cell, but then the actual efficiency must then
include the energy required to increase the ambient temperature above that of the fuel cell.
Le Chatelier’s Principle: Open-Circuit Voltage Dependence on Temperature and Pres-
sure Le Chatelier’s principle can be stated a follows: “Any change in one of the variables
that determines the state of a system in equilibrium causes a shift in the position of equilib-
rium in a direction that tends to counteract the change in the variable under consideration”
[7]. There is a deep meaning beyond the field of fuel cells to this statement, and it can be
considered an incarnation of nature’s balance. In the context of the fuel cell, Le Chatelier’s
principle can be applied to understand and predict the effect of a change in temperature or
pressure on voltage. Nature tries to balance out new stresses to the system to a change in
equilibrium. If the temperature or pressure is increased, the new equilibrium will be shifted
to counteract the effect of the temperature or pressure change. Consider the hydrogen fuel
cell global reaction:
H
2
+
1
2
O
2
1.5molgas
−−−−→ H
2
O
g
1mol
gas phase
c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
102 Thermodynamics of Fuel Cell Systems
1. If pressure of the reactants increases, nature will work to relieve the new stress,
favoring the forward reaction toward hydrogen oxidation, because this will reduce
the number of gas-phase moles to counteract the increase in pressure. The effect on
voltage would be to shift the reaction toward the products, which would increase
the voltage (i.e., potential for reaction) at a given condition.
2. If the pressure of the reactants decreases, nature will work to relieve the new
stress, favoring the reverse reaction toward reactants, to counteract the decrease in
pressure. The effect on voltage would be to shift the reaction toward the reactants,
which would decrease the voltage at a given condition.
Later in this chapter we will see the mathematical reasoning for this and develop the Nernst
equation to predict the expected OCV as a function of temperature and pressure. Using
this principle, however, we can already qualitatively predict the functional dependence of
temperature and pressure on the OCV.
Heating Value For reactions involving water as a product, there is a choice in the calcu-
lation of thermodynamic voltages between a high heating value (HHV) and a low heating
value (LHV), defined as follows for a given reaction:
High Heating Value: It is assumed all the product water is in the liquid phase.
Low Heating Value: It is assumed all the product water is in the gas phase.
Note that calculation based on HHV or LHV is an arbitrary decision and does not necessarily
correspond to the actual physical state of the product water at the fuel cell electrode.The
terms HHV and LHV are used in combustion calculations as well, where the product water
is nearly always in the gas phase. The difference between the two values is proportional
to the latent heat of vaporization of the liquid. Use of the LHV (gas-phase vapor product)
will result in a lower calculated thermal voltage, since some energy is used for the latent
heat of vaporization of the liquid. In practice, the LHV is completely appropriate for high-
temperature fuel cells, but the HHV is also commonly used. An important point regarding
low-temperature fuel cells that is often confusing is that the choice of HHV or LHV is
arbitrary and 100
◦
C is not a point of demarcation between the two. Often 100
◦
C is thought
of as a natural boundary between the HHV and LHV because it is the phase change
temperature of water at 1 atm pressure. The delineation between liquid and gas, however,
is more complex and is related to the local vapor pressure and total pressure, as discussed
in Section 3.5.
Example 3.9 Calculation of Efficiencies and Trends in OCV
(a) Use Le Chatelier’s principle to predict if the maximum possible voltage of a direct
liquid methanol fuel cell, E
◦
, will increase or decrease with temperature. Assume
a gas-phase product water product.
(b) Calculate the maximum HHV and LHV cell voltage E
◦
for a methanol–air fuel
cell. Assume a gas-phase water product and all constituents are at 1 atm, 298 K.
(c) Predict if the maximum possible thermodynamic efficiency η
th
of a hydrogen fuel
cell will increase or decrease with temperature.

c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
3.6 Thermodynamic Efficiency of a Fuel Cell 103
(d) Prove your result in part (c) by calculating the maximum thermodynamic efficiency
of a hydrogen fuel cell at 298 and 1000 K. Assume LHV and all constituents are
at 1 atm.
(e) What do you notice about the voltages calculated for a hydrogen cell compared to
the methanol fuel cell?
SOLUTION (a) Consider the direct methanol fuel cell overall reaction:
CH
3
OH
l
+
3
2
O
2
→ 2H
2
O
g
+ CO
2
Since there is 3 mol of gas in the products, compared to 1.5 mol in the reactants, the entropy
increases with the forward reaction. Even at 500 K, where the methanol is in vapor form,
there are still more moles of gas phase in the products than in the reactants. If temperature
is increased, we expect the reverse reaction to be more favored, reducing OCV.
(b)
G = products − reactants =
n
i=1
n
i
¯
g
P,i
−
m
j=1
n
j
¯
g
R, j
H = products − reactants =
n
i=1
n
i
¯
h
P,i
−
m
j=1
n
j
¯
h
R, j
For the HHV
G = products − reactants =
n
i=1
n
i
¯
g
P,i
−
m
j=1
n
j
¯
g
R, j
Since we are at 1 atm for all constituents, 298 K, only the Gibbs function of formation
remains, which we can get from Table 3.3:
G = products − reactants = (2 mol)(−237,180 J/mol) + (1 mol)(−394,380 J/mol)
− (1 mol)(−166,290 J/mol) −
3
2
mol
(0 J/mol) =−702,450 J
Maximum OCV = E
◦
(298):
E
◦
(298) =−
G
nF
=
−702,450
6 × 96,485
= 1.21 V
For the LHV (gas-phase water product)
G = products − reactants = (2 mol)(−228,500 J/mol) + (1 mol)(−394,380 J/mol)
− (1 mol)(−166,290 J/mol) −
3
2
mol
(0 J/mol) =−685,090 J
Maximum OCV = E
◦
(298):
E
◦
=−
G
nF
=
−685,090
6 × 96,485
= 1.18 V
So 0.03 V of potential is used to vaporize the water in the product at these conditions.

c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
104 Thermodynamics of Fuel Cell Systems
(c)
H
2
+
1
2
O
2
1.5molgas
−−−−→ H
2
O
g
1mol
gas phase
The maximum possible thermodynamic efficiency η
th
of a hydrogen fuel cell will de-
crease with temperature, because the entropy change is negative (for both HHV and LHV
assumptions), and
η
t
(T ) ∝ S
(d) Prove your result in part (c) by calculating the maximum thermodynamic efficiency
of a hydrogen fuel cell at 298 and 1000 K. Assume LHV and all constituents are at 1 atm.
What do you notice about the voltage calculated compared to the methanol fuel cell? The
hydrogen fuel cell overall reaction is
H
2
+
1
2
O
2
→ H
2
O
G = products − reactants =
n
i=1
n
i
¯
g
P,i
−
m
j=1
n
j
¯
g
R, j
H = products − reactants =
n
i=1
n
i
¯
h
P,i
−
m
j=1
n
j
¯
h
R, j
For the gas-phase water product, assuming 1 mol of hydrogen reacts, at 298 K
G = products − reactants = (1 mol)(−228,590 J/mol)
−
1
2
mol
(0 J/mol) − (1 mol)(0 J/mol)
H = products − reactants = (1 mol)(−241,820 J/mol)
−
1
2
mol
(0 J/mol) − (1 mol)(0 J/mol)
Maximum OCV = E
◦
:
E
◦
(298) =−
G
nF
=
−228,590
2 × 96,485
= 1.18 V
Thermal voltage E
◦◦
:
E
◦◦
(298) =−
H
nF
=
−241,820
2 × 96,485
= 1.25 V
Maximum thermodynamic efficiency η
th
:
η
th,max
(298) =
−G/nF
−H/nF
=
1.18 V
1.25 V
= 0.94
At 1000 K
G = 1[
¯
g
◦
f
+
¯
h
s
− T
(
¯
s
1000
−
¯
s
298
)
]
H
2
O
−
1
2
[
¯
g
◦
f
+
¯
h
s
− T
(
¯
s
1000
−
¯
s
298
)
]
O
2
− 1[
¯
g
◦
f
+
¯
h
s
− T
(
¯
s
1000
−
¯
s
298
)
]
H
2
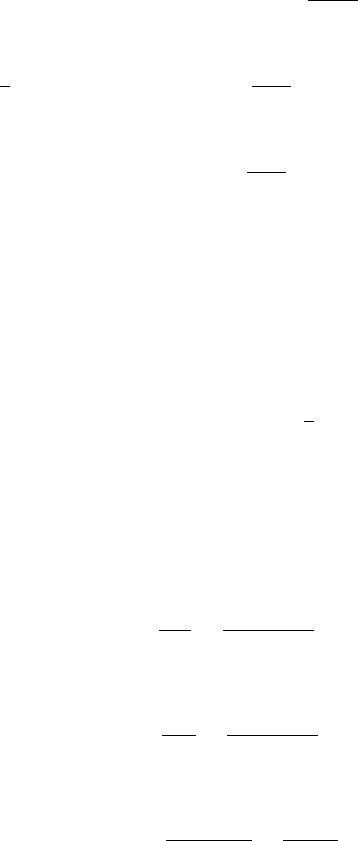
c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
3.6 Thermodynamic Efficiency of a Fuel Cell 105
= 1
−237,180 +
1000
298
¯
c
p,H
2
O
dT − T
1000
298
¯
c
p,H
2
O
T
dT
H
2
O
−
1
2
0 +
1000
298
¯
c
p,O
2
dT − T
1000
298
¯
c
p,O
2
T
dT
O
2
− 1
0 +
1000
298
¯
c
p,H
2
dT − T
1000
298
¯
c
p,H
2
T
dT
H
2
Using the polynomial expressions for the specific heat and an analytical solver on a com-
puter, the above expression can be solved:
G =−223,766 kJ
Similarly, the total enthalpy change can be found:
H = 1
−285,830 +
1000
298
¯
c
p,H
2
O
dT
H
2
O
−
1
2
0 +
1000
298
¯
c
p,O
2
dT
O
2
− 1
0 +
1000
298
¯
c
p,H
2
dT
H
2
=−247,876 kJ
Maximum OCV = E
◦
(1000):
E
◦
(
1000
)
=−
G
nF
=
−228,590
2 × 96,485
= 1.16 V
Thermal voltage E
◦◦
(1000):
E
◦◦
(
1000
)
=−
H
nF
=
−220,876
2 × 96,485
= 1.28 V
Maximum thermodynamic efficiency η
th
:
η
th,max
(
1000
)
=
−G/nF
−H/nF
=
1.16 V
1.28 V
= 0.90
So the maximum thermodynamic efficiency does decrease with temperature, as predicted
in part (c).
(e) The voltages calculated for the methanol- and hydrogen-based fuel cells were
similar, around 1.2 V. This trend is continues despite the choice of fuel. The higher heat of
formation is also accompanied by a greater number of electrons per mole of fuel, n. The
result is that most fuels have a similar range of predicted OCV.
COMMENTS: Note that the actual OCV is also a function of the mole fractions of the
constituents and several other factors, so that the calculated value is only the maximum

c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
106 Thermodynamics of Fuel Cell Systems
potential value for unit activity (i.e., 1 atm of all constituents). Pressure variation also results
in a voltage change, as described in the following section.
3.7 MAXIMUM EXPECTED OPEN-CIRCUIT VOLTAGE:
NERNST VOLTAGE
While the thermal (E
◦◦
) voltage is a function of only temperature, the reversible voltage
(E
◦
) is actually a function of temperature and pressure of the reactants and products. The
Nernst equation is an expression of the maximum possible open-circuit (zero-cell-current)
voltage as a function of temperature and pressure and is an expression of an established
thermodynamic equilibrium. Consider a global redox reaction in a fuel cell:
ν
A
A + ν
B
B ↔ ν
C
C + ν
D
D (3.107)
where the v’s are the stoichiometric coefficients of the balanced electrochemical reaction.
From thermodynamics of systems in equilibrium [1]
G = G
◦
(T ) − R
u
T ln
$
a
ν
A
A
a
ν
B
B
a
ν
C
C
a
ν
D
D
%
(3.108)
where the a’s are the thermodynamic activity coefficients for the reacting species. To convert
to voltage, we can divide by nF:
E(T, P) =
−G
◦
(T )
nF
I
+
R
u
T
nF
ln
$
a
ν
A
A
a
ν
B
B
a
ν
C
C
a
ν
D
D
%
II
(3.109)
where I is the temperature dependence on the voltage evaluated at 1 atm pressure for all
components and II accounts for the thermodynamic activity dependence on the Nernst
voltage. The thermodynamic activity can be calculated or approximated in several ways:
1. For a concentrated solution, the activity coefficient of the species is taken to be
unity.
2. For an ideal gas, a = P
i
/P
◦
, where P
i
is the partial pressure of the species of interest
and P
◦
is the reference pressure, 1 atm (101,325 Pa).
3. For water vapor, the partial pressure of the vapor cannot exceed the saturation
pressure, P
sat
, which is a function of temperature. Thus, the reference pressure is
set to P
sat
, and a = P
v
/P
sat
, which is the relative humidity, RH. This can normally
be considered to be 1.0 in the immediate molecular region of the water-generating
electrode. This is a reasonable assumption because water generation is always at
the catalyst surface, and the activity of water here is 1. Also, the reaction itself is
not limited by the product water concentration at this surface.
4. For a dilute solution, a more complex theory is beyond the scope of this text. This
is treated in advanced electrochemistry texts such as [8].

c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
3.7 Maximum Expected Open-Circuit Voltage: Nernst Voltage 107
For an ideal gas reaction mixture, we can substitute the partial pressures for the activities
in Eq. (3.109):
E(T, P) =
−G
◦
(T )
nF
+
R
u
T
nF
ln
&
(
P
A
/P
◦
)
ν
A
(
P
B
/P
◦
)
ν
B
(
P
C
/P
◦
)
ν
C
(
P
D
/P
◦
)
ν
D
'
(3.110)
where the partial pressures are evaluated at the particular electrode where the reaction
involving the species occurs. Using this expression, we can solve for the expected maximum
(Nernst) voltage for a given fuel cell reaction. Two important points are as follows:
1. The Nernst equation is a result of the equilibrium established at the electrode
surfaces. A significant gradient can exist between the concentration of a species in
the channel of a fuel cell and the electrode, especially under high-current-density
conditions, which cannot be considered a true thermodynamic equilibrium situation
anyway.
2. Only species directly involved in the electrochemical reaction of Eq. (3.107) are
represented directly in the activity terms of Eq. (3.109). Species not participating in
the electrochemical charge transfer reaction only indirectly alter the voltage through
the species mole fractions of the participating species.
To solve problems using the Nernst equation, the following steps should be taken:
Step 1: Write down the Nernst equation (3.109) in symbols.
Step 2: Determine the number of electrons released per mole of fuel oxidized (n); then
determine the stoichiometric coefficients (ν’s) of the balanced overall cell redox
reaction equation.
Step 3: Determine the activities of all reactants/products and insert into Eq. (3.109).
Step 4: Reduce as needed for convenience to solve.
Example 3.10 Nernst Equation for Hydrogen Air Fuel Cell Given a hydrogen air fuel
cell operating at 353 K. Solve for the expected LHV open-circuit voltage if the hydrogen
and water vapor mole fractions in the anode are 0.8 and 0.2, respectively, and the oxygen,
nitrogen, and water vapor mole fractions in the cathode are 0.15, 0.75, and 0.1, respectively.
The cathode and anode pressures are 3 and 2 atm, respectively.
SOLUTION For an H
2
+
1
2
O
2
→ H
2
O cell, the Nernst equation can be written as
E(T, P) =
−G
◦
(T )
nF
+
R
u
T
nF
ln
&
a
H
2
(a
O
2
)
1/2
a
H
2
O
'
Substituting in the proper activity coefficients for the ideal gases, we can reduce this to
E(T, P) = E
◦
(T ) +
R
u
T
2F
ln
(y
H
2
P
anode
/P
◦
)(y
O
2
P
cathode
/P
◦
)
1/2
y
H
2
O
P
cathode
/P
sat
(T )
The first term on the right, the standard voltage E
◦
(T), can be determined as
E
◦
(T ) =
−G
◦
(T )
nF
=−
H(T ) − T S(T )
nF

c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
108 Thermodynamics of Fuel Cell Systems
Recall
H
P–R
=
n
i=1
n
i
¯
h
i
P
−
m
j=1
n
j
¯
h
j
R
For the reacting mixture, we can write
H
P–R
=
¯
h
◦
f,H
2
O
+
T =353
T
ref
=298
¯
c
P,H
2
O
(T ) dT
H
2
O
−
1
2
¯
h
◦
f,O
2
+
T =353
T
ref
=298
¯
c
p,o
2
(T ) dT
O
2
−
¯
h
◦
f,H
2
+
T =353
T
ref
=298
¯
c
p,H
2
(T ) dT
H
2
We can either directly integrate the specific heat functions or assume constant specific heat.
Since the operating temperature is only about 50 K above the standard temperature, there is
not much error associated with assuming a constant specific heat at an average temperature
of 325 K:
H =
"
¯
h
◦
f,H
2
O
+
¯
c
p,H
2
O,ave
(353 − 298)
#
H
2
O
−
1
2
"
¯
h
◦
f,O
2
+
¯
c
p,O
2
,ave
(353 − 298)
#
O
2
−
"
¯
h
◦
f,H
2
+
¯
c
p,H
2
,ave
(353 − 298)
#
H
2
The heats of formation are available in thermodynamics reference books, and online, Tables
3.2 and 3.3. The average specific heats can be found by using an average temperature of
325 K in Eqs. (3.24), (3.25), and (3.28):
¯
c
p
(
325
)
H
2
,ave
= [3.057 + 2.677 ×10
−3
(325) − 5.810 × 10
−6
(325)
2
+ 5.521 × 10
−9
(325)
3
− 1.812 × 10
−12
(325)
4
]R
u
= 28.9kJ/kmol · K
¯
c
p
(
325
)
O
2
,ave
= [3.626 − 1.878 ×10
−3
(325) + 7.055 × 10
−6
(325)
2
− 6.764 × 10
−9
(325)
3
+ 2.156 × 10
−12
(325)
4
]R
u
= 29.5kJ/kmol · K
¯
c
p
(
325
)
H
2
O,ave
= [4.070 − 1.108 ×10
−3
(325) + 4.152 × 10
−6
(325)
2
− 2.964 × 10
−9
(325)
3
+ 0.807 × 10
−12
(325)
4
]R
u
= 33.7kJ/kmol · K
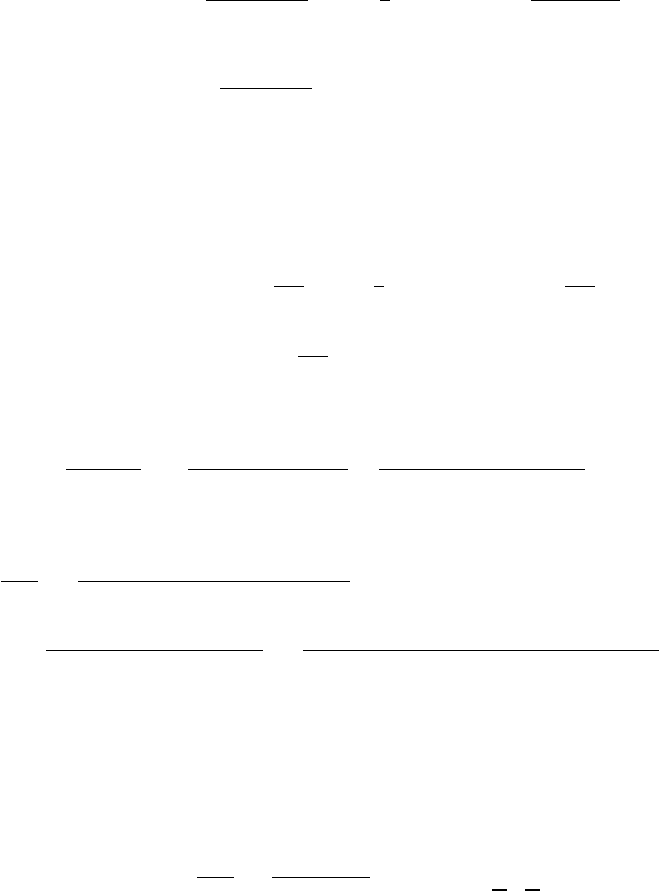
Plugging in all the numbers, we can solve for the change in enthalpy for the reaction for a
LHV solution:
H =
[
−241,820 + 33.7
(
353 − 298
)
]
H
2
O
−
1
2
[
29.5
(
353 − 298
)
]
O
2
−
[
28.9
(
353 − 298
)
]
H
2
=−242,370 kJ
c03 JWPR067-Mench December 18, 2007 1:59 Char Count=
3.7 Maximum Expected Open-Circuit Voltage: Nernst Voltage 109
The change in entropy with respect to temperature can be found:
S =
¯
s
◦
f,H
2
O
+
T =353
T
ref
=298
¯
c
p,H
2
O
(T ) dT
T
H
2
O
−
1
2
¯
s
◦
f,O
2
+
T =353
T
ref
=298
¯
c
p,o
2
(T ) dT
T
O
2
−
¯
s
◦
f,H
2
+
T =353
T
ref
=298
¯
c
p,H
2
(T ) dT
T
H
2
Again, the specific heat terms can be easily integrated, but the error is quite small in
assuming constant specific heats at an average temperature. The entropy of formation at
1 atm pressure is available in thermodynamics reference books and the Appendix. Plugging
in the numbers we find that
S =
188.7 +
¯
c
p,H
2
O,ave
ln
353
298
H
2
O
−
1
2
205.0 +
¯
c
p,O
2
,ave
ln
353
298
O
2
−
130.57 +
¯
c
p,H
2
,ave
ln
353
298
H
2
=−43.61 kJ/K
Now we can solve for the reversible voltage E
◦
:
E
◦
(T ) =
−G(T )
nF
=−
H(T ) − T S(T )
nF
=
−242,370 +353 × 43.61
2 × 96,485
= 1.176 V
The pressure effect is more directly calculated:
R
u
T
2F
ln
(y
H
2
P
anode
/P
◦
)(y
O
2
P
cathode
/P
◦
)
1/2
y
H
2
O
P
cathode
/P
sat
(T )
=
[8.314 J/(mol ·K)](353 K)
(2 eq/mol)(96,485 C/eq)
ln
(0.8 × 2atm/1atm)(0.15 × 3atm/1atm)
1/2
1
= 7.92 mV
where we have assumed the relative humidity at the cathode electrode surface is 1.0 since
water is generated at this location and the reaction is limited not by the amount of water
but by the amount of reactants.
Finally, the maximum expected voltage can be determined:
E(T, P) = E
◦
(T ) +
R
u
T
nF
ln
&
a
H
2
O
(a
O
2
)
1/2
a
H
2
O
'
= 1.176 + 0.00792
3 Partial
pressure
effect
= 1.184 V
COMMENTS: The species chosen in the Nernst equation pressure term are only the
species which participate in the overall electrochemical reaction. For example, the nitrogen
in the cathode is not represented directly since it does not participate in the electrochemical
