Lyons W.C. (ed.). Standard handbook of petroleum and natural gas engineering.2001- Volume 1
Подождите немного. Документ загружается.

Strength of Materials
185
A
=
n(
=
1.767
in.'
Assuming
C
=
1
and applying Equation
2-66
gives
Q
=
(864)(1)(1.767)
=
3214
scfm
From Figure
2-20
the viscosity of the gas is
=
0.0106
cp
and from Equation
2-70
=
2.84
x
lo6
(28.8)( 3,2 14) (0.65)
(0.0106)(2)
R,
=
From Figure
2-24,
using
p
=
0.75,
the value of the discharge coefficient is read as
c
=
1.2.
Now a new estimate of
Q
can be found as
Q=
-
3214=3,857scfm
(3
Because further increases in the flowrate (see Figure
2-24)
will produce no increase
For further information on this subject refer to Reference
1
and References
6-9.
in the discharge coefficient, it is unnecessary to do any further iterations.
STRENGTH
OF
MATERIALS
The principles
of
strength of materials are applied to the design of structures
to
assure that the elements of the structures will operate reliably under a known set
of
loads. Thus the field encompasses both the calculation of the strength and deformation
of members and the measurement of the mechanical properties of engineer-
ing materials.
Stress
and
Strain
Consider a bar
of
length
L
and uniform cross-sectional area
A
to which an axial,
uniformly distributed load with a magnitude,
P,
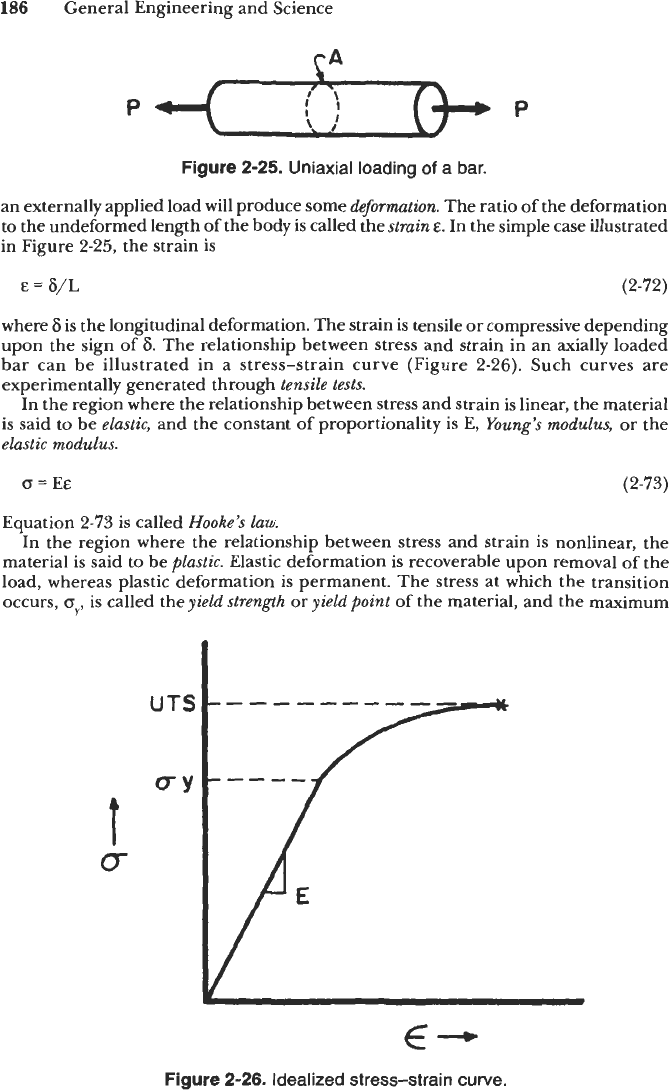
is applied at each end (Figure
2-25).
Then within the bar there is said to be
uniaxial
stress
c,
defined as the load, or force
per unit area
(2-71)
P
(3=-
A
If the load acts to elongate the bar, the stress is said to be
tensile
(+),
and
if
the load
acts to compress the bar, the stress is said to be
compressive
(-).
For all real materials,
186
General Engineering and Science
P
Figure
2-25.
Uniaxial loading
of
a bar.
an externally applied load will produce some
deformation.
The ratio of the deformation
to the undeformed length of the body is called the
strain
E.
In the simple case illustrated
in Figure
2-25,
the strain
is
E
=
6/L
(2-72)
where
6
is
the longitudinal deformation. The strain is tensile or compressive depending
upon the sign
of
6.
The relationship between stress and strain in an axially loaded
bar can be illustrated in
a
stress-strain curve (Figure
2-26).
Such curves are
experimentally generated through
tensile tests.
In the region where the relationship between stress and strain is linear, the material
is said to be
elastic,
and the constant of proportionality is
E,
Young's modulus,
or
the
elastic modulus.
(T
=
E&
(2-73)
Equation
2-73
is called
Hooke's law.
In the region where the relationship between stress and strain is nonlinear, the
material is said
to
be
plastic.
Elastic deformation
is
recoverable upon removal of the
load, whereas plastic deformation is permanent. The stress at which the transition
occurs,
(T",
is called the
yield strength
or
yield point
of the material, and the maximum
UTS
CY
t
cr
€-
Figure
2-26.
Idealized stress-strain
curve.

Strength of Materials
187
stress is called the
ultimate tensile strength,
UTS,
of
the material. Standard engineering
practice is to define the yield point
as
0.2% permanent strain.
When
a
bar is elongated axially, as in Figure 2-25, it will contract laterally. The
negative ratio of the lateral strain to the axial strain is called
Poisson's ratio
v.
For
isotropic
materials, materials that have the same elastic properties in all directions,
Poisson's ratio has
a
value
of
about
0.3.
Now consider a block to which
a
uniformly distributed load of magnitude
P
is
applied parallel to opposed faces with area
A
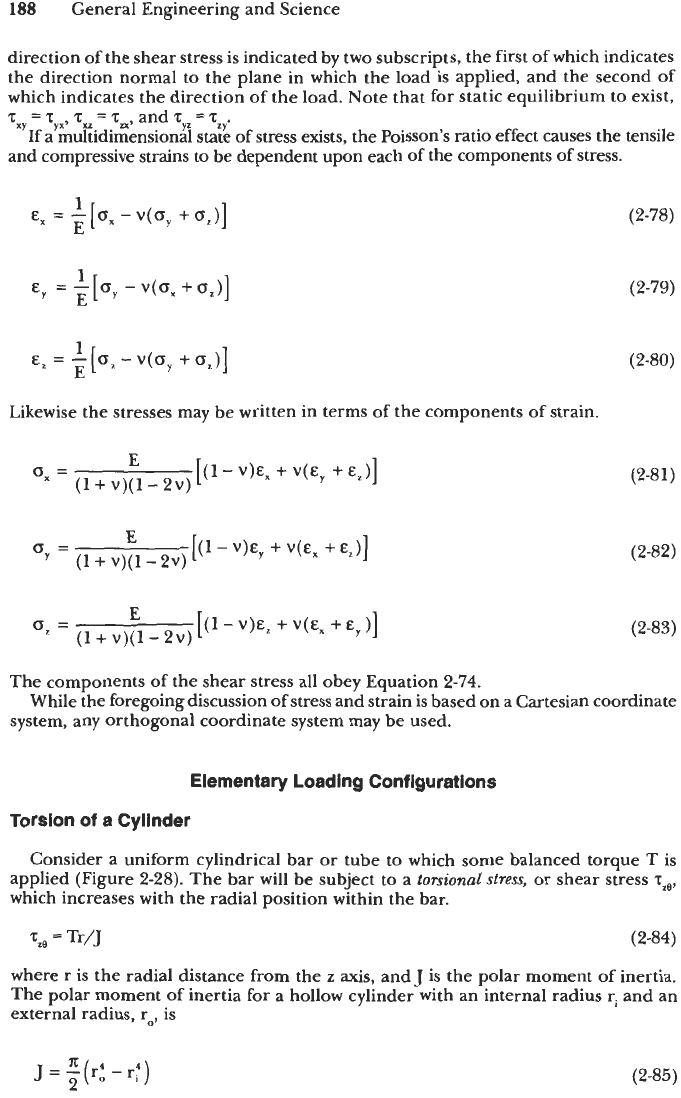
(Figure 2-27). These loads produce
a
shear stress
within the material
T.
T
=
P/A
(2-74)
Note that in order for the block
of
Figure 2-27 to be in static equilibrium, there
must also be a load
P
applied parallel to each of the faces
B.
Thus any given shear
stress always implies
a
second shear stress of equal magnitude acting perpendicularly
to the first
so
as to produce a state of static equilibrium. The shear stress will produce
a deformation
of
the block, manifested as
a
change in the angle between the face
perpendicular to the load and the face over which the load
is
applied. This change in
angle
is
called the
shear strain
y.
y=
Aa
(2-75)
For an elastic material the shear stress is related
to
the shear strain through a
constant of proportionality
G,
called the
shear
modulus.
The shear strain
is
dimensionless, and the shear modulus has units
of
force per unit area.
T
=
c;y
(2-76)
The shear modulus
is
related to Young's modulus and Poisson's ratio by
E
G=-
2(
1
+
v)
(2-77)
In practice, loads are not necessarily uniformly distributed nor uniaxial, and cross-
sec~ional areas are often variable. Thus it becomes necessary
to
define the stress at a
point
ab
the limiting value of the load per unit area
as
the area approaches zero.
Furthermore, there may be tensile or compressive stresses
(ox,
oY,
0,)
in each
of
three
orthogonal directions and
as
many
as
six shear stresses
(T~", T~~,
T~,,
T,~,
zyL,
TJ.
The
P
Figure
2-27.
Shear
loading
of
a
block.
188
General Engineering and Science
direction of the shear stress is indicated by two subscripts, the first of which indicates
the direction normal to the plane in which the load
is
applied, and the second of
which indicates the direction of the load. Note that for static equilibrium
to
exist,
zxy
=
zyx,
zu
=
z=,
and
zYz
=
T~~.
If a multidimensional state of stress exists, the Poisson’s ratio effect causes the tensile
and compressive strains to be dependent upon each of the components of stress.
1
E
E,
=
-[ox
-
V(OY
+o,)]
(2-78)
1
(2-79)
E,
=
-[oy
E
-
v(0,
+
(T,)]
1
E
E,
=
-[oz
-
v(0,
+
(T,)]
Likewise the stresses may be written in terms of the components of strain.
(T=
E
((1
-
V)E,
+
V(E,
+
4
(1
+
v)(l-
2v)
E
(T=
[
(1
-
V)E,
+
V(E,
+
E,
)]
(1
+
v)(l-
2v)
(2-80)
(2-81)
(2-82)
(2-83)
The components of the shear stress all obey Equation
2-74.
system, any orthogonal coordinate system may be used.
While the foregoing discussion of stress and strain is based on a Cartesian coordinate
Elementary Loading Configurations
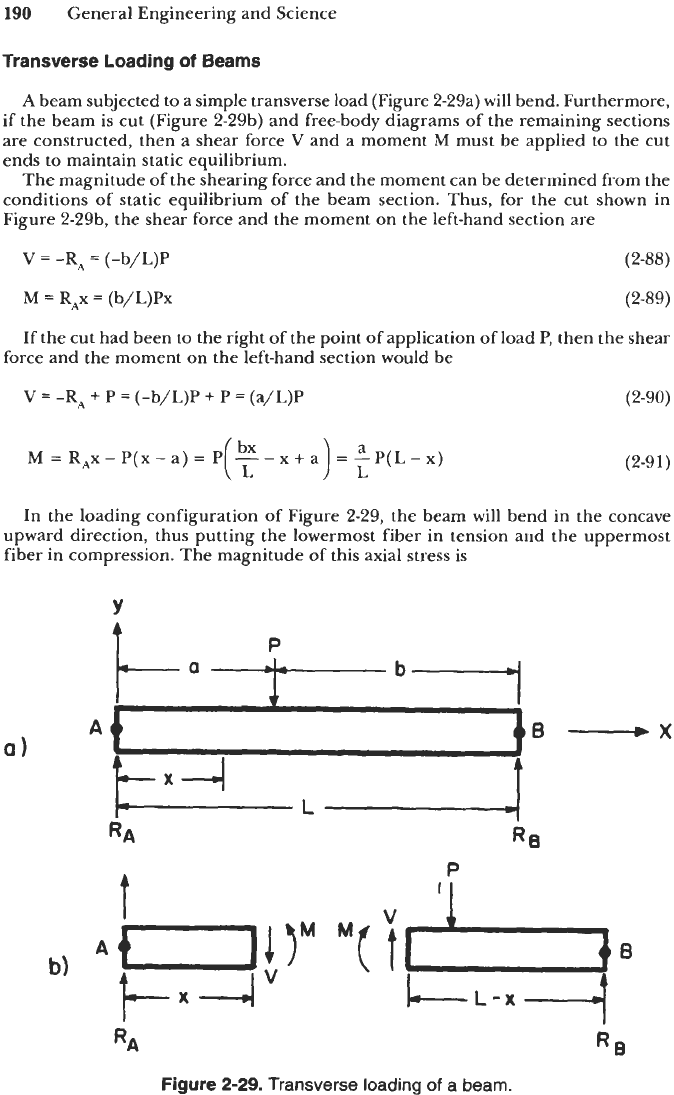
Torsion of a Cylinder
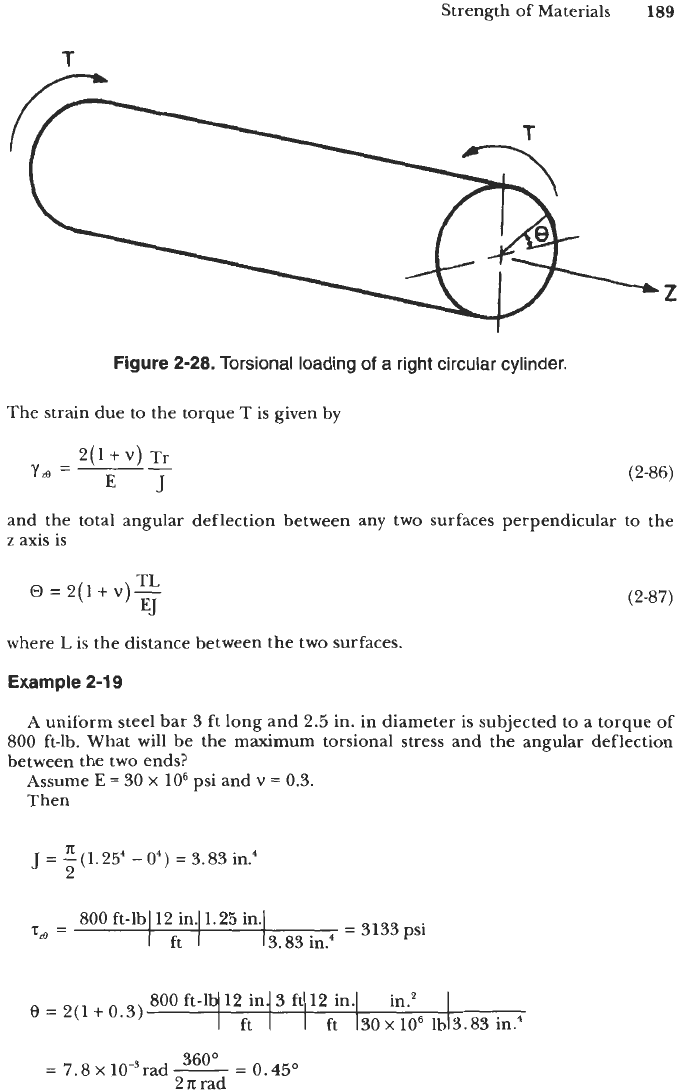
Consider a uniform cylindrical bar or tube to which some balanced torque T is
applied (Figure
2-28).
The bar will be subject to a
torsional
stress,
or shear stress
z,,
which increases with the radial position within the bar.
zze
=
Tr/J (2-84)
where r is the radial distance from the
z
axis,
and
J
is the polar moment of inertia.
The polar moment of inertia for a hollow cylinder with an internal radius ri and an
external radius, ro, is
1
R
J
=
-(r:
-
r:
2
(2-85)
Strength of Materials
189
Figure
2-28.
Torsional loading
of
a right circular cylinder.
The strain due to the torque
T
is given by
2(1+V)
Tr
y&
=--
EJ
(2-86)
and the total angular deflection between any two surfaces perpendicular to the
z
axis is
TL
0
=
2(1+v)-
EJ
(2-87)
where
L
is the distance between the two surfaces.
Example
2-19
A
uniform steel bar 3 ft long and 2.5 in. in diameter is subjected to a torque of
800
ft-lb. What will be the maximum torsional stress and the angular deflection
between the two ends?
Assume E
=
30
x
lo6
psi and
v
=
0.3.
Then
n
J
=
-(1.254
-
04)
=
3.83 in.4
2
800
ft-lbll2 in.ll.25 in.1
=
3133 psi
T&
=
I
ft
I
13.83 in.4
800
ft-lb) 12 in1 3 ftll2 in.[ in.'
I
e
=
2(1+
0.3)
lftl
I
ft 130
x
lo6
1b13.83 in.*
360"
2
x:
rad
=
7.8xlO-'rad-
=
0.45"
190
General Engineering and Science
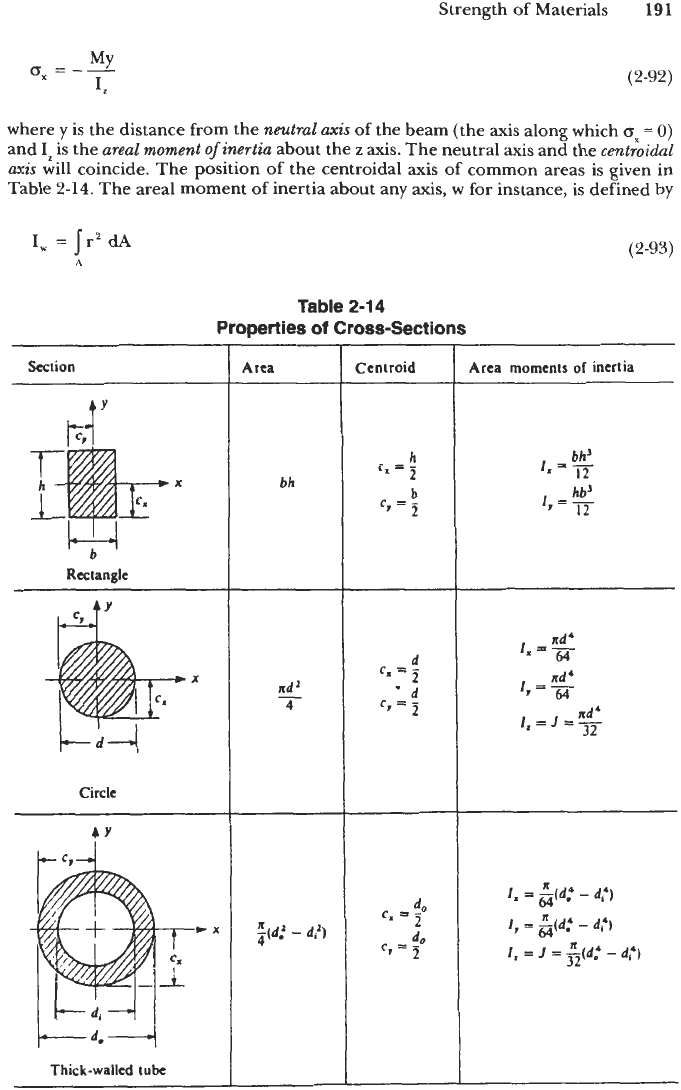
Transverse Loading
of
Beams
A
beam subjected to a simple transverse load (Figure 2-29a)
will
bend. Furthermore,
if the beam is cut (Figure 2-2913) and free-body diagrams of the remaining sections
are constructed, then a shear force
V
and a moment
M
must be applied to the cut
ends to maintain static equilibrium.
The magnitude of the shearing force and the moment can be determined from the
conditions of static equilibrium of the beam section. Thus, for the cut shown in
Figure 2-29b, the shear force and the moment on the left-hand section are
V
=
-RA
=
(-b/L)P
(2-88)
M
=
RAx
=
(b/L)Px
(2-89)
If the cut had been to the right of the point of application of load P, then the shear
force and the moment on the left-hand section would be
V
=
-R,
+
P
=
(-b/L)P
+
P
=
(a/L)P
(2-90)
(2-91)
In the loading configuration of Figure 2-29, the beam will bend in the concave
upward direction, thus putting the lowermost fiber in tension and the uppermost
fiber in compression. The magnitude of this axial stress is
Y
RA
t
P
RR
RA
Figure
2-29.
Transverse loading
of
a
beam.
Strength of Materials
191
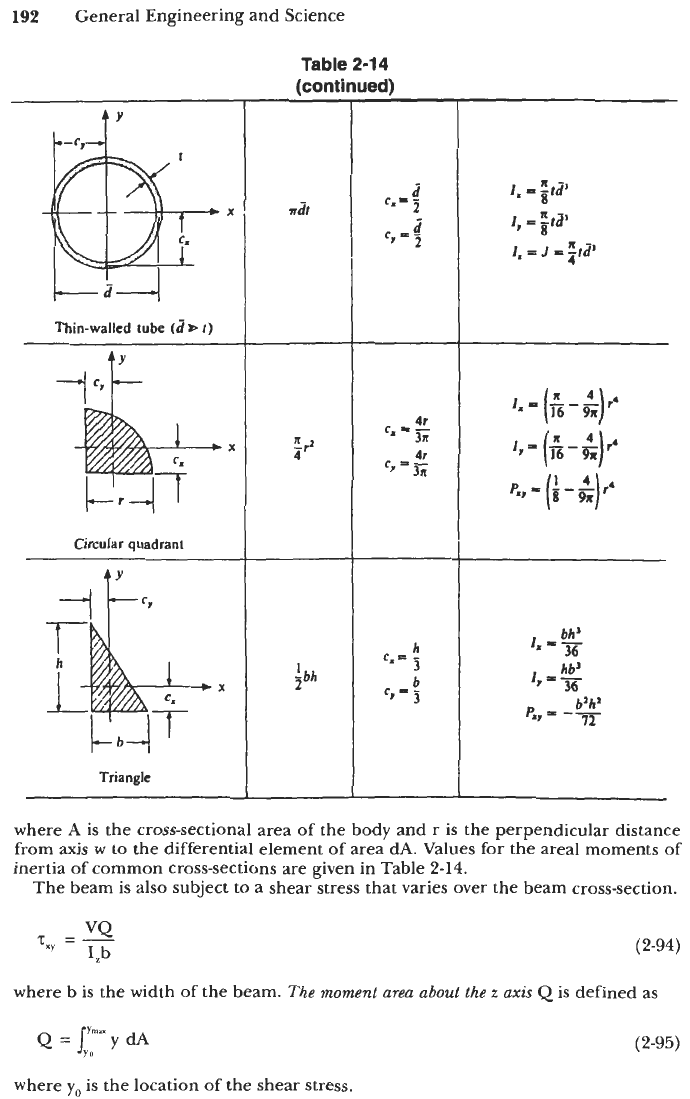
(2-92)
where
y
is the distance from the
neutral axis
of the beam (the axis along which
ox
=
0)
and
Iz
is the
areal moment
of
inertia
about the
z
axis. The neutral axis and the
centroidal
axis
will coincide. The position
of
the centroidal axis of common areas is given in
Table
2-14.
The areal moment of inertia about any
axis,
w
for instance, is defined by
I,
=
J’r‘
d~
A
Table
2-1
4
Properties
of
Cross-Sections
(2-93)
Section
Rectangle
Circle
tX
+d*4
Thick-walled tube
Area
bh
nd
’
4
-
%(d:
-
d’)
Centroid Area moments
of
inertia
bh’
I‘
=
-
12
hb’
I
=-
I
I2
I,
=
64
[(d,O
-
dl)
I,
=
“(d:
64
-
dl)
I,
=
J
=
‘(d:
32
-
d:)
192
General Engineering and Science
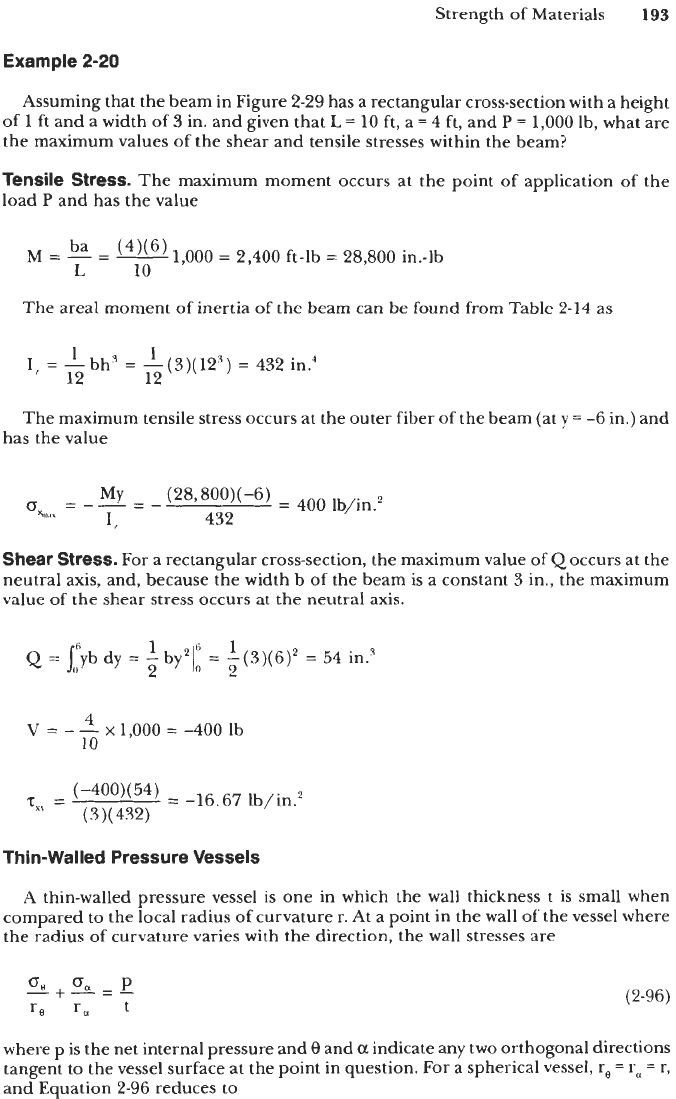
Table
2-14
(continued)
4Y
Thin-walled tube
(d
S
I)
X
Circular quadrant
X
Triangle
Wdl
ibh
1
h
b
e.=
J
5
J
bh3
hb’
36
I,
=
sij-
I,
=
-
PaI
=
--
b’h’
I2
where
A
is the cross-sectional area of the body and r is the perpendicular distance
from axis
w
to
the differential element of area
dA.
Values for the areal moments
of
inertia of common cross-sections are given in Table
2-14.
The beam is also subject to a shear stress that varies over the beam cross-section.
(2-94)
where b is the width of the beam.
The moment
area
about
the
z
axis
Q
is defined as
Q=FydA
Y
II
(2-95)
where
yo
is the location of the shear stress.
Strength of Materials
193
Example
2-20
Assuming that the beam in Figure 2-29 has a rectangular cross-section with a height
of
1
ft and a width of
3
in. and given that
L
=
10 ft, a
=
4 ft, and
P
=
1,000
lb, what are
the maximum values
of
the shear and tensile stresses within the beam?
Tensile Stress.
The maximum moment occurs at the point of application of the
load
P
and has the value
M=-=-
(4)(6)
1,000
=
2,400 ft-lb
=
28,800 in.-lb
L
10
The areal moment
of
inertia
of
the beam can be found from Table
2-14
as
1 1
12 12
I,
=
-
bh'
=
-(3)(12')
=
432 in.4
The maximum tensile stress occurs at the outer fiber of the beam (at
y
=
-6 in.) and
has the value
Shear Stress.
For a rectangular cross-section, the maximum value
of
Qoccurs at the
neutral axis, and, because the width
b
of
the beam is a constant
3
in., the maximum
value
of
the shear stress occurs at the neutral axis.
161
2
2
Q
=
j'yb dy
=
-
by21
=
-(3)(6)'
=
54 in.'
4
10
V
=
-
-
x
1,000
=
-400 lb
Thin-Walled Pressure Vessels
A
thin-walled pressure vessel is one in which the wall thickness
t
is small when
compared to the local radius
of
curvature r. At a point in the wall
of
the vessel where
the radius
of
curvature varies with the direction, the wall stresses are
(2-96)
where p is the net internal pressure and
8
and
~1
indicate any two orthogonal directions
tangent
to
the vessel surface at the point in question. For a spherical vessel, re
=
ra
=
r,
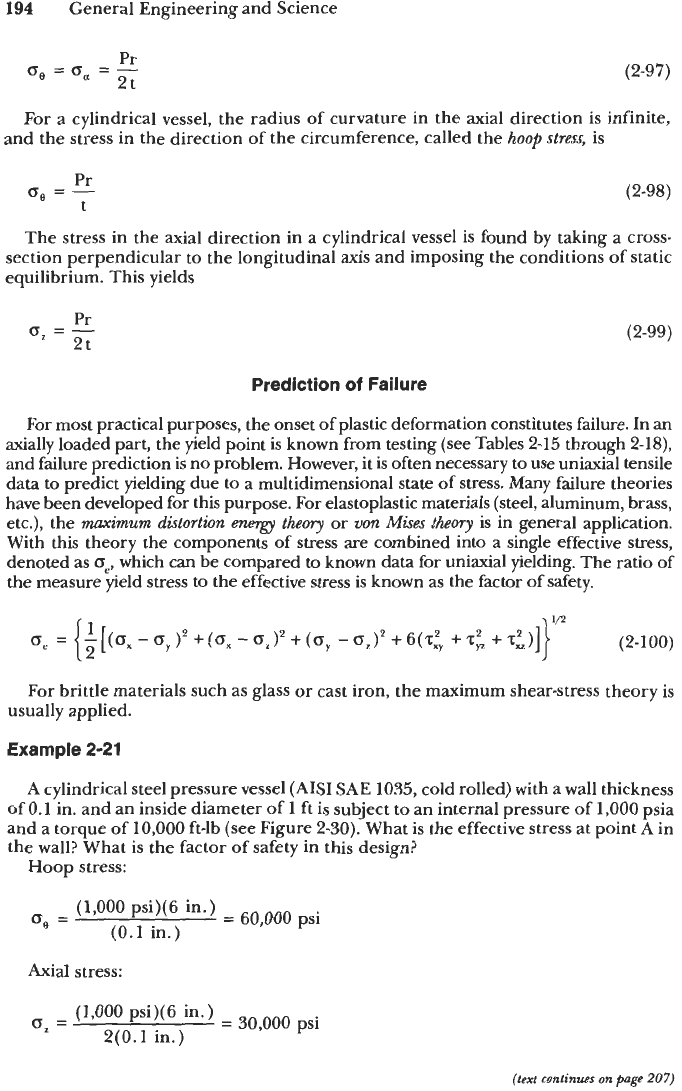
and Equation 2-96 reduces
to
194
General Engineering and Science
(2-97)
Pr
(T =(T
=-
a
2t
For a cylindrical vessel, the radius of curvature in the axial direction is infinite,
and the stress in the direction of the circumference, called the
hoop
stress,
is
Pr
fl=-
t
(2-98)
The stress in the axial direction in a cylindrical vessel is found by taking a cross-
section perpendicular to the longitudinal axis and imposing the conditions of static
equilibrium. This yields
Pr
2t
(T
=-
(2-99)
Prediction
of
Failure
For most practical purposes, the onset of plastic deformation constitutes failure. In an
axially loaded part, the yield point is known from testing (see Tables 2-15 through
2-18),
and failure prediction is
no
problem. However, it is often necessary to use uniaxial tensile
data to predict yielding due to a multidimensional
state
of stress. Many failure theories
have been developed
for
this purpose. For elastoplastic materials (steel, aluminum, brass,
etc.), the
maximum distortion
energy
theory
or
urn
Misa
theory
is in general application.
With this theory the components of stress are combined into a single effective stress,
denoted as
G~,
which can be compared to known data for uniaxial yielding. The ratio of
the measure yield stress to the effective stress is known as the factor of safety.
1/2
1
(T,
=
{
[
(
(T,
-
(T,
)2
+
(
6,
-
0.
)'
+
(
(T,
-
6,
)*
+
6
(
T:,
+
zfZ
+
T:
I]}
(2-1
00)
For brittle materials such as glass or cast iron, the maximum shear-stress theory is
usually applied.
Example
2-21
A
cylindrical steel pressure vessel
(AIS1
SAE
1035, cold rolled) with a wall thickness
of 0.1 in. and an inside diameter of 1 ft is subject to an internal pressure of 1,000 psia
and a torque of 10,000 ft-lb (see Figure 2-30). What is the effective stress at point
A
in
the wall? What is the factor of safety in this design?
Hoop stress:
(1,000 psi)(6 in.)
(0.1 in.)
(To
=
=
60,000 psi
Axial stress:
(1,000 psi)(6 in.)
2(0.1 in.)
OZ
=
=
30,000 psi
(text
continues
on
page
207)
