Leroy C., Rancoita P.-G. Principles Of Radiation Interaction In Matter And Detection

Подождите немного. Документ загружается.

January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

220 Principles of Radiation Interaction in Matter and Detection

and, ﬁnally, Eq. (3.9) can be re-written as

≈ M

u. (3.11)

In summary, to a ﬁrst approximation the relative atomic mass, A (also referred to

as atomic weight, see page 15), of a nuclide is expressed by its mass number. Fur-

thermore (see Appendix A.2), if we assume m

nucl

≈ 938.92 MeV/c

, i.e., the mean

value between the proton and neutron masses, from an inspection of Eqs. (3.8, 3.9)

we can note that the variation

of the binding energy per nucleon divided by m

nucl

slightly aﬀects the resulting value of M

and the expression (3.11) is approximated

to about or better than a percent also for light nuclei. From Eq. (3.10) one obtains

≈ 7.42 MeV/nucleon. Since numerically A ∼ M

, in this chapter the symbol A

may be found to replace M

, particularly in theoretical expressions or calculations

(e.g., see Sects. 3.2.2-3.2.3) to indicate the number of nucleons in a nucleus with a

compact notation.

3.1.1 Radius of Nuclei and the Liquid Droplet Model

The size or the radius of a nucleus is not completely deﬁned because the nucleus

cannot be considered as a rigid sphere. However, under the approximation of a

spherical shape, the mean nuclear radius can be determined by scattering with

particles such as electrons, neutrons, alpha’s, etc. From these measurements, it was

concluded that the nuclear radii are proportional to the cubic root of their mass

numb er. From the earliest scattering investigations by Rutherford and Chadwick,

it was derived that, except for the lightest nuclei, the nuclear radius, r

, is given by

the relation [Bethe and Ashkin (1953); Povh, Rith, Scholz and Zetsche (1995)]:

' r

1/3

[fm], (3.12)

where r

' 1.2 fm = 1.2 × 10

−13

cm. For these nuclei, the mean nuclear density,

, is approximately constant and given by:

πr

1/3

3 m

4 πr

(3.13)

≈ 2 × 10

[g/cm

The mean density of the nuclear matter is extremely large relatively to ordinary

matter.

For all isotopes from

H up to

238

U, the binding energy per nucleon ranges from ≈

2.6 MeV/nucleon up to ≈ 8.8 MeV/nucleon; for

H (deuterium) it is ≈ 1.1 MeV/nucleon.

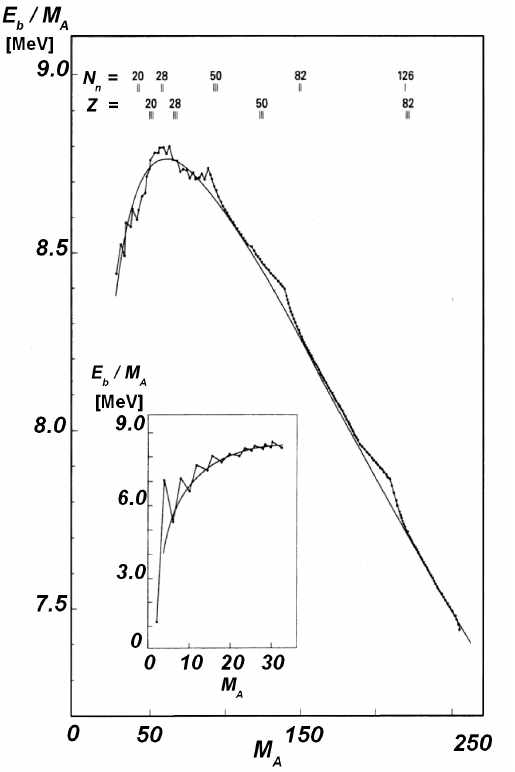
January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

Nuclear Interactions in Matter 221

Fig. 3.1 Binding energy in units of MeV per nucleon in case of stable nuclei with even values

of mass number M

as a function of M

(adapted and reprinted with permission from Povh,

B., Rith, K., Scholz, C. and Zetsche, F. (1995), Particles and Nuclei: an Introduction to the

Physical Concepts, Figure 2.4, page 18, Springer-Verlag Publ., Berlin Heidelberg New-York;

° by

Springer-Verlag 1995). The continuous line is determined by the Weizs¨acker–Bethe mass formula

given in Eq. (3.14) (at page 223).

3.1.1.1 Droplet Model and Semi-empirical Mass Formula

In nuclear physics, the analogy with the physics of an incompressible ﬂuid was

suggested by the fact that the nuclear density (Sect. 3.1.1) and binding energy per

nucleon (Sect. 3.1) are almost constant. Thus, the nucleus can be regarded as a

liquid drop, in which an almost constant binding energy per nucleon corresponds to

a constant heat of vaporization independent of the droplet size.

January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

222 Principles of Radiation Interaction in Matter and Detection

The liquid drop model was used by von Weizs¨acker and Bethe to evaluate nuclear

masses ([von Weizs¨acker (1936); Blatt and Weisskopf (1952); Bethe and Ashkin

(1953); Finkelnburg (1964)]; see also Chapter 2, Section 3 in [Povh, Rith, Scholz

and Zetsche (1995)]). In addition, a semi-empirical mass formula was proposed in

which the overall nuclear binding energy can be computed on the base of several

contributions, whose relative magnitudes are given empirically by adapting a few

parameters to measured nuclear masses. In this framework with the parameters a

, a

and δ

deﬁned at page 223 (from [Povh, Rith, Scholz and Zetsche (1995)]),

ﬁve contributions to the binding energy, E

, are considered:

• Volume Energy

The major contribution to E

is given by nucleon interactions mediated by

the nuclear forces. As already mentioned, the binding energy per nucleon

slightly varies, except for light (and very heavy) nuclei. Further-

more, the quasi-constancy of the mean nuclear-matter density indicates

that the nuclear forces are short ranged and involve the nearest nucleon

neighbors. The binding volume-energy can be expressed by:

' a

• Surface Energy

Nucleons located at the nuclear surface are necessarily less bound than

those inside the nucleus, because less nucleons are surrounding them. The

number of surface nucleons is proportional to the nucleus surface, which, in

turn, is proportional to M

2/3

[Eq. (3.12)]. The eﬀect of the binding surface

energy is to decrease the overall binding-energy, thus:

' −a

2/3

• Coulomb Energy

The electrostatic repulsion among protons has a long range characteri-

stic. The resultant Coulomb-energy will decrease the total amount of the

nuclear binding-energy. In the electrostatic theory, the energy due to a

net charge Ze uniformly distributed over a sphere of radius R is given by

−

(Ze)

. To a ﬁrst approximation, the Coulomb-energy term can be eva-

luated as:

' −a

1/3

• Asymmetry Energy (neutron excess)

Light stable nuclei are those for which N

≈ Z. For heavier nuclei, the

number of neutrons usually exceed the number of protons. The neutron

excess increases as the mass number increases. Therefore, a neutron excess

term depending on N

−Z must be present in the equation. In addition, it

has to vanish for N

' Z. The asymmetry term is given by:

' −a

− Z)

January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

Nuclear Interactions in Matter 223

• Pairing Energy

Nuclei with an even number of both protons and neutrons show a trend

of high stability. Nuclei having an odd number of one type of nucleon and

an even number of the other type are less stable. Nuclei with doubly odd

numbers of protons and neutrons are even more unstable. This pairing eﬀect

is taken into account by the additional term:

' −

√

As mentioned above, the semi-empirical mass formula was derived by von

Weizs¨acker and Bethe for the atomic mass M

(Z, M

) of an atom constituted

by M

nucleons, Z of them being protons; it can be summarized in the following

way:

(Z, M

) = N

+ Zm

+ Zm − E

= N

+ Zm

+ Zm − a

2/3

+ a

1/3

+ a

− Z)

√

, (3.14)

where the parameters are (from [Povh, Rith, Scholz and Zetsche (1995)])

= 15.67 MeV/c

= 17.23 MeV/c

= 0.714 MeV/c

= 93.15 MeV/c

= −11.2 MeV/c

for even-even nuclei (even value of M

= 0 MeV/c

for even-odd and odd-even nuclei (odd value of M

= +11.2 MeV/c

for odd-odd nuclei (even value of M

3.1.2 Form Factor and Charge Density of Nuclei

The classical interaction of a charged particle, like an electron, with a massive

object of charge Ze, like a nucleus, is described by the classical Rutherford diﬀe-

rential cross section (Sect. 1.5), in which spin dependent eﬀects and target recoil

are neglected. The same equation is derived following a non-relativistic quantum-

mechanical approach, using the Born approximation, for the case of a point-like

target of charge Ze. For a non-point like target, it is possible to demonstrate that

the scattering cross section on nucleus can be rewritten as (see for instance Sec-

tion 5.2 of [Povh, Rith, Scholz and Zetsche (1995)], and also Section 6.3 of [Segre

(1977)]):

dσ

dΩ

Rutherford, non point like

dσ

dΩ

Rutherford

|F (~q)|

, (3.15)

where ~q is the tri-momentum transfer in the scattering process and F (~q) is the

so-called nuclear form-factor given by

F (~q) =

(~r) exp

i~q ·~r

January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

224 Principles of Radiation Interaction in Matter and Detection

where ~r is the radial position with respect to the scattering center, d

r is the

inﬁnitesimal volume located at ~r, and n

(~r) is the normalized charge-density distri-

bution at ~r. The latter is related to the charge-density distribution by:

(~r, Z) = Zen

(~r)

with

(~r) d

r = Ze

(~r, Z) d

r = Ze.

As a consequence, we have:

F (0) =

(~r) d

r = 1.

At relativistic energies, spin eﬀects cannot be neglected anymore and, for an

electron interaction, they are taken into account by the Mott diﬀerential cross sec-

tion (as mentioned at pages 85 and 123). Neglecting recoil eﬀects, the Mott cross

section can be written as:

dσ

dΩ

Mott

dσ

dΩ

Rutherford

1 − β

sin

, (3.16)

where θ is the scattering angle. The meaning of the right-hand multiplicative term

in Eq. (3.16) can be understood, for instance, considering that for β → 1 it becomes

∼ cos

(θ/2). Thus, for θ ' 0

◦

, the cross section becomes ' 0, which accounts for

helicity conservation in the scattering process.

As seen above, the extended charge-distribution of the nucleus can be described

by introducing the nuclear form-factor. Often, for charge distributions which have a

spherical symmetry, form factors depend on the value of the tri-momentum transfer

(|~q|) and is indicated as F (q), or F (q

). The form factor can be determined by

measuring the experimental diﬀerential cross section and taking the ratio to the

Mott diﬀerential cross section (see for instance [Povh, Rith, Scholz and Zetsche

(1995)]), namely:

dσ

dΩ

exp

dσ

dΩ

Mott

|F (q)|

The ﬁrst measurements of nuclear form-factors were carried out during the years

1950s. In Fig. 3.2, the diﬀerential cross section of electrons on

C nuclei is shown

as a function of the electron diﬀusion angle. It was obtained by electron scattering

at 420 MeV [Hofstadter (1957)]: the shape of the diﬀerential cross section depends

on the nuclear form-factor. The dashed line corresponds to the expected diﬀerential

cross section calculated using the Born approximation for the electron scattering

on the diﬀused surface of a homogeneous sphere. The diﬀerential cross section exhi-

bits the typical diﬀraction-pattern, in which a minimum is present at θ ' 51

◦

i.e., |~q|/~ ' 1.8 fm

−1

Systematic experimental measurements have shown that the nucleus has a charge

distribution decreasing gradually towards the surface. Therefore, one could conclude

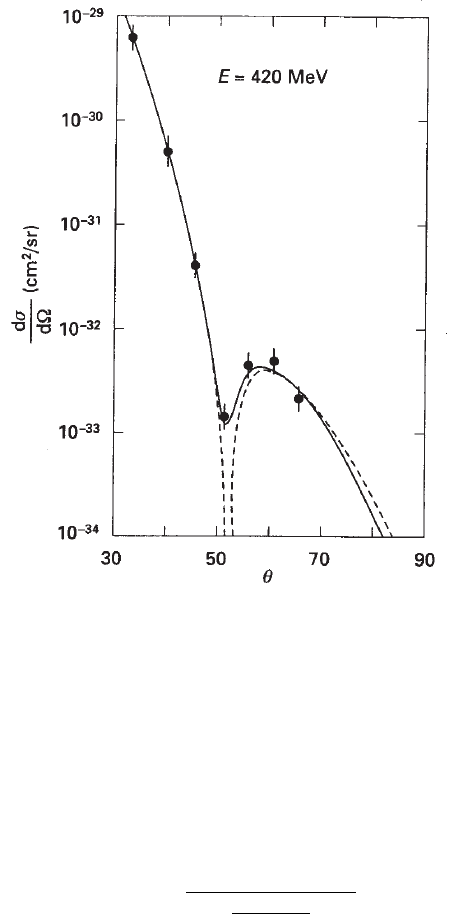
January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

Nuclear Interactions in Matter 225

Fig. 3.2 Measurement of the form factor of

C nucleus obtained by the scattering of 420 MeV

electrons (adapted and reprinted, with permission, from the Annual Review of Nuclear Science,

Volume 7

° 1957 by Annual Reviews www.annualreviews.org; [Hofstadter (1957)]). The diﬀerential

cross section is shown as a function of the electron scattering angle. The dashed line corresponds

to the expected electron scattering on the diﬀused surface of a homogeneous sphere, following the

Born approximation. The continuous line corresponds to a ﬁt to the experimental data.

that Eq. (3.13) is valid only as a simpliﬁed expression. Under the approximation of

nuclear spherical charge-symmetry and in agreement with the experimental data,

the radial charge-density ρ

(r, Z) is given by

(r, Z) =

(0, Z)

exp

r−C

)

+ 1

, (3.17)

where r is the radial distance from the center of the nucleus in fm, C

) '

1.07 M

1/3

fm for M

≥ 30, Z

' 0.545 fm and ρ

(0, Z) is determined from the

condition:

Ze = 4π

∞

(r, Z) r

dr.

January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

226 Principles of Radiation Interaction in Matter and Detection

3.1.3 Angular and Magnetic Moment, Shape of Nuclei

Many nuclei have an intrinsic angular momentum

, which represents the total

angular momentum of the nucleus and is given by the sum of the orbital momentum

and the spin of all protons and neutrons inside the nucleus.

is always an integer

or a half integer in units of ~ :

| = I

~. (3.18)

Often, I

is referred to as the nuclear spin

∗

and varies between 0 and

for all

known nuclei in their ground state. Nuclei with an even number of nucleons (even

mass number) have an integral value of I

. In most cases, they do not have angular

momentum at all, i.e., I

= 0. In particular, even-even nuclei have a ground state

with I

= 0. Nuclei with odd mass number always have a half-integral spin.

Nuclei can exist in excited states of higher energy, as will be discussed in the next

part of this section. The angular momenta of excited states may diﬀer from those of

the corresponding nuclear ground states. It has been shown that there are selection

rules for transitions between energy states of the nucleus or from neighboring nuclei.

Since transitions between nuclear states with very diﬀerent angular momenta

are strongly forbidden, there exist long-lived excited nuclear states. Nuclei in these

long-lived excited states are called Nuclear isomers. Isomeric nuclei, have the same

charge and mass, but are in diﬀerent energy states, i.e., have diﬀerent arrangements

of their nucleons. An important diﬀerence between a normal excited nuclear state

and a nuclear isomer is that, for the latter one, the transition probability to a

more stable state, particularly to the ground state, is very small. So, like in atomic

physics, the isomeric nucleus can be called a metastable nuclear state.

As for the case of electron shell, a magnetic moment is related to the angular mo-

mentum of the electrically charged constituents of the nucleus. These constituents,

by their orbital motion, generate electric current densities which contribute to the

overall magnetic moment in addition to other eﬀects, like the intrinsic magnetic

moment of nucleons. In Appendix A.2, the measured proton and neutron magnetic

moments are given in units of the so-called nuclear magneton, µ

, deﬁned as:

The positive (negative) sign of the proton (neutron) magnetic moment indicates

that it is directed along (opp osite) to its angular momentum.

Heavy nuclei show a deviation from a spherical nuclear shape of the order

of 1%. An ellipsoidal shape agrees better with the experimental results. Usually,

there is a contraction in the direction of the spin axis. This asymmetry is repre-

sented by associating an electric quadrupole moment with the nucleus. The p ositive

∗

At page 232, Finkelnburg (1964) noted that this notation is misleading: this designation is

correct only for elementary particles, like protons and neutrons. For nuclei consisting of protons

and neutrons,

represents the total angular momentum of the nucleus.

January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

Nuclear Interactions in Matter 227

quadrupole sign is assigned to an extension in the direction of the spin axis, while

a negative value corresponds to a shortening along this direction.

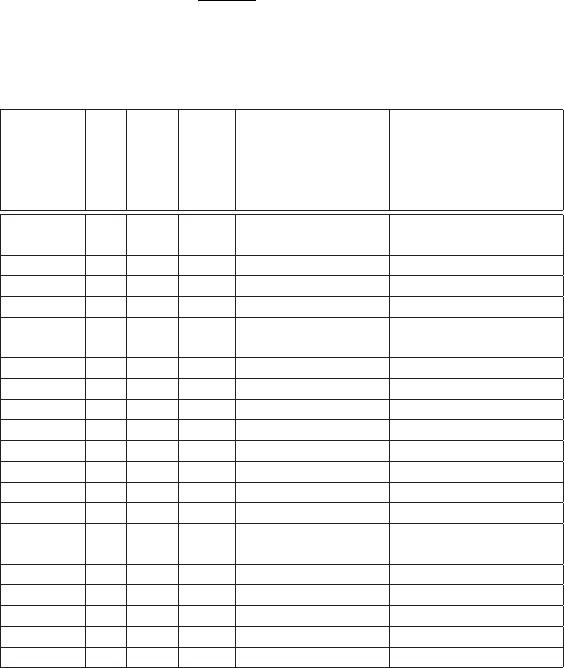
In Table 3.1, values of the nuclear spin, nuclear magnetic and electrical

quadrupole are shown for several nuclides ([Stone (2001)] and references therein).

3.1.4 Stable and Unstable Nuclei

An aggregate of nucleons may become a stable and bound nucleus only if the num-

ber of one nucleon-type does not largely exceed the other nucleon-type. There is

no experimental evidence of a stable nucleus consisting exclusively of neutrons or

exclusively of protons.

Unless replenished artiﬁcially, unstable nuclides in any given sample decay at a

rate −dN

(t)/dt, where the minus sign is introduced because N

(t) diminishes as

the time, t, increases. The decay rate is proportional to the number of nuclides in

the sample at the time t and is expected to become independent of t, i.e.,

(t)

= −λ

(t), (3.19)

Table 3.1 Spin, nuclear magnetic moment and electric quadrupole moment

for some stable nuclides in their ground state determined by recent measure-

ments (see [Stone (2001)] and references therein).

Element Z M

Spin Nuclear Magnetic Electric Quadrupole

Moment Moment

−24

−2

H 1 1 1/2 2.79284734(3)

2 1 0.857438228(9) 0.00286(2)

He 2 3 1/2 -2.12749772(3)

Be 4 9 3/2 -1.1778(9) 0.0529(4)

C 6 13 1/2 0.7024118(14)

N 7 14 1 0.40376100(6) 0.02001(10)

15 1/2 -0.28318884(5)

O 8 17 5/2 -1.89379(9) -0.26(3)

Al 13 27 5/2 3.6415068(7) 0.1402(10)

Si 14 29 1/2 -0.55529(3)

K 19 41 3/2 0.21489274(12) 0.060(5)

Ca 20 43 7/2 -1.317643(7) -0.049(5)

Mn 25 51 5/2 3.5683(13) 0.42(7)

Ga 31 69 3/2 2.01659(5) 0.17(3)

Zr 40 91 5/2 -1.30362(2) -0.206(10)

Ru 44 99 5/2 -0.641(5) 0.079(4)

101 5/2 -0.719(6) 0.46(2)

Nd 60 145 7/2 -0.656(4) -0.314(12)

Ta 73 181 7/2 2.3705(7) 3.17(2)

W 74 183 1/2 0.11778476(9)

Ir 77 191 3/2 0.1507(6) 0.816(9)

Pb 82 207 1/2 0.58219(2)

January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

228 Principles of Radiation Interaction in Matter and Detection

where λ

is called decay constant: it is the inverse of the mean lifetime, τ

≡

Finally, by integrating Eq. (3.19) between the limits N

(t = 0) = N

at t = 0 and

(t) = N

at the time t, we have:

= N

exp (−tλ

) = N

exp

−

The treatment, extended to the case of more than one nuclide in a substance and

to ﬂuctuations in radioactive decays, can be found in [Bethe and Ashkin (1953)].

Among the combinations of nucleons which form bound nuclear aggregates, only

a relatively small fraction of them generates stable nuclides. For instance, β-stable

nuclides for are the ones whose number of neutrons and protons belong to the

narrow band shown

∗

in Fig. 3.3 [Bohr and Mottelson (1969)]. For stable nuclei, an

approximated empirical relationship between the mass number M

and the atomic

numb er Z is given by [Marmier and Sheldon (1969)]:

Z =

1.98 + 0.0155 M

2/3

. (3.20)

This formula shows, for M

< 40, that stable nuclides are those for which Z '

. For the heaviest nuclei, the number of neutrons can exceed the number of

protons up to ≈ 50%.

Dynamical instabilities lead to spontaneous nuclear break-up into two or more

parts, i.e., α-decay, ﬁssion and other related phenomena. The β-instability proceeds

via a change in the atomic number Z by the emission or absorption of an electron,

namely via β-decay or electron capture.

A large amount of nuclei emits positrons or electrons under reactions, result-

ing in a net charge change of one unit. The β-radioactive decay originates from

fundamental weak processes involving nucleons:

n → p + e

−

+ ¯ν

(3.21)

p → n + e

+ ν

, (3.22)

where ¯ν

and ν

are the electronic antineutrino and neutrino, associated with the

electron and the positron, respectively. Also, the process, in which an external

orbital electron is absorbed, can occur:

p + e

−

→ n + ν

. (3.23)

The process

n + e

→ p + ¯ν

is theoretically possible, but there is no positron available around the nucleus. Pro-

cesses which proceed following Eqs. (3.21, 3.22) are called electron (β

−

) and positron

(β

) β-decay, respectively. Equation (3.23) describes the electron capture pro-

cess. The other nucleons in the nucleus can supply to or remove the energy needed

for these processes from the nucleon, undergoing the transformation.

∗

The Fig. 3.3 is known as a Segr`e chart.

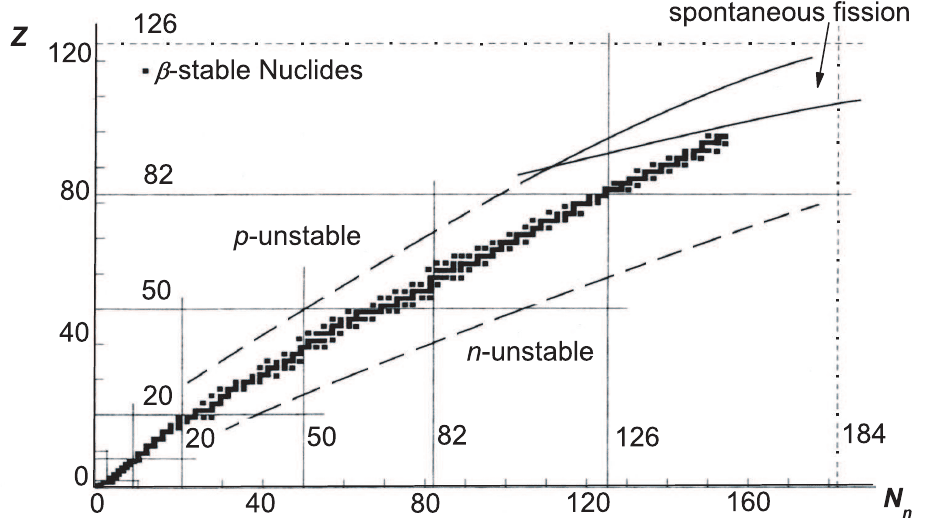
January 9, 2009 10:21 World Scientiﬁc Book - 9.75in x 6.5in ws-bo ok975x65˙n˙2nd˙Ed

Nuclear Interactions in Matter 229

Fig. 3.3 Z versus N

distribution of β-stable nuclides (adapted with permission from [Bohr and Mottelson (1969)]).