Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


new
phenomena,
such
as
gaps
in
Hopf
branches,
periodic
motions
not
stably
connected
to
steady
states,
and
the
discovery
of
formation
of
islands,
which
one
can
expect
to
find
in
general
systems
of
evolution
equations.
Many
of
the
new
phenomena,
predicted
on
topological
grounds
alone,
still
await
experimental
confirmation
[Dangelmayr
and
Armbruster
1983;
Armbruster
1983].
As
we
have
mentioned
in
Sections
1.2
and
6.1,
structurally
stable
systems
may
not
be
dense.
Indeed,
Peixoto
and
Pugh
[1968)
have
shown
that
structurally
stable
systems
are
not
dense
on
any
noncompact
manifold
of
dimension
~
2.
Finally,
Williams
[1970]
showed
that
structurally
stable
diffeomorphisms
are
not
dense
on
the
two-dimensional
torus.
Thus,
we
are
left
with
two
courses
of
approach.
We
can
either
alter
the
equivalence
relation
on
the
space
of
all
dynamical
systems
hoping
that
stability
with
respect
to
the
new
equivalence
relation
may
be
dense,
or
we
can
ask
for
some
structures
which
are
less
than
dense
in
the
given
topology.
one
of
the
new
equivalence
relation
which
aroused
most
interest
is
n-stability.
This
is
based
on
n-equivalence
discussed
in
Chapter
4.
n-stability
is
stability
with
respect
to
n-quivalence.
Unfortunately
n-stability
is
not
any
more
successful
than
structural
stability
as
far
as
the
dense
of
the
structure
is
concerned.
For
examples,
see
Abraham
and
Smale
[1970]
and
Newhouse
[1970a,b].
For
the
second
approach,
it
is
natural
to
ask
the
following
question:
Given
an
arbitrary
dynamical
system,
can
we
deform
it
into
a
structurally
stable
system?
If
we
can,
how
small
a
deformation
is
necessary?
Clearly,
we
cannot
make
it
arbitrarily
c
1
-small,
otherwise
it
would
imply
c
1
-density
of
structural
stability.
Thus
we
may
be
able
to
deform
it
by
an
arbitrarily
C
0
-
small
deformation.
Here
we
only
talk
about
the
size
of
the
deformation
needed
to
produce
structural
stability,
and
we
leave
the
smoothness
of
the
maps
and
the
definition
of
structural
stability
as
before.
The
following
theorem
by
Smale
[1973]
and
Shub
264

[1972]
answers
these
questions.
Theorem
6.5.4
Any
cr
diffeomorphism
(1
~
r
~
oo)
of
a
compact
manifold
is
cr
isotopic
to
a c
1
structurally
stable
system
by
an
arbitrarily
C
0
-small
isotopy.
Thus
the
structural
stability
is
dense
in
Diffrx
with
respect
to
the
C
0
-topology.
It
should
be
noted
that
the
structural
stability
is
no
longer
open
in
this
topology.
De
Oliviera
[1976]
showed
an
analogous
theorem
for
flows.
Recall
that
the
motivation
of
studying
the
structural
stability
of
a
dynamical
system
is
because
one
is
required
to
make
measurements,
but
since
the
measurements
are
limited
by
their
measurement
uncertainties
(the
measured
systems
are
only
approximations
of
the
true
systems.
It
is
important
to
know
whether
the
qualitative
behavior
of
the
approximate
system
and
the
true
system
are
the
same.
Structural
stability
of
a
system
guarantees
this
if
the
approximation
is
sufficiently
good.
To
make
things
more
complicated
(also
more
interesting),
in
most
of
the
situations
the
measured
quantities
would
not
be
completely
time
independent,
but
only
be
approximately
constant
during
the
measuring
interval.
In
other
words,
the
true
dynamical
system
is
not
really
autonomous
but
to
a
certain
extent,
time
dependent.
Thus,
we
are
asking
under
what
conditions
an
autonomous
system
is
structurally
stable
when
it
is
perturbed
to
a
time
dependent
system.
Franks
[1974]
gave
a
solution
for
C
2
diffeomorphisms
on
compact
manifolds:
Theorem
6.5.5
If
f:
M
~
M
is
a C
2
diffeomorphism
of
a
compact
manifold,
then
f
is
time
dependent
stable
iff
f
satisfies
Axiom
A
and
the
strong
transversality
condition.
In
closing,
we
would
like
to
point
out
that
several
other
notions
of
stability
have
been
proposed
in
hope
that
they
might
be
generic,
nonetheless,
none
as
yet
has
been
completely
sucessful.
After
all,
maybe
it
is
too
optimistic
to
expect
to
find
a
single
natural
equivalence
relation
with
respect
to
which
stability
is
dense.
More
recently,
attention
has
been
focused
on
the
interesting
and
important
question
of
bifurcation
of
systems
due
mainly
to
R.
Thorn
265

[1975].
Which
we
shall
discuss
very
briefly
in
the
next
section.
In
a
series
of
papers,
Hirsch
[1982,
1985, 1988,
1989a,
1989b,
1989c]
has
studied
a
vector
field
in
n-space
determines
a
competitive
(or
cooperative)
system
of
differential
equations
provided
all
the
off-diagonal
terms
of
its
Jacobian
matrix
are
nonpositive
(or
nonnegative).
He
has
found
that
orthogonal
projection
along
any
positive
direction
maps a
limit
set
homeomorphically
and
equivariantly
onto
an
invariant
set
of
a
Lipschitz
vector
field
in
a
hyperplane.
And
limit
sets
are
nowhere
dense,
unknotted
and
unlinked.
In
other
words,
most
trajectories
are
stable
and
approach
stationary
points,
and
limit
sets
are
invariant
sets
of
systems
in
one
dimension
lower.
In
dimension
2
every
trajectory
is
eventually
monotone,
and
in
dimension
3 a
compact
limit
set
which
does
not
contain
an
equilibrium
is
a
closed
orbit
or
a
cylinder
of
closed
orbits.
Furthermore,
Hirsch
[1985]
has
found
that
a
cooperative
system
cannot
have
nonconstant
attracting
periodic
solutions.
The
persistent
trajectories
of
the
n-dimensional
system
are
studied
under
the
assumptions
that
the
system
is
competitive
and
dissipative
with
irreducible
Jacobian
matrices.
Then
it
is
shown
that
there
is
a
canonically
defined
countable
family
of
disjoint
invariant
open
(n-1)-cells
which
attract
all
nonconvergent
persistent
trajectories.
These
cells
are
Lipschitz
submanifolds
and
are
transverse
to
positive
rays.
Furthermore,
if
the
Jacobian
matrices
are
strictly
negative
then
there
is
a
closed
invariant
(n-1)-cell
which
attracts
every
persistent
trajectory.
In
3
dimensional
system,
the
existence
of
a
persistent
trajectory
implies
the
existence
of
a
positive
equilibrium.
It
is
then
shown
that
among
3-dimensional
systems
which
are
competitive
or
cooperative,
those
satisfying
the
generic
conditions
of
Kupka-
Smale
also
satisfy
the
conditions
of
Morse-Smale
and
are
therefore
structurally
stable.
This
provides
a new
and
easily
recognizable
class
of
systems
which
can
be
approximated
by
266

structurally
stable
systems.
For
three-dimensional
systems,
a
certain
type
of
positive
feedback
loop
is
shown
to
be
structurally
stable.
6.6
Bifurcation
As
we
have
discussed
earlier
at
the
beginning
of
Chapter
5,
the
most
important
systems
are
the
ones
which
can
be
used
to
model
the
dynamics
of
real
life
situations.
But
rarely
can
real
life
situations
ever
be
exactly
described,
and
we
should
expect
to
lead
to
slight
variations
in
the
model
system.
Consequently,
a
theory
making
use
of
qualitative
features
of
a
dynamical
system
is
not
convincing
nor
has
its
utility
unless
the
features
are
shared
by
"nearby"
systems.
That
is
to
say
that
good
models
should
possess
some
form
of
qualitative
stability.
Hence
our
contempt
for
extremely
unstable
systems.
Furthermore,
in
a
given
physical
situation,
there
may
be
factors
present
which
rule
out
certain
dynamical
systems
as
models.
For
instance,
conservation
laws
or
symmetry
have
this
effect.
In
this
case,
the
subset
of
these
dynamical
systems
that
are
admissible
as
models
may
be
nowhere
dense
in
the
space
of
all
systems,
and
thus
the
stable
systems
that
we
are
considering
are
really
irrelevant.
Thus
one
has
to
consider
afresh
which
properties
are
generic
in
the
space
of
admissible
systems!
On
the
other
hand,
even
if
the
usual
space
of
systems
is
the
relevant
one,
the
way
in
which
a
system
loses
its
stability
due
to
perturbation
may
be
of
importance,
since
the
model
for
an
event
consists
of
a
whole
family
of
systems.
In
his
theory
of
morphogenesis,
Thom
envisions
a
situation
where
the
development
of
a
biological
organism,
say,
is
governed
by
a
collection
of
dynamical
systems,
one
for
each
point
of
space
time.
Bifurcation
is
a
term
which
has
been
used
in
several
areas
of
mathematics.
In
general,
it
refers
to
a
qualitative
change
of
the
object
under
study
due
to
change
of
parameters
on
which
the
object
depends.
For
the
kinds
of
applications
267

we
have
in
mind,
the
following
more
precise
definition
suffices.
Let
X
andY
be
Banach
spaces,
U c X,
and
F:
U
~
Y.
Suppose
there
is
a
one-to-one
curve
r =
(x(t):
t E
(0,1)}
c
u
such
that
for
z E
r,
F(z)
=
o.
A
point
p e r
is
a
bifurcation
point
for
F
with
respect
to
r
(more
simply
a
bifurcation
point)
if
every
neighborhood
of
p
contains
zeros
of
F
not
in
r.
In
most
applications,
possibly
after
making
a
change
of
variables,
one
usually
has
X = RxB
where
B
is
a
real
Banach
space,
F =
F(a,u),
and
r =
((a,O):
a E
(a,b)
c
R}.
Here,
the
members
of
r
will
be
called
trivial
solutions
of
F(a,u)
= o.
Thus,
we
are
interested
in
nontrivial
zeros
of
F.
We
would
like
to
mention
several
models
of
phenomena
to
illustrate
the
motivation
for
studying
bifurcation.
First,
an
infinite
horizontal
layer
of
a
viscous
incompressible
fluid
lies
between
a
pair
of
perfectly
conducting
plates.
A
temperature
gradient
T
is
maintained
between
the
plates,
the
lower
plate
being
warmer.
If
T
is
appropriately
small,
the
fluid
remains
at
rest,
the
heat
is
transported
through
the
fluid
solely
by
conduction,
and
the
temperature
is
a
linear
function
of
the
vertical
height.
When T
exceeds
a
certain
value,
the
fluid
undergoes
time-independent
motions
called
convection
current
and
heat
is
transpoted
through
the
fluid
by
convection
and
conduction.
In
actual
experiments,
the
fluid
breaks
up
into
cells
whose
shape
depends
in
part
on
the
shape
of
the
container.
This
is
called
Benard
instability.
Mathematically,
the
equilibrium
configuration
of
the
fluid
is
described
by
a
system
of
nonlinear
partial
differential
equations.
Formulated
in
the
general
Banach
space
framework,
the
pure
conduction
solutions
correspond
to
the
trivial
solutions,
while
the
value
of
T
at
which
convection
begins
corresponds
to
a
bifurcation
point.
For
reference,
see,
for
instance
the
classic,
Chandrasekar
[1961].
See
also
Kirchgassner
and
Kielhofer
[1973].
Another
interesting
problem
in
fluid
motion
is
the
Taylor
problem
of
rotating
fluid.
A
viscous
incompressible
268

fluid
lies
between
a
pair
of
concentric
cylinders
whose
axis
of
rotation
is
the
symmetry
axis
which
is
vertical.
The
inner
cylinder
rotates
at
a
constant
angular
velocity
n
while
the
outer
one
remains
at
rest.
If
n
is
sufficiently
small,
the
fluid
particles
move
in
circular
orbit
with
velocity
depending
on
their
distance
from
the
axis
of
rotation.
Equilibrium
states
of
the
fluid
are
solutions
of
the
time-independent
Navier-Stokes
equations,
and
they
are
called
Couette
flow.
When n
exceeds
a
critical
value,
the
fluid
breaks
up
into
horizontal
bands
called
Taylor
vortices
and
a
new
periodic
motion
in
the
vertical
direction
is
superimposed
on
the
couette
flow.
Here
Couette
flow
corresponds
to
the
trivial
solutions
in
the
general
framework,
and
the
values
n
at
which
the
onset
of
Taylor
vortices
taking
place
corresponds
to
a
bifurcation
point.
Buckling
phenomena
of
a
flat
plate
is
another
example
of
bifurcation.
A
thin,
planar,
clamped
elastic
plate
is
subjected
to
a
compressive
force
along
its
edges.
If
the
magnitude
of
this
compressive
force
f
is
small
enough,
the
plate
remains
motionless
and
in
equilibrium.
But
if
f
exceeds
a
certain
value,
the
plate
deflects
out
of
the
plane
and
assumes
a
nonplanar
equilibrium
position
called
a
buckled
state.
Equilibrium
configurations
of
the
plate
satisfy
a
system
of
nonlinear
partial
differential
equation
called
the
von
Karman
equations.
The
unbuckled
states
are
trivial
solutions
of
these
equations,
while
the
value
of
f
at
which
buckling
taking
place
corresponds
to
a
bifurcation
point.
See,
for
example,
Friedrichs
and
Stoker
[1941);
Berger
and
Fife
[1968);
Keller
and
Autman
[1969);
Berger
[1977].
Thompson
[1979]
has
shown
that
elastic
structure
under
dead
and
rigid
loadings
can
assess
the
stable
regions
through
a
succession
of
folds,
and
the
examples
of
buckling
of
elastic
arches,
shallow
domes
and
the
incipient
gravitational
collapse
of
a
massive
cold
star
are
demonstrated.
A
closely
related
mechanical
phenomenon
has
been
described
by
Duffing's
equation.
The
book
by
Guckenheimer
and
Holmes
[1983]
gives
a
very
detailed
study
269

of
the
four
systems,
namely
Vander
Pol's
equation
for
nonlinear
electronic
oscillator,
Duffing's
equation
for
stiffed
spring
with
cubic
stiffness,
Lorenz
equation
for
two-dimensional
fluid
layer
heated
from
below,
and
a
bouncing
ball
on
a
vibrating
table.
This
book
is
highly
recommended.
The
applications
of
these
systems
are
far
beyond
their
original
problems.
We
shall
see
this
in
Chapter
7.
For
an
elementary
yet
detailed
discussion
of
low
dimensional
bifurcation,
the
book
by
Iooss
and
Joseph
[1980]
is
recommended.
A
very
recent
book
by
Ruelle
[1989]
is
also
highly
recommended.
A
much
more
advanced
treatment
of
bifurcation
theory,
Chow
and
Hale
[1982]
is
indispensible.
There
are
also
many
interesting
applications
of
bifurcation
theory
in
other
physical
and
nonphysical
sciences,
such
as
chemical
reactions,
geophysics,
atmospherical
science,
biology,
and
social
science.
Let
us
return
to
the
general
theory
of
bifurcation,
where
there
are
three
main
questions
of
interest:
(a)
What
are
the
necessary
and
sufficient
conditions
for
(a,O)
E r
to
be
a
bifurcation
point?
(b)
What
is
the
structure
of
the
set
of
zeros
of
F(a,u)
near
(a,O)?
(c)
In
problems
such
as
described
above,
where
there
is
an
underlying
evolution
equation
of
which
the
solutions
described
are
equilibrium
solutions,
determine
which
solutions
are
stable
or
unstable.
For
the
detailed
"mechanism"
of
the
bifurcation,
see
Holmes
and
Rand
[1978].
For
the
forced
van
der
Pol-Duffing
oscillator
applies
to
the
trubulence
flow,
the
routes
to
turbulence
are
discussed
in
Coullet,
Tresser
and
Arneodo
[1980].
A
catastrophe
is
a
point
where
the
form
of
the
organism
changes
discontinuously,
this
corresponds
to
topological
change
in
the
orbit
structure
of
the
dynamical
systems.
We
say
that
the
family
of
dynamical
systems
bifurcates
at
the
point
where
the
change
is
discontinuous.
Let
us
give
some
simple
examples
of
bifurcations
of
flows.
There
are
local
changes
which
can
happen
on
any
manifold.
For
convenience
and
simplicity,
let
us
take
a
270
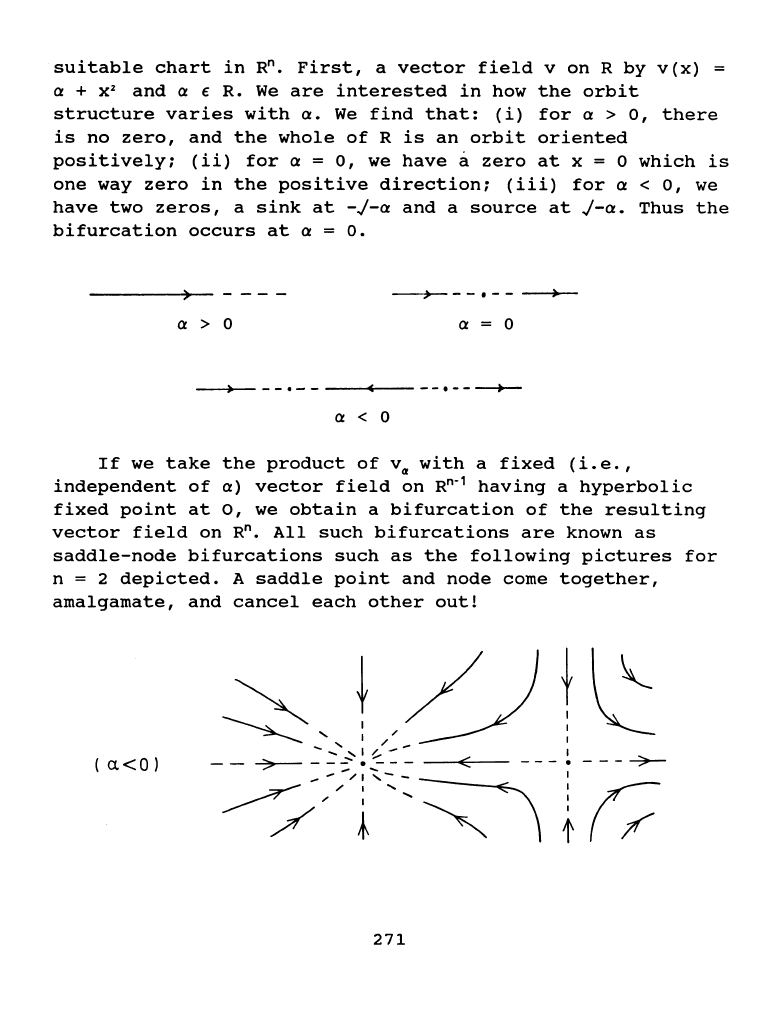
suitable
chart
in
R".
First,
a
vector
field
v
on
R
by
v(x)
=
a + X
2
and
a f
R.
We
are
interested
in
how
the
orbit
structure
varies
with
a.
We
find
that:
(i)
for
a > o,
there
is
no
zero,
and
the
whole
of
R
is
an
orbit
oriented
positively;
(ii)
for
a =
0,
we
have
a
zero
at
X = 0
which
is
one
way
zero
in
the
positive
direction;
(iii)
for
a <
0,
we
have
two
zeros,
a
sink
at
-J-a
and
a
source
at
J-a.
Thus
the
bifurcation
occurs
at
a =
o.
----~-----
~--·---
a > 0
a = o
-
--·--
--f----•----+--
a < 0
If
we
take
the
product
of
va
with
a
fixed
(i.e.,
independent
of
a)
vector
field
on
R""
1
having
a
hyperbolic
fixed
point
at
0,
we
obtain
a
bifurcation
of
the
resulting
vector
field
on
R".
All
such
bifurcations
are
known
as
saddle-node
bifurcations
such
as
the
following
pictures
for
n = 2
depicted.
A
saddle
point
and
node
come
together,
amalgamate,
and
cancel
each
other
out!
( a.<O J
271
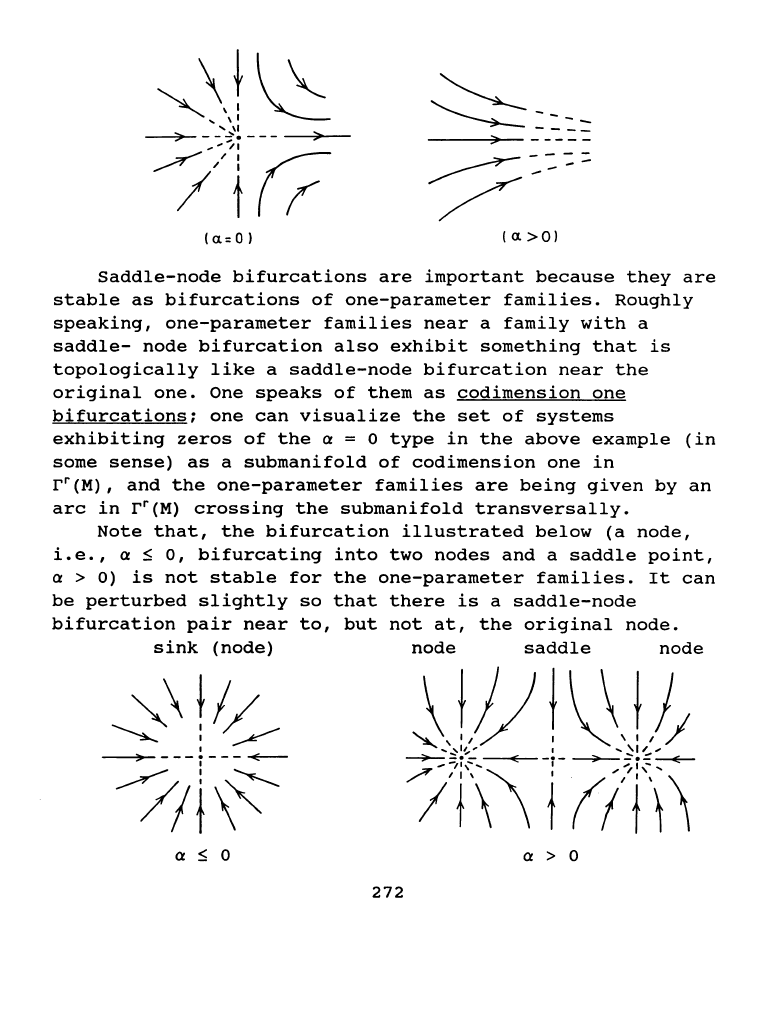
"\t~
'\I
~--~----
--i'--
'//irr
~
............._
~--
~
--
----
..------:::
::
: ::
/
(a=O
l
(«>0)
Saddle-node
bifurcations
are
important
because
they
are
stable
as
bifurcations
of
one-parameter
families.
Roughly
speaking,
one-parameter
families
near
a
family
with
a
saddle-
node
bifurcation
also
exhibit
something
that
is
topologically
like
a
saddle-node
bifurcation
near
the
original
one.
One
speaks
of
them
as
codimension
one
bifurcations:
one
can
visualize
the
set
of
systems
exhibiting
zeros
of
the
a = 0
type
in
the
above
example
(in
some
sense)
as
a
submanifold
of
codimension
one
in
r•(M),
and
the
one-parameter
families
are
being
given
by
an
arc
in
r•(M)
crossing
the
submanifold
transversally.
Note
that,
the
bifurcation
illustrated
below
(a
node,
i.e.,
aS
o,
bifurcating
into
two
nodes
and
a
saddle
point,
a >
0)
is
not
stable
for
the
one-parameter
families.
It
can
be
perturbed
slightly
so
that
there
is
a
saddle-node
bifurcation
pair
near
to,
but
not
at,
the
original
node.
sink
(node)
node
saddle
node
a S 0
a > o
272

The
saddle-node
bifurcation
is
the
typical
bifurcation
resulting
when
the
sign
of
a
real
eigenvalue
of
the
differential
at
a
zero
is
changed
by
varying
a
single
parameter
governing
the
system.
There
is
a
typical
codimension
one
bifurcation
which
comes
about
when
the
sign
of
the
real
part
of
a
complex
conjugate
pair
of
eigenvalues
is
changed
by
varying
a
single
parameter.
This
is
known
as
the
Hopf
bifurcation
[Hopf
1943].
For
instance,
consider
the
vector
field
va
on
R
2
given
by
va(x,y)
=
(-y-
x(a
+ X
2
+
y•),
x-
y(a
+ X
2
+ Y
2
)).
Here
a
is
a
single
real
parameter.
For
all
a £ R,
va
has
a
zero
at
the
origin,
and
the
linear
terms
make
this
a
spiral
source
for
a < 0
and
a
spiral
sink
for
a >
0.
For
a = o,
the
linear
terms
would
give
a
center,
but
the
cubic
terms
make
the
orbits
spiral
weakly
inwards.
The
interesting
feature
is
the
unique
closed
orbit
one
obtains
at
x•
+
y•
= - a
for
each
a
<
0.
The
bifurcation
is
illustrated
in
the
following:
a < 0
a S o
That
is,
the
periodic
attractor
(or
orbit)
decreases
in
size
until
it
amalgamates
with
the
spiral
source
to
form
a
spiral
sink.
It
is
intriguing
to
note
that
the
reverse
bifurcation
can
happen
where
a
spiral
sink
splits
into
a
spiral
source
and
a
periodic
attractor.
This
is
because
when
one
has
something
from
an
inert
(or
dead)
source,
one
creates
a
pulsating
(and
alive)
periodic
orbit.
Even
for
a
simple
recurrence
equation,
x(t+1)
=
Ax(t)[1-
x(t-1)],
which
is
analogous
to
the
logistic
model,
is
found
to
show
Hopf
bifurcation
[Morimoto
1988].
It
has
a
fixed
point
at
zero
for
A£
[0,1),
and
at
1-
1/A
for
A£
[1,2),
and
the
fixed
point
is
destabilized
at
A=
2.
For
A > 2
the
273
