Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


oscillatory
behavior
appears,
which
is
Hopf
bifurcation.
Recently,
Hale
and
Scheurle
(1985)
investigated
the
smoothness
of
bounded
solutions
of
nonlinear
evolution
equations
and
they
have
found
that
in
many
cases
globally
defined
bounded
soutions
of
evolution
equations
are
as
smooth
in
time
as
the
corresponding
operator,
even
if
a
general
solution
of
the
initial
value
problem
is
much
less
smooth.
In
other
words,
initial
values
for
bounded
solutions
are
selected
in
such
a way
that
optimal
smoothness
is
attained.
In
particular,
solutions
which
bifurcate
from
certain
steady
states,
such
as
periodic
orbits,
almost
periodic
orbits,
homo-
and
heteroclinic
orbits,
have
this
property.
Recently,
Baer
and
Erneux
(1986]
have
studied
the
singular
Hopf
bifurcation
from
a
basic
steady
state
to
relaxation
oscillation
characterized
by
two
quite
different
time
scales
of
the
form
dxjdt
=
f(x,y,a,e)
and
dyjdt
=
eg(x,y,a,e)
where
e << 1
and
is
the
control
parameter.
Their
bifurcation
analysis
shows
how
the
harmonic
oscillations
near
the
bifurcation
point
progressively
change
to
become
pulsed,
triangular
oscillations.
They
further
presented
a
numerical
study
of
the
FitzHugh-Nagumo
equations
for
nerve
conduction.
They
also
considered
the
switching
from
a
stable
steady
state
to
a
stable
periodic
solution,
or
the
reverse
transition.
Baer
et
al
(1989)
further
expanded
their
study
of
the
FitzHugh-Nagumo
model
of
nerve
membrane
excitability
as
a
delay
or
memory
effect.
It
can
occur
when a
parameter
passes
slowly
through
a
Hopf
bifurcation
point
and
the
system's
response
changes
from
a
slowly
varying
steady
state
to
slowly
varying
oscillations.
Next,
let
us
briefly
discuss
and
state
the
center
manifold
theorem,
which
provides
a mean
for
systematically
reducing
the
dimension
of
the
state
spaces
needed
to
be
considered
when
analyzing
bifurcations.
Later
in
this
chapter,
we
shall
use
the
Lorenz
system
and
its
bifurcations
as
an
example
to
illustrate
the
role
of
center
manifold
theorem
in
bifurcation
calculations.
274

Suppose
we
have
an
autonomous
dynamical
system
dxjdt
=
f(x)
such
that
f(O)
= o.
If
the
linearization
of
f
at
the
origin
has
no
pure
imaginary
eigenvalues,
then
Hartman's
linearization
theorem
(Theorem
4.6.6)
states
that
the
numbers
of
eigenvalues
with
positive
and
negative
real
parts
determine
the
topological
equivalence
of
the
flow
near
the
origin.
If
there
are
eigenvalues
with
zero
real
parts,
then
the
flow
can
be
quite
complicated
near
the
origin.
We
have
seen
such
situations
before.
Let
us
consider
the
following
system:
dxjdt
=
xy
+ xl,
dyjdt
=
-y
-x•y.
We
will
not
go
into
any
detail
to
analyze
the
above
system.
It
suffices
to
say
that
one
of
the
eigenvalues
is
-1
and
hence
a
one-dimensional
stable
manifold
exists
(indeed,
the
y-axis).
Direct
calculation
shows
that
the
x-axis
is
a
second
invariant
set
tangent
to
the
center
eigenspace
Ec.
This
is
an
example
of
a
center
manifold,
an
invariant
manifold
tangent
to
the
center
eigenspace.
Let
us
give
a
simple
example
before
stating
the
main
theorem.
The
following
example
is
due
to
Kelley
[1967]
which
also
gave
the
first
full
proof
of
the
main
theorem
we
shall
state
shortly.
Let
us
consider
the
very
simple
system:
dxjdt
= x• ,
dyjdt
=
-y.
The
parametric
solutions
to
this
system
have
the
following
form:
x(t)
=
a/(1-
at),
y(t)
=
be-t.
By
eliminating
t,
we
have
the
solution
curves
which
are
graphs
of
the
functions
y(x)
=
(be-
118
)e
11
x.
Clearly,
for
x <
o,
all
of
these
solution
curves
approach
the
origin
in
such
a way
that
all
of
their
derivatives
vanish
at
x =
0.
While
for
x
~
o,
the
only
solution
curve
approaches
the
origin
is
the
x-axis.
Thus,
the
center
manifold
is
not
unique.
Indeed,
we
can
obtain
a
~
center
manifold
by
piecing
together
any
solution
curve
in
the
left
half
plane
with
the
positive
half
of
the
x-axis.
The
center
manifolds
(heavy
curves)
are
shown
in
the
following
figure.
Nonetheless,
the
only
analytic
center
manifold
is
the
x-axis
itself.
275

Theorem
6.6.1
(Center
manifold
theorem)
Let
f
be
a cr
vector
field
on
R"
vanishing
at
the
origin
and
let
A =
Df(O).
Let
us
divide
the
spectrum
of
A
into
three
parts,
namely,
as,
ac,
au
with
Re
~
< 0
if
~
e
as,
Re
~
= 0
if
~
e
ac,
and
Re
~
> 0
if
~
e au.
Let
us
denote
the
eigenspaces
of
a
5
, ac,
and
au
by
Es, Ec,
and
Eu
respectively.
Then
there
exist
cr
stable
and
unstable
invariant
manifolds
ws
and
wu
tangent
to
E
5
and
Eu
at
0
and
a
cr-l
center
manifold
we
tangent
to
Ec
at
0.
The
manifolds
Ws
are
all
invariant
to
the
flow
of
f,
and
both
the
stable
and
unstable
manifolds
are
unique,
but
the
center
manifold
need
not
be.
In
general,
the
center
manifold
method
isolates
the
complicated
asymptotic
behavior
by
locating
an
invariant
manifold
tangent
to
the
subspace
spanned
by
the
eigenspace
of
eigenvalues
on
the
imaginary
axis.
As
we
have
noted
in
the
example
and
in
the
theorem,
there
are
technical
difficulties
involving
the
nonuniqueness
and
the
loss
of
smoothness
of
the
invariant
center
manifold
which
are
not
present
in
the
invariant
stable
manifold.
For
further
examples
and
detailed
discussion
including
the
existence,
uniqueness,
and
smoothness
of
center
manifolds
and
the
proof
of
the
above
theorem,
see,
e.g.,
Marsden
and
McCracken
[1976],
Carr
[1981],
Chow
and
Hale
[1982],
Guckenheimer
and
Holmes
[1983].
After
Guckenheimer
and
Holmes
[1983],
Guckenheimer
published
a
long
paper
about
multiple
bifurcations
with
276

multiple
degeneracy
in
some
features
of
the
system
and
a
multi-parameter
in
its
definition.
Multiple
bifurcations
occur
in
the
mathematical
descriptions
of
many
natural
phenomena,
and
more
importantly
provide
a
means
of
organizing
the
understanding
of
simple
bifurcations
and
also
provide
a
powerful
analytic
tool
for
locating
complicated
dynamical
behavior
in
some
models.
This
paper
is
highly
recommended,
and
one
may
consider
this
paper
as
an
appendix
to
the
book
mentioned.
Although
the
phenomena
of
Hopf
bifurcations
depending
on
some
autonomous
external
parameters
are
well
understood,
nonetheless,
the
parametrically
perturbed
Hopf
bifurcations
have
not
received
enough
attention.
The
effects
of
periodic
perturbation
of
a
bifurcating
system
have
been
considered
by
Rosenblat
and
Cohen
[1980,
1981]
and
Kath
[1981],
nonetheless,
they
neglected
to
examine
any
possible
secondary
bifurcations
which
may
exist
in
these
systems.
Sri
Namachchivaya
and
Ariaratnam
[1987]
studied
small
periodic
perturbations
on
two-dimensional
systems
exhibiting
Hopf
bifurcations
in
detail,
and
obtained
explicit
results
for
various
primary
and
secondary
bifurcations,
and
their
stabilities.
Here
the
center
manifold
theorem
and
other
techniques
are
utilized.
In
addition
to
the
above
suggested
reading
list,
Iooss
and
Joseph
[1980]
and
Ruelle
[1989]
are
recommended.
Before
we
end
this
section,
let
us
briefly
discuss
the
unfolding
of
singularities
and
describe
the
elementary
catastrophes.
Intuitively,
unfoldings
means
that
we
embed a
singularity
of
a map
in
a
higher
dimensional
domain,
so
that
the
"bigger"
map
offers
some
insights
and
advantages.
Let
f
be
a
finite
sum
of
products
of
two
elements,
each
of
which
from
those
germs
f:
R"
~
R
for
which
f(O)
=
o.
So,
f
has
a
singularity
at
o.
An
unfolding
off
is
a
germ
f':
R~r
~
R,
with
f'(O)
=
0,
such
that
if
x E R",
f'(x,O)
=
f(x).
Of
course,
here
0 =
(0,
•••
,0)
with
r
entries.
The
unfolding
f'
have
r-parameters.
Note
that
the
constant
unfolding
f',
defined
by
f(x,y)
=
f(x).
We
say
that
f'
(with
277
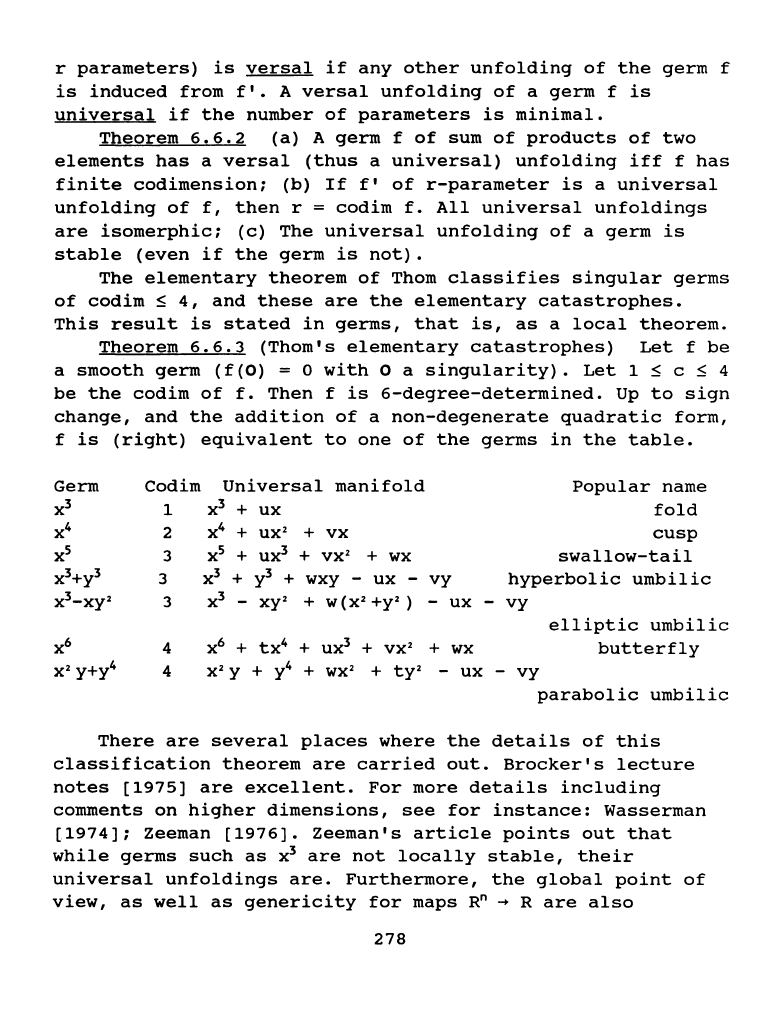
r
parameters)
is
versal
if
any
other
unfolding
of
the
germ
f
is
induced
from
f'.
A
versal
unfolding
of
a
germ
f
is
universal
if
the
number
of
parameters
is
minimal.
Theorem
6.6.2
(a)
A
germ
f
of
sum
of
products
of
two
elements
has
a
versal
(thus
a
universal)
unfolding
iff
f
has
finite
codimension;
(b)
If
f'
of
r-parameter
is
a
universal
unfolding
of
f,
then
r =
codim
f.
All
universal
unfoldings
are
isomerphic;
(c)
The
universal
unfolding
of
a
germ
is
stable
(even
if
the
germ
is
not).
The
elementary
theorem
of
Thorn
classifies
singular
germs
of
codim
~
4,
and
these
are
the
elementary
catastrophes.
This
result
is
stated
in
germs,
that
is,
as
a
local
theorem.
Theorem
6.6.3
(Thorn's
elementary
catastrophes)
Let
f
be
a
smooth
germ
(f(O)
= 0
with
0 a
singularity).
Let
1
~
c
~
4
be
the
codim
of
f.
Then
f
is
6-degree-determined.
Up
to
sign
change,
and
the
addition
of
a
non-degenerate
quadratic
form,
f
is
(right)
equivalent
to
one
of
the
germs
in
the
table.
Germ
Codim
x3
1
x4
2
xs
3
x3+y3
3
x3-xy2
3
x6
4
x2
y+y4
4
Universal
manifold
x3
+
ux
x
4
+
ux
2
+
vx
xs
+
ux
3
+
vx
2
+ wx
x3+y3+
wxy
-
ux
-
x3
-
xy2
+
w(x2+y2)
x6 +
tx
4
+
ux
3
+
vx
2
x2
y +
y4
+
wx2
+
ty2
vy
-
ux
+ wx
-
ux
Popular
name
fold
cusp
swallow-tail
hyperbolic
umbilic
-
vy
-
vy
elliptic
umbilic
butterfly
parabolic
umbilic
There
are
several
places
where
the
details
of
this
classification
theorem
are
carried
out.
Brocker's
lecture
notes
[1975)
are
excellent.
For
more
details
including
comments
on
higher
dimensions,
see
for
instance:
Wasserman
[1974);
Zeeman
[1976).
Zeeman's
article
points
out
that
while
germs
such
as
x
3
are
not
locally
stable,
their
universal
unfoldings
are.
Furthermore,
the
global
point
of
view,
as
well
as
genericity
for
maps
R"
~
R
are
also
278

discussed.
For
those
readers
who
may
want
to
pursue
the
details
and
specifics
of
this
theory,
one
needs
at
least
the
following
background:
(i)
Some
ring
theory
to
get
to
the
basic
results
of
Mather
et
al
on
finite
determination
and
codimension.
(ii)
The
Malgrange
preparation
theorem
which
generalizes
to
the
smooth
case
a
famous
theorem
of
Weierstrass
from
several
complex
variables.
See
for
instance:
Brocker
[1975],
Malgrange
[1964];
a
chapter
in
Golubitsky
and
Guillemin
[1973].
(iii)
Some
basic
algebraic
geometry.
Catastrophe
theory
has
brought
with
it
a
wealth
of
applications,
however
the
risk
of
oversimplification
in
applications
are
enormous.
Prudent
caution
is
required!
There
are
several
concrete
examples
of
applications
of
catastrophe
theory,
such
as:
in
optics
[Berry
and
Upstill
1980],
relativity
[Barrow
1981,
1982a,b],
geophysics
[Gilmore
1981],
particle
scattering
from
surface
[Berry
1975],
rainbow
effect
in
ion
channeling
in
very
thin
crystal
[Neskovic
and
Perovic
1987],
elastic
structure
under
dead
and
rigid
loadings
assess
the
stable
regions
of
an
equilibrium
path
which
exhibits
a
succession
of
folds
[Thompson
1979],
just
to
name
a
few.
Gilmore
[1981]
also
provides
some
other
applications
of
catastrophe
theory.
swallow
tail
279
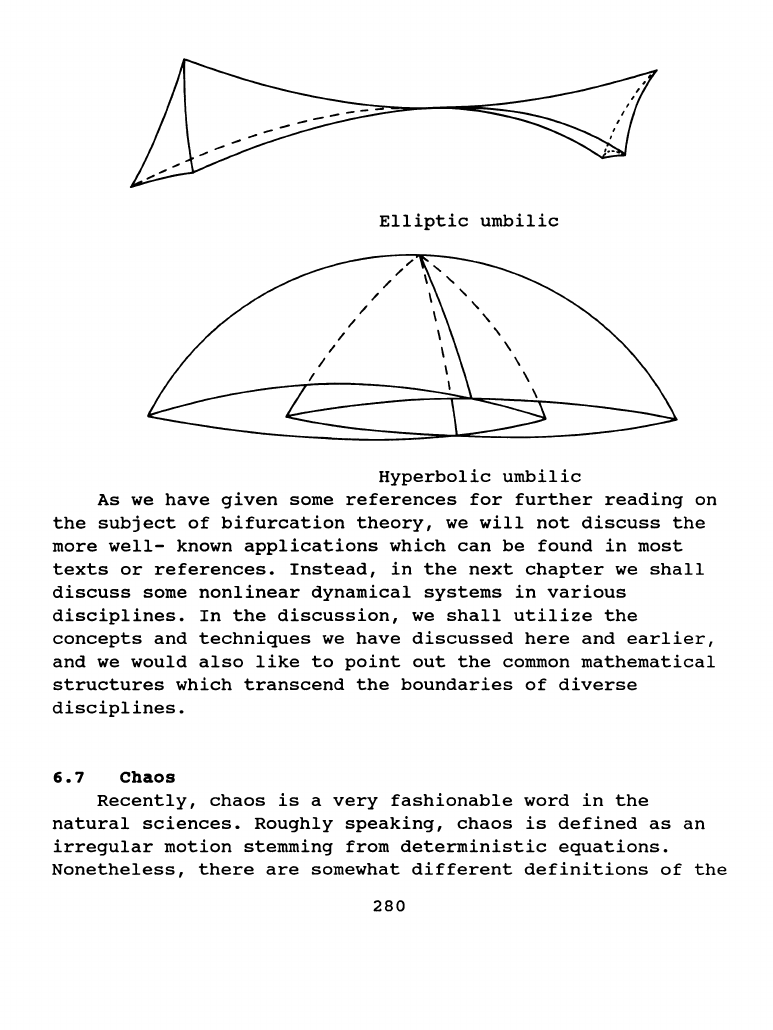
Elliptic
umbilic
Hyperbolic
umbilic
As
we
have
given
some
references
for
further
reading
on
the
subject
of
bifurcation
theory,
we
will
not
discuss
the
more
well-
known
applications
which
can
be
found
in
most
texts
or
references.
Instead,
in
the
next
chapter
we
shall
discuss
some
nonlinear
dynamical
systems
in
various
disciplines.
In
the
discussion,
we
shall
utilize
the
concepts
and
techniques
we
have
discussed
here
and
earlier,
and
we
would
also
like
to
point
out
the
common
mathematical
structures
which
transcend
the
boundaries
of
diverse
disciplines.
6.7
Chaos
Recently,
chaos
is
a
very
fashionable
word
in
the
natural
sciences.
Roughly
speaking,
chaos
is
defined
as
an
irregular
motion
stemming
from
deterministic
equations.
Nonetheless,
there
are
somewhat
different
definitions
of
the
280

term
chaos
in
the
literature.
The
difference
is
mainly
due
to
different
ways
of
defining
"irregular
motion".
Irregular
motion
of
stochastic
processes,
such
as
Brownian
motion,
occurs
due
to
random,
i.e.,
unpredictable,
causes
or
sources.
Thus
these
random
irregular
motions
are
uncorrelated.
We
do
not
consider
this
type
of
irregular
motion
as
chaotic.
Instead,
one
can
discuss
the
atypical
behavior
of
the
correlation
function
of
chaotic
processes.
There
are
a
number
of
introductory
articles
on
chaos.
The
following
are
a
partial
list.
Chernikov,
Sagdeev
and
Zaslavsky
(1988],
Chua
and
Madan
(1988],
Bak
(1986]
Crutchfield
et
al
(1986],
May
(1976]
and
Gleick
[1987].
The
following
review
articles
on
chaos
and
routes
to
chaos
are
highly
recommended:
Eckmann
[1981],
Ott
(1981],
and
Tomita
(1982]
for
nonlinear
oscillators.
There
are
also
many
introductory
or
popular
books
on
chaos.
Holden
[1986]
is
highly
recommended.
Prigogine
and
Stengers
[1984]
gives
a
nontechnical
account
of
order
and
chaos
and
raises
some
philosophical
issues.
It
stimulates
and
challenges
numerous
questions
and
thoughts,
nonetheless,
due
to
its
lack
of
specifics,
it
does
not
help
the
"advanced
beginners"
to
find
ways
or
approaches
for
the
solutions.
The
idea
of
chaos
has
been
applied
to
almost
any
subject.
In
addition
to
the
classical
applications
in
hydrodynamics
(for
instance,
even
in
the
periodical
laminar
flows
through
curved
pipes,
under
certain
conditions
the
flows
exhibit
period-
tripling,
which
is
reminiscent
of
one
of
the
routes
to
chaos
(Hamakiotes
and
Berger
1989]),
plasma
physics,
classical
mechanics,
nonlinear
feedback
control,
there
are
chaotic
phenomena
observed
or
predicted
in
optics,
chemical
and
biochemical
reactions,
semiconductor
physics,
interacting
population
and
delayed
feedback,
competitive
economy,
just
to
name
a
few.
We
shall
discuss
these
applications
in
next
chapter.
Here
we
just
want
to
point
out
a
few
examples
which
we
will
not
go
into
any
further
detail,
but
give
references
for
interested
readers
to
pursue
the
subjects.
For
instance,
Papantonopoulos,
Uematsu
and
281

Yanagida
[1987)
presented
a
chaotic
inflationary
model
of
the
universe,
in
which
nonlinear
interaction
of
dilaton
and
axion
fields,
in
the
context
of
the
super-conformal
theory,
can
dynamically
give
rise
to
the
initial
conditions
for
the
inflation
of
the
universe.
Buchler
and
Eichhorn
[1987]
discussed
various
chaotic
phenomena
in
astrophysics.
Also,
Fesser,
Bishop
and
Kumar
[1983]
have
shown
numerically
that
there
are
parameter
ranges
of
radio
frequency
(rf)
superconducting
quantum
interference
device
(SQUID)
for
chaotic
behavior.
They
have
shown
that
the
strange
attractor
characterizing
the
chaotic
regimes
can
be
described
by
a
one-dimensional
return
map.
Recently,
Herath
and
Fesser
[1987],
motivated
by
the
rf-SQUID
device
and
using
different
mode
expansions,
investigated
nonlinear
single
well
oscillators
driven
by
a
periodic
force
with
damping.
Very
recently,
Miles
[1988)
investigated
the
symmetric
oscillations
of
an
inverted,
lightly
damped
pendulum
under
direct
sinusoidal
force,
and
analytically
predicted
symmetry-breaking
bifurcations
and
numerically
confirmed.
Similar
results
were
also
obtained
for
Josephson
junction
circuit
by
Yeh
and
Kao
[1982],
Kautz
and
Macfarlane
[1986],
Yao
[1986]
and
Hadley
and
Beasley
[1987].
Damped,
driven
pendulum
systems
have
been
used
to
model
complicated
behavior
in
nonlinear
systems
successfully.
Varghese
and
Thorp
[1988]
chose
to
study
the
transiently
forced
pendulums
and
they
shifted
their
emphasis
to
the
composition
of
the
boundaries
separating
the
domains
of
attraction
of
the
various
asymptotically
stable
fixed
points.
A
simple
proof
of
the
existence
of
diffeomorphisms
from
connected
basins
to
striated
basins
is
also
presented.
Since
we
are
on
the
subject
of
effects
of
superconducting
material,
it
is
interesting
to
note
that
a
series
of
papers
on
the
nonlinear
hysteretic
forces
due
to
superconducting
materials
have
been
published
by
the
Cornell
group
[Moon,
Yanoviak
and
Ware
1989,
Moon,
Weng
and
Chang
1989].
Moon
[1988)
has
demonstrated
the
period
doubling
and
chaos
for
the
forced
vibration
due
to
the
nonlinear
282

hysteretic
force
of
a
small
permanent
magnet
near
the
surface
of
a
high
temperature
superconducting
disk.
The
forces
are
believed
to
be
related
to
flux
pinning
and
flux
dragging
effects
in
the
superconductor
of
type
II
state.
Based
on
the
displacement
of
the
magnet,
a
return
map
under
iteration
exhibits
a
bifurcation
structure
similar
to
the
experimental
results
obtained.
In
fact,
much
earlier,
Huberman
and
Crutchfield
[1979],
Huberman,
Crutchfield
and
Packard
[1980]
have
shown
that
the
nonlinear
dynamics
of
anharmonically
interacting
particles
under
periodic
fields
resulted
in
a
set
of
cascading
bifurcations
and
into
chaos.
Turschner
[1982]
has
presented
an
analytic
calculation
of
the
Poincare
section
of
the
driven
anharmonic
oscillator
based
on
proper
canonical
transformations.
Linsay
[1981]
has
demonstrated
periodic
doubling
and
chaotic
behavior
of
a
driven
anharmonic
oscillator
and
the
experimental
results
are
in
quantitative
agreement
with
the
theory
by
Feigenbaum
[1978,
1979].
Testa,
Perez
and
Jeffries
[1982]
have
also
experimentally
observed
successive
subharmonic
bifurcations,
onset
of
chaos,
and
noise
band
merging
from
a
driven
nonlinear
semiconductor
oscillator.
See
also,
[Wiesenfeld,
Knobloch,
Miracky
and
Clarke
1984].
Rollins
and
Hunt
[1982]
have
shown
that
both
a
finite
forward
bias
and
a
finite
reverse
recovery
time
are
required
if
the
diode
resonator
is
to
exhibit
chaos.
Furthermore,
this
anharmonic
oscillator
also
exhibits
period
tripling
and
quintupling.
Nozaki
and
Bekki
[1983]
have
shown
that
a
nonlinear
Schrodinger
soliton
behaves
stochastically
with
random
phases
in
both
time
and
space
in
the
presence
of
small
external
oscillating
fields
and
emits
small-amplitude
plane
waves
with
random
phases.
They
also
have
found
that
the
statistical
properties
of
random
phases
give
the
energy
spectra
of
the
soliton
and
plane
waves.
Bryant
and
Jeffries
[1984]
studied
a
forced
symmetric
oscillator
containing
a
saturable
inductor
with
magnetic
hysteresis,
approximated
by
a
noninvertible
map
of
the
plane.
The
system
displays
a
Hopf
bifurcation
to
quasiperiodicity,
entrainment
horns,
and
283
