Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


on
the
set
Rn
- K
and
for
all
( i
1
,
•••
, in)
with
i
1
+
•••
+
in
=
i
s k
we
have
I
cai;ax,i•
..•
axni") (F -
G)
I <
E.
F
and
G
can
also
be
vector
fields.
Note
also
that
this
definition
can
be
stated
in
terms
of
k-jets
as
introduced
in
Chapter
3.
Let
us
recall
that
two
cr
maps F
and
G
are
ck
(k
S
r)
equivalent
or
ck
conjugate
if
there
exists
a
ck
homeomorphism
h
such
that
h·F
=
G·h.
C
0
equivalence
is
called
topological
equivalence.
Two
cr
vector
fields
f
and
g
are
ck
(k
S
r)
equivalent
if
there
exists
a
ck
diffeormorphism
h
which
takes
orbits
of
f
to
orbits
of
g,
preserving
senses
but
not
necessarily
parameterization
by
time.
If
h
does
preserve
parameterization
by
time,
then
it
is
called
a
conjugacy.
Intuitively,
it
is
not
difficult
to
see
that
parameterization
by
time
cannot
be
preserved
in
general.
This
is
because
the
periods
of
closed
orbits
in
flows
can
be
different.
We
say
a map F e cr(R")
(or
a
cr
vector
field
f
respectively)
is
structurally
stable
if
there
is
an
e > 0
such
that
all
c
1
e-
perturbations
of
F
(resp.
of
f)
are
topologically
equivalent
to
F
(resp.
f).
Let
us
consider
a
small
perturbation
of
a
linear
system
dxjdt
=Ax,
x e R
2
:
dxjdt
=Ax+
ef(x),
where
f
has
support
in
some
compact
set.
Since
A
is
invertible,
then
by
the
implicit
function
theorem,
the
equation
Ax
+
ef(x)
= 0
continues
to
have
a
unique
solution
~
= o +
O(e)
near
x =
o,
for
sufficiently
small
e.
Since
the
matrix
of
the
linearized
system
dtjdt
=
(A+
eDf(~))t
has
eigenvalues
which
depend
continuously
on
e,
no
eigenvalues
can
cross
the
imaginary
axis
if
e
remains
small
with
respect
to
the
magnitude
of
the
real
parts
of
the
eigenvalues
of
A.
Thus
the
perturbed
system
has
a
unique
fixed
point
and
invariant
manifolds
of
the
same
dimensions
as
those
of
the
unperturbed
system,
and
which
are
e-close
locally
in
position
and
slope
to
the
unperturbed
manifolds.
The
problem
is
finding
a
homeomorphism
which
takes
orbits
of
the
linear
system
to
those
of
the
perturbed
nonlinear
system.
Structurally
stable
systems
have
"nice"
properties,
244

namely,
any
sufficiently
close
system
has
the
same
qualitative
behavior.
Nonetheless,
structurally
stable
behavior
can
be
very
complex
for
flows
of
dimension
~
3
or
diffeomorphisms
of
dimension
~
2.
It
should
be
stressed
that
the
definition
of
structural
stability
is
relative
to
the
class
of
systems
we
will
be
dealing
with.
In
fact,
structural
stability
is
not
even
a
generic
property,
we
shall
come
to
this
in
Section
6.3,
because
we
can
find
structurally
unstable
systems
which
remain
unstable
under
small
perturbations,
and
some,
in
fact,
continually
change
their
topological
equivalence
class
as
we
perturb
them.
We
shall
meet
such
systems
shortly.
The
idea
is
the
following.
Let
Diffr(M)
(1
~
r
~
oo)
be
the
set
of
all
cr-diffeomorphisms
of
the
ce-manifold
M,
provided
with
the
cr-topology.
We
say
that
f €
diffr(M)
is
structurally
stable
if
it
is
in
the
interior
of
its
topological
conjugacy
class.
In
other
words,
f
is
structurally
stable
iff
any
cr-
"small"
perturbation
takes
it
into
a
diffeomorphism
that
is
topologically
conjugate
to
f.
Similarly,
let
xr(M)
be
the
set
of
all
cr
vector
fields
on
M
topologized
with
the
cr
topology.
Then
the
vector
field
X € xr(M)
(or
the
corresponding
integral
flow
¢)
is
structurally
stable
if
it
is
in
the
interior
of
its
topological
equivalence
class.
This
type
of
definition
of
structural
stability
is
due
to
Andronov
and
Pontryagin
[1937].
This
concept
of
structural
stability
has
played
a
dominant
and
extremely
important
role
in
the
development
of
dynamical
systems
during
the
past
thirty
years.
It
not
only
has
helped
to
emphasize
the
global
point
of
view,
but
also
has
been
very
fruitful
in
stimulating
conjectures
culminating
in
the
powerful
theorems
we
shall
discuss
shortly.
Before
we
get
into
any
details
of
the
notion
of
structural
stability,
we
would
like
to
discuss
the
stable
manifolds
of
the
dynamical
system
at
a
fixed
point.
245

&.2
Stable
manifolds
of
diffeomorphisms
and
flows
If
we
look
at
a
phase
diagram
of
a
saddle
point
(Fig.6.2.1)
and
try
to
analyze
which
qualitative
features
give
the
saddle
point
its
characteristic
appearance,
we
are
bound
to
single
out
the
four
special
orbits
that
begin
or
end
at
the
fixed
point.
These
four
orbits
together
with
the
fixed
point
form
the
stable
manifolds
and
unstable
manifolds
of
the
dynamical
system
at
the
fixed
point.
In
Chapter
4
we
have
noted
the
importance
of
hyperbolicity
in
the
theory
of
dynamical
systems,
and
a
hyperbolic
structure
always
implies
the
presence
of
such
manifolds.
Thus,
if
we
know
where
the
"singular
elements"
of
a
system
are
(including
periodic
points
for
diffeomorphisms,
fixed
points
and
closed
orbits
of
flows),
and
if
we
also
know
the
way
in
which
their
stable
and
unstable
manifolds
fit
together,
we
then
have
a
very
good
idea
on
the
orbit
structure
of
the
system.
I~
,'~
I
"'
.J"'
I
t /
Fig.
6.2.1
Let
f:
M
~
M
be
a
diffeomorphism,
p
be
a
fixed
point
of
f.
The
stable
set
of
f
at
p
is
the
set
{x
E M :
f"(x)
~
p
as
n
~
~
}.
Note
that
this
set
is
always
non-empty
because
it
contains
p.
The
unstable
set
of
f
at
p
is
the
stable
set
of
f"
1
at
p.
For
any
open
neighborhood
U
of
p,
the
local
stable
set
of
flu
at
pis
the
set
of
all
x e U
such
that
{f"(x):
n
246
~
O}
is
a
sequence
in
U
converging
to
p.
One
can
show
that
if
p
is
hyperbolic
then
the
global
stable
set
is
an
immersed
submanifold
of
M
and
is
at
least
as
smooth
as
the
diffeomorphism
f.
This
submanifold
is
called
the
stable
manifold
off
at
p,
and
denoted
by
W
5
(p).
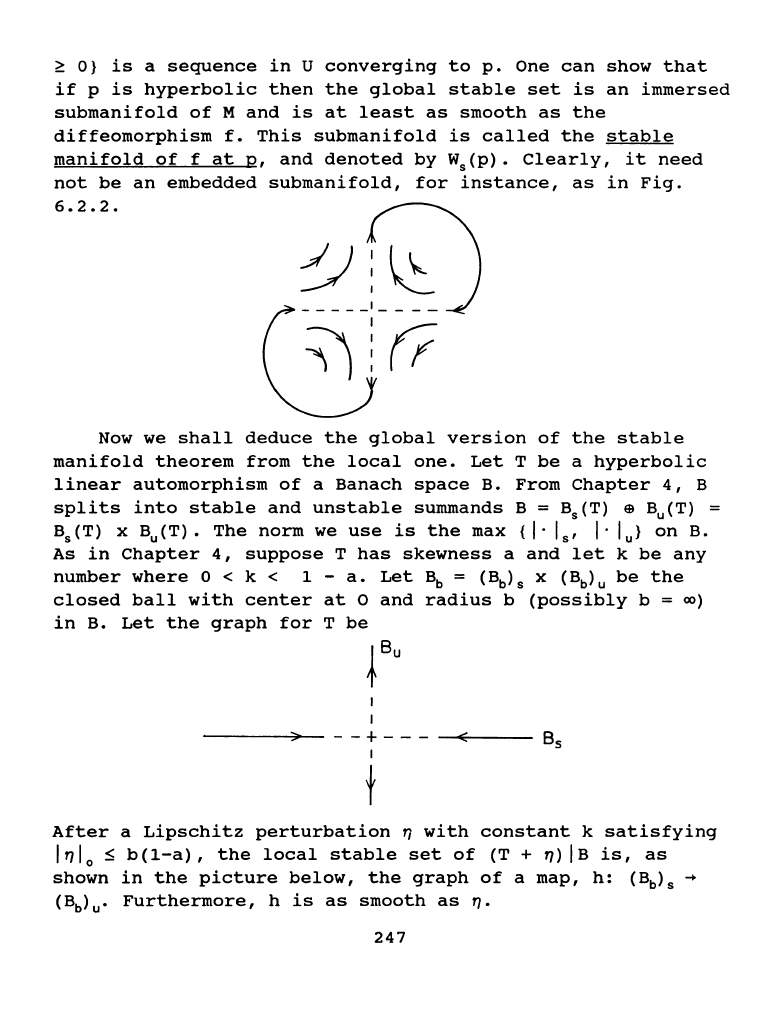
Clearly,
it
need
not
be
an
embedded
submanifold,
for
instance,
as
in
Fig.
6.2.2.
~:rr
Now
we
shall
deduce
the
global
version
of
the
stable
manifold
theorem
from
the
local
one.
Let
T
be
a
hyperbolic
linear
automorphism
of
a
Banach
space
B. From
Chapter
4,
B
splits
into
stable
and
unstable
summands B = B
5
(T) e Bu(T) =
B
5
(T) x
Bu(T).
The
norm
we
use
is
the
max
{I·
I
8
,
I· I
u>
on
B.
As
in
Chapter
4,
suppose
T
has
skewness
a
and
let
k
be
any
number
where
0 < k < 1 -
a.
Let
Bb
=
(Bb)
s x
(Bb)
u
be
the
closed
ball
with
center
at
o
and
radius
b
(possibly
b =
m)
in
B.
Let
the
graph
for
T
be
I
I
----~--
-+---
-E----
Bs
I
t
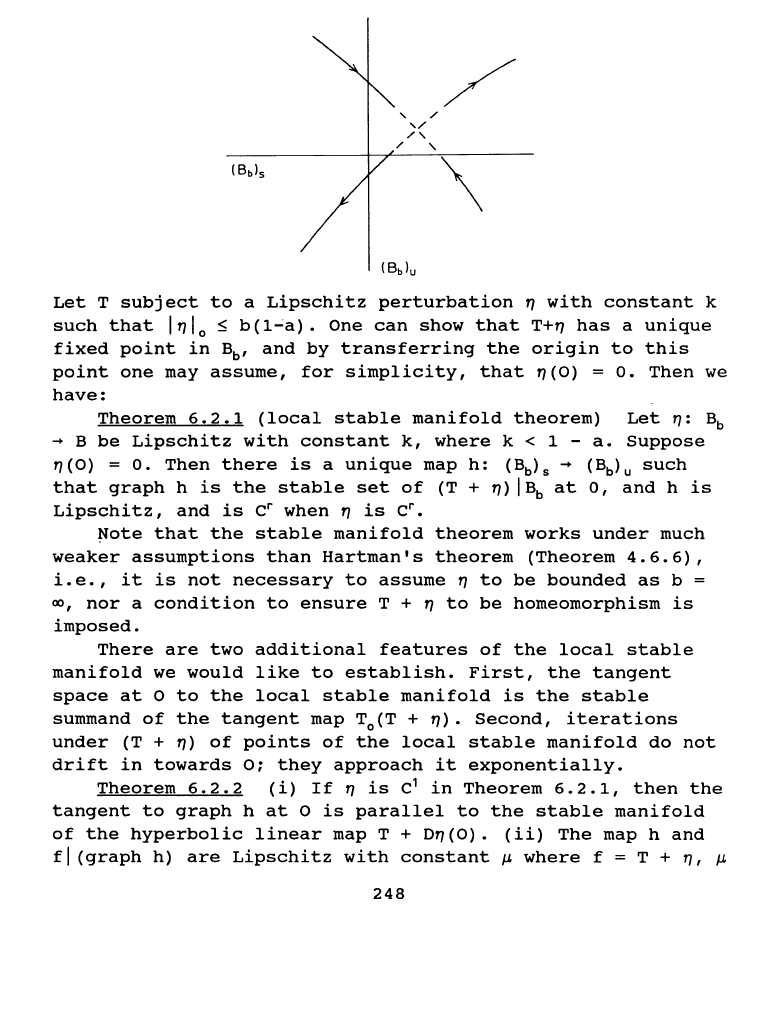
After
a
Lipschitz
perturbation
~
with
constant
k
satisfying
l~lo
S
b(l-a),
the
local
stable
set
of
(T +
~)IBis,
as
shown
in
the
picture
below,
the
graph
of
a map,
h:
(Bb)s
~
(Bb>u·
Furthermore,
h
is
as
smooth
as
~·
247
\
Let
T
subject
to
a
Lipschitz
perturbation
~
with
constant
k
such
that
l~lo
S
b(1~a).
One
can
show
that
T+~
has
a
unique
fixed
point
in
Bb,
and
by
transferring
the
origin
to
this
point
one
may
assume,
for
simplicity,
that
~(0)
= o.
Then
we
have:
Theorem
6.2.1
(local
stable
manifold
theorem)
Let
~=
Bb
~
B
be
Lipschitz
with
constant
k,
where
k < 1 -
a.
Suppose
~
(0)
=
0.
Then
there
is
a
unique
map
h:
(Bb) s
~
(Bb) u
such
that
graph
h
is
the
stable
set
of
(T
+
~)lab
at
o,
and
h
is
Lipschitz
I
and
is
cr
when
~
is
cr.
~ote
that
the
stable
manifold
theorem
works
under
much
weaker
assumptions
than
Hartman's
theorem
(Theorem
4.6.6),
i.e.,
it
is
not
necessary
to
assume~
to
be
bounded
as
b =
~,
nor
a
condition
to
ensure
T +
~
to
be
homeomorphism
is
imposed.
There
are
two
additional
features
of
the
local
stable
manifold
we
would
like
to
establish.
First,
the
tangent
space
at
0
to
the
local
stable
manifold
is
the
stable
summand
of
the
tangent
map
T
0
(T
+~).Second,
iterations
under
(T
+
~)
of
points
of
the
local
stable
manifold
do
not
drift
in
towards
o;
they
approach
it
exponentially.
Theorem
6.2.2
(i)
If
~
is
C
1
in
Theorem
6.2.1,
then
the
tangent
to
graph
h
at
0
is
parallel
to
the
stable
manifold
of
the
hyperbolic
linear
map
T +
D~(O).
(ii)
The
map
hand
fl
(graph
h)
are
Lipschitz
with
constant
~
where
f = T +
~'
~
248

= a + k <
1.
For
any
norm
II II
on
B
equivalent
to
norm
on
B,
there
exists
A > 0
such
that
for
all
n
~
0
and
for
all
x,y
e
graph
h,
llf"(x)
-
f"(y)
II
S
A~"llx
- Yll.
Theorem
6.2.3
Let
p
be
a
hyperbolic
fixed
point
of
a
c•
diffeomorphism
(r
~
1)
f
of
M.
Then,
for
some
open
neighborhood
U
of
p,
the
local
stable
set
of
flU
at
p
is
a
c•
imbedded
submanifold
of
M,
tangent
at
p
to
the
stable
summand
of
TPf.
Theorem
6.2.4
(global
stable
manifold
theorem)
Let
p
be
a
hyperbolic
fixed
point
of
c•
diffeomorphism
f
(r
~
1)
of
M.
Then
the
global
stable
set
S
of
f
at
p
is
a
c•
immersed
submanifold
of
M,
tangent
at
p
to
the
stable
summand
of
TPf.
If
one
looks
at
the
proof
of
the
above
theorem,
one
notices
that
the
nature
of
the
charts
~i
strongly
suggests
that
the
global
stable
manifold
of
f
at
p
is
an
immersed
copy
of
the
stable
manifold
of
the
linear
approximation.
Indeed,
by
extending
the
map
~
to
the
whole
of
B,
we
can
construct
a
locally
Lipschitz
bijection
of
the
Banach
space
onto
the
stable
manifold
of
f.
With
the
help
of
Theorem
6.2.3,
one
can
show
that
the
bijection
gives
a
c•
immersion
of
(Bb)s
in
X.
In
fact,
one
may
extend
the
above
theorem
from
fixed
points
to
periodic
points
of
a
diffeomorphism
with
little
extra
effort.
Indeed,
if
p
is
a
periodic
point
of
a
diffeomorphism
f:
M
~
M,
then
p
is
a
fixed
point
of
the
diffeomorphism
fk,
where
k
is
the
period
of
p.
Thus:
Theorem
6.2.5
Let
p
be
a
hyperbolic
periodic
point
of
period
k
of
a
c•
diffeomorphism
f
of
M
(r
~
1).
Then
the
stabe
set
of
f
at
p
is
a
c•
immersed
submanifold
of
M
tangent
at
p
to
the
stable
summand
of
TPfk.
Now
we
turn
to
the
stable
manifold
theory
for
flows.
Note
that
the
stable
manifold
theorem
for
a
hyperbolic
fixed
point
of
a
flow
is
a
simple
corollary
of
the
corresponding
theorem
for
diffeomorphisms.
Let
~
be
a
flow
on
M,
and
let
d
be
an
admissible
distance
function
on
M.
The
stable
set
of
0
at
p
eM
is
{x
eM:
d(~(t,x),~(t,p))
~
0
as
t
~
oo}
and
the
249

unstable
set
of
0
at
pis
(x
eM:
d(~(t,x),~(t,p))
~
0
as
t
~
~}.
Recall
that
if
pis
a
fixed
point
of~
then
~(t,x)
~
p
as
t
~
~
iff
~(n,x)
~
p
as
n
~
~,
where
t e R
and
n e z.
Thus
the
stable
set
of
the
flow
~
at
p
is
precisely
the
stable
set
of
the
diffeomorphism
~
1
at
p,
or
of
~t
for
any
other
t > o.
Thus
we
obtain
the
following
theorem.
Theorem
6.2.6
(global
stable
manifold
theorem)
Let
p
be
a
hyperbolic
fixed
point
of
a
c•
flow
(r
~
1)
~
on
M.
Then
the
stable
set
of
~
at
p
is
a
c•
immersed
submanifold
of
M,
tangent
at
p
to
the
stable
summand
of
TP~
1
•
Theorem
6.2.7
(stable
manifold
theorem
for
closed
orbits)
Let
r
be
a
hyperbolic
closed
orbit
of
a
c•
(r
~
1)
flow
~
on
a
manifold
M
with
distance
function
d.
The
stable
set
W
5
(p)
of
~
at
p
is
a
c•
immersed
submanifold
tangent
at
p
to
the
stable
summand
of
M~
with
respect
to
TP~T,
where
1
is
the
period
of
r.
If
q =
~
(p),
then
W
5
(q)
=
~t(W
5
(p)).
The
stable
set
W
5
(I')
of
~
at
r
is
a
c•
immersed
submanifold
which
is
cr
foliated
by
{W
5
(q):
q E
I'}.
Here
the
submanifold
W
5
(p)
is
called
the
stable
manifold
of
0
at
p.
Since
W
5
(p)
is
also
the
stable
manifold
of
the
diffeomorphism
~T
at
p,
it
is
independent
of
the
distance
function
d,
as
also
is
W
5
(r)
= Uq,r W
5
(q).
We
have
seen
that
when
a
point
p e X
is
periodic
and
X
has
a
hyperbolic
structure
with
respect
to
f,
its
stable
set
is
a
manifold.
We
want
to
know
whether
we
can
extend
this
notion
of
hyperbolicity
to
a
non-periodic
point
p
and
get
a
stable
manifold
theorem
for
such
p.
The
problem
is
that
TPfn
does
not
map
TPX
to
itself
for
n >
o,
and
thus
we
cannot
define
hyperbolicity
in
terms
of
eigenvalues
of
this
map.
Let
A
be
any
invariant
subset
of
a
Riemannian
manifold
X,
and
let
TAX
be
the
tangent
bundle
of
X
over
A.
We
say
that
A
has
a
hyperbolic
structure
with
respect
to
f
if
there
is
a
continuous
splitting
of
TAX
into
the
direct
sum
of
Tf-invariant
sub-bundles
E
5
and
Eu
such
that,
for
some
constants
K
and
a
and
for
all
v e E
5
,
w e
Eu
and
n
~
o,
ITfn(v)
I S
Kanlvl,
ITf-n(w)
I S
Kanlwl,
where
0 < a <
1.
That
is,
Tf
will
eventually
contract
on
E
5
and
expand
on
Eu.
A
250

hyperbolic
subset
of
X
(with
respect
to
f)
is
a
closed
invariant
subset
of
X
that
has
a
hyperbolic
structure.
For
a
general
manifold,
the
existence
of
a
hyperbolic
structure
on
the
manifold
implies
there
are
some
topological
restrictions
(or
requirements)
on
the
manifold.
For
instance,
the
frequently
used
hyperbolic
structure
on
a
manifold
is
the
Lorentz
structure,
which
requires
the
existence
of
a
nowhere
zero
vector
field
on
the
manifold.
For
more
complex
hyperbolic
structures
(locally,
indefinite
metric),
the
topological
requirements
are
given
by
a
series
of
theorems
in
Steenrod
[1951].
We
shall
not
consider
these
here,
only
that
such
a
hyperbolic
structure
exists.
Before
we
state
the
generalized
stable
manifold
theorem,
let
us
make
several
definitions
so
that
the
theorem
can
be
stated.
Let
B(x,a)
be
an
open
ball
in
X
with
center
x
and
radius
a,
with
respect
to
the
Riemannian
distance
function
d,
and
let
D(x,b)
be
the
set
{y
£X:
d(f"(x),f"(y))
< b
for
all
n
~
0}.
Forb~
a~
0
we
define
the
stable
set
of
size
Cb.al
off
at
x
to
be
B(x,a)nD(x,b).
A map
of
an
open
subset
of
the
total
space
of
a
vector
bundle
into
a
manifold
is
denoted
by
Fr
(r
times
continuously
fiber
differentiable)
if,
with
respect
to
admissible
atlases,
all
partial
derivatives
in
the
fib;r
direction
up
to
order
r
exist
and
are
continuous
as
functions
on
the
total
space.
Theorem
6.2.8
(Generalized
stable
manifold
theorem)
Let
f
be
a
cr
diffeomorphism
of
X,
and
let
A
be
a
compact
hyperbolic
subset
of
X,
with
associated
decomposition
TAX
E
5
e
Eu.
Then
there
exists
an
open
neighborhood
W
of
the
zero
section
in
E
5
and
an
Fr
map
h:
W
~
X
such
that
for
some
b
~
a
~
0
and
for
all
x £ A, g
restricted
to
the
fiber
Wx
over
xis
a
cr
imbedding
with
image
W
5
1
~(x),
the
stable
set
of
size
(b,a)
at
x.
The
tangent
space
to
W
8
1
~(x)
at
x
is
(Es>x•
The
above
theorem
is
a
corollary
of
an
even
more
general
theorem
formulated
by
Smale
[1967],
proved
by
Hirsch
and
Pugh
[1970],
with
addendum
by
Hirsch,
Pugh
and
Shub
[1970].
251
&.3
Low
dimensional
stable
systems
For
the
simplest
example
of
a
low
dimensional
stable
system,
consider
a
vector
field
v
on
s
1
with
no
zeros.
Then
there
is
precisely
one
orbit
and
it
is
periodic.
In
fact,
we
may
identify
T(S
1
)
= s
1
x
R.
Let
v:
s
1
~
R
be
the
principal
part
of
v.
Since
v
is
continuous
and
s
1
is
compact,
lv(x)
I
is
bounded
below
by
some
constant
a >
0.
Then
any
perturbation
of
v
less
than
a
does
not
introduce
any
zero.
Thus
the
perturbed
vector
field
still
has
only
one
orbit,
and
is
topologically
equivalent
to
v.
so
v
is
structurally
stable
(in
fact,
C
0
-structurally
stable).
Now
suppose
that
v
has
finitely
many
zeros,
all
of
which
are
hyperbolic.
We
shall
call
such
a
vector
field
on
s
1
a
Morse-Smale
vector
field
on
s
1
•
(We
shall
give
a
definition
of
a
Morse-Smale
vector
field
on
a
higher
dimensional
space
later.
Here
we
merely
want
to
demonstrate
the
idea
for
the
simplest
case
first).
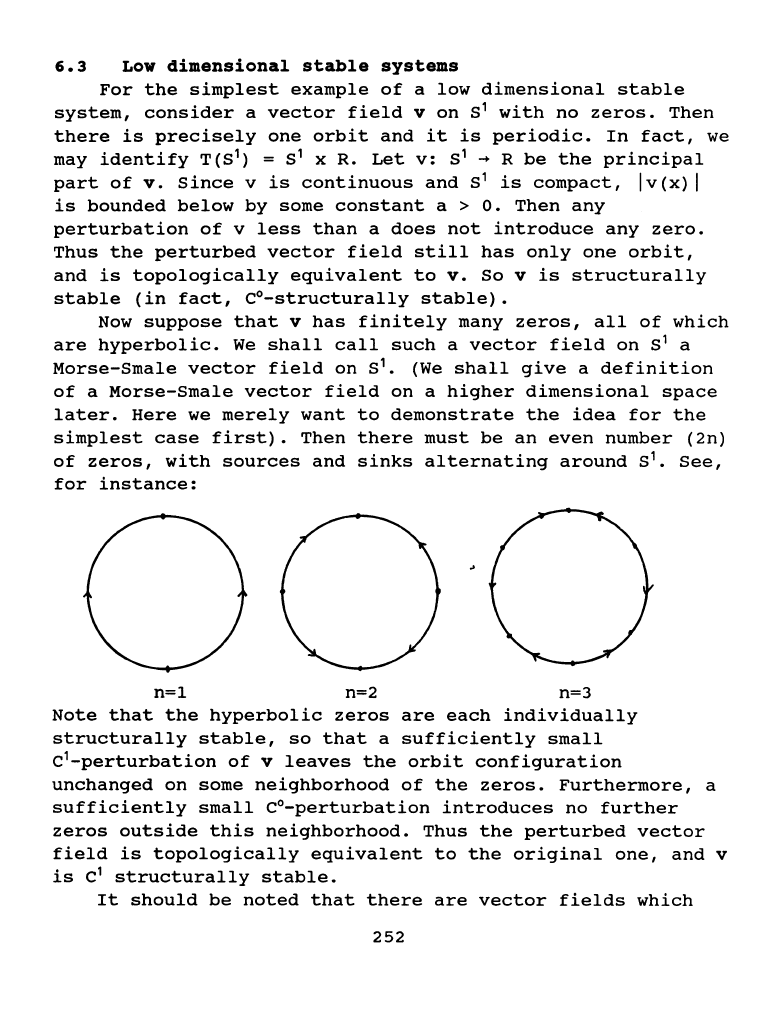
Then
there
must
be
an
even
number
(2n)
of
zeros,
with
sources
and
sinks
alternating
around
s
1
•
See,
for
instance:
n=l
n=2 n=3
Note
that
the
hyperbolic
zeros
are
each
individually
structurally
stable,
so
that
a
sufficiently
small
C
1
-perturbation
of
v
leaves
the
orbit
configuration
unchanged
on
some
neighborhood
of
the
zeros.
Furthermore,
a
sufficiently
small
C
0
-perturbation
introduces
no
further
zeros
outside
this
neighborhood.
Thus
the
perturbed
vector
field
is
topologically
equivalent
to
the
original
one,
and
v
is
c
1
structurally
stable.
It
should
be
noted
that
there
are
vector
fields
which
252

are
not
Morse-smale
but
nonetheless
have
the
above
orbit
structures.
One
can
show,
through
the
construction
of
examples,
that
structurally
stable
vector
fields
on
s
1
with
finitely
many
zeros
are
Morse-
Smale.
In
fact
the
following
stronger
result
is
true.
Theorem
6.3.1
A
vector
field
on
s
1
is
c
1
structurally
stable
iff
it
is
Morse-Smale.
Moreover,
Morse-Smale
vector
fields
form
an
open,
dense
subset
of
rrs
1
(1
s r s
~).
In
attempting
to
prove
that
certain
properties
are
open
and
dense
(i.e.,
it
holds
for
systems
in
an
open,
dense
subset
of
Diff(M)
or
rr(M))
or
generic,
one
uses
transversality
theory
(Chapter
2).
Recall
that
this
theory
investigates
the
way
submanifolds
of
a
manifold
cross
each
other,
and
how
a
map
of
one
manifold
to
another
throws
the
first
across
a
submanifold
of
the
second.
Sard's
theorem
(Theorem
2.4.14)
usually
provides
the
opening
shots.
Also
note
that
if
a
property
is
dense,
then
clearly
a
stable
system
is
equivalent
to
a
system
has
the
property.
For
instance,
the
property
of
having
only
hyperbolic
zeros
is
open
and
dense
for
flows
on
a
compact
manifold
of
any
dimension,
and any
structurally
stable
flow
possesses
this
property.
The
proof
is
essentially
the
one
for
s
1
•
Similarly,
Theorem
6.3.2
[Kupka
1963,
Smale
1963]
For
diffeomorphisms
of
a
compact
manifold
M
the
following
properties
are
generic,
and
satisfied
by
structurally
stable
diffeomorphisms:
(i)
all
periodic
points
are
hyperbolic,
(ii)
for
any
two
periodic
points
x
and
y,
the
stable
manifold
of
x
and
the
unstable
manifold
of
y
intersect
transversally.
(That
is,
the
tangent
spaces
of
the
two
submanifolds
at
any
point
of
intersection
p
generate
(span)
the
whole
tangent
space
of
the
manifold
M
at
p.
In
other
words,
(W
9
(x)
)P + (Wu(Y) )P =
MP
and
it
is
denoted
by
W
9
(x)
~
Wu(Y).
We
say
that
a
dynamical
system
is
Morse-Smale
if
(i)
its
non-
wandering
set
is
the
union
of
a
finite
set
of
fixed
points
and
periodic
orbits,
all
of
which
are
hyperbolic,
and
253
