Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.

d(Id9jdt)/dt
=
-k(~-
~
0
),
d(I'd~/dt)/dt-
((d9/dt)
2
/2l
cai;a~>
=
au;a~,
where
I=
c +
2ml•sin•~,
I'=
2m1•,
U =
2mlcos~
and
c,
1,
m,
k
and
g
are
positive
constants.
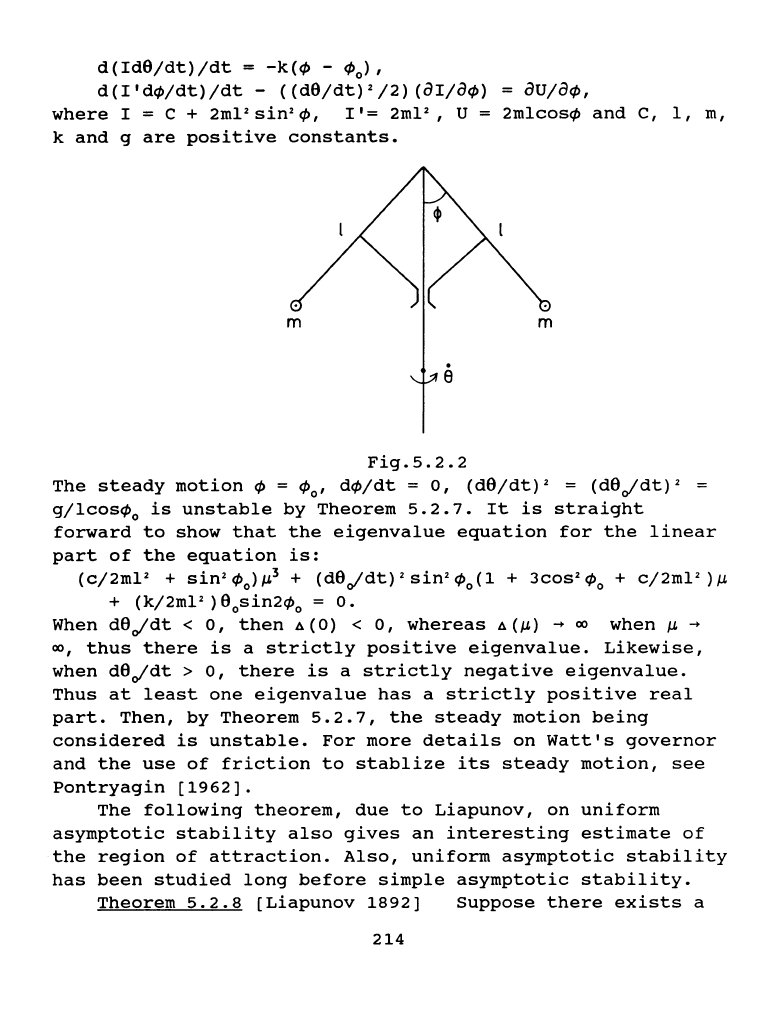
Fig.5.2.2
The
steady
motion~=
~
0
,
d~/dt
= O,
(d9/dt)
2
=
(d9cfdt)
2
=
gjlcos~
0
is
unstable
by
Theorem
5.2.7.
It
is
straight
forward
to
show
that
the
eigenvalue
equation
for
the
linear
part
of
the
equation
is:
(c/2ml•
+
sin
2
~
0
)~
3
+
(d9cfdt)•sin
2
~
0
(1
+
3cos
2
~
0
+
cj2ml•)~
+
(k/2ml
2
)9
0
sin2~
0
=
0.
When
d9cfdt
< o,
then
A(O)
< o,
whereas
A(~)
~
oo
when~~
oo,
thus
there
is
a
strictly
positive
eigenvalue.
Likewise,
when
d9cfdt
>
o,
there
is
a
strictly
negative
eigenvalue.
Thus
at
least
one
eigenvalue
has
a
strictly
positive
real
part.
Then,
by
Theorem
5.2.7,
the
steady
motion
being
considered
is
unstable.
For
more
details
on
Watt's
governor
and
the
use
of
friction
to
stablize
its
steady
motion,
see
Pontryagin
[1962].
The
following
theorem,
due
to
Liapunov,
on
uniform
asymptotic
stability
also
gives
an
interesting
estimate
of
the
region
of
attraction.
Also,
uniform
asymptotic
stability
has
been
studied
long
before
simple
asymptotic
stability.
Theorem
5.2.8
[Liapunov
1892]
Suppose
there
exists
a
214

c
1
function
V:
IxD
~
R
such
that
for
some
functions
a,
b,
c
€ K
and
every
(t,
x)
€
IxD:
(
i)
a ( llxll)
:5
V(t,
x)
:5
b (
llxll);
(ii)
dV(t,x)jdt
:5
-
c(llxlll.
Choosing
a > 0
such
that
Ba
c
D,
let
us
set
for
every
t E
I,
V"\x
=
{x
€ D:
V(t,x)::;
a(a)}.
Then
(a)
for
any
t
0
E I
and
any'x
0
E v-\ ,a:
x(t;t
0
,X
0
)
-+
0
uniformly
in
t
0
,
X
0
when
t
~
oo;
(b)
the
origin
is
uniformly
asymptotically
stable.
Note
that,
assumptions
(i)
and
(ii)
are
equivalent
to
(i)
and
(ii')
dV(t,x)jdt
::;·-
c'(V(t,x))
for
some
c'e
K.
Also,
the
existence
of
a c
1
function
V(t,x)
such
that:
(i)
V(t,x)
~
a<llxlll;
V(t,O)
= O;
(ii)
dV(t,x)/dt
:5
-c<llxll),
for
some
a,
c E K
and
every
(t,x)
E
IxD
does
not
imply
uniform
asymptotic
stability,
nor
even
asymptotic
stability!
This
is
demonstrated
by
Massera
[1949]
by
a
counter-example.
Corollary
5.2.9
The
origin
is
uniformly
globally
asymptotically
stable
if
the
assumptions
of
Theorem
5.2.8
are
satisfied
for
D =
R"
and
a(r)
~
oo
as
r
-+
oo.
To
illustrate
Theorem
5.2.8,
let
us
consider
the
scalar
equation,
which
represents
an
RLC
circuit
with
parametric
excitation,
(time
varying
capacitance),
d
2
xjdt
2
+ a
dxjdt
+
b(t)x
= o
(5.2-9)
where
a>
O, b = b
0
(1
+
Ef(t))
with
b
0
~
O,
and
f(t)
is
a
bounded
function
from
R
....
R.
Or
one
can
view
this
equation
representing
a
mechanical
oscillator
with
viscous
friction
and
a
time-varying
spring
"constant".
Eq.
(5.2-9)
is
equivalent
to
dx/dt
=
y,
dyjdt
=
-ay
-
b(t)x.
The
auxiliary
function
V(x,y)
=
(y
+
ax/2)
2
/2
+
(a
2
/4
+
b
0
)X
2
/2,
is
positive
definite.
Then
according
to
Theorem
5.2.8,
the
origin
will
be
uniformly
asymptotically
stable
if
the
time
derivative
of
V,
dV(t,x)jdt
=-
ay
2
/2-
(b-
b
0
)xy
-
abx
2
/2
is
negative
definite.
Following
Sylvester's
criterion,
this
is
so
if
for
some
a,
E
2
b
0
f(t)
2
- a
2
(1
+
Ef(t))
::;
-a<
0.
(5-2-10)
This
condition
is
satisfied
for
any
sufficiently
small
E.
One
can
view
the
differential
equation
as
representing
two
opposing
forces
at
work:
a
parametric
excitation
215

proportional
to
E,
and
a
load
which
is
the
damping
force
adxjdt.
Satisfying
Eq.(5.2-10)
tantamount
to
choosing
the
resistance,
a,
large
enough
for
the
load
to
absorb
all
the
energy
provided
by
the
excitation.
In
this
case,
the
origin
is
asymptotically
stable.
Otherwise,
if
the
load
is
not
large
enough,
one
may
expect
that
the
balance
of
energy
of
the
system
increases
and
the
origin
becomes
unstable.
This
is,
of
course,
a
heuristic
view.
Another
example
is
the
damped
pendulum,
which
we
discussed
at
the
very
beginning
in
Chapter
1.
We
have
shown
that
the
origin
is
asymptotically
stable
by
choosing
the
total
energy
as
the
auxiliary
function.
Nonetheless,
this
is
not
a
good
choice
because
the
time
derivative
of
E
is
not
negative
definite.
It
proves
stability
but
not
asymptotic
stability.
Therefore,
a
"natural"
choice
may
not
always
fit
Theorem
5.2.8.
Finding
a
suitable
auxiliary
function
is
often
a
matter
of
intuition,
experience
or
an
"art".
As
we
have
noticed
that
it
is
often
difficult
to
exhibit
an
auxiliary
function
whose
time
derivative
is
negative
definite,
so
an
alternative
way
of
proving
asymptotic
stability
will
be
to
work
out
some
more
elaborate
theorems
and
propositions
to
allow
one
to
use
auxiliary
functions
whose
time
derivative
is
non-
positive
(i.e.,
~
0),
but
of
course
along
with
some
more
information.
Theorem
5.2.8
can
be
used
to
prove
asymptotic
stability
by
considering
the
linear
approximation
as
illustrated
in
the
RLC-
circuit
with
parametric
excitation.
Now
suppose
dxjdt
=
f(t,x)
is
of
the
form
dxjdt
= Ax +
g(t,x)
as
specified
before
Theorem
5.2.7,
then
we
have
the
corresponding
theorem.
Theorem
5.2.10
[Liapunov
1892]
If
all
eigenvaleues
of
A
have
strictly
negative
real
parts
and
if
~g(t,x)~/~x~
~
0
as
x
~
0,
uniformly
for
all
t f
I,
then
the
origin
is
uniformly
asymptotically
stable.
As
an
example,
let
us
go
back
to
the
problem
of
the
glider
considering
non-vanishing
drag,
i.e.,
described
by
Eqs.(5.2-7).
These
equations
admit
a
critical
point,
216

Yo
=
(1
+
az)
-1/4
,
ao
= -
tan·1a,
which
corresponding
to
a
rectilinear
downward
motion
at
constant
velocity.
Without
loss
of
generality,
we
assume
that
0 > ao > -
'lr/2.
Change
the
variables
y =
Yo
+ y
1
, a =
ao
+ a
1
, we
transfer
the
origin
to
the
critical
point.
By
computing
the
terms
of
first
order
in
y,,
a,,
we
obtain
for
the
linear
variational
equation,
dy
1
jdt
=
-(2a)y
1
j(1+a
2
)
1
'
4
-
a,/(1+a
2
)112,
da,;dt
= 2y, -
aa,;
(
1+a
2
)
1
'
4
•
one
can
verify
that
the
eigenvalues
of
this
set
of
equations
have
strictly
negative
real
part.
Therefore,
according
to
Theorem
5.2.10,
the
critical
point
is
asymptotically
stable.
The
following
couple
of
theorems
are
variations
of
Theorem
5.2.8.
Theorem
5.2.11
[Massera
1949)
If
we
replace
(ii)
in
Theorem
5.2.8
by
(ii'),
there
exist
a
function
U:
IxD
~
R
and
a
function
c E K
such
that:
U(t,x)
?::
c(llxli),
U(t,O)
=
0,
and
for
any
a
1
, a
2
with
0 < a
1
< a
2
,
dV(t,x)jdt
+
U(t,x)
~
0
as
t
~
oo
,
uniformly
on
a
1
:5
llxll
:5
a
2
,
then
the
origin
is
equi-asymptotically
stable.
Theorem
5.2.12
[Antosiewicz
1958)
If
the
origin
is
uniformly
stable,
and
if
there
exists
a c
1
function
V:
IxD
~
R
such
that
for
some
functions
a,
c e K
and
every
(t,x)
e
IxD:
(i)
V(t,x)
?::
a(lixll);
V(t,O)
=
O;
(ii)
dV(t,x)jdt
:5
-
c(llxll>;
then
the
origin
is
equi-asymptotically
stable.
Next,
we
shall
introduce
a
theorem
which
makes
use
of
two
auxiliary
functions.
Theorem
5.2.13
[Salvadori
1972]
Suppose
there
exist
two
c
1
functions
V:
IxD
~
R
and
w:
IxD
~
R
such
that
for
some
functions
a,
b,
c e K
and
every
(t,x)
e
IxD:
(i)
V(t,x)
?::
a(llxll);
V(t,O)
O;
(ii)
W(t,x)
?::
b(lixli)
i
W(t,O)
=
0;
(iii)
dV(y,x)jdt
:5
-c(W(t,x));
(iv)
W(t,x)
is
bounded
from
below
or
from
above.
Choosing
a
> 0
such
that
a
..
c
D,
for
any
t e
I,
we
put
v-\_
..
=
{x
e
D:
V(t,x)
:5
a(a)}.
Then
(a)
the
region
of
attraction
A(t
0
)
~
v-\_
..
;
(b)
the
origin
is
asymptotically
stable.
217

Note
that,
the
function
W
in
the
above
theorem
can
be
of
special
form.
For
instance,
if
we
identify
W(t,x)
with
(xlx),
or
W(t,x)
=
V(t,x),
we
obtain
Corollary
5.2.14
or
5.2.15
respectively.
In
fact,
these
two
corollaries
were
known
long
before
Theorem
5.2.13
was
established.
Corollary
5.2.14
(Marachkov
1940]
Suppose
there
exists
a c
1
function
v: IxD
~
R
such
that
for
some
functions
a,
c E
K,
and
every
(t,x)
E
IxD:
(i)
V(t,x)
~
a<llxll):
V(t,O)
=
O;
(ii)
dV(t,x)/dt
S-
c(xlx).
If,
moreover,
f(t,x)
is
bounded
on
IxD,
then
(a)
for
every
a > 0
such
that
B
4
c
D,
the
region
of
attraction
A(t
0
)
:;:)
v·\
4
;
(b)
the
origin
is
asymptotically
stable.
Corollary
5.2.15
(Massera
1956)
Suppose
there
exists
a
c
1
function
V:
IxD
~
R
such
that
for
some
functions
a,
c E
K,
and
every
(t,x)
E
IxD:
(i)
V(t,x)
~
a(llxll):
V(t,O)
=
O;
(ii)
dV(t,x)/dt
S-
c(V(t,x));
then
(a)
for
every
a>
0
such
that
B
4
c
D,
the
region
of
attraction
A(t
0
)
:;:)
v·\
,«;
(b)
the
origin
is
asymptotically
stable.
As
an
example
for
the
application
of
Theorem
5.2.13,
let
us
generalize
the
damped
pendulum
by
considering
the
pendulum
with
a
variable
friction
h(t),
thus
the
equation
of
motion
becomes:
d'8/dt'
+
h(t)d8/dt
+
sin8
= o,
where
h
is
a
c
1
function
from
I
~
R,
and
we
set
the
gravitational
constant
g = 1
just
for
simplicity.
We
are
looking
for
some
hypotheses
concerning
h(t),
as
mild
as
possible,
and
the
system
will
entail
asymptotic
stability
of
the
origin
of
the
phase
plane.
Let
us
try
the
auxiliary
function
V(t,8,d8/dt)
=
(d8/dt
+
asin8)'/2
+
b(t)
(1-
cos8),
which
is
the
sum
of
a
quadratic
function.
If
we
put
b(t)
= 1 +
ah(t)
-
a',
then
dV(t,8,d8/dt)/dt
=-
(h(t)
-
acos8)
(d8/dt)'
-a
sin'8
+
ah'(t)(l-
cos8)
- a•
(1-
cos8)sin8
d8/dt
=-
(h(t)
-a)
(d8jdt)'
-
a(2-
h'(t))
(1-
cos8)
+ 0
3
,
where
0
3
contains
terms
of
at
least
third
order
in
8
and
d8/dt,
all
independent
of
t.
Now,
if
there
exist
two
constants
a >
a,
and
p < 2
such
that
(i)
h(t)
~
a > a >
O;
(ii)
h'(t)
S P <
2:
then
V
and-
dV/dt
are
positive
definite.
Furthermore,
let
us
define
W(8,d8/dt)
=
(d8/dt)'/2
218

+
(1-
cosO)
and
dW(t,O,dOjdt)jdt
=-
h(t)(dOjdt)'
S 0
is
bounded
from
above.
Then
from
Theorem
5.2.13,
the
origin
is
asymptotically
stable.
Note
that,
because
of
the
requirement
of
f(t,x)
be
bounded,
therefore
a
bound
should
be
assumed
for
h(t).
Thus,
corollary
5.2.14
could
not
be
used.
Corollary
5.2.15
could
not
be
used
either,
at
least
not
the
V(t,O,dO/dt)
chosen
above,
because
satisfying
(ii)
of
Corollary
5.2.15
would
also
require
further
hypotheses
on
h(t).
One
can
also
show
equi-asymptotical
stability
of
the
origin
by
using
an
"variation"
of
Theorem
5.2.13.
Theorem
5.2.16
[Salvadori
1972]
Suppose
there
exist
two
c
1
functions
V:
IxD
~
R,
and
W:
IxD
~
R
such
that
for
some
a
e
R,
some
function
b,
c e K,
and
every
(t,x)
e
IxD:
(i)
V(t,x)
~
a;
(ii)
W(t,x)
~
bCIIxll>;
W(t,O)
= O;
(iii)
dV(t,x)jdt
S-
c(W(t,x));
(iv)
dW(t,x)jdt
S O;
then
the
origin
is
equi-asymptotically
stable.
Return
to
partial
stability
by
getting
back
to
Eq.(5.2-2)
along
with
the
accompanying
assumptions
as
presented
there.
We
suppose
further
that
all
solutions
of
Eq.(5.2-2)
exist
on
[t
0
,~).
The
solution
z = 0
of
Eq.(5.2-2)
is
said
to
be
uniformly
asymptotically
stable
with
respect
to
x,
if
it
is
uniformly
stable
with
respect
to
x,
and
if
for
given
e >
o,
t
0
e
I,
there
exist
a > 0
and
~
> 0
such
that
llx(t,t
0
,Z
0
)
II
< e
for
all
llz
0
ll
<
~.
and
all
t
~
t
0
+
a.
Theorem
5.2.17
Suppose
there
exists
a c
1
function
V:
IxDxRm
~
R
such
that
for
some
functions
a,
b,
c e K
and
every
(t,z)
e IxDxRm:
(i)
a(llxll>
S
V(t,z)
S
b(llxll);
(ii)
dV(t,z)/dt
S -
c(llxll).
Then
(a)
for
any
a > 0
and
any
(t
0
,
z
0
)
e
Ix(B,.
n
D)
xRm,
x(t;t
0
,
Z
0
)
~
0
uniformly
in
t
0
,
Z
0
when
t
~
~;
(b)
the
origin
is
uniformly
asymptotically
stable
with
repsect
to
x.
Theorem
5.2.18
Assume
u e
R~k,
0 S k S m,
as
a
vector
219

containing
all
components
of
x
and
k
components
of
y.
Suppose
there
exists
a c
1
function
V:
IxDxRm
~
R
such
that
for
some
functions
a,
b,
c e K
and
every
(t,z)
e
IxDxRm:
(i)
a(llxll)
!'>
V(t,z)
!'>
bCIIxll>;
(ii)
dV(t,z)/dt
!'>
-
c(llxll);
then
the
origin
is
uniformly
asymptotically
stable
with
respect
to
x.
When
the
whole
space
is
the
region
of
asymptotic
stability,
we
say
that
we
have
complete
stability.
Then
we
have
the
following:
Theorem
5.2.19
For
an
autonomous
system,
let
there
exists
a c
1
function
V:
IxD
~
R
such
that,
V(x)
> o
for
all
x + 0
and
dV(x)jdt
!'>
o.
Let
E
be
the
locus
dV/dt
= 0
and
let
S
be
the
largest
invariant
set
contained
in
E.
Then
all
solutions
bounded
for
t > 0
tend
to
S
as
t
~
~.
In
addition,
if
we
know
that
V(x)
~
~
as
llxll
~
~,
then
each
solution
is
bounded
for
t
~
o,
and
one
can
conclude
that
all
solutions
approach
S
as
t
~
~.
Furthermore,
if
S
is
the
origin,
we
then
have
complete
stability.
Thus,
in
order
to
establish
complete
stability
we
need
to
show
that
every
solution
is
bounded
for
t
~
0
and
that
S
is
the
origin.
Suppose
that
V(x)
~
~
as
llxll
~
~
and
that
dV(x)jdt
< 0
for
x + o.
Then
S
is
certainly
the
origin,
and
it
is
easy
to
show
that
every
solution
is
bounded
for
t
~
o.
Let
x(t)
be
the
solution
through
x
0
,
then
for
some
sufficiently
large
r,
V(x)
>
V(x
0
)
for
all
llxll
~
r.
But
since
V(x(t))
decreases
with
t,
we
note
that
llx(t)
II
< r
for
all
t
~
0.
Hence
every
soulution
is
bounded.
Thus,
Theorem
5.2.20
Let
V
be
C
1
•
Suppose
(i)
V(x)
> 0
for
x
+
O;
(ii)
dV/dt
< 0
for
x +
O;
and
(iii)
V
~
~
as
llxll
~
~.
Then
the
autonomous
system
is
completely
stable.
In
many
applications,
it
occurs
that
one
can
construct
a
Liapunov
function
V
satisfying
Theorem
5.2.20.
Examples
will
be
given
in
Section
7.7.
The
question
arises
whether
it
is
true
that
stability,
asymptotic
stability,
etc.,
imply
the
existence
of
Liapunov
functions
such
as
described
in
various
theorems
in
this
220

section.
We
shall
answer
this
in
the
next
section.
It
should
be
pointed
out
that
even
though
this
is
a
good
mathematical
problem,
it
is
not
of
great
practical
importance
as
we
shall
see
in
the
next
section.
5.3
Converse
theorems
So
far,
in
most
theorems
given,
the
existance
of
a
Liapunov-
like
function
is
assumed.
The
question
arises
naturally
whether
such
a
function
actually
exists,
i.e.,
given
some
stability
or
attractivity
properties
of
the
origin,
can
one
build
up
an
auxiliary
function
suitable
for
the
corresponding
theorem?
It
is
answered
in
the
so-called
converse
theorems.
Before
we
get
to
the
converse
theorems,
there
are
several
comments
are
in
order.
First
of
all,
most
converse
theorems
are
proved
by
actually
constructing
the
suitable
auxiliary
function.
Such
construction
almost
always
assumes
the
knowledge
of
the
solutions
of
the
differential
equation.
This
is
why
converse
theorems
give
no
clue
to
the
practical
search
for
Liapunov's
functions.
Second,
when
the
existence
of
such
and
such
function
is
necessary
and
sufficient
for
certain
stability
property,
then
any
other
sufficient
condition
will
imply
the
originial
one.
Nonetheless,
this
observation
should
not
prevent
anyone
from
looking
for
other
sufficient
conditions
which
might
be
more
practical,
easier
to
apply.
Third,
sometimes
a
stability
property
of
a
system
can
be
studied
by
considering
first
a
simplified
system.
Let
us
suppose
that
the
stability
of
a
simplified
system
can
be
established
easily.
Then
we
can
deduce
from
a
converse
theorem
the
existence
of
a
suitable
auxiliary
function.
Then
under
appropriate
conditions,
it
might
also
be
a
good
auxiliary
function
for
the
original
system
and
prove
the
stability
property
of
the
original
system.
Hence
we
shall
give
the
converses
of
three
important
theorem
given
earlier.
The
general
setting
is
the
same
as
before,
i.e.,
considering
a
continuous
function
f:
IxD
~
R",
221

I=
(r,oo),
D c
Rn
containing
the
origin,
and
the
differential
equation
dxjdt
=
f(t,x),
where
the
origin
is
an
equilibrium
or
critical
point.
Theorem
5.3.1
(converse
of
Theorem
5.2.1)
[Persidski
1933]
If
the
function
f
is
c
1
and
if
the
origin
is
stable,
then
there
exist
a
neighborhood
U c D
of
the
origin
and
a c
1
function
V:
IxU
~
R
such
that
for
some
a E K
and
every
(t,x)
€
IxU:
(i)
V(t,x)
~
a(iixll>;
V(t,O)
= O;
(ii)
dV(t,x)jdt
:$
0.
Theorem
5.3.2
(converse
of
Theorem
5.2.2)
[Kurzweil
1955)
If
the
function
f
is
c
1
and
if
the
origin
is
uniformly
stable,
then
there
exist
a
neighborhood
U c D
of
the
origin
and
a c
1
function
V:
IxU
~
R
such
that
for
some
a,
b f K,
and
every
(t,x)
€
IxU:
(i)
b(llxll)
~
V(t,x)
~
a(llxll);
V(t,O)
=
o;
(ii)
V(t,x)
:$
0.
Theorem
5.3.3
(converse
of
Theorem
5.2.8)
[Massera
1949,
1956)
If
the
function
f
on
IxD
is
locally
Lipschitzian
in
x
uniformly
with
respect
to
t,
and
if
the
origin
is
uniformly
asymptotically
stable,
then
there
exist
a
neighborhood
U c D
of
the
origin
and
a
function
V:
IxU
~
R
possessing
partial
derivatives
in
t
and
x
of
arbitrary
order,
such
that
for
some
functions
a,
b,
c € K
and
every
(t,x)
€
IxU:
(i)
a(llxll)
:$
V(t,x)
:$
b(llxll);
(ii)
dV(t,x)jdt
:;;
-
c(llxll).
Corollary
5.3.4
If
f
on
IxD
is
Lipschitzian
in
x
uniformly
with
respect
to
x,
V
can
be
chosen
such
that
all
partial
derivatives
of
any
order
of
V
are
bounded
on
IxU,
with
the
same
bound
for
all
of
them.
If
on
IxD
f
is
independent
of
t,
or
periodic
in
t,
V
can
be
chosen
independent
of
t
or
periodic
in
t
respectively.
5.4
comparison
methods
In
calculus
or
even
in
algebra,
we
have
learned
that
there
are
many
methods
of
testing
the
convergence
of
a
series.
Among
them
is
the
comparison
test.
Similarly,
in
determining
the
stability
of
a
equation
at
a
point,
one
can
222

find
the
relationship
between
the
said
equation
and
a
given
equation
with
stability
properties
known
at
the
origin.
More
explicitly,
consider
the
equation
dxjdt
=
f(t,x),
(5.4-1)
and
suppose
that
there
exists
a
scalar
differential
equation
dujdt
=
g(t,u)
(5.4-2)
with
a
critical
point
at
the
origin
u = 0
and
some
known
stability
properties.
The
comparison
method
studies
the
relationship
which
should
exist
between
Eqs.(5.4-1)
and
(5.4-2)
in
order
that
the
stability
properties
of
Eq.(5.4-2)
entail
the
corresponding
properties
for
Eq.(5.4-1).
In
order
to
avoid
needless
intricacies
in
computation,
we
shall
define
g(t,u)
of
Eq.(5.4-2)
on
IxR+,
where
R+
=
[0,~),
in
this
section.
Of
course,
the
uniqueness
of
the
solutions
of
the
comparison
equation
is
assumed
here
for
convenience.
Furthermore,
when
it
is
supposed
that
Eq.(5.4-2)
admits
of
u = 0
as
a
critical
point
and
that
point
is
stable,
or
asymptotically
stable,
only
positive
perturbations
will
have
to
be
considered.
Lemma
5.4.1
(Wazewski
1950]
Let
g:
IxR+
~
R+,
(t,u)
~
g(t,u)
be
continuous
and
such
that
Eq.(5.4-2)
has
a
unique
solution
u(t;t
0
,U
0
)
through
any
(t
0
,U
0
)
e
IxR+.
Let
[t
0
,b)
be
the
maximal
future
interval
where
u(t;t
0
,u
0
)
is
defined.
Let
v:
[t,b)
~
R
be
(i)
v(t
0
)
S U
0
i
(ii)
dv(t)jdt
S
g(t,v(t))
on
[t
0
,b);
then
v(t)
S
u(t)
on
(t
0
,b).
Theorem
5.4.2
(Corduneanu
1960]
Suppose
that
there
exists
a
function
gas
in
Lemma
5.4.1,
with
g(t,o)
=
o,
and
a c
1
function
V:
rxo
~
R,
such
that
for
some
function
a e K
and
every
(t,x)
e
IxD:
(i)
V(t,x)
~
a<llxlll,
V(t,O)
=
O;
(ii)
dV(t,x)jdt
S
g(t,V(t,x));
then
(a)
stability
of
u = o
implies
stability
of
x = O;
(b)
asymptotic
stability
of
u = 0
implies
equi-asymptotic
stability
of
x =
o.
Furthermore,
if
for
some
function
b e K
and
every
(t,x)
e
IxD:
(iii)
V(t,x)
S
b(llxlll;
then
(c)
uniform
stability
of
u = 0
implies
uniform
stability
of
223
