Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.

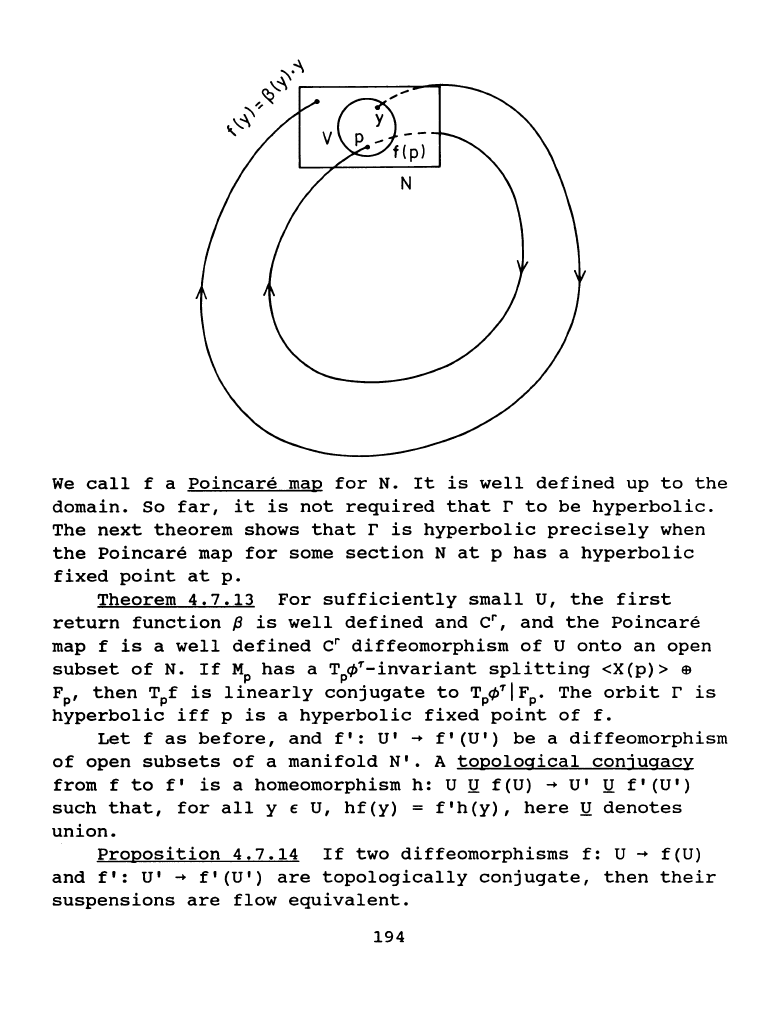
we
call
f a
Poincare
map
for
N.
It
is
well
defined
up
to
the
domain.
So
far,
it
is
not
required
that
r
to
be
hyperbolic.
The
next
theorem
shows
that
r
is
hyperbolic
precisely
when
the
Poincare
map
for
some
section
N
at
p
has
a
hyperbolic
fixed
point
at
p.
Theorem
4.7.13
For
sufficiently
small
u,
the
first
return
function
p
is
well
defined
and
c•,
and
the
Poincare
map
f
is
a
well
defined
c•
diffeomorphism
of
U
onto
an
open
subset
of
N.
If
MP
has
a
TP~~-invariant
splitting
<X(p)>
$
FP,
then
TPf
is
linearly
conjugate
to
TP~~IFP.
The
orbit
r
is
hyperbolic
iff
p
is
a
hyperbolic
fixed
point
of
f.
Let
f
as
before,
and
f':
U'
~
f'(U')
be
a
diffeomorphism
of
open
subsets
of
a
manifold
N'.
A
topological
conjugacy
from
f
to
f'
is
a
homeomorphism
h:
U M
f(U)
~
U'
M
f'(U')
such
that,
for
ally
E u,
hf(y)
=
f'h(y),
hereM
denotes
union.
Proposition
4.7.14
If
two
diffeomorphisms
f:
U
~
f(U)
and
f':
u•
~
f'(U')
are
topologically
conjugate,
then
their
suspensions
are
flow
equivalent.
194

The
above
result
simplifies
the
problem
of
classifying
suspension
(see
Sect.4.1).
The
main
connection
between
the
flow
near
a
closed
orbit
r
of
a
c•
flow
~
on
M
and
the
Poincare
map
at
a
cross-section
N
of
the
flow
at
p
of
r
is
the
following
theorem.
Theorem
4.7.15
Let
f:
U
~
f(U)
be
a
Poincare
map
at
p
for
the
cross-section
N.
Then
there
is
a
c•
orbit
preserving
diffeomorphism
h
from
some
neighborhood
of
the
orbit
R·p
of
the
suspension
E(f)
to
some
neighborhood
of
the
orbit
r
of
~
such
that
h(p)
=
p.
Since
if
r
is
hyperbolic,
then
any
Poincare
map
f
has
a
hyperbolic
fixed
point
at
p.
Then
by
Corollary
4.7.8
and
Proposition
4.7.14
we
have
Corollary
4.7.16
If
r
is
hyperbolic
then
the
flow~
is
topologically
equivalent
at
r
to
E(TPf)
at
its
unique
closed
orbit.
From
Corollary
4.7.9
we
have:
Corollary
4.7.17
There
are
precisely
4n
different
hyperbolic
closed
orbits
that
can
occur
in
a
flow
on
an
(n+l)-dim.
manifold
(n
~
1).
Hyperbolic
closed
orbits
are
structurally
stable,
in
the
sense
that,
if
r
is
such
an
orbit
of
a C
1
vector
field
X
on
M,
and
Y
is
a
vector
field
on
M
that
is
c
1
-closed
to
X,
then
for
some
neighborhood
U
of
r
in
M,
Y
has
a
unique
closed
orbit
in
u,
and
this
closed
orbit
is
topologically
equivalent
to
r.
We
shall
discuss
structural
stability
in
more
detail
in
Chapter
6.
Recall
that
in
Hartman's
theorem
we
altered
a
hyperbolic
linear
homeomorphism
T
by
a
perturbation
ry
and
found
a
topological
conjugacy
h =
id+g
from
T
to
T+ry.
It
was
pointed
out
that
h
is
not
necessarily
c
1
even
when
ry
is
~.
since
differentiating
the
conjugacy
relation
would
place
algebraic
restrictions
on
the
first
derivatives
of
T+ry.
The
question
arises
naturally
as
to
whether
further
differentiation
places
further
restrictions
on
higher
derivatives,
and
even
if
these
algebraic
restrictions
are
satisfied,
if
the
smoothness
of
ry
has
any
effect
on
h.
It
turns
out
that,
in
195

finite
dimensions,
further
restrictions
are
the
exception
rather
than
the
rule.
We
have
some
positive
results
on
the
smoothness
of
conjugacy
relations.
The
main
theorem
is
due
to
sternberg
[1957,
1958],
for
more
details,
see
for
instance,
Nelson
1969],
and
a
couple
of
relevant
theorems
by
Hartman.
Theorem
4.7.18
(Sternberg)
LetT
E
L(R")
have
eigenvalues
a
1
,
•••
,an
(can
be
complex
or
degenerated)
satisfying
ai
+ a,m,
..•.
anm"
for
all
1
!>
i
!>
n
and
for
all
non-negative
integers
m
1
,
•••
,
mn
with
!:i=l
mi
~
2.
Let
TJ:
U
....
R"
be
a C
8
map
(s
~
1)
defined
on
some
neighborhood
U
of
0
with
TJ(O)
=
DTJ(O)
= o.
Then
(T+TJ)
lo
is
cr
conjugate
to
Tlo,
where,
for
a
given
T,
r
depends
only
on
s
and
tends
to
oo
as
s
does.
Notice
that
the
eigenvalue
condition
implies
that
T E
HL(R").
Also,
if
TJ
is
CO,
the
maps
T
and
T+"
are
CG
conjugate
at
0.
Theorem
4.7.19
LetT
E L(R")
be
a
contraction
and
T]:
U
....
R"
be
a c
1
map
defined
on
some
neighborhood
u
of
o,
with
TJ(O)
=
DTJ(O)
= o.
Then
(T+TJ)
lo
is
c
1
conjugate
to
Tlo.
Theorem
4.7.20
(Hartman)
LetT
E
HL(R"),
where
n = 1
or
2,
and
let
TJ
be
the
same
as
above.
Then
(T+TJ)
lo
is
c
1
conjugate
to
Tlo.
Nitecki
[1979]
studied
the
dynamic
behavior
of
solutions
for
systems
of
the
form
dxjdt
=
G(F(x))
where
F
is
a
real-valued
function
on
R"
near
equilibria
and
periodic
orbits.
He
has
found
that
for
n
~
3,
the
behavior
near
periodic
orbits
is
arbitrary,
i.e.,
any
differomorphism
of
the
(n-1)-disk
isotopic
to
the
identity
arises
as
the
Poincare
map
near
a
periodic
orbit
for
some
choice
of
F
and
G. On
the
other
hand,
for
n
~
2
the
behavior
near
equilibria
is
severely
restricted.
Indeed,
(a)
if
dG/dF
+ 0
at
an
equilibrium
point,
the
flow
in
a
neighborhood
is
conjugate
to
that
of
a
constant
vector
field
multiplied
by
a
function;
(b)
if
an
equilibrium
point
is
isolated,
it
is
an
extremum
of
F,
and
if
F
satisfies
a
convexity
condition
near
the
equilibrium,
then
the
flow
in
a
neighborhood
resembles
that
196

described
in
(a),
except
that
the
stable
and
unstable
sets
may
be
cones
instead
of
single
orbits;
and
(c)
when n =
2,
a
stronger
condition
on
F,
together
with
a
weaker
condition
of
G,
again
yields
the
description
in
(a).
Finally,
let
us
end
this
chapter
by
giving
a
list
of
further
readings.
First
of
all,
the
classic
review
article
by
Smale
[1967]
is
the
one
on
the
"must-read"
list.
Irwin
[1980]
is
the
source
of
most
of
the
notes
in
this
chapter.
A
most
recent
introductory
book,
which
takes
the
reader
a
long
way,
by
Ruelle
[1989]
is
highly
recommended.
197

Chapter
5
Stability
Theory
and
Liapunov•s
Direct
Method
5.1
Introduction
Earlier
in
Chapter
1,
we
described
the
general
idea
of
stability
in
the
normal
sense.
Very
early
on,
the
stability
concept
was
specialized
in
mechanics
to
describe
some
type
of
equilibrium
of
a
particle
(or
a
celestial
object)
or
system
(such
as
our
solar
system).
For
instance,
consider
a
particle
subject
to
some
forces
and
posessing
an
equilibrium
point
~·
The
equilibrium
is
stable
if
after
any
sufficiently
small
perturbations
of
its
position
and
velocity,
the
particle
remains
arbitrarily
near
q
0
with
arbitrarily
small
velocity.
As
we
have
discussed
in
Chapter
1,
the
well
known
example
of
a
pendulum,
and
we
shall
not
dwell
on
it.
It
suffices
to
say
that
the
lowest
position
(equilibrium
point),
associated
with
zero
velocity,
is
a
stable
equilibrium,
whereas
the
hightest
one
also
with
zero
velocity
is
an
unstable
one.
We
have
also
briefly
mentioned
that
when
fomulated
in
precise
mathematical
terms,
this
"mechanical"
definition
of
stability
was
found
very
useful
in
many
situations,
yet
inadequate
in
many
others.
This
is
why a
host
of
other
concepts
have
been
introduced
and
each
of
them
relates
to
the
"mechanical"
definition
and
to
the
common
sense
meaning
of
stability.
Contrary
to
the
"mechanical"
stability,
Liapunov's
stability
has
the
following
features:
1".
it
does
not
pertain
to
a
material
particle
(or
the
equation),
but
to
a
general
differential
equation;
2".
it
applies
to
a
solution,
i.e.,
not
only
to
an
equilibrium
or
critical
point.
In
his
memoire,
Liapunov
[1892]
dealt
with
stability
by
two
distinct
methods.
His
'first
method'
presupposes
an
explicit
solution
known
and
is
only
applicable
to
some
restricted,
but
important
situations.
While
this
•second
method'
(also
called
'direct
method')
does
not
require
the
prior
knowledge
of
the
solutions
themselves.
Thus,
Liapunov's
direct
method
is
of
great
power
and
advantage.
An
198

elementary
introduction
to
Liapunov's
direct
method
can
be
found
in
La
Salle
and
Lefschetz
[1961].
Suppose
for
an
autonomous
system
dx/dt
=
f(x),
in
which
the
system
is
initially
in
an
equilibrium
state,
then
it
remains
in
that
state.
But
this
is
only
a
mathematical
statement,
yet
a
real
system
is
subject
to
perturbations
and
it
is
impossible
to
control
its
initial
state
exactly.
This
begs
the
question
of
stability,
that
is,
under
an
arbitrary
small
perturbation
will
the
system
remain
near
the
equilibrium
state
or
not?
In
the
following,
we
shall
make
these
concepts
precise,
and
we
will
discuss
these
questions
extensively.
Let
dx/dt
=
f(x,t)
where
x
and
f
are
real
n-vectors,
t e
R
("time"),
f
is
defined
on
RxR".
We
assume
f
is
smooth
enough
to
ensure
its
existence,
uniqueness,
and
continuous
dependence
of
the
solutions
of
the
initial
value
problem
associated
with
the
differential
equation
over
RxR".
For
simplicity,
we
assume
that
all
solutions
to
be
mentioned
later
exist
for
every
t E R.
Let
II·
II
denote
any
norm
on
R".
A
solution
x'(t)
of
dxjdt
=
f(x,t)
is
stable
at
t,
in
the
sense
of
Liapunov
if,
for
every
e >
0,
there
is
a 6 > 0
such
that
if
x(t)
is
any
other
solution
with
llx(t
0
)
-
x•
(t
0
)
II
<
6,
then
llx(t)
-
x•
(t)
II
< E
for
all
t
~
t
0
•
Otherwise,
x'(t)
is
unstable
at
t
0
•
Thus
the
stability
at
t
0
is
nothing
but
continuous
dependence
of
the
solution
on
x
0
=
x(t
0
),
uniform
with
respect
to
t E
[t
0
,~).
We
can
gain
some
geometric
insight
into
this
stability
concept
by
considering
the
pendulum.
As
before,
the
set
of
first
order
differential
equations
have
the
form:
d8/dt
=
n,
dn/dt
= -
gsin8.
The
origin
of
the
phase
space
(8,n)
represents
the
pendulum
hanging
vertically
downward
and
is
at
rest.
As we
have
shown
before
in
the
phase
portrait,
all
solutions
starting
near
the
origin
form
a
family
of
non-intersecting
closed
orbits
encircling
the
"origin".
Given
e >
0,
consider
an
orbit
entirely
contained
in
the
disk
a.
of
radius
e
centered
at
the
"origin".
Further
choose
any
other
disk
B
6
of
radius
6
contained
in
this
chosen
199

orbit.
Due
to
the
non-intersecting
nature
of
the
orbits,
every
solution
starting
in
B
6
at
any
initial
time
remains
in
B,.
This
demonstrates
stability
of
the
equilibrium
for
any
initial
time.
On
the
other
hand,.
any
other
solution
corresponding
to
one
of
the
closed
orbits
is
unstable.
This
is
because
the
period
of
the
solution
varies
with
the
orbit
and
two
points
of
(8,n)-plane,
very
close
to
each
other
at
t
=
t
0
,
but
belonging
to
different
orbits,
will
appear
in
opposition
after
some
time.
This
happens
no
matter
how
small
the
difference
of
periods
is.
Yet,
it
remains
that
the
orbits
are
close
to
each
other.
This
leads
to
the
concept
of
orbital
stability.
We
have
discussed
the
dissipative
system,
such
as
the
damped
pendulum,
whereby
the
stable
equilibrium
becomes
asymptotically
stable.
That
is,
if
all
neighboring
solutions
x(t)
of
x'(t)
tend
to
x'(t)
when t
~~.We
have
also
said
a
few
words
about
our
Solar
system
and
the
notion
of
Lagrange
stability.
In
the
following
we
shall
give
various
definitions
of
stability
and
attractivity
for
our
future
use.
We
shall
note
that
if
we
replace
x
by
a new
variable
z
=
x-
x'(t),
then
dxjdt
=
f(t,x)
becomes
dz/dt
=
g(t,z)
=
f(t,z+x'(t))
-
f(t,x'(t)),
where
g(t,O)
= 0
for
all
t e R.
The
origin
is
a
critical
point
of
the
transformed
equation
and
stability
of
the
solution
x(t)
of
the
original
equation
is
equivalent
to
stability
of
this
critical
point
for
the
transformed
equation.
Of
course,
such
a
transformation
is
not
always
possible,
nor
is
it
always
rewarding.
Nonetheless,
for
the
time
being,
we
shall
concentrate
on
stability
of
critical
points.
Let
us
consider
a
continuous
function
f:
I x D
~
R",
(t,x)
~
f(t,x)
where
I=
(r,~)
for
some r e R
or
r
=-
~,
and
D
is
a
domain
of
R",
containing
the
origin.
Assume
that
f(t,O)
= o
for
all
t e I
so
that
for
the
differential
equation
dxjdt
=
f(t,x),
the
origin
is
an
equilibrium
or
critical
point.
Furthermore,
assume
f
to
be
smooth
enough
so
that
through
every
(t
0
,X
0
)
e
IxD,
there
passes
one
and
only
one
solution
of
dxjdt
=
f(t,x).
We
represent
this
solution
200

by
x(t;t
0
,x
0
),
thus
displaying
its
dependence
on
initial
conditions.
By
definition,
x(t
0
,t
0
,X
0
)
= x
0
•
For
the
right
maximal
interval
where
x(·
;t
0
,X
0
)
is
defined,
we
write
J+(t
0
,x
0
)
or
simply
J+.
We
recall
that
Bu
=
{x
£ R":
llxll
<a}.
The
solution
x = 0
of
dxjdt
=
f(t,x)
is
stable
if
for
a
given
£ > 0
and
t
0
£
I,
there
is
a 6 > 0
such
that
for
all
X
0
£ B
6
and
t £
J+,
one
has
llx(t;t
0
,X
0
)
II
<
£.
The
solution
is
unstable
if
for
some £ > 0
and
t
0
£ I
and
each
6 > 0
there
is
an
X
0
£ B
6
and
a t £
J+
such
that
llx(t;t
0
,X
0
)
II
~
£.
The
solution
is
uniformly
stable
if
given
£ > O,
there
is
a 6 =
6(£)
such
that
llx(t;t
0
,X
0
)
II
< £
for
all
t
0
£
I,
all
llx
0
ll
< 6
and
all
t
~
t
0
•
Since
J+
c
[t
0
,oo),
thus
in
principle
any
solution
may
cease
to
exist
after
a
certain
finite
time.
Nonetheless,
if
B,
c
D,
the
solutions
mentioned
in
the
definitions
of
stability
and
uniform
stability
may
continue
up
to
oo.
The
solution
x = 0
of
dxjdt
=
f(t,x)
is
attractive
if
for
each
t
0
£I
there
is
an~=
~(t
0
),
and
for
each£>
0
and
each
II
x
0
ll
<
~,
there
is
a a = a
(t
0
,
£,
X
0
)
> 0
such
that
t+a
£
J+
and
llx(t;t
0
,X
0
)
II
< £
for
all
t
~
t
0
+a.
The
solution
is
egui-
attractive
if
for
each
t
0
£ I
there
is
an
~
=
~(t
0
)
and
for
each
£ > 0 a a =
a(t
0
,£)
such
that
t
0
+a
£
J+
and
llx(t;t
0
,x
0
)
II
< £
for
all
llx
0
ll
<
~
and
all
t
~
t
0
+a.
The
solution
is
uniformly
attractive
if
for
some
~
> 0
and
each
£ > 0
there
is
a a =
a(£)
> 0
such
that
t
0
+a
£
J+
and
llx(t;t
0
,x
0
)
II
< £
for
all
llxoll
<
~.
all
t
0
£ I
and
all
t
~
t
0
+a.
As
remarked
earlier,
if
B,
c
D,
the
solutions
mentioned
immediately
above
exist
over
[t
0
,oo).
Thus,
in
the
definition
of
attractivity,
all
solutions
starting
from
B.
approach
the
origin
as
t
~
oo.
For
equi-attractivity,
they tend
to
0
uniformly
with
respect
to
X
0
£
B~
whereas
for
uniform
attractivity
they tend
to
o
uniformly
with
respect
to
x
0
£
B~
and
t
0
£
I.
We
can
define
attraction
slightly
differently.
Let
the
phase
space
X
be
locally
compact,
and
M a
non-empty
compact
subset
of
X.
The
region
of
weak
attraction
of
M,
Aw(M)
=
{x
201

£
X:
n(x)
n M +
0},
the
region
of
attraction
of
M,
A(M)
=
{x
£ X:
n(x)
+ 0
and
n(x)
a
subset
of
M},
and
the
region
of
uniform
attraction
of
M,
Au(M)
=
{x
£
X:
J+(x)
+ 0
and
J+(x)
a
subset
of
M}.
Furthermore,
any
point
x
in
Aw(M),
A(M),
~(M)
is
said
to
be
weakly
attracted.
attracted.
or
uniformly
attracted
to
M
respectively.
Proposition
5.1.1
Given
M,
a
point
x
is:
(i)
weakly
attracted
to
M
iff
there
is
a
sequence
{tn}
in
R
with
tn
~~and
d(xtn,M)
~
0,
where
d(.,.)
is
the
metric
distance,
(ii)
attracted
to
M
iff
d(xt,M)
~
o
as
t
~
oo,
(iii)
uniformly
attracted
to
M
iff
for
every
neighborhood
v
of
M
there
is
a
neighborhood
U
of
x
and
a T > o
with
Ut
a
subset
of
V
for
t
~
T.
Theorem
5.1.2
For
any
given
M,
Au(M)
is
a
subset
of
A(M),
which
is
a
subset
of
Aw(M),
and
they
all
are
invariant.
A
given
set
M
is
said
to
be:
(i)
a
weak
attractor
if
~(M)
is
a
neighborhood
of
M,
(ii)
an
attractor
if
A(M)
is
a
neighborhood
of
M,
(iii)
a
uniform
attractor
if
Au(M)
is
a
neighborhood
of
M,
(iv)
stable
if
every
neighborhood
u
of
M
contains
a
positively
invariant
neighborhood
V
of
M,
(v)
asymptotically
stable
if
it
is
stable
and
is
an
attractor.
A
weak
attractor
will
be
called
a
global
weak
attractor
if
Aw(M)
= X.
Similarly
for
global
attractor,
global
uniform
attractor.
An
attractor
is
a
strange
attractor
if
it
contains
a
transversal
homoclinic
orbit.
The
basin
of
attraction
of
A(M)
is
the
set
of
initial
points
p £ M
such
that
~(p)
approaches
A(M)
as
t
~
oo,
where
~
is
the
flow.
It
is
important
to
point
out
at
this
point
that
attractivity
does
not
imply
stability!
For
instance,
an
autonomous
system
in
R
2
presented
in
Hahn
[1967].
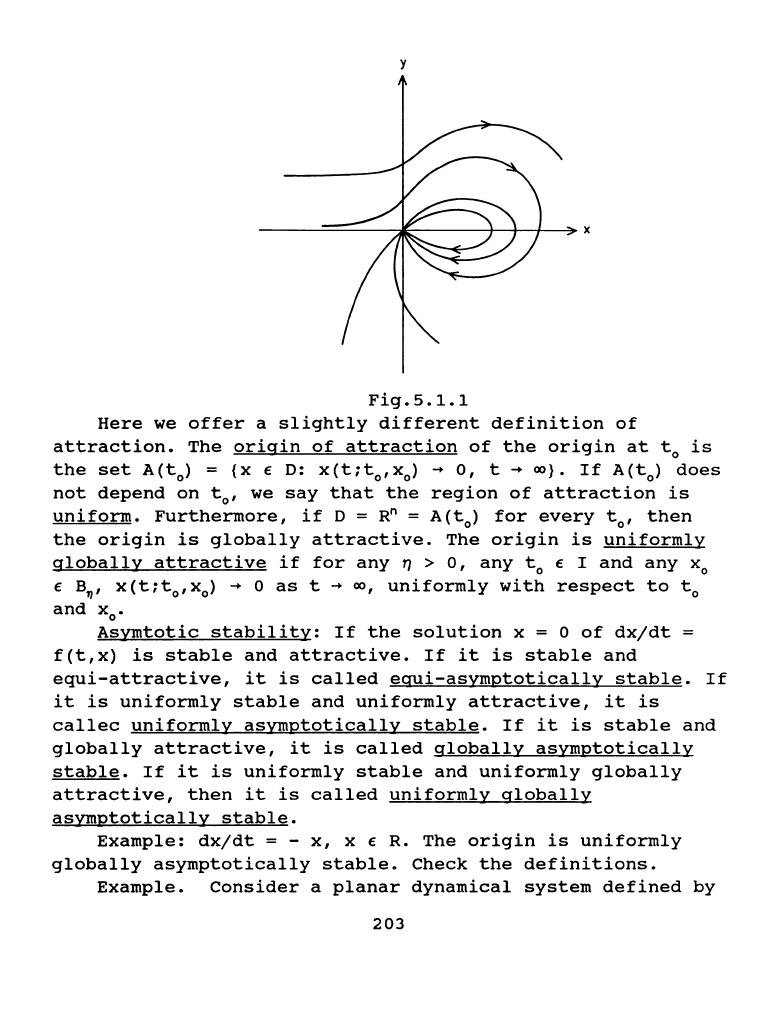
As
in
Fig.5.1.1,
where
r
is
a
curve
separating
bounded
and
unbounded
orbits.
The
origin
is
unstable,
in
spite
of
the
fact
that
every
solution
tends
to
it
as
t
~
oo.
202
y
Fig.S.l.l
Here
we
offer
a
slightly
different
definition
of
attraction.
The
origin
of
attraction
of
the
origin
at
t
0
is
the
set
A(t
0
)
=
{X
E
D:
x(t;t
0
,x
0
)
-+
0,
t
-+
oo}.
If
A(t
0
)
does
not
depend
on
t
0
,
we
say
that
the
region
of
attraction
is
uniform.
Furthermore,
if
D =
Rn
=
A(t
0
)
for
every
t
0
,
then
the
origin
is
globally
attractive.
The
origin
is
uniformly
globally
attractive
if
for
any
ry
>
O,
any
t
0
E I
and any
X
0
E
B~,
x(t:t
0
,x
0
)
-+
0
as
t -+ oo,
uniformly
with
respect
to
t
0
and
x
0
•
Asymtotic
stability:
If
the
solution
x = 0
of
dxjdt
f(t,x)
is
stable
and
attractive.
If
it
is
stable
and
equi-attractive,
it
is
called
egui-asymptotically
stable.
If
it
is
uniformly
stable
and
uniformly
attractive,
it
is
callec
uniformly
asymptotically
stable.
If
it
is
stable
and
globally
attractive,
it
is
called
globally
asymptotically
stable.
If
it
is
uniformly
stable
and
uniformly
globally
attractive,
then
it
is
called
uniformly
globally
asymptotically
stable.
Example:
dxjdt
= -
x,
x E R. The
origin
is
uniformly
globally
asymptotically
stable.
Check
the
definitions.
Example.
Consider
a
planar
dynamical
system
defined
by
203
